
Mathematical exercises often present challenges that require a solid understanding of core principles. For students tackling these problems, having access to structured solutions can significantly enhance their comprehension. By focusing on step-by-step methods, learners can develop a clearer understanding of each process and improve their ability to solve complex problems independently.
In this section, we provide detailed explanations for a set of exercises that cover essential mathematical topics. Each solution is designed to break down the problem into manageable steps, ensuring that the process is easy to follow. By reviewing these solutions carefully, students can reinforce their knowledge and gain confidence in their problem-solving skills.
Understanding the methods behind each solution is key to grasping the underlying concepts. This approach ensures that learners are not just memorizing answers, but truly understanding the logic and strategies required to solve similar challenges in the future. Whether you are revisiting foundational concepts or advancing to more complex material, these solutions serve as a valuable resource for strengthening your mathematical abilities.
Pre Algebra with Pizzazz Answer Key Insights
In the world of mathematics, mastering the fundamentals is crucial for progressing to more complex topics. The provided solutions serve as a guide for students to grasp key concepts and develop effective problem-solving skills. By following a structured approach to solving problems, students can better understand the methodology and logic behind each step.
Understanding the Structure of Solutions
The solutions are designed to simplify the learning process, breaking down each task into clear, digestible steps. This method allows learners to focus on the logic rather than just the end result. Here are some important insights:
- Each problem is carefully dissected to highlight critical thinking techniques.
- Step-by-step explanations ensure that students are not just solving problems but also understanding the process.
- Common pitfalls are addressed, helping students avoid typical mistakes.
How This Helps Students Improve
By reviewing these detailed solutions, students are able to gain a deeper understanding of mathematical principles. The clarity of the methods used aids in retaining knowledge and applying it to future exercises. The following benefits are crucial for student growth:
- Enhances problem-solving abilities by fostering a logical approach.
- Builds confidence in tackling more difficult exercises.
- Promotes independent learning by showing how to break down complex tasks.
Utilizing this resource will help students not only solve current problems but also prepare them for more challenging material ahead. The insights provided here are essential for mastering foundational concepts that form the basis for more advanced mathematical studies.
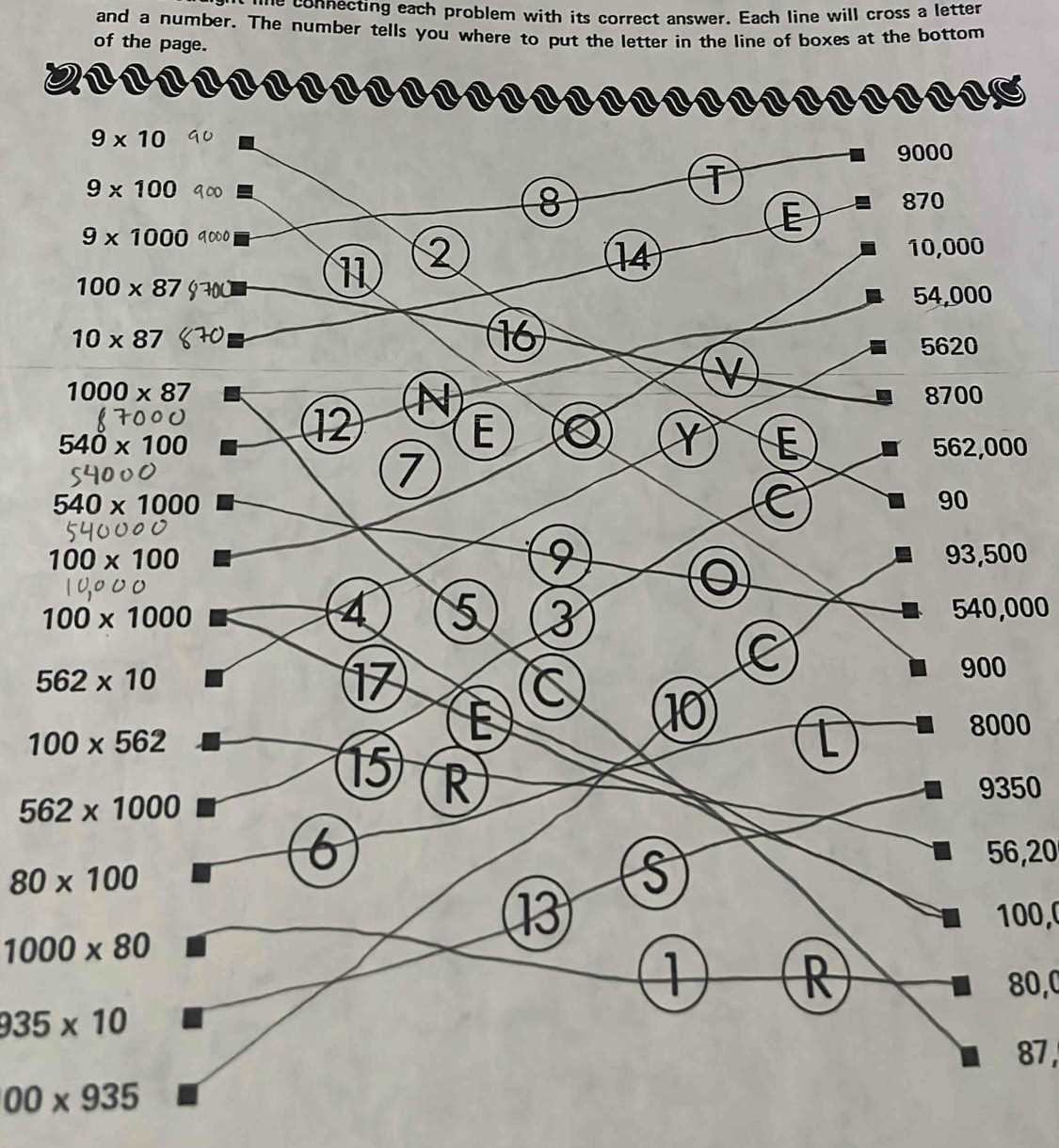
Understanding the Problem on Page 211
When approaching a new mathematical challenge, the first step is always understanding the problem itself. Before jumping into solving, it’s important to identify the key components and objectives of the exercise. In this section, we break down the given problem and explain the concepts involved to provide a clear pathway for tackling similar questions in the future.
Key Elements of the Exercise
The problem requires recognizing specific patterns and relationships between numbers, which are essential for finding the correct solution. Key components to focus on include:
- Identifying the variables involved and their roles.
- Understanding the operation required to manipulate these variables.
- Recognizing any underlying mathematical principles that guide the solution.
Approach and Strategy
Once the essential elements are understood, the next step is to determine an approach for solving the problem. This typically involves:
- Breaking down the problem into smaller, manageable steps.
- Applying known methods to simplify the equation or expression.
- Checking each step to ensure that the solution aligns with the given conditions.
By following this structured approach, students can ensure they are tackling the problem effectively, laying the groundwork for a deeper understanding of mathematical concepts. This methodology not only aids in solving the current task but also builds a strong foundation for more advanced problems.
How to Approach Mathematical Problems
Solving mathematical problems requires a systematic approach to break down complex tasks into manageable steps. Understanding the problem, planning your solution, and applying the right techniques are crucial for finding the correct answer. Whether the problem involves equations, expressions, or basic number manipulation, a clear strategy helps in developing confidence and accuracy.
The first step is always to carefully read and understand the problem. Identify what is being asked and the information provided. Pay attention to keywords that indicate the operations you need to perform, such as “sum,” “difference,” “product,” or “quotient.” Recognizing these cues ensures that you’re applying the correct methods from the start.
Next, organize your work. Write down the known values, variables, and relationships clearly. This helps to visualize the problem, which can lead to discovering patterns or insights that may not be obvious at first. It also minimizes the chances of errors during calculations.
Finally, solve the problem step-by-step. Break the task into smaller parts and focus on one step at a time. Ensure each step follows logically from the previous one, checking your work along the way. After arriving at a solution, always double-check the results to confirm that they meet the original conditions of the problem.
Step-by-Step Solutions for Math Exercises
When tackling mathematical challenges, following a methodical approach can make solving problems more manageable and effective. Breaking down each task into a series of logical steps helps to clarify the process, avoid errors, and build a stronger understanding of the concepts. Below, we outline a step-by-step guide to solving common mathematical problems, demonstrating how to handle each part of the exercise with precision and care.
Step 1: Understand the Problem
Before diving into any calculations, it’s important to fully understand the problem. Carefully read the given information, identify what is being asked, and note any values, variables, or constraints provided. This initial step sets the stage for a clear and focused solution process.
Step 2: Break Down the Problem
Once the problem is understood, break it down into smaller parts. This can be done by organizing the data into a table or list to make the steps more manageable. Below is an example of how a problem might be presented and simplified:
| Step | Description | Action |
|---|---|---|
| 1 | Identify the known values | Write down all given numbers and variables |
| 2 | Determine the required operation | Look for clues such as “sum,” “difference,” etc. |
| 3 | Perform the calculations | Apply the correct mathematical methods |
| 4 | Check your results | Review the solution to ensure accuracy |
By organizing the solution process in this way, it becomes easier to manage complex problems and ensure each step is completed correctly. This method helps avoid overlooking important details and ensures that the final result is accurate and well-supported by logical reasoning.
Common Mistakes in Math and How to Avoid Them
Mathematical exercises often present opportunities for mistakes, especially when concepts are being learned for the first time. These errors can stem from misunderstanding the problem, skipping steps, or misapplying methods. Recognizing common pitfalls and understanding how to avoid them is essential for building a solid foundation in math and improving overall problem-solving skills.
Overlooking Negative Signs
One of the most frequent errors in math problems is failing to properly account for negative signs. Whether subtracting numbers or working with negative variables, it’s easy to overlook these signs, leading to incorrect answers. To avoid this mistake, always double-check each step and carefully monitor the placement of negative signs. When working with equations or expressions, take extra care to observe how they affect the overall result.
Rushing Through the Steps
Another common mistake is rushing through the process without carefully following each step. Skipping over important details, like ensuring each calculation is correct before moving on, can result in errors that compound as the problem progresses. To avoid this, take your time with each part of the exercise. Write down every step, even if it seems obvious, and check your work before proceeding to the next stage. A slower, more deliberate approach leads to more accurate results.
By being mindful of these common mistakes and applying these strategies, students can significantly reduce the chance of errors and gain confidence in their problem-solving abilities. Practicing patience and attention to detail ensures a smoother learning experience and a better grasp of mathematical concepts.
Helpful Tips for Solving Mathematical Equations
Solving mathematical equations can be challenging, especially when dealing with multiple steps or unknown variables. However, with the right approach and strategies, even the most complex problems become more manageable. This section provides essential tips for effectively solving equations, helping you to improve both accuracy and speed while building a stronger understanding of the process.
Tip 1: Isolate the Variable
One of the key steps in solving an equation is isolating the variable on one side of the equation. This simplifies the problem and makes it easier to find the solution. Here’s how to approach it:
- Start by identifying the variable you are solving for.
- Perform inverse operations (addition, subtraction, multiplication, division) to move other terms away from the variable.
- Continue simplifying until the variable is isolated and you can easily solve for it.
Tip 2: Check Your Work
After solving the equation, always double-check your work. Mistakes can be subtle, and a quick review can help catch any errors. Here’s a checklist to follow:
- Substitute the solution back into the original equation to verify it holds true.
- Ensure all steps were followed correctly, especially when distributing or combining like terms.
- Check that all signs (positive/negative) were correctly accounted for throughout the process.
By applying these tips, you can solve equations more confidently and avoid common mistakes. Mastery of these methods will make tackling more complex problems easier and more intuitive over time.
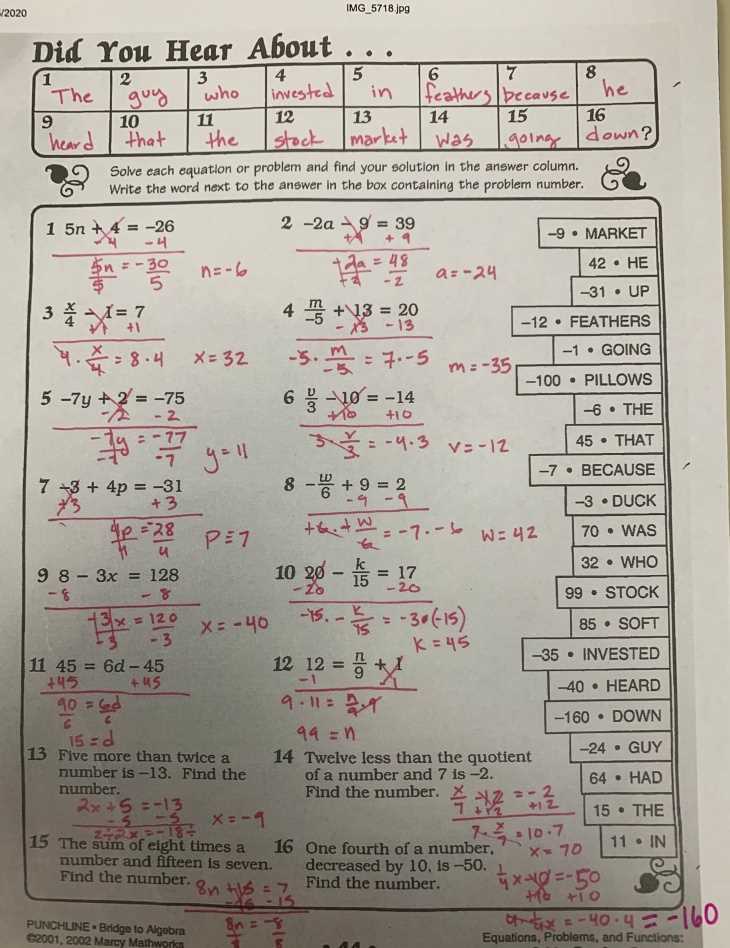
Key Concepts Covered in Page 211
In this section, we focus on the fundamental principles that serve as the foundation for solving various mathematical exercises. The concepts presented here are designed to strengthen your understanding and provide the tools necessary to tackle more complex challenges. By grasping these core ideas, you will be able to approach a wide range of problems with confidence and accuracy.
Understanding Variable Manipulation
One of the primary concepts introduced is how to work with variables. This involves recognizing how to isolate unknowns within an equation and use basic operations like addition, subtraction, multiplication, and division to simplify and solve for these variables. Mastery of variable manipulation is essential for progressing in mathematics and is applicable across a variety of problems.
Applying Order of Operations
Another crucial concept is the order in which mathematical operations should be performed. The correct sequence–often remembered by the acronym PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, and Subtraction)–ensures that calculations are done in the proper order to achieve accurate results. Understanding this principle helps prevent errors and ensures consistency in solving more advanced exercises.
These foundational concepts are vital for developing strong problem-solving skills. With practice, students can enhance their ability to manipulate expressions and equations effectively, leading to greater success in future mathematical endeavors.
Improving Math Skills with Fun and Engagement
Enhancing your mathematical abilities doesn’t have to be a tedious task. By making the learning process engaging and enjoyable, you can boost both your understanding and retention of key concepts. This section explores effective methods for improving your skills while keeping the experience fun and interactive, which helps solidify your knowledge for the long term.
Practice Through Interactive Exercises
One of the most effective ways to improve math skills is by engaging with interactive problems. These exercises allow you to apply the concepts you’ve learned in a hands-on way, reinforcing your understanding. Whether it involves solving equations, manipulating expressions, or applying formulas, interactive exercises provide immediate feedback that helps you learn from mistakes and track your progress.
Incorporating Creativity into Problem Solving
Another helpful approach is to add a creative element to the problem-solving process. This can include visualizing problems, creating drawings or diagrams to represent equations, or coming up with real-world examples that relate to the concepts you’re learning. By making math feel more relevant and fun, you create a deeper connection to the material, which leads to better comprehension and greater enjoyment of the subject.
By adopting these strategies, you’ll not only improve your math skills but also develop a more positive and enthusiastic approach to learning. With consistent practice and a creative mindset, complex problems will become easier to solve and more enjoyable to work through.
Breaking Down Complex Math Problems
Mathematical problems can sometimes appear overwhelming, especially when they involve multiple steps or operations. However, by breaking them down into smaller, more manageable parts, solving them becomes much easier. This section will guide you through the process of dissecting complicated problems, allowing you to approach them in a structured and logical way, step by step.
Step 1: Identify the Given Information
The first step in tackling any complex problem is identifying all the relevant information provided. This includes constants, variables, and any other details mentioned in the problem. Organizing this information clearly helps in understanding the relationships between different elements and guides the next steps of solving.
| Step | Action |
|---|---|
| 1 | Identify known values or terms in the problem. |
| 2 | Highlight the unknown values or variables to solve for. |
Step 2: Break Down the Operations
Next, identify the operations you need to apply, such as addition, subtraction, multiplication, or division. Sometimes, more advanced techniques like factoring or distributing terms may be necessary. Breaking the problem into smaller operations allows for a more methodical approach, ensuring you don’t miss any crucial steps along the way.
By following these two key steps–identifying the knowns and unknowns, and breaking down the operations–you can approach even the most complex problems with confidence and clarity. This process not only makes problems more approachable but also builds your problem-solving skills for future challenges.
Using Visual Aids to Understand Math
Incorporating visual tools into learning can significantly improve understanding, especially when tackling abstract mathematical concepts. Visual aids such as graphs, charts, diagrams, and number lines allow students to visualize relationships between variables and operations, making complex problems more accessible. These tools help transform abstract ideas into something tangible, which can enhance both comprehension and retention.
For example, drawing out a problem on a number line can help clarify the relationships between numbers, while graphs can provide a clear picture of how variables change in relation to each other. Visualizing equations or expressions in this way can also assist in understanding operations like addition, subtraction, multiplication, and division, as well as how they interact with each other.
By leveraging visual aids, learners can develop a deeper understanding of mathematical principles and gain more confidence in solving problems. This approach not only makes learning more engaging but also provides a structured way to break down complex concepts into simpler, more manageable parts.
Practice Problems for Math Mastery
To master any mathematical concept, consistent practice is essential. By regularly solving problems, you reinforce your understanding and improve your problem-solving skills. This section provides a variety of exercises designed to help you hone your skills and gain confidence in applying different techniques. Whether you are focusing on basic operations or more complex problem-solving strategies, these problems will offer the opportunity to apply what you’ve learned.
Start with simpler problems to build your foundation, and gradually work towards more challenging ones. Each problem is an opportunity to develop critical thinking and sharpen your ability to recognize patterns, manipulate variables, and apply the right methods for solving. With practice, you’ll improve both your speed and accuracy.
By practicing regularly and staying persistent, you will master the concepts at hand, enhancing your overall mathematical ability and preparing yourself for more advanced topics.
How to Check Your Mathematical Solutions
Verifying your solutions is an essential step in the problem-solving process. Ensuring that your results are correct not only boosts your confidence but also helps identify any mistakes that may have been made during the process. This section explores different methods you can use to check your work and confirm that your solutions are accurate.
One common approach is to substitute your solution back into the original problem to see if it satisfies all conditions. If the equation holds true when you replace the variable with your answer, then the solution is likely correct. Another useful method is to double-check each step of your work to ensure that you haven’t skipped any important operations or made any calculation errors.
In addition to these methods, using alternative techniques or tools like graphing calculators or online solvers can provide a quick way to verify your results. By practicing these verification techniques, you can become more proficient in catching errors early and mastering problem-solving strategies.
Reviewing Mathematical Fundamentals
Strengthening your foundation is key to advancing in mathematics. Understanding the basic principles allows you to tackle more complex problems with ease. This section focuses on revisiting core concepts that form the building blocks of more advanced topics. A solid grasp of these fundamentals is essential for progressing confidently and effectively in mathematical studies.
Key Concepts to Review:
- Basic arithmetic operations (addition, subtraction, multiplication, division)
- Understanding variables and expressions
- Solving linear equations and inequalities
- Identifying patterns and relationships between numbers
- Mastering fractions, decimals, and percentages
Focusing on these foundational topics helps ensure that you are equipped to handle more challenging material. Regular practice and review are essential for reinforcing these concepts and building your problem-solving abilities. By revisiting these core ideas, you set yourself up for success in future learning and applications.
Why Solutions Are Essential
Having access to correct solutions plays a critical role in mastering any mathematical topic. When learning complex concepts, it’s important to have a reliable reference to verify your work and ensure that your approach aligns with the correct methods. This section discusses the value of having solutions available, as they offer clarity and guide you through common challenges in problem-solving.
Clarity and Confidence: Solutions serve as a benchmark, providing insight into how to structure and solve problems effectively. They help reinforce the learning process by showing step-by-step procedures and highlighting the logical flow behind each step. By reviewing these solutions, students can gain confidence in their ability to tackle similar problems independently.
Error Identification: Sometimes mistakes go unnoticed during the solving process. Solutions give learners an opportunity to pinpoint where errors may have occurred, whether in calculation or method. By understanding where things went wrong, students can correct their understanding and avoid similar pitfalls in the future.
Overall, solutions offer invaluable support in refining your skills and improving your understanding. They provide not just answers, but a deeper insight into the process of solving mathematical problems.
Boosting Confidence in Problem Solving
Mastering complex mathematical challenges can feel overwhelming, especially when encountering unfamiliar problems. However, building confidence in solving these tasks is crucial for long-term success. With the right approach, students can transform uncertainty into clarity and strengthen their problem-solving abilities.
Breaking Down Problems Step by Step
One effective way to build confidence is to break down each problem into manageable steps. Tackling smaller, individual components helps prevent feeling overwhelmed by the complexity of a problem. By following a systematic approach, students can focus on one step at a time, making the overall process seem more achievable.
Practicing Consistently for Improvement
Regular practice plays a significant role in reinforcing understanding and boosting confidence. The more you practice solving problems, the more familiar the concepts will become. By repeatedly applying problem-solving strategies, learners not only improve their skills but also gain the assurance needed to tackle more challenging problems with ease.
In addition, reviewing and analyzing solutions to previous problems is an excellent way to reinforce learning. This allows students to see the connections between concepts and methods, building both competence and confidence over time. By embracing this iterative process, students will gradually develop a strong foundation for future problem-solving challenges.
Using the Solution Guide Effectively
Having access to a solution guide can be a valuable resource when working through mathematical exercises. However, using this tool effectively requires a thoughtful approach to ensure that it supports learning rather than simply providing quick fixes. Proper utilization of a solution guide can help strengthen understanding and build problem-solving skills.
Step-by-Step Review
Instead of jumping straight to the solutions, it’s important to attempt solving each problem independently first. Once you’ve made an attempt, refer to the guide to check your work. This review process allows you to identify where you may have gone wrong and offers insight into the correct approach.
- Attempt the problem: Work through the steps on your own, applying your knowledge and methods.
- Check the solution: Compare your method to the provided solution, noting any differences.
- Understand the reasoning: Focus on how the solution is derived to see if there are any strategies or shortcuts you missed.
Avoid Relying Too Heavily on the Guide
While the solution guide can be an excellent tool, relying on it too often can undermine your learning. The goal is to use it as a reference, not as a crutch. By actively engaging with each problem and only using the guide for clarification or confirmation, you can build more confidence in your abilities and retain the knowledge better.
Additionally, regularly reviewing the problem-solving methods in the guide can help reinforce concepts and improve your critical thinking skills, making you better prepared for more complex challenges in the future.