
In this section, we explore various solutions and methods for tackling the exercises and challenges presented in a key learning area. The aim is to provide a clear understanding of the concepts while guiding through the necessary steps for accurate results. Mastering these techniques is essential for improving problem-solving abilities and building a strong foundation for more complex topics in the future.
By breaking down the provided exercises and offering detailed explanations, this resource serves as a valuable tool for students aiming to enhance their skills. Each solution is carefully structured to demonstrate logical approaches, helping learners understand the reasoning behind each step and ensuring a deeper grasp of the subject matter.
Whether revisiting foundational concepts or exploring new techniques, this guide is designed to assist learners at different levels, offering both clarity and practice. The provided solutions not only ensure correct results but also encourage critical thinking and better comprehension of core principles.
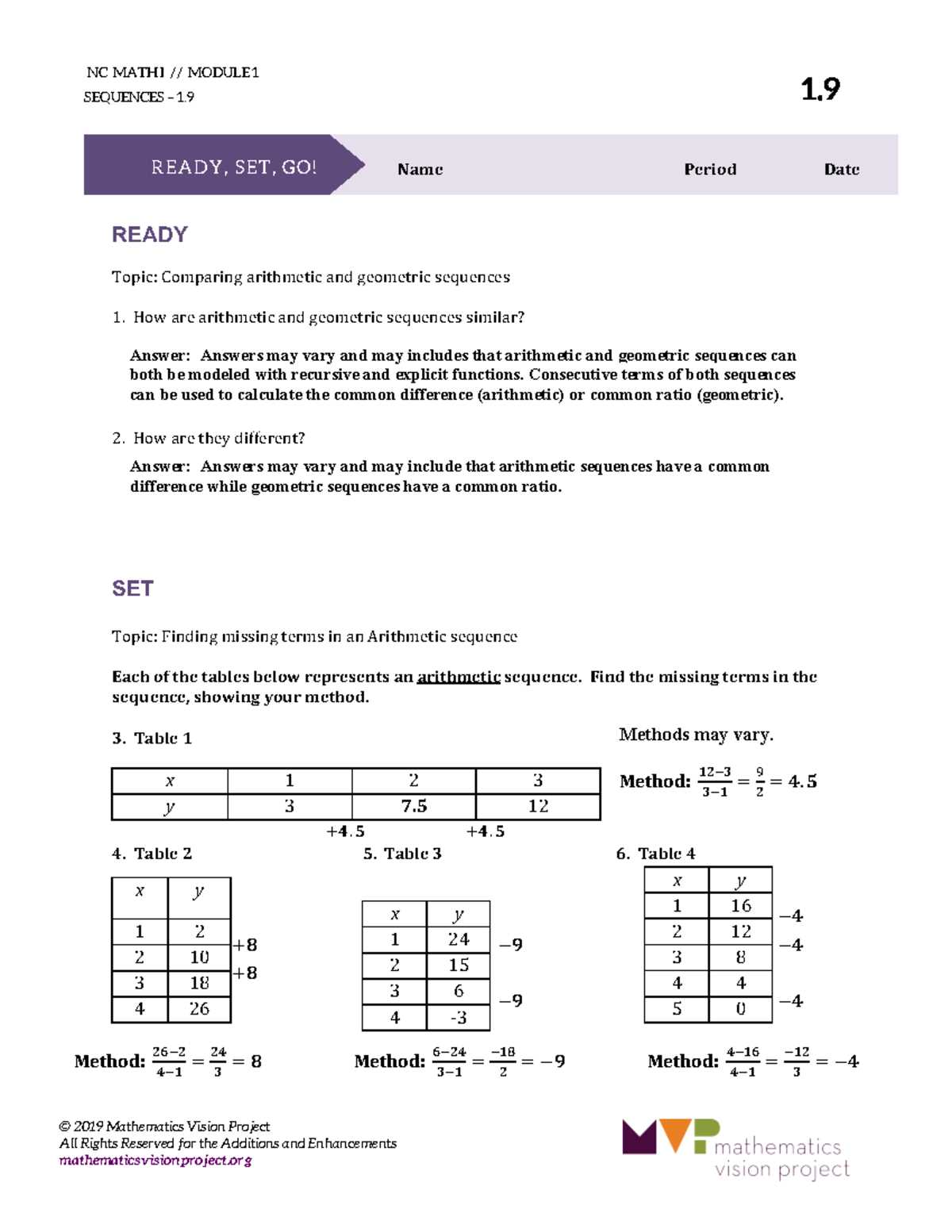
Secondary Math 1 Module 4 Answer Key
This section provides a comprehensive guide to understanding the solutions for various problems found within this educational unit. By walking through each question, students can grasp the reasoning and methodologies used to arrive at correct solutions. This resource aims to help learners build confidence and enhance their problem-solving skills through structured examples and explanations.
Below, you’ll find a detailed breakdown of the approach to solving each problem, including important tips and techniques to keep in mind. Reviewing these explanations will help reinforce key concepts and improve your overall comprehension of the material.
- Understand the core principles behind each problem.
- Review step-by-step solutions to ensure clarity of thought.
- Apply the methods to similar problems for further practice.
- Focus on common areas where mistakes tend to happen.
- Use the provided examples to help recognize patterns in problems.
For each exercise, we cover the critical concepts, offering detailed steps that lead to the correct outcome. Whether you are revisiting the basics or facing more advanced challenges, these solutions are crafted to support learners at different stages of their educational journey.
- First, identify the main elements of the problem.
- Next, break it down into smaller, manageable steps.
- Carefully apply the appropriate formulas or strategies.
- Finally, review the result and ensure all conditions are met.
By practicing regularly with these solutions, students can improve their accuracy and speed in solving similar exercises, making them better prepared for more advanced topics ahead.
Overview of Secondary Math 1 Module 4
This section covers the essential concepts and techniques introduced in this learning unit. The focus is on strengthening problem-solving skills and applying different strategies to tackle a variety of challenges. It is designed to provide students with a deeper understanding of fundamental principles that are critical for advancing to more complex topics.
Main Objectives of the Unit
- Introduce core strategies for solving equations and inequalities.
- Enhance skills in analyzing mathematical relationships and patterns.
- Build a strong foundation for approaching future mathematical concepts.
- Encourage critical thinking and logical reasoning in problem-solving.
Key Topics Covered
- Linear equations and their solutions.
- Graphical representation of functions.
- Solving word problems through algebraic expressions.
- Applying mathematical concepts to real-world situations.
- Understanding and manipulating ratios and proportions.
This unit offers a balanced approach to understanding both theoretical concepts and practical applications. By working through these topics, students will gain a more comprehensive grasp of the material, preparing them for future learning challenges.
Understanding Key Concepts in Module 4
This section delves into the core principles that underpin the exercises and problems in this learning segment. By focusing on these concepts, students can develop a stronger grasp of the material and approach problems with greater confidence. Understanding the fundamental ideas is essential for progressing to more advanced topics and ensuring mastery of the subject.
Linear Relationships play a central role in this unit, as they help students recognize how variables interact with each other. Mastery of these relationships is critical for solving equations and graphing functions accurately.
Graphical Representations provide visual insights into mathematical problems, allowing learners to interpret data and functions in a more intuitive way. These representations not only make complex topics easier to understand but also build problem-solving skills applicable to real-life scenarios.
By focusing on the relationships between variables and practicing with different types of problems, students can enhance both their theoretical knowledge and practical application of these concepts.
How to Use the Answer Key Effectively
Utilizing a solution guide properly can significantly enhance your learning experience. It’s not just about checking your work but understanding the reasoning behind each step. By approaching the provided solutions strategically, you can reinforce your understanding and improve problem-solving skills for future challenges.
- Review the Solutions Carefully – Read through each step to ensure you understand the logic and method used to reach the result.
- Identify Key Concepts – Take note of the principles or formulas applied in each solution to build a stronger foundation.
- Analyze Mistakes – If you made an error, focus on where you went wrong and try to understand why the correct approach works better.
- Apply Similar Problems – After reviewing the solutions, attempt similar exercises without checking the guide immediately to test your comprehension.
- Use as a Learning Tool – Rather than just copying the answers, use the guide to enhance your understanding of the process behind each solution.
By following these steps, you can transform the solution guide from a simple reference into a powerful tool for mastering key topics and improving overall skills.
Common Mistakes in Module 4 Solutions
Even the most experienced learners can make errors when working through mathematical problems. Understanding the common pitfalls in this unit can help students avoid frustration and improve accuracy. By identifying these mistakes, learners can refine their problem-solving techniques and build a stronger foundation for future topics.
- Misinterpreting Problem Statements – It’s easy to overlook key details in the wording of a problem. Always take time to carefully read the question and highlight important information before proceeding.
- Incorrect Application of Formulas – A common mistake is misapplying formulas, especially when they involve multiple steps. Ensure that you understand the formula’s context and components before using it.
- Sign Errors in Equations – When working with equations, sign mistakes (positive vs. negative) are frequent. Double-check your work to avoid incorrect results that stem from simple sign errors.
- Skipping Steps – Rushing through the solution process can lead to skipped steps or overlooked details. Always write out each step methodically to avoid careless mistakes.
- Confusing Operations – When dealing with operations like multiplication and division, it’s easy to confuse which operation to apply first. Pay attention to the order of operations to avoid errors in solving the problem.
- Failure to Check Results – Many students fail to check their work, which can lead to unnoticed mistakes. Always take a moment to review your final answer and verify that it makes sense in the context of the problem.
By recognizing and addressing these common mistakes, students can improve their problem-solving efficiency and reduce the likelihood of errors in future exercises.
Step-by-Step Problem Solving Techniques
Effective problem-solving involves breaking down complex tasks into manageable steps. By following a structured approach, students can work through challenges more systematically, ensuring they address each part of the problem thoroughly. This method encourages clarity and helps avoid errors that may occur when trying to solve problems hastily.
1. Understand the Problem
The first step in solving any problem is to fully comprehend what is being asked. Read the problem carefully and identify key information. Highlight any values, relationships, or instructions that are essential to finding the solution.
- Identify the unknowns in the problem.
- Clarify what the problem is asking you to find.
- Look for any relevant formulas or concepts that can help solve the problem.
2. Plan Your Approach
Once the problem is clear, plan your next steps. This involves choosing the appropriate methods or strategies to apply. Depending on the nature of the problem, you may need to use algebraic manipulation, logical reasoning, or geometric principles.
- Determine the formulas or methods that might apply.
- Decide whether to solve the problem algebraically or visually (e.g., graphing).
- Write down any steps you plan to take to avoid skipping important calculations.
By approaching each problem in a methodical, step-by-step manner, you not only increase your chances of finding the correct solution but also develop strong problem-solving habits that will help in more complex topics.
Tips for Mastering Module 4 Topics
Mastering the concepts in this section requires consistent practice and a deep understanding of the principles involved. By focusing on key techniques and actively engaging with the material, students can build confidence and improve their problem-solving skills. The following tips are designed to help learners succeed in mastering the core topics covered in this unit.
| Tip | Explanation |
|---|---|
| Practice Regularly | Frequent practice helps reinforce the material and improves retention. Solve problems daily to build familiarity with the concepts. |
| Break Down Complex Problems | Start by identifying the main elements of a problem. Break it into smaller steps to make it easier to solve and understand. |
| Review Mistakes | When you make a mistake, take the time to understand why it happened. Reviewing errors can help prevent them in the future. |
| Use Visual Aids | Drawing graphs, diagrams, or tables can help visualize the problem and make abstract concepts clearer. |
| Seek Help When Needed | If you’re stuck, don’t hesitate to ask for help. Discussing problems with peers or instructors can provide new insights. |
By following these strategies, students can enhance their ability to tackle problems with greater accuracy and efficiency, paving the way for success in the unit’s topics and beyond.
Alternative Methods for Solving Problems
When faced with a challenging problem, it’s essential to consider multiple approaches to find the most efficient solution. Different strategies can offer new perspectives and help you tackle the problem from various angles. By expanding your toolkit of problem-solving methods, you can enhance your flexibility and creativity in addressing different scenarios.
1. Trial and Error
Trial and error involves making an educated guess and refining your approach based on the outcome. This method is particularly useful when you’re unsure of the exact path to take but have a general understanding of the problem.
- Start by testing a possible solution or approach.
- Evaluate the result and adjust accordingly.
- Repeat the process until you find a satisfactory outcome.
2. Graphical Methods
Graphical methods involve representing the problem visually through graphs or diagrams. This approach is helpful when dealing with functions, equations, or relationships between variables.
- Plot the key points or equations on a graph.
- Look for patterns or intersections that suggest the solution.
- Use the graph to identify solutions or check your work.
Both trial and error and graphical methods allow you to experiment and see the problem in different ways, which can lead to a deeper understanding of the material and more effective solutions.
Practical Applications of Module 4 Content
The concepts covered in this section have numerous real-world applications that extend beyond the classroom. By understanding how to apply these principles in everyday situations, students can see the value of what they are learning and recognize how mathematics plays a vital role in various fields. The following table highlights some practical uses of the skills acquired in this unit.
| Application | Description |
|---|---|
| Financial Planning | Understanding proportions, percentages, and ratios is crucial for budgeting, saving, and managing finances in daily life. |
| Engineering and Design | Algebraic concepts help engineers and architects calculate dimensions, angles, and materials needed for construction and product design. |
| Computer Science | Many algorithms in computer programming rely on logical thinking and problem-solving techniques taught in this unit. |
| Cooking and Recipes | Proportions and measurements are used to scale recipes up or down, ensuring proper ingredient ratios for various servings. |
| Sports and Statistics | Understanding averages, percentages, and performance analysis is essential in sports to track progress, improve skills, and strategize. |
By connecting the abstract concepts of this section to real-life scenarios, students can gain a deeper appreciation for the practical value of these skills, motivating them to engage with the material more actively.
How to Check Your Answers
Verifying the accuracy of your solutions is a crucial part of the problem-solving process. Checking your work not only helps ensure correctness but also reinforces your understanding of the material. By systematically reviewing each step and considering alternative methods, you can identify any mistakes and strengthen your problem-solving skills.
1. Review Each Step
Carefully go through the steps you took to arrive at your solution. Often, errors occur during the calculation or in the application of formulas. Re-check each step to ensure that the logic and arithmetic are sound.
- Verify that you used the correct formula or method for the problem.
- Double-check the arithmetic operations to ensure no mistakes in addition, subtraction, multiplication, or division.
- Confirm that you interpreted the problem correctly and didn’t overlook any important details.
2. Use a Different Approach

If possible, solve the problem using an alternative method to cross-check your result. By applying a different technique or strategy, you can compare results and see if they match, which helps verify the accuracy of your solution.
- If you solved algebraically, try graphing the solution to see if it aligns with your answer.
- Alternatively, check your result by plugging it back into the original problem to ensure consistency.
- If applicable, use estimation techniques to quickly determine if your solution is reasonable.
By following these steps, you can be more confident in the accuracy of your solutions and improve your problem-solving process over time.
Exploring Sample Problems and Solutions
Working through examples is an effective way to understand the core concepts and enhance your problem-solving skills. By examining sample problems, you can see how to apply theoretical knowledge to practical situations, reinforcing key ideas and boosting confidence in your ability to solve similar challenges. This section covers a range of example problems with detailed solutions to guide you through the process.
Sample Problem 1: Solving Linear Equations
Let’s solve a basic linear equation step-by-step to illustrate the process:
- Start with the equation: 2x + 5 = 15.
- Subtract 5 from both sides: 2x = 10.
- Divide both sides by 2: x = 5.
- Check your work by substituting x = 5 back into the original equation: 2(5) + 5 = 15, which is true.
Sample Problem 2: Working with Ratios
Next, let’s explore a problem involving ratios:
- Problem: The ratio of apples to oranges is 3:4. If there are 12 apples, how many oranges are there?
- Set up the proportion: 3/4 = 12/x.
- Cross-multiply: 3x = 48.
- Divide both sides by 3: x = 16.
- Check the solution: The ratio of apples to oranges is 12/16 = 3/4, which is correct.
By working through these examples, you gain a better understanding of the steps involved in solving different types of problems. Regular practice with such problems can greatly improve your ability to apply these techniques in various scenarios.
Key Formulas to Remember in Module 4
Having a strong grasp of essential formulas is fundamental to successfully solving problems and mastering the content. These formulas serve as tools that help simplify complex concepts and enable you to efficiently tackle a wide range of challenges. Below are some important formulas to keep in mind as you progress through the material.
Basic Algebraic Formulas
These formulas are crucial for solving linear equations and inequalities:
| Formula | Purpose |
|---|---|
| y = mx + b | Equation of a line, where m is the slope and b is the y-intercept. |
| ax + b = 0 | Standard form of a linear equation, useful for solving one-variable equations. |
| x = -b/2a | Formula for finding the x-coordinate of the vertex of a quadratic equation ax² + bx + c = 0. |
Geometry Formulas
Understanding the following formulas will assist with solving geometry-related problems:
| Formula | Purpose |
|---|---|
| Area = πr² | Formula for the area of a circle, where r is the radius. |
| Perimeter = 2πr | Formula for the perimeter (circumference) of a circle. |
| Area = ½bh | Formula for the area of a triangle, where b is the base and h is the height. |
By memorizing and understanding these formulas, you will have the tools necessary to approach and solve a variety of problems with confidence. Keep practicing these formulas and apply them in different contexts to enhance your problem-solving skills.
Strategies for Improving Math Skills
Developing strong problem-solving abilities and mathematical reasoning is essential for success in any related subject area. Whether you’re preparing for assessments or aiming to deepen your understanding, certain strategies can help improve your skills and build confidence. The following techniques will guide you in enhancing your overall performance in various types of calculations and conceptual tasks.
Practice Consistently
Regular practice is crucial for mastering any subject. Repetition allows you to internalize concepts and identify patterns that make problem-solving easier. Here are some tips for effective practice:
- Work on a variety of problems: By solving different types of questions, you gain a broader understanding and can adapt to various problem formats.
- Set aside dedicated study time: Consistency is key. Scheduling regular practice sessions helps reinforce what you’ve learned.
- Review mistakes: Analyze your errors and identify where you went wrong. This reflective practice can help prevent similar mistakes in the future.
Understand the Concepts Behind Procedures
Instead of just memorizing formulas and steps, focus on understanding why each method works. This deeper comprehension allows for more flexible thinking when tackling complex problems. To achieve this:
- Ask why: After learning a new method, ask yourself why it works. Understanding the reasoning behind each procedure strengthens your problem-solving foundation.
- Connect concepts: Relate new material to previously learned topics. Building a conceptual network helps in retaining information and recognizing connections between ideas.
- Visualize problems: Drawing diagrams or using visual aids can help you better understand abstract concepts.
By incorporating these strategies into your routine, you will gradually improve your ability to solve problems accurately and efficiently, ultimately boosting your mathematical skills and confidence.
Breaking Down Complex Problems
Tackling complicated questions can seem daunting, but breaking them into smaller, more manageable steps makes them easier to solve. By dividing a complex problem into individual components, you can focus on solving each part one at a time, leading to a more organized and efficient approach. This technique helps reduce errors and increases your confidence when facing difficult challenges.
Step 1: Identify the Core Problem
The first step in breaking down a complex task is to clearly define the core issue. Often, problems can seem overwhelming because they appear too broad or vague. Start by pinpointing the central question or the main objective you need to solve. Once identified, you can focus your efforts on resolving that key aspect.
- Clarify the objective: Understand exactly what the problem is asking you to find or calculate.
- Highlight key information: Extract relevant details or data that will help you solve the problem.
- Ignore distractions: Stay focused on the main goal and avoid overcomplicating the situation with unnecessary details.
Step 2: Break it into Smaller Tasks
After identifying the main objective, the next step is to decompose the problem into smaller, more manageable tasks. Each smaller part should be solvable on its own, helping you build a solution piece by piece. Here are some techniques for breaking down tasks:
- Identify related sub-problems: Look for logical steps that lead towards the overall solution. These might be smaller calculations or processes that need to be completed first.
- Group similar components: If the problem involves multiple steps, group similar tasks together. For instance, if the problem requires multiple calculations, do them sequentially rather than simultaneously.
- Work incrementally: Complete one part of the problem before moving to the next. This allows you to manage your time and effort more effectively.
By following these steps and simplifying each element of the problem, you will gradually make progress towards a solution, avoiding feelings of frustration and improving your overall problem-solving skills.
Identifying Patterns in Module 4 Answers
Recognizing recurring themes and sequences is crucial for understanding the underlying structure of any set of problems. By observing these patterns, learners can predict solutions and develop strategies that enhance problem-solving skills. In this section, we explore how identifying consistent trends can simplify complex tasks and make the process of finding solutions more efficient.
One of the key elements in uncovering these patterns is looking at the progression of numbers or operations. Often, a specific approach or method can be applied repeatedly across different problems, yielding similar results. By carefully analyzing each step and the relationships between components, it becomes easier to spot these patterns and use them to your advantage in future challenges.
Through repeated practice and careful examination, learners develop the ability to recognize these trends intuitively. This skill is not only useful for solving problems quickly but also helps in building a deeper understanding of the material, laying a strong foundation for more advanced concepts.
Additional Resources for Further Learning
To deepen your understanding and enhance your skills, a variety of resources are available that offer additional practice and insights. These tools can guide you through more complex concepts, providing alternative explanations and examples that might not be covered in the standard materials. Exploring different sources allows for a more comprehensive grasp of the subject.
Online platforms offer interactive exercises, step-by-step tutorials, and video lessons, making learning engaging and accessible. Websites such as Khan Academy and Coursera provide structured courses that complement traditional study methods. These platforms break down challenging topics into digestible parts, allowing learners to progress at their own pace.
Books and workbooks can also serve as excellent references. Many textbooks offer practice questions with detailed solutions that help reinforce concepts. Moreover, visiting educational blogs or forums can expose you to different perspectives and problem-solving techniques that may be beneficial when facing difficulties.
Final Tips for Success in Math
Achieving success in any subject requires dedication, practice, and the right strategies. The following tips will help you build a strong foundation and enhance your ability to tackle challenging problems with confidence.
- Practice regularly: Consistent practice is essential to mastering any skill. Solve problems every day to improve your understanding and speed.
- Review mistakes: When you make an error, take the time to understand why it happened. Correcting mistakes is one of the best ways to learn and avoid similar issues in the future.
- Stay organized: Keep your notes and materials well-organized. A tidy workspace can help you focus better and find information more easily.
- Ask for help: Don’t hesitate to seek assistance when you’re stuck. Whether it’s from a teacher, tutor, or online resource, getting clarification can save you time and frustration.
- Stay positive: Maintain a positive attitude even when things seem difficult. Patience and perseverance are key to overcoming challenges.
By following these tips and staying committed to improvement, you can build confidence and achieve long-term success in your studies.