
Understanding fundamental concepts in higher mathematics is essential for success in various assessments. This section will guide you through some of the most important ideas, helping you to build a strong foundation. By focusing on core principles, you’ll be able to approach more complex problems with confidence and clarity.
Effective preparation for mathematical challenges involves recognizing patterns, grasping critical theories, and practicing problem-solving strategies. Through this process, you’ll not only enhance your theoretical knowledge but also improve your practical application skills.
In this article, we’ll explore key ideas that are central to mastering the core concepts. Whether you’re preparing for a standardized test or aiming to strengthen your mathematical abilities, this guide will provide clear explanations and practical examples to assist you in your studies.
AP Calculus AB Exam Overview
This assessment is designed to test your understanding of advanced mathematical concepts. It focuses on your ability to solve problems involving functions, derivatives, integrals, and related topics. The goal is to evaluate not only your theoretical knowledge but also your ability to apply these concepts in practical situations.
Structure of the Assessment
The test consists of multiple sections that assess both your conceptual understanding and problem-solving skills. You’ll be asked to work through a variety of questions, ranging from basic to more complex, and demonstrate your ability to apply learned principles effectively. Time management is crucial as the questions are designed to challenge your quick thinking and accuracy.
Key Areas of Focus
In preparation, it’s important to review the primary areas of the curriculum, such as function behavior, rate of change, and accumulation of quantities. These topics form the foundation for the majority of the questions you will encounter. Additionally, being familiar with the different types of problems that may appear on the assessment can greatly enhance your ability to respond quickly and accurately.
Understanding Limits in Mathematics
In advanced mathematics, understanding how functions behave as they approach certain values is crucial. These concepts help to describe the behavior of equations when direct evaluation isn’t possible. By studying how a function behaves near a specific point, you can gain valuable insights into its overall structure and trends.
One of the core ideas is to explore the behavior of a function as it nears a particular input. This allows you to understand its tendency or value without needing to evaluate it directly. Whether approaching a finite value or infinity, these principles help solve problems related to rates of change, behavior at boundaries, and more.
Grasping this concept is essential for solving many higher-level problems, as it forms the foundation for other important topics. By recognizing how different functions approach certain values, you can make more accurate predictions and better understand their behavior in various contexts.
Continuity and Its Importance in Mathematics
In advanced mathematical study, understanding the smoothness of a function is essential. This concept refers to whether a function behaves without abrupt changes, jumps, or breaks as its input varies. It plays a key role in analyzing real-world problems, as many phenomena in physics, economics, and engineering rely on smooth transitions between states or values.
A function is considered well-behaved if its graph can be drawn without lifting the pencil, meaning that it doesn’t experience any sudden jumps or interruptions. This property ensures that calculations involving rates of change, integration, and other operations are valid and reliable. In practical terms, it allows mathematicians and scientists to model natural processes that evolve smoothly over time or space.
Understanding this concept is crucial when working with equations that describe continuous processes, as it ensures the validity of various operations performed on the function. Whether evaluating physical systems or solving optimization problems, knowing when a function remains uninterrupted enables more accurate predictions and analyses.
Key Theorems for Limits and Continuity
In mathematics, several fundamental theorems provide a foundation for understanding how functions behave in various contexts. These key principles allow mathematicians to predict the behavior of functions and justify the results obtained from them. Mastery of these theorems is essential for solving complex problems involving smooth transitions and precise calculations.
Some of the most important theorems include:
- The Squeeze Theorem: This theorem helps determine the value of a function by comparing it to two other functions that are easier to evaluate.
- Intermediate Value Theorem: It asserts that if a continuous function takes opposite signs at two points, then there must be at least one point where the function equals zero between them.
- Horizontal and Vertical Asymptotes: These theorems explain the behavior of functions as they approach infinity or a specific point, providing valuable insights into their long-term behavior.
- Continuous Function Theorem: It ensures that a function with no breaks or jumps will allow for the application of key operations like differentiation and integration over intervals.
These theorems serve as powerful tools in both theoretical studies and practical applications. Understanding how to apply them correctly can simplify problem-solving processes and lead to more accurate solutions across various fields.
How to Evaluate Limits Effectively
Evaluating the behavior of a function as it approaches a particular value is a critical skill in higher mathematics. This process involves determining the function’s trend near a specific point, which is essential for understanding how it behaves under certain conditions. By applying systematic methods, you can often solve these problems quickly and accurately.
To evaluate these expressions, the following strategies are often useful:
- Direct Substitution: Start by substituting the value directly into the function. If it results in a valid number, this is typically the answer.
- Simplification: If direct substitution leads to an indeterminate form, simplify the expression by factoring or using algebraic techniques to cancel out common terms.
- Rationalizing: In cases where the function involves square roots, rationalize the numerator or denominator to remove radicals and simplify the expression.
- Use of Special Theorems: Apply established theorems, such as the Squeeze Theorem, when direct evaluation is challenging, to estimate the value of the function effectively.
By mastering these techniques, you can evaluate functions efficiently and solve complex problems with confidence. Practicing various types of problems will help you develop a strong intuition for identifying the most effective approach in each situation.
Continuity at Specific Points Explained
Understanding how a function behaves at a particular point is essential for identifying whether it follows a smooth path without interruptions. This concept ensures that there are no sudden jumps or breaks in the graph at certain inputs. A function is considered continuous at a point if it satisfies several key criteria, which are fundamental to solving many mathematical problems.
Conditions for Continuity at a Point
For a function to be continuous at a given point, it must meet the following conditions:
| Condition | Description |
|---|---|
| Defined at the Point | The function must have a value at that specific point. |
| Limit Exists | The limit of the function must exist as the input approaches the point. |
| Equality of Limit and Value | The value of the function at the point must equal the limit as the input approaches it. |
Identifying Discontinuities
Discontinuities occur when any of these conditions are not met. Common examples include holes, jumps, or vertical asymptotes in the graph of a function. Recognizing these irregularities helps in understanding where the function may behave erratically or fail to be well-defined.
Limit Laws and Their Applications
In advanced mathematics, certain rules govern the behavior of functions as they approach specific values. These fundamental principles allow you to simplify complex expressions and solve problems efficiently. By applying these laws, you can predict the behavior of functions in various situations, making them essential tools for analyzing mathematical models.
Some of the key laws include:
- Sum Law: The limit of the sum of two functions is the sum of their individual limits. This is useful when combining multiple functions or terms in a problem.
- Product Law: The limit of the product of two functions is the product of their individual limits. This helps simplify expressions involving products of terms.
- Quotient Law: The limit of a quotient is the quotient of the limits, provided the denominator’s limit is not zero. This law is vital when dealing with rational functions.
- Constant Multiple Law: The limit of a constant multiplied by a function is the constant multiplied by the limit of the function. This allows for straightforward scaling of functions.
By mastering these laws, you can handle a variety of functions with confidence and precision, especially when working with more complicated expressions. These principles are widely used in many areas of mathematics, from solving algebraic problems to analyzing rates of change and optimization tasks.
Indeterminate Forms and L’Hopital’s Rule
When evaluating certain mathematical expressions, you may encounter forms that do not provide clear results. These indeterminate forms arise when direct substitution of values leads to undefined or ambiguous outcomes. To resolve these issues, specific techniques, such as L’Hopital’s Rule, offer a systematic approach to determine the correct value of a function under these conditions.
Indeterminate forms commonly encountered include:
- 0/0: This occurs when both the numerator and denominator approach zero as the input value is substituted. It requires further manipulation or techniques to evaluate.
- ∞/∞: Similar to 0/0, this form arises when both parts approach infinity, which requires a more refined method to resolve.
- 0 × ∞: This form represents the product of a value approaching zero and one approaching infinity, which can result in different possible outcomes.
- ∞ – ∞: This occurs when subtracting two terms that both approach infinity, leading to an undefined result.
L’Hopital’s Rule provides a powerful technique for resolving indeterminate forms, particularly 0/0 and ∞/∞. The rule states that if the limit of a quotient results in one of these indeterminate forms, you can differentiate the numerator and denominator separately and then re-evaluate the limit. This process can be repeated if necessary until a determinate result is obtained.
Applying L’Hopital’s Rule allows you to simplify complex expressions and obtain accurate values even in cases where direct evaluation is not possible. It is a fundamental tool for handling challenging problems in mathematical analysis.
Piecewise Functions and Continuity
In mathematics, certain functions are defined by different expressions based on specific intervals or conditions. These functions, known as piecewise functions, can present challenges when analyzing their behavior, particularly when determining whether they are smooth and free of disruptions at certain points. Understanding how to evaluate these functions is key to ensuring their proper definition across different segments.
Defining Piecewise Functions
Piecewise functions are created by combining several smaller functions, each applying to a particular part of the domain. The function takes on different forms depending on the value of the input. For example, a function may be quadratic for values less than 0, linear for values between 0 and 5, and constant for values greater than 5. The key challenge with piecewise functions is ensuring that there are no discontinuities where the different pieces meet.
Ensuring Smooth Transitions
For a piecewise function to be well-behaved, there must be no sudden jumps or breaks at the points where the function changes form. This requires that the function be continuous at the boundaries of each interval. To check this, you need to verify that the function’s value at the boundary matches the value that the function approaches from both sides. If these conditions are met, the function is said to be continuous at that point.
In cases where the boundary conditions are not satisfied, the function may experience a jump or gap, which leads to a discontinuity. Identifying and handling these discontinuities is an essential step in understanding the overall behavior of piecewise functions.
Common Pitfalls in Limit Calculations
When solving problems involving the behavior of functions as they approach specific points, it’s easy to make mistakes if you are not careful. Many common errors can arise when handling tricky expressions or dealing with indeterminate forms. Being aware of these pitfalls can help you avoid miscalculations and improve your problem-solving skills.
Some of the most frequent mistakes include:
- Ignoring Undefined Values: Sometimes, a function might involve division by zero or other undefined operations. Failing to recognize these situations can lead to incorrect conclusions.
- Incorrectly Applying Algebraic Simplifications: While simplifying expressions to find the behavior of a function, it’s easy to overlook necessary steps, especially when factoring or expanding terms.
- Misinterpreting Indeterminate Forms: Not all indeterminate forms can be simplified immediately. For instance, 0/0 does not always lead to a simple answer and might require advanced techniques such as differentiation or other methods.
- Overlooking Piecewise Functions: When dealing with functions defined in pieces, it’s important to check the function’s behavior at the boundaries of each interval. Failing to do so can lead to missing discontinuities or other issues.
- Not Considering Left-Hand and Right-Hand Limits: Some functions may behave differently depending on whether the value is approached from the left or right. Failing to check both directions can lead to incomplete or inaccurate results.
By carefully reviewing the steps in your calculations and paying close attention to the function’s behavior at key points, you can avoid these common pitfalls and ensure accurate results. Taking the time to check for special conditions and indeterminate cases will improve your overall understanding of how functions behave near critical points.
Graphical Interpretation of Limits
Visualizing the behavior of a function as it approaches a particular point can provide a deeper understanding of its overall characteristics. By examining the graph of a function, you can gain valuable insights into how it behaves near specific values, even before performing detailed calculations. This graphical approach is a powerful tool for interpreting the function’s behavior, particularly when dealing with complex or abstract problems.
Identifying Behavior from a Graph
When analyzing the graph of a function, the behavior of the graph near a point is crucial in understanding its limits. Here are some key things to look for:
- Approaching a Single Value: If the graph of the function approaches a single value as the input nears a certain point, the function is said to have a limit at that point. This is often depicted as the graph getting closer and closer to a horizontal or vertical line.
- Unbounded Behavior: Sometimes, a function’s graph may increase or decrease without bound as it approaches a particular point. This indicates that the function does not have a finite limit at that point.
- Discontinuity: If there is a sudden break or jump in the graph, it suggests that the function is not continuous at that point, and thus the limit does not exist in the usual sense.
Left-Hand and Right-Hand Limits
When evaluating the behavior of a function near a particular point, it is important to consider the approach from both the left and the right. On a graph, this means examining the values as the input approaches the point from values smaller (left-hand) and larger (right-hand) than the point in question. The behavior on both sides must be the same for the function to have a limit at that point.
- Left-Hand Limit: This is the value that the function approaches as the input gets closer to the point from values less than the point.
- Right-Hand Limit: This is the value the function approaches as the input gets closer from values greater than the point.
If the left-hand and right-hand limits are equal, the overall limit exists. However, if they differ, the limit does not exist at that point.
Continuity on the Real Line
Understanding how functions behave across the real number line is essential for analyzing their smoothness and predictability. Functions that exhibit no sudden jumps, breaks, or interruptions are considered well-behaved, allowing for continuous progression from one value to the next. When studying these functions, it is important to recognize where the function transitions smoothly from one point to another, without any disruptions.
On the real number line, a function is said to be continuous at a point if its value matches the expected value from both sides of that point. This means there are no unexpected shifts or gaps at any given location. The behavior at specific points can help determine whether the function is continuous over an entire interval or if there are isolated disruptions that need to be addressed.
For a function to be continuous over an interval, it must satisfy several conditions:
- Defined at Every Point: The function must be well-defined at each point within the interval, meaning there are no undefined values or holes.
- Approaches the Same Value from Both Sides: As the input approaches any given point, the function should approach the same value from both the left and right directions.
- No Jumps or Gaps: There should be no sudden jumps or gaps in the graph. The function must smoothly connect all the points on the real number line.
By recognizing these properties, it becomes easier to determine when a function behaves predictably and when there might be disruptions or irregularities in its progression. These principles help identify both smooth and erratic behaviors across the real number line.
Analyzing Limits at Infinity
When examining the behavior of functions as the input grows without bound or approaches extremely large values, it is essential to understand how these functions behave at the extremes. This analysis helps determine whether the function approaches a specific value, diverges, or continues to grow without limit. Understanding these behaviors is key to interpreting long-term trends and overall growth patterns of functions.
As values of the independent variable become extremely large, functions can behave in a variety of ways. Some functions tend toward a specific value as the input increases, while others grow infinitely large, either in the positive or negative direction. The behavior at these extreme points can often be predicted using mathematical methods that help identify how the function behaves as it moves toward infinity.
Common Outcomes When Analyzing Behavior at Infinity
There are several typical outcomes to look for when analyzing the behavior of functions at extreme values:
- Approaching a Finite Value: Some functions stabilize and approach a specific finite value as the input grows larger. These functions have horizontal asymptotes that describe their limiting behavior.
- Diverging to Infinity: Some functions increase or decrease without bound. These functions do not approach a finite value but instead tend to grow infinitely in either direction.
- Oscillating Behavior: Certain functions oscillate as the input grows, never settling on a particular value but instead continuing to alternate between different values or ranges.
Techniques for Analyzing Behavior at Infinity
Several techniques can be used to analyze the behavior of functions as they approach extreme values. Key methods include:
- Dividing by Leading Terms: For rational functions, dividing both the numerator and denominator by the highest power of the independent variable can simplify the expression and make it easier to determine the behavior as the input grows.
- Horizontal Asymptotes: Identifying horizontal asymptotes is a common technique for understanding the long-term behavior of a function. These asymptotes indicate the value the function approaches as the input increases without bound.
- End Behavior Analysis: Analyzing the end behavior of a function, or how it behaves as the independent variable approaches positive or negative infinity, provides insight into the overall growth or decay pattern.
By applying these techniques, we can gain a clearer understanding of the overall behavior of a function as it approaches infinity, offering valuable insights into its long-term trends.
Advanced Topics in Continuity
As we delve deeper into the study of functions, it becomes crucial to explore more complex scenarios where the smoothness and unbroken nature of a function can be tested in unique ways. While basic ideas of function behavior have been widely discussed, advanced topics help refine our understanding of how certain functions behave under specific conditions, particularly when confronted with irregularities or intricate structures.
Advanced exploration involves looking at situations where a function might seem continuous under normal circumstances but exhibits subtle nuances when analyzed more carefully. These topics are especially relevant when studying piecewise functions, highly oscillating functions, or functions that approach infinity in peculiar ways.
Types of Discontinuities
When examining more intricate functions, we encounter various types of discontinuities that influence how a function behaves. These include:
- Jump Discontinuities: These occur when a function “jumps” between different values as the input crosses a particular point. This type of discontinuity is often seen in piecewise functions.
- Infinite Discontinuities: An infinite discontinuity happens when a function tends toward positive or negative infinity near a specific point, creating a vertical asymptote.
- Removable Discontinuities: This type of discontinuity is present when a function is undefined at a point, but its limit exists. This discontinuity can often be “removed” by redefining the function at that point.
Advanced Techniques for Studying Functions
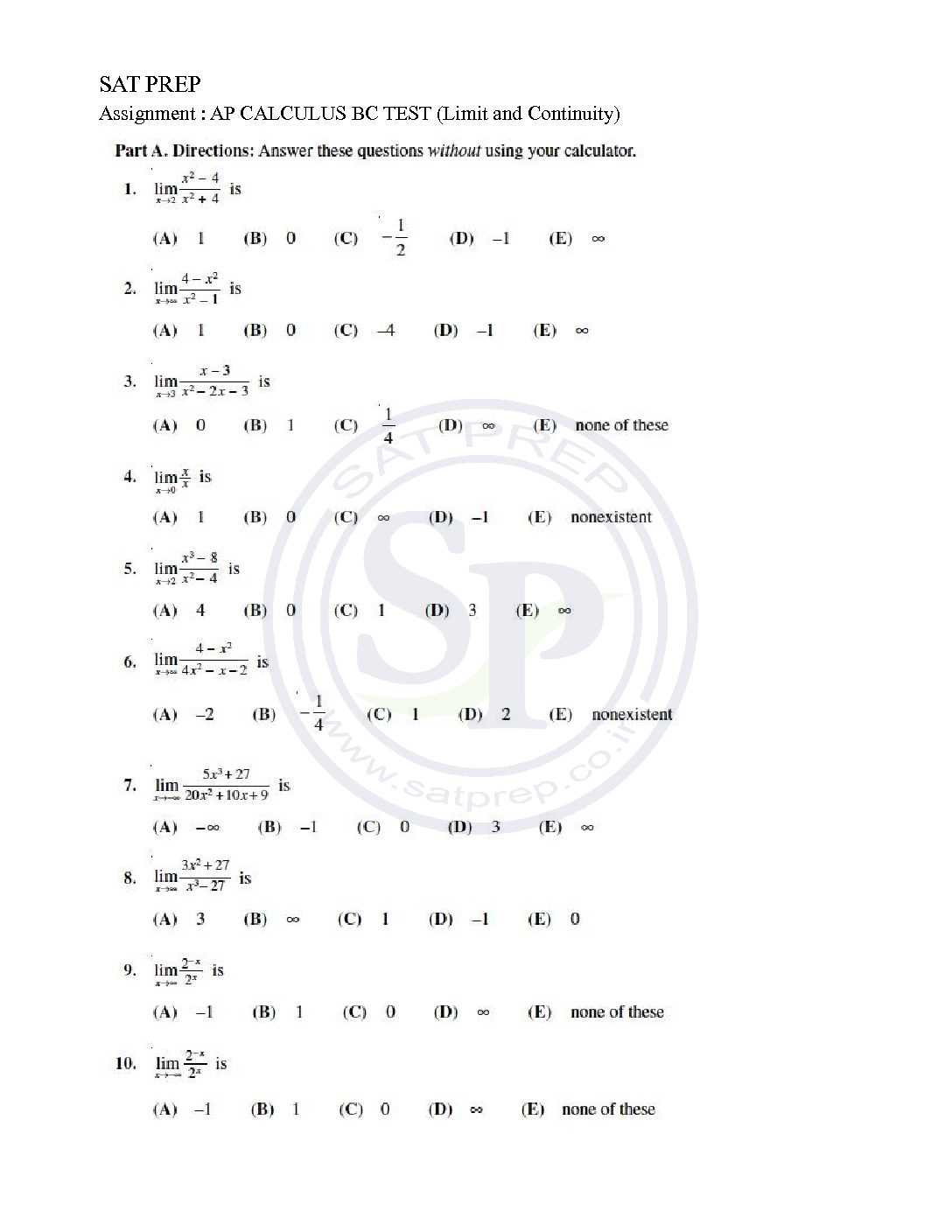
To fully understand the nuances of functions that display complex behaviors, several advanced methods can be employed:
- Asymptotic Analysis: Analyzing the behavior of a function at infinity or near vertical asymptotes allows for a deeper understanding of its long-term behavior and potential discontinuities at extreme values.
- Behavior Near Critical Points: Investigating how a function behaves near critical points, where derivatives may not exist or where the function experiences abrupt changes, provides valuable insight into the overall shape and structure of the function.
- Piecewise Function Analysis: Special attention is given to functions defined by multiple expressions, particularly how their individual pieces join together and where discontinuities may arise at their boundaries.
Understanding these advanced topics provides a more comprehensive view of the complexities involved in the study of function behavior, enabling a more precise evaluation of how functions behave under various conditions.
Practice Problems for Limits and Continuity
To solidify your understanding of how functions behave near specific points or as they approach infinity, it’s essential to work through a variety of problems. These exercises help reinforce concepts, identify potential pitfalls, and enhance problem-solving skills. By practicing, you’ll become more confident in analyzing function behavior and identifying key characteristics that determine if a function is smooth and well-behaved across its domain.
In the following sections, you will find a selection of problems that challenge you to apply the principles of function behavior analysis. These problems cover a range of scenarios, from simple functions to more intricate examples that require deeper understanding and critical thinking. The goal is to build your intuition for recognizing different types of behavior, such as approaching a particular value or dealing with undefined points.
Problem 1: Identifying Discontinuities
Given the function:
f(x) =
{ x² - 4, for x
Determine if this function is continuous at x = 2. If not, identify the type of discontinuity present.
Problem 2: Evaluating Behavior at Infinity
Consider the function:
g(x) = 1 / (x² + 1)
Evaluate the behavior of this function as x approaches infinity. What is the function's behavior as x → ∞ and x → -∞?
Problem 3: Removable Discontinuity
Given the function:
h(x) = (x² - 4) / (x - 2)
Evaluate the function's behavior at x = 2. Is there a removable discontinuity? If so, find the limit as x approaches 2.
Problem 4: Infinite Discontinuity
Given the function:
k(x) = 1 / (x - 3)
Determine the nature of the discontinuity at x = 3. Does the function approach positive or negative infinity near this point?
Problem 5: Behavior of Piecewise Functions
Consider the piecewise function:
m(x) =
{ x + 1, for x
Determine if the function is continuous at x = 1. If it is not, identify the type of discontinuity.
By solving these practice problems, you will be able to sharpen your understanding of function behavior and improve your ability to handle more advanced challenges. Keep in mind the key concepts and techniques discussed, and use these problems as opportunities to deepen your knowledge.
Strategies for AP Calculus AB Exam Success
Achieving success in your upcoming assessment requires more than just knowledge of mathematical concepts. It involves mastering problem-solving techniques, managing your time effectively, and understanding the format of the test. With the right approach, you can enhance your performance and approach each question with confidence. This section will provide you with strategies to optimize your preparation, tackle problems efficiently, and avoid common pitfalls on test day.
The key to success is consistent practice and familiarizing yourself with various question types. Here are some strategies to help you navigate the process with ease:
| Strategy | Description |
|---|---|
| Understand the Concepts | Focus on grasping the core ideas behind each topic. Understanding the “why” and “how” will allow you to approach any problem with a solid foundation. |
| Practice with Past Questions | Use previous assessments to familiarize yourself with the format and question types. This helps you identify trends and patterns in the way questions are asked. |
| Time Management | Practice solving problems within a set time frame. This will help you improve your speed and efficiency on the actual day. |
| Work on Problem-Solving Techniques | Learn various methods for solving problems, such as substitution, graphing, or analyzing functions. Mastering multiple approaches ensures you have options during the assessment. |
| Review Mistakes | After practicing, take time to review your mistakes. Understanding why an answer was wrong helps you avoid repeating the same errors. |
| Stay Calm and Focused | On test day, maintain a calm and focused mindset. If a problem seems difficult, move on to the next and return to it later if needed. |
By incorporating these strategies into your study routine, you can approach the test with greater confidence. Stay consistent in your practice, focus on understanding key concepts, and manage your time wisely to maximize your chances of success.
Final Tips for Studying Limits and Continuity
As you approach the final stages of your preparation, it’s essential to focus on strengthening your understanding of key concepts. Having a solid grasp of foundational ideas will help you tackle complex problems with ease. These final tips are designed to guide you through effective study practices that reinforce your knowledge, build confidence, and ensure you are fully prepared to handle various challenges.
Consolidate your understanding by reviewing core principles, practicing problem-solving techniques, and ensuring you can apply strategies across different scenarios. Consistency is key as you refine your skills. Here are a few final pointers to help you optimize your study time:
- Clarify Key Concepts: Make sure you fully understand essential ideas. Don’t just memorize formulas or steps; focus on why certain methods work and how to apply them in different situations.
- Practice Varied Problems: Exposure to a range of problem types is crucial. It will help you develop the flexibility to approach any question from different angles.
- Work on Your Weak Areas: Identify any topics that are still unclear and dedicate additional time to mastering them. Strengthening your weaknesses ensures that you’re not caught off guard by challenging questions.
- Understand Graphical Representations: Recognize how the graphical behavior of functions relates to key principles. Visualizing problems can sometimes provide valuable insights and aid in understanding.
- Simulate Test Conditions: Practice under timed conditions to improve both speed and accuracy. This will help you manage time effectively and avoid rushing through questions on the day of the assessment.
- Stay Organized: Keep track of important formulas, theorems, and techniques in a well-organized notebook or summary sheet. Having quick access to these references can be helpful during last-minute review sessions.
By focusing on these tips, you will build a strong foundation, refine your problem-solving abilities, and approach the test with confidence. Keep practicing, stay organized, and approach each question with a calm, analytical mindset. Best of luck in your preparation!