Geometry Midterm Review Packet Answers Guide

Preparing for an upcoming exam requires understanding the core principles and methods behind problem-solving. This guide focuses on providing clear explanations and practical techniques to ensure you are well-equipped to tackle the challenges ahead. Whether you are working with shapes, equations, or logical reasoning, mastering these concepts will help you approach each problem with confidence and clarity.

Focus on clarity when solving complex tasks. By breaking down each question into manageable steps, you can avoid confusion and ensure accuracy. Many common pitfalls can be avoided with the right approach and careful attention to detail. With consistent practice and strategic study, you’ll be able to sharpen your skills and improve your performance.

Throughout this guide, we emphasize practical methods for strengthening your understanding and improving your test-taking abilities. By focusing on the most important techniques, you can streamline your preparation and achieve the best results possible. Mastering these foundational skills will help you excel not only on your exams but in future problem-solving situations as well.

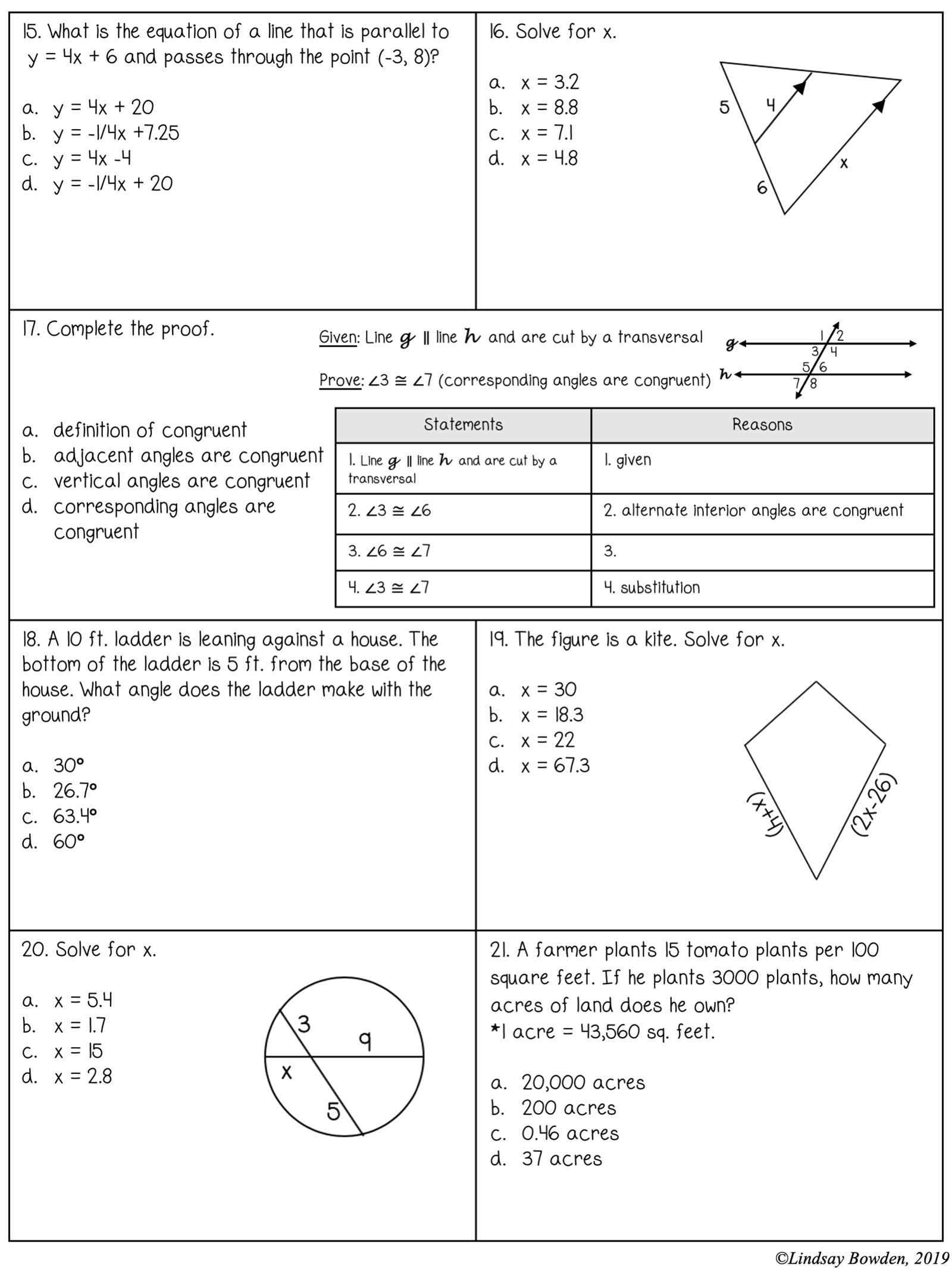
Geometry Exam Preparation and Key Solutions

Success in any exam is largely determined by a clear understanding of key principles and consistent practice. The ability to apply theoretical knowledge to real-world problems is essential for achieving high scores. In this section, we focus on providing essential techniques and step-by-step methods to help you solve complex tasks efficiently and with accuracy.

Key Strategies for Problem Solving

When approaching questions, breaking them down into simpler components allows you to focus on the most important aspects. For example, identifying relevant formulas, understanding how to apply them, and ensuring that each calculation is correctly performed can significantly improve your ability to handle complex tasks. Always double-check your work to avoid common mistakes, and don’t hesitate to review your approach if a solution doesn’t seem to match expectations.

Common Pitfalls and How to Avoid Them

Common errors often occur when the focus shifts from solving problems to rushing through them. Stay mindful of basic concepts and the logical progression needed to reach the correct solution. Take your time to ensure that each step aligns with the rules you’ve studied. A focused approach will not only prevent mistakes but will also help build a deeper understanding of the material.

By consistently practicing and applying these methods, you’ll strengthen your problem-solving abilities and improve your overall performance in any assessment. With a solid foundation and a clear strategy, tackling even the most challenging tasks will become second nature.

Essential Concepts for Exam Preparation

Mastering the fundamental principles is crucial for tackling complex problems with ease. This section highlights key concepts that form the backbone of problem-solving. Understanding these foundational ideas will enhance your ability to approach questions systematically and with confidence.

Focus on the following core areas to strengthen your understanding:

Shapes and Their Properties – Recognizing different forms, their characteristics, and how they relate to one another.
Measurement and Calculation Techniques – Knowing how to measure angles, lengths, and areas accurately.
Logical Reasoning and Proofs – Applying structured thinking to prove statements and solve problems.
Transformations and Symmetry – Understanding how objects change under specific operations like rotation, reflection, and scaling.

Each of these concepts provides a foundation for more advanced topics. Regular practice with these areas will ensure that you are well-prepared for any task that comes your way.

Key Formulas to Remember

Equations and formulas serve as the tools that enable efficient problem solving. Ensure you have a solid grasp of:

Area and Perimeter Formulas – Knowing the right equations for various shapes.
Angle Sum Theorems – Understanding the relationship between angles in different figures.
Volume and Surface Area – Mastering how to calculate 3D space and surface measurements.

By reviewing these concepts and regularly applying them in practice, you’ll build a strong foundation for tackling more complex problems with ease and precision.

Key Formulas to Memorize for Exams

Having a strong grasp of essential equations and formulas is crucial for efficiently solving problems under time constraints. These formulas are the building blocks of many calculations and provide the framework for logical thinking during an assessment. Familiarity with them will not only speed up your work but also increase your accuracy.

The following are fundamental formulas that are important to commit to memory:

Area and Perimeter – These are vital for calculating the dimensions of two-dimensional shapes:

Area of a rectangle: Area = length × width
Perimeter of a square: Perimeter = 4 × side
Area of a circle: Area = π × radius²
Perimeter of a circle (circumference): Circumference = 2 × π × radius

Volume and Surface Area – Important for three-dimensional shapes:

Volume of a cube: Volume = side³
Surface area of a sphere: Surface area = 4 × π × radius²
Volume of a cylinder: Volume = π × radius² × height

Angle Relationships – Crucial for understanding and solving geometric properties:

Sum of angles in a triangle: Sum = 180°
Sum of interior angles of a polygon: Sum = (n – 2) × 180°, where n is the number of sides

By memorizing these formulas, you’ll have the tools necessary to tackle a wide range of problems quickly and accurately. Regular practice will help you recall them effortlessly during the exam, allowing you to focus on solving rather than searching for the right equations.

How to Approach Word Problems

Word problems require a methodical approach to translate the given information into a solvable equation or set of operations. The challenge lies in understanding the context, identifying key elements, and applying the correct principles. By following a clear process, you can simplify seemingly complex problems and solve them step by step.

Start by carefully reading the problem and identifying the key facts. Underline or highlight important numbers, shapes, or relationships mentioned in the text. Next, think about which concepts or formulas are most relevant to the situation. This could involve recognizing the properties of shapes, angles, or measurements that will help you find a solution.

Once you’ve identified the relevant information, break the problem into smaller, manageable parts. If the problem involves multiple steps, solve each part one at a time and check your work as you go. Don’t be afraid to draw diagrams or sketches to visualize the problem, as this can often make it easier to understand and solve.

Finally, after solving the problem, review your solution to ensure that it makes sense within the context of the question. Double-check your calculations and verify that the answer is reasonable given the details provided. By following this structured approach, you can handle word problems with greater ease and accuracy.

Tips for Solving Proofs

Solving proofs requires a logical and systematic approach. The goal is to demonstrate the truth of a statement based on previously established facts, definitions, and theorems. By following a structured method, you can break down complex problems into smaller, manageable steps.

Understand the Given Information

Before starting a proof, take time to clearly understand the given information. Identify what is provided in the problem, such as specific angles, side lengths, or relationships between shapes. This is the foundation upon which the entire proof will be built.

Work Backwards and Use Known Theorems

Start from the conclusion and think about how you can work backwards. Consider the desired outcome and what needs to be shown. Apply known theorems, definitions, and properties that can help bridge the gap between the given information and what needs to be proved. Using logical reasoning is key to finding the most efficient path to the solution.

Throughout the process, write each step clearly, making sure to justify your reasoning with appropriate theorems or postulates. Each step should logically follow from the previous one, leading to a well-supported conclusion. With practice, solving proofs will become a more intuitive and structured process.

Common Mistakes to Avoid on Tests

While preparing for exams, it’s important to be aware of common pitfalls that can lead to unnecessary errors. Often, these mistakes occur when students rush through problems or overlook key details. By recognizing these mistakes and taking steps to avoid them, you can improve your accuracy and performance during the test.

One of the most frequent errors is misinterpreting the question. Always read each problem carefully, ensuring you understand what is being asked before proceeding. Taking the time to comprehend the problem fully can prevent you from making assumptions that lead to incorrect solutions.

Another common issue is skipping necessary steps. Some students try to solve problems too quickly, cutting corners by skipping intermediate steps. However, it’s crucial to work through each stage of the problem to ensure that your solution is well-supported and logically consistent. Even if you are confident in the answer, missing a step can result in a loss of marks.

Rushed calculations are also a major contributor to errors. It’s easy to make simple arithmetic mistakes when working too quickly. Double-check your work, especially when dealing with complex numbers or fractions, to catch any minor mistakes that could affect your final answer.

Lastly, neglecting to review your answers before submitting can be a costly mistake. Take a few minutes at the end of the test to go back and check your work, ensuring that you haven’t overlooked anything important. This quick review can help you catch errors you might have missed initially.

Effective Study Methods for Geometry

Achieving success in any subject requires a structured approach to learning. Developing strong study habits and utilizing effective techniques can help reinforce your understanding of complex concepts. The right study methods not only enhance retention but also improve problem-solving skills and reduce test anxiety.

Here are some proven study strategies that can help you excel:

Study Method	Benefits	How to Implement
Practice Problems	Reinforces understanding and improves problem-solving speed.	Solve a variety of problems from different topics, ensuring each concept is applied correctly.
Flashcards	Helps with memorizing important formulas and theorems.	Create flashcards for key concepts and practice regularly to strengthen memory.
Study Groups	Allows for discussion and clarifying doubts.	Work with peers to solve problems and explain concepts to each other.
Timed Quizzes	Simulates real exam conditions and improves time management.	Set a timer and take practice quizzes to assess your speed and accuracy.
Visual Aids	Assists in understanding complex shapes and relationships.	Use diagrams, charts, and models to visualize problems and solutions.

By integrating these strategies into your study routine, you’ll improve both your confidence and proficiency. Consistency is key–review concepts regularly and seek help when needed to ensure that you are fully prepared for any challenge that comes your way.

Understanding Theorems and Postulates

Mastering key theorems and postulates is essential for solving many types of problems. These foundational principles provide the framework for logical reasoning and are often the stepping stones toward proving more complex relationships. Once understood, they can serve as powerful tools for proving new results and simplifying difficult concepts.

The Role of Theorems

Theorems are statements that have been proven based on established axioms and definitions. They are often used to justify steps in a proof or calculation. The strength of a theorem lies in its logical certainty, and applying it correctly allows you to draw conclusions that follow from known truths.

For example, the Pythagorean Theorem is a widely used result that relates the sides of a right triangle. It states that the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. This theorem can be used to solve many types of problems involving right triangles.

The Role of Postulates

Postulates, or axioms, are basic assumptions that are accepted without proof. These serve as the starting points for building logical arguments and are critical in forming the foundation of any deductive system. While postulates may seem simple, they form the bedrock of more complex reasoning.

For example, one of the fundamental postulates is the idea that through any two points, there exists exactly one straight line. This assumption is taken as true and is used in countless proofs and problem-solving scenarios.

By familiarizing yourself with these principles and practicing their application, you can strengthen your understanding and improve your ability to solve problems efficiently. Both theorems and postulates are indispensable tools in the study of logic and reasoning.

Step-by-Step Guide for Problem Solving

Approaching complex problems requires a systematic method that breaks down each element logically. By following a structured process, you can simplify even the most challenging tasks. Whether you’re working with shapes, angles, or measurements, having a clear plan helps ensure you cover all necessary steps to reach a solution.

1. Read and Analyze the Problem

The first step in solving any problem is to carefully read through the question. Make sure you understand the given information and what is being asked. Identify the known variables, such as lengths, angles, or relationships between different elements. Often, sketching a diagram or marking key points can help visualize the scenario and clarify the problem.

2. Identify Relevant Principles and Formulas

Once you have a clear understanding of the problem, think about the concepts or formulas that might be relevant. This could include relationships like the properties of parallel lines, theorems related to triangles, or formulas for area and volume. Choose the right tools that will help you connect the known values to the unknowns, forming the basis of your solution.

Ensure that you apply these principles correctly, keeping track of any assumptions you may have made during the process. Once the formulas are set, solve step by step, checking each result before moving to the next stage. This methodical approach reduces errors and improves the overall accuracy of your work.

By following these steps, you can tackle problems more efficiently and confidently, ensuring that you’ve considered all necessary information and used the correct techniques throughout the process.

How to Solve Problems Faster

Improving speed in problem-solving is a skill that comes with practice and strategy. By focusing on efficient techniques, you can reduce the time it takes to solve even the most complex problems. Mastering certain strategies not only helps you solve problems faster but also ensures that your solutions are accurate and reliable.

Here are some methods to help you work through problems more quickly:

Method	Benefits	How to Apply
Understand the Problem First	Prevents confusion and wasted time.	Take a moment to carefully read the problem, highlighting key information and clarifying the objective before starting.
Memorize Key Formulas	Reduces time spent recalling formulas during calculations.	Regularly review and memorize essential formulas to have them ready when needed.
Break Down the Problem	Makes complex problems easier to solve.	Divide large problems into smaller, more manageable parts, solving each step-by-step.
Use Elimination	Helps you focus on the most relevant information.	Identify and discard unnecessary information or irrelevant steps that do not contribute to the solution.
Practice with Timed Drills	Improves speed and builds confidence under time pressure.	Set a timer while practicing to simulate exam conditions and gradually increase the time limit as your speed improves.

By incorporating these strategies into your practice routine, you will become more proficient at solving problems quickly and accurately. The key is consistency–regular practice will help you develop faster instincts and improve your ability to work efficiently under pressure.

Trigonometry in Geometry: Key Points

Trigonometry plays a significant role in solving various problems that involve angles and distances, particularly when dealing with triangles. By applying specific ratios and relationships, you can easily calculate unknown values in right triangles and other geometric shapes. Understanding the basic concepts of trigonometry will enhance your problem-solving skills and simplify complex calculations.

Here are some essential points to keep in mind when using trigonometry in geometric problems:

Right Triangle Ratios – The three primary trigonometric ratios are sine, cosine, and tangent. These ratios help relate the angles of a right triangle to its sides.
Pythagorean Theorem – The Pythagorean theorem is a fundamental concept that helps find the length of a side in a right triangle when the lengths of the other two sides are known. It is essential when solving problems involving right angles.
Sine Rule and Cosine Rule – For non-right triangles, the sine and cosine rules allow you to solve for unknown sides and angles. The sine rule relates the sides of a triangle to the sines of its angles, while the cosine rule helps find missing sides when two sides and the included angle are known.
Angle of Elevation and Depression – These concepts are used in real-life applications, such as determining the height of objects or the distance between two points, based on angles and distances.
Unit Circle – The unit circle is a crucial concept for understanding trigonometric functions, as it helps define sine, cosine, and other trigonometric functions for all angles.

Incorporating these trigonometric principles into your geometric problem-solving toolkit will give you the tools to approach a wide variety of problems with confidence. The key to mastering these techniques is practice, as real-world applications often combine multiple concepts, requiring you to apply several methods to find the solution.

Proof Strategies and Techniques

Proving statements and solving problems in mathematics often require a logical sequence of steps to demonstrate the validity of a given proposition. A well-structured proof relies on applying theorems, postulates, and previously established results in a systematic way. Understanding different strategies and techniques will help you break down complex problems and present clear, concise solutions.

Here are some effective strategies and techniques to keep in mind when approaching proof problems:

Start with Known Information

Begin by identifying all the given information and any definitions that apply. Highlight key elements that will help you build the proof. Starting with the basics allows you to create a strong foundation for the argument.

Use Logical Reasoning and Deductive Steps

Each step of your proof should follow logically from the previous one. Deductive reasoning is essential, as it ensures that every conclusion is based on valid premises. This process involves using accepted facts, such as theorems and postulates, to connect information step-by-step.

Direct Proof: Start with known facts and work your way towards the desired conclusion.
Indirect Proof: Assume the opposite of what you want to prove and show that this leads to a contradiction, thereby proving the original statement.
Proof by Contradiction: Assume that the statement is false, then show that this assumption leads to an inconsistency.
Proof by Induction: Used to prove statements that hold for all natural numbers by showing the base case is true and that if it holds for one case, it holds for the next.

As you work through your proof, be mindful of each step’s logical progression and always support your conclusions with valid reasoning. Practice is key to becoming proficient in proof strategies, and with time, you’ll develop an intuition for the most effective techniques to use in various situations.

How to Use Diagrams in Geometry

Diagrams are powerful tools for visualizing and solving problems, especially when dealing with shapes, angles, and spatial relationships. By translating the written description of a problem into a clear and organized visual representation, you can better understand the elements at play and identify relationships that may not be immediately obvious. A well-drawn diagram can make complex concepts much easier to approach and solve.

Here are some tips on how to effectively use diagrams in problem-solving:

Label Important Elements: Always mark key points, lines, angles, and segments in your diagram. Labeling these components ensures you can refer to them easily when applying formulas or logical steps.
Highlight Known Information: Use different colors or markings to distinguish known values or given information. This visual distinction helps you quickly identify the facts you can work with in your solution process.
Draw Auxiliary Lines: Sometimes adding extra lines, such as altitudes, medians, or diagonals, can simplify a problem by creating smaller, more manageable parts. These auxiliary lines often reveal symmetries or relationships that are not immediately obvious.
Use Symmetry: Look for symmetry in your diagram. Symmetrical elements can often simplify calculations and help you make assumptions about angles and distances.
Check for Consistency: Once your diagram is complete, double-check it to ensure it accurately reflects the problem’s description. A flawed diagram can lead to incorrect conclusions.

By practicing these strategies, diagrams will become an indispensable part of your problem-solving toolkit. The clearer and more organized your visual representations are, the easier it will be to apply mathematical principles and reach the correct solutions.

Exploring Coordinate Geometry Basics

Understanding how to work with points, lines, and shapes in a coordinate plane is a foundational skill in mathematics. By using a system that places objects within a two-dimensional grid, you can analyze relationships between various geometric elements, calculate distances, and solve for unknown values. This branch of mathematics provides a bridge between algebraic equations and geometric visualizations, allowing for a more comprehensive approach to solving problems.

Here are some key concepts to grasp when exploring coordinate-based problems:

Coordinate System: The plane is defined by two perpendicular axes: the horizontal axis (x-axis) and the vertical axis (y-axis). The point where the axes intersect is called the origin (0, 0).
Points and Coordinates: Every point on the plane can be identified by a pair of numbers called coordinates (x, y). These numbers represent the position of the point relative to the origin.
Distance Formula: The distance between two points can be calculated using the formula: d = √((x₂ – x₁)² + (y₂ – y₁)²). This formula is derived from the Pythagorean theorem and helps determine the length of a segment connecting two points.
Slope of a Line: The slope of a line represents its steepness and is calculated as the change in y-coordinates divided by the change in x-coordinates. The formula is m = (y₂ – y₁) / (x₂ – x₁).
Equation of a Line: Once you know the slope and a point on the line, you can write the equation in slope-intercept form: y = mx + b, where m is the slope and b is the y-intercept.

Mastering these basics allows you to navigate more complex problems with ease. By understanding how to plot points, calculate distances, and analyze slopes, you can apply these concepts to a wide range of problems in both algebra and geometry. The more you practice, the more intuitive these relationships will become, making problem-solving faster and more efficient.

Preparing for Word Problem Questions

Word problems can often seem daunting, but with the right approach, they can become manageable and even enjoyable. The key to solving these types of questions is breaking them down into smaller, more straightforward steps. By understanding the problem, identifying the relevant information, and applying appropriate mathematical methods, you can efficiently work through word problems and find accurate solutions.

Here are some tips to help you prepare for these types of questions:

Read Carefully: Always read the problem more than once to ensure you understand what is being asked. Pay attention to details such as measurements, conditions, and the relationships between objects or quantities.
Identify Known and Unknown Values: Identify all the given information in the problem and write it down. Then, determine what you need to find or solve for. This will help you focus your efforts on the relevant data.
Draw a Diagram: Visualizing the problem can often make it much easier to understand. Sketch a diagram or figure that represents the situation described in the problem. Label all known values and variables.
Choose the Right Formula: Based on the problem’s context, select the appropriate formula or mathematical method that can help you solve for the unknowns. For example, use the Pythagorean theorem for right triangles or the distance formula for measuring lengths.
Break It Down: Avoid trying to solve the entire problem in one step. Break the problem into smaller, manageable parts and solve each one systematically. Check your work as you go.
Double-Check Your Work: After solving the problem, review your solution and make sure it makes sense within the context of the question. Look for any mistakes in calculations, logic, or interpretation of the information.

By following these strategies, you’ll be better prepared to tackle word problems in an organized and effective manner. Practice regularly, and over time, you will become more confident in approaching these questions with ease.

Understanding Angles and Their Properties

Angles are fundamental components in various mathematical problems, playing a crucial role in understanding the relationships between different shapes and lines. These elements are created when two rays share a common endpoint, and their properties help determine how different geometric figures interact. Understanding the different types of angles and their properties is essential for solving problems effectively and efficiently.

Types of Angles

There are several types of angles, each with distinct characteristics:

Acute Angle: An angle that is less than 90 degrees.
Right Angle: An angle exactly equal to 90 degrees.
Obtuse Angle: An angle greater than 90 degrees but less than 180 degrees.
Straight Angle: An angle that measures exactly 180 degrees.
Reflex Angle: An angle greater than 180 degrees but less than 360 degrees.

Key Properties of Angles

Understanding the key properties of angles is crucial for solving various problems, especially when multiple angles are involved. Some important properties include:

Complementary Angles: Two angles are complementary if their sum equals 90 degrees.
Supplementary Angles: Two angles are supplementary if their sum equals 180 degrees.
Vertical Angles: When two lines intersect, the opposite angles formed are equal. These are known as vertical angles.
Adjacent Angles: These are two angles that share a common side and vertex but do not overlap.

By understanding these types and properties, you can approach problems involving angles with a clear strategy. Properly identifying and applying these principles will allow for more effective problem-solving, particularly in complex geometric scenarios.

How to Tackle Practice Tests

Approaching practice exams effectively requires both strategy and focus. These assessments provide valuable insight into your understanding of various concepts and can significantly boost your problem-solving skills. The key to success lies not only in reviewing the material but also in understanding how to navigate through the problems with precision and confidence.

Start by carefully reading each question and identifying what is being asked. Pay attention to key details, such as specific measurements, shapes, or relationships between elements, as these will often guide your solution process. Break down each problem into manageable parts to avoid feeling overwhelmed.

As you work through each question, make sure to apply the appropriate formulas or rules, ensuring that each step is logical and aligned with the question’s requirements. Keep an eye on time management to ensure that you do not rush through the test, but also do not get stuck on one problem for too long.

After completing the test, review your solutions thoroughly. Double-check your calculations and verify that each step makes sense in the context of the problem. Reflect on any mistakes or areas where you hesitated, as this will help strengthen your approach for the next practice session.

By practicing these techniques, you will enhance both your understanding and confidence, setting you up for success when facing more complex challenges in exams or real-life applications.

Improving Exam Performance

Performing well on assessments requires a combination of preparation, focus, and effective strategies during the test. The key is not only mastering the concepts but also developing a clear approach to solving problems efficiently and accurately. This section outlines essential tips to help you boost your performance and gain confidence for your next evaluation.

Mastering Key Concepts

Start by thoroughly understanding the foundational principles related to the subject. Focus on mastering the essential formulas, theorems, and methods that are commonly used to solve problems. This foundation will serve as the base for solving more complex problems during the exam. Additionally, practice applying these principles in different contexts to reinforce your understanding.

Time Management and Strategy

Effective time management is crucial during exams. Allocate sufficient time for each question, and don’t spend too much time on one problem. If you get stuck, move on to the next question and return later if you have time. Prioritize questions based on your strengths, and aim to complete the easier ones first to build confidence.

In addition to time management, develop a strategy for solving each problem step-by-step. Read each question carefully, identify what is being asked, and outline a plan for solving it. This systematic approach will help you stay organized and reduce the likelihood of making careless mistakes.

By incorporating these strategies into your study and exam routine, you can increase your chances of performing well on tests and achieve the results you’re aiming for.