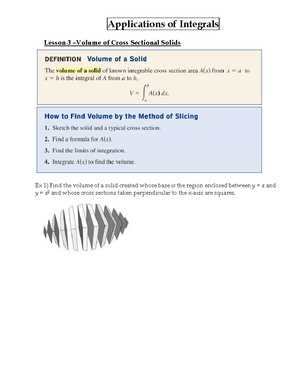
In this section, you will dive into a series of important mathematical problems that challenge your problem-solving abilities. By working through these exercises, you will gain a deeper understanding of key concepts and enhance your skills. The material is designed to guide you step by step, ensuring that you grasp the core principles while building your confidence.
Detailed explanations accompany each problem, allowing you to follow the logical flow from start to finish. By practicing these exercises, you can strengthen your knowledge and approach similar challenges with ease. Whether you’re reviewing for an exam or refining your skills, this guide serves as a valuable resource to clarify complex topics.
Make sure to tackle each problem carefully and refer to the provided solutions to check your progress. With consistent practice, you’ll develop a strong foundation and be well-equipped to solve even more advanced mathematical tasks in the future.
Algebra Nation Answer Key Overview
This part of the guide offers a comprehensive solution set that addresses key problems presented in the learning material. By reviewing these detailed steps, students can better understand how to approach complex mathematical challenges. The solutions serve as a practical reference to ensure clarity in every aspect of the problem-solving process, helping learners to grasp difficult concepts with greater ease.
What You’ll Find in This Guide
The provided solutions include clear, step-by-step instructions, designed to help users follow the logical process behind each problem. By breaking down each exercise into manageable parts, students are encouraged to solve similar problems on their own, fostering independence in learning.
How to Use the Provided Solutions
These solutions are structured to guide learners progressively, with each step offering valuable insights into the mathematical principles at work. Reviewing them not only helps reinforce learning but also allows students to check their understanding and correct any errors in their approach.
| Step | Description |
|---|---|
| 1 | Carefully read the problem and identify key information. |
| 2 | Break the problem into smaller parts to simplify the solution. |
| 3 | Apply the relevant techniques or formulas to each part. |
| 4 | Verify your results and ensure all steps are correctly followed. |
How Section 3 Helps with Algebra

This part of the curriculum is designed to support learners in building a strong foundation in mathematical problem-solving. By working through the exercises, students gain a deeper understanding of key techniques and concepts, reinforcing their ability to tackle more complex challenges in the future. The problems are carefully structured to enhance both critical thinking and practical application.
Improving Problem-Solving Skills

Each task focuses on a specific skill, allowing students to practice and improve their approach to solving problems. As learners move through the exercises, they become more proficient in applying different methods, increasing their confidence in handling similar tasks on their own. The gradual progression ensures that foundational skills are solid before advancing to more intricate concepts.
Developing a Strong Conceptual Understanding
Beyond simply solving problems, this section also helps clarify the underlying principles that govern mathematical operations. By breaking down concepts into digestible steps, learners are better equipped to grasp abstract ideas and apply them in various contexts. This comprehensive approach fosters a deeper, more intuitive understanding of the material.
Understanding Key Concepts in Section 3
In this part of the material, learners are introduced to essential principles that form the backbone of the problems being solved. Mastering these concepts is critical for successfully progressing through the exercises and developing a thorough understanding of the subject. Each concept is explained with clarity to ensure students can apply their knowledge effectively.
Important Principles to Focus On
Several key ideas are emphasized to help strengthen your understanding. These principles are fundamental to solving the problems and will appear in various forms throughout the learning process. Some of the most important concepts include:
- Identifying variables and their relationships.
- Recognizing patterns and how they influence outcomes.
- Applying appropriate operations to simplify complex expressions.
- Understanding how different approaches lead to the same result.
How These Concepts Build Understanding
By focusing on these critical concepts, students develop the ability to approach problems from multiple angles. This helps to foster a more flexible and adaptable mindset. Once these foundational principles are mastered, learners will be better prepared for more challenging topics that require advanced reasoning skills.
- Start by reviewing the basic rules and definitions.
- Apply these rules to simple problems to build confidence.
- Progress to more complex problems as you gain a deeper understanding.
Step-by-Step Solutions for Section 3
This part of the guide offers a structured approach to solving the problems presented in the exercises. By breaking each problem down into smaller, manageable steps, learners can gain a clearer understanding of the process and how to apply the right methods effectively. The goal is to provide a logical progression that helps students solve similar problems with confidence.
How to Approach Each Problem
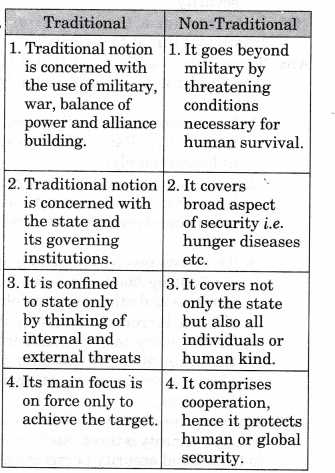
Each solution is divided into clear steps, making it easier for students to follow along and replicate the process. The breakdown is designed to reinforce key concepts while encouraging learners to think critically. Here’s how to work through each exercise:
- Read the problem carefully to understand the given information.
- Identify the most appropriate method to apply based on the type of question.
- Break the problem into smaller parts, solving each one individually.
- Double-check your calculations and make sure all steps are followed correctly.
Example Walkthrough
To illustrate the process, let’s walk through an example step-by-step:
- Start with the basic equation or expression.
- Apply the necessary operations, such as simplifying terms or isolating variables.
- Check your work as you go to ensure no steps are skipped.
- Conclude by verifying that the final result aligns with the original question.
Following this approach helps ensure that no important detail is missed, and it builds a strong problem-solving foundation for tackling more challenging questions in the future.
Tips for Using the Answer Key Effectively
While reviewing the solutions provided for the exercises, it is important to use the guide as a tool to enhance your understanding, not just to check answers. By approaching it strategically, you can maximize its value and improve both your problem-solving skills and overall comprehension of the material.
Best Practices for Reviewing Solutions
Here are some helpful tips to ensure you are using the solutions effectively:
- Don’t rush through the answers: Take time to carefully analyze each step in the solution to understand the reasoning behind it.
- Work through problems on your own first: Attempt the exercises before checking the solutions, so you can identify areas where you may need improvement.
- Use the solutions as a learning tool: If you get stuck, refer to the guide to understand the method used to solve the problem, then try similar problems on your own.
- Focus on the process, not just the result: Pay attention to how each problem is broken down and solved step by step. This helps build your problem-solving abilities.
Additional Tips for Maximizing Learning
Incorporating these additional strategies can further enhance your learning experience:
- Review the solutions multiple times for complex problems to reinforce the concepts.
- Make note of any areas where you feel uncertain and revisit those concepts until you feel confident.
- Try solving a similar problem using the same method, to practice applying the concepts in different scenarios.
By following these tips, you can make the most out of the provided solutions, turning them into a valuable resource that will help strengthen your skills and deepen your understanding of the material.
Common Mistakes to Avoid in Section 3
When working through exercises, it’s easy to make simple errors that can lead to incorrect results and confusion. Recognizing these common mistakes ahead of time allows you to approach the problems more carefully and avoid unnecessary setbacks. Understanding where things often go wrong can help you stay focused and improve your overall performance.
Some of the most frequent mistakes that learners encounter include:
- Misunderstanding the problem: Failing to properly interpret the question can lead to choosing the wrong method or approach. Always read the problem carefully before attempting a solution.
- Skipping steps: Rushing through the process often results in missing key steps. It’s crucial to follow every step methodically, even if it seems repetitive or simple.
- Incorrect calculations: Simple math errors, like adding or multiplying incorrectly, can derail an entire solution. Double-checking your calculations helps ensure accuracy.
- Not reviewing the solution: After completing a problem, it’s important to go back and verify your work. This helps catch any mistakes that may have been overlooked during the first pass.
- Overlooking variables: In some problems, it’s easy to forget to include all variables or constants in equations. Always make sure each component is accounted for before moving on.
By staying mindful of these common mistakes, you can avoid unnecessary confusion and build a stronger understanding of the material. The more practice you get, the more natural these processes will become, leading to greater success in future problems.
How to Improve Your Algebra Skills
Improving your problem-solving abilities requires both practice and understanding the underlying concepts. By developing a structured approach to each task and regularly reviewing the techniques, you can build a solid foundation and boost your proficiency. Here are some strategies to enhance your mathematical skills and tackle problems with greater ease.
Key Strategies for Skill Improvement
To progress in solving mathematical challenges, focus on these key strategies:
- Consistent Practice: The more problems you solve, the better you’ll become. Aim for daily practice to reinforce your skills and build confidence.
- Master the Basics: A strong understanding of fundamental concepts is crucial. Make sure you’re comfortable with basic operations and rules before moving to more complex topics.
- Break Down Problems: When faced with a difficult task, break it into smaller, manageable steps. Solving each part individually makes the overall problem easier to tackle.
- Seek Help When Needed: Don’t hesitate to ask for clarification on challenging topics. Whether it’s from a teacher, tutor, or online resources, getting guidance helps clear up confusion.
Tracking Your Progress
Tracking your improvement allows you to identify strengths and areas for growth. Here’s a simple table to monitor your progress over time:
| Practice Session | Topics Covered | Confidence Level (1-5) |
|---|---|---|
| Session 1 | Basic Operations | 4 |
| Session 2 | Equations and Inequalities | 3 |
| Session 3 | Factoring and Simplification | 5 |
By regularly assessing your performance, you can identify areas that need more attention and ensure steady improvement over time.
Importance of Practice in Algebra
Regular practice is essential for mastering mathematical concepts and improving problem-solving abilities. The more you engage with exercises and work through problems, the more you strengthen your understanding and ability to apply various techniques. Practice helps solidify your grasp of essential principles and ensures you can tackle increasingly complex challenges with confidence.
Building Familiarity and Confidence
One of the key benefits of consistent practice is becoming familiar with common problem types and methods. The more you work on exercises, the more patterns you will recognize, making it easier to approach new problems. Additionally, the act of solving problems helps you build confidence in your abilities, which is crucial for overcoming difficult tasks.
Developing Critical Thinking and Problem-Solving Skills
Through continuous practice, you not only improve your knowledge but also sharpen your critical thinking skills. Each problem provides an opportunity to evaluate different methods and decide on the best approach. This helps you develop the mental agility required to solve problems efficiently and accurately.
Ultimately, practice is the key to mastery. Without it, even the best strategies and techniques remain ineffective. Regularly engaging with problems ensures that you continuously improve and are ready to handle more challenging material in the future.
Breaking Down Algebraic Problems
Approaching complex mathematical problems can often feel overwhelming, but breaking them down into smaller, more manageable steps can make them significantly easier to solve. By deconstructing the problem, you can identify key components and systematically tackle each part. This approach not only simplifies the process but also enhances understanding, making it easier to apply solutions accurately.
The first step in breaking down a problem is to identify the given information. Take note of the variables, constants, and any relationships or operations specified in the problem. Once you have a clear picture of what’s provided, you can move on to the next phase.
Next, simplify the problem by eliminating unnecessary complexities. This could involve combining like terms, isolating variables, or recognizing patterns that reduce the amount of work needed. Simplification helps streamline your approach, allowing you to focus on solving rather than getting bogged down by extraneous details.
Finally, solve step by step. Focus on each individual calculation or transformation required to solve the problem. Take your time with each step and make sure you’re following the right procedure. Don’t skip any phases, as even small errors in one step can lead to incorrect solutions later on.
By adopting this methodical approach, you’ll find that complex problems become more approachable and less intimidating. Each part of the process builds upon the previous one, ensuring a logical flow toward the final solution.
Section 3 Answer Key Explained
In this part, we will break down the solutions to the problems presented, helping you understand the logic and reasoning behind each step. The goal is to provide clarity and deepen your comprehension of how to approach similar challenges. By looking at the process step by step, you’ll gain insight into how each part of the problem is tackled and how to apply these techniques in your own work.
Step-by-Step Breakdown
The solution to each problem follows a logical progression that ensures accuracy. We begin by identifying the necessary information, then apply the correct method to manipulate the equation or expression. At each stage, it’s important to check that the steps align with the operations being performed. This ensures that no errors are made along the way.
Common Approaches Used in the Process
There are several key approaches that are regularly used in solving these types of problems:
- Simplification: Reducing the problem to its simplest form is often the first step. This might involve combining like terms, removing parentheses, or factoring expressions.
- Substitution: In certain cases, substitution is used to replace variables with known values to make the equation easier to handle.
- Solving for unknowns: Once the problem is simplified, the next step typically involves isolating the unknown variable and finding its value.
- Verification: After arriving at a potential solution, it is crucial to substitute the value back into the original equation to confirm its correctness.
By following these steps and understanding how each method is applied, you will improve your problem-solving skills and increase your confidence in tackling similar exercises in the future.
How to Navigate Algebra Nation Answers
Finding the correct solutions to complex problems can sometimes feel challenging, but understanding how to navigate and use the provided solutions can make the process easier. Whether you’re working through a set of problems or reviewing your results, knowing how to effectively access and interpret these solutions is key to mastering the material. This section will guide you on how to efficiently navigate and utilize the resources at your disposal for problem-solving success.
Understanding the Layout of Solutions
The provided solutions are typically organized in a clear and systematic way. Each problem is broken down into individual steps, showing the method used to reach the solution. It is important to read through the explanations carefully and follow the logical progression of each step. This helps you understand the approach being used and allows you to replicate it for similar problems.
Using the Resources for Better Understanding
Rather than simply looking for the final answer, focus on the method used in the solution process. Here are a few tips for getting the most out of the solutions:
- Step-by-step review: Break down each solution into its individual steps. This allows you to see how each part of the problem is addressed and gives you a clearer understanding of the reasoning involved.
- Practice similar problems: After reviewing the solution, practice similar problems to reinforce the concepts. The more you practice, the easier it will become to recognize patterns and apply methods independently.
- Use the solutions as a learning tool: If you’re unsure about a particular concept, use the solutions as a guide to review related topics. This can help clarify difficult concepts and improve your problem-solving skills.
By effectively navigating and engaging with the provided solutions, you can enhance your learning and strengthen your problem-solving techniques.
Focus Areas for Section 3 Success
To excel in this part of the material, it’s essential to concentrate on key concepts and methods that are frequently tested. These focus areas are foundational to understanding the core principles and solving problems efficiently. By honing in on these areas, you’ll be better equipped to approach complex challenges and enhance your problem-solving skills.
Below are some critical focus areas that will help ensure your success:
| Focus Area | Description |
|---|---|
| Equation Manipulation | Mastering the ability to simplify and manipulate equations is crucial. This includes performing operations, isolating variables, and understanding the relationships between terms. |
| Problem Deconstruction | Breaking down complex problems into smaller, manageable steps allows you to approach each part systematically, making it easier to solve. |
| Application of Formulas | Understanding and applying the correct formulas to solve problems ensures accuracy and efficiency, particularly in calculations involving variables and constants. |
| Checking Solutions | After solving a problem, verifying your solution is essential. Substituting your answer back into the original problem helps confirm its correctness. |
By focusing on these areas, you will build a strong foundation that supports both your learning and practical application of the concepts in this part of the material. Make sure to dedicate time to practice each of these skills to reinforce your understanding and boost your confidence when tackling similar problems.
Using Section 3 for Homework Help

When tackling challenging homework problems, utilizing the available resources in a structured way can make a significant difference in your understanding and ability to solve the tasks. This part of the material is designed to provide clarity and support, helping you navigate difficult questions and improve your overall performance. By approaching the solutions and explanations strategically, you can turn homework sessions into productive learning experiences.
Here are some tips for effectively using this resource to enhance your homework process:
- Review the Step-by-Step Process: Rather than just looking for the final result, take the time to study the method used to solve each problem. Understanding the steps will help you apply similar strategies to your own homework problems.
- Identify Common Problem Types: Look for patterns in the types of problems and solutions presented. Identifying these recurring problem structures will make it easier to recognize how to approach your own assignments.
- Use as a Study Aid: Don’t rely solely on the provided solutions; use them as a study tool. Revisit the concepts, try to solve the problems again on your own, and then compare your method with the solution to check for accuracy.
- Break Down Complex Problems: For particularly difficult tasks, break them down into smaller, more manageable parts. The provided materials often show how to deconstruct a complex problem, which you can replicate for other similar tasks.
- Practice with Similar Questions: Use the examples from the resource as a guide to practice additional problems. The more you practice, the more confident you will become in your ability to solve homework questions independently.
By effectively using this resource for homework assistance, you can enhance your understanding of the material and develop problem-solving skills that will benefit you in future assignments and exams. Make sure to use the available solutions not just to find answers but as tools for deeper learning and practice.
Strategies for Problem Solving
Solving mathematical problems can be a challenging yet rewarding process. Having effective strategies in place can significantly improve your problem-solving skills and help you approach tasks with confidence. Developing a systematic approach allows you to break down complex problems into simpler, more manageable steps, ultimately leading to clearer solutions.
Below are some key strategies to keep in mind when working through mathematical challenges:
- Understand the Problem: Before diving into solving, take time to fully understand the problem at hand. Identify what is being asked, and recognize the given information and what needs to be found.
- Break Down the Problem: Complex problems can often be simplified by breaking them down into smaller, more manageable parts. Approach each smaller task one at a time rather than trying to tackle everything at once.
- Choose the Right Strategy: Depending on the type of problem, you may need to use different approaches, such as substitution, elimination, or factoring. Choose the method that best fits the specific problem.
- Work Backwards: If you’re stuck, sometimes it helps to work backwards from the desired solution. This approach can provide insight into how to approach the problem and help you spot potential mistakes.
- Check Your Work: Once you’ve found a solution, always go back and double-check your calculations. Look for any mistakes or misinterpretations of the problem that might have been overlooked.
- Practice Regularly: Regular practice with a variety of problems strengthens your problem-solving skills and helps you become familiar with common patterns and strategies. The more you practice, the more intuitive these strategies will become.
- Use Available Resources: Take advantage of study guides, resources, and solutions to gain a deeper understanding of how to approach similar problems in the future. Learning from examples can be especially beneficial for reinforcing concepts.
By consistently applying these strategies, you can improve your ability to solve problems efficiently and accurately. Over time, you’ll develop a problem-solving mindset that will enable you to tackle even the most complex challenges with confidence and clarity.
Commonly Asked Questions About Section 3
When navigating through complex mathematical problems, it’s common to have questions that arise about the process, methods, or resources available to help solve them. Below are some frequently asked questions that can help clarify common doubts and enhance your understanding of the material.
1. How do I know if I’m using the right approach?
It’s important to analyze the type of problem you’re solving. Ensure you are applying the appropriate strategy based on the given information. If you’re unsure, refer to similar examples or consider working backward to verify your method.
2. What if I can’t understand the steps in the solution?
Sometimes the steps might seem confusing at first. It’s helpful to break down the process into smaller parts and try to understand each one individually. Reviewing similar problems can also provide additional clarity.
3. How can I improve my problem-solving speed?
Practice is key to improving speed. Regularly solving problems, reviewing common techniques, and memorizing essential formulas can help you become quicker in recognizing patterns and applying the right methods.
4. What if my answer doesn’t match the solution provided?
If your solution differs from the expected result, double-check each step of your work. Look for common mistakes like arithmetic errors, misinterpreting the problem, or applying the wrong technique. It can also help to compare your solution process with the provided one to spot discrepancies.
5. How do I use the materials available to me effectively?
To maximize your learning, make sure to review all available resources, including practice problems, solutions, and guides. Work through examples step-by-step and revisit difficult areas to build a stronger understanding of the concepts.
By addressing these common concerns, you can approach your work with greater confidence and enhance your ability to tackle challenges successfully.
Getting the Most Out of Algebra Nation
To maximize the benefits of any educational platform, it’s essential to approach it with a strategy that aligns with your learning goals. Whether you’re looking to strengthen your problem-solving abilities or seeking additional practice to reinforce your skills, there are ways to optimize the use of available resources. By following these tips, you can ensure you are making the most of the tools and materials provided for effective learning.
Utilize All Available Resources
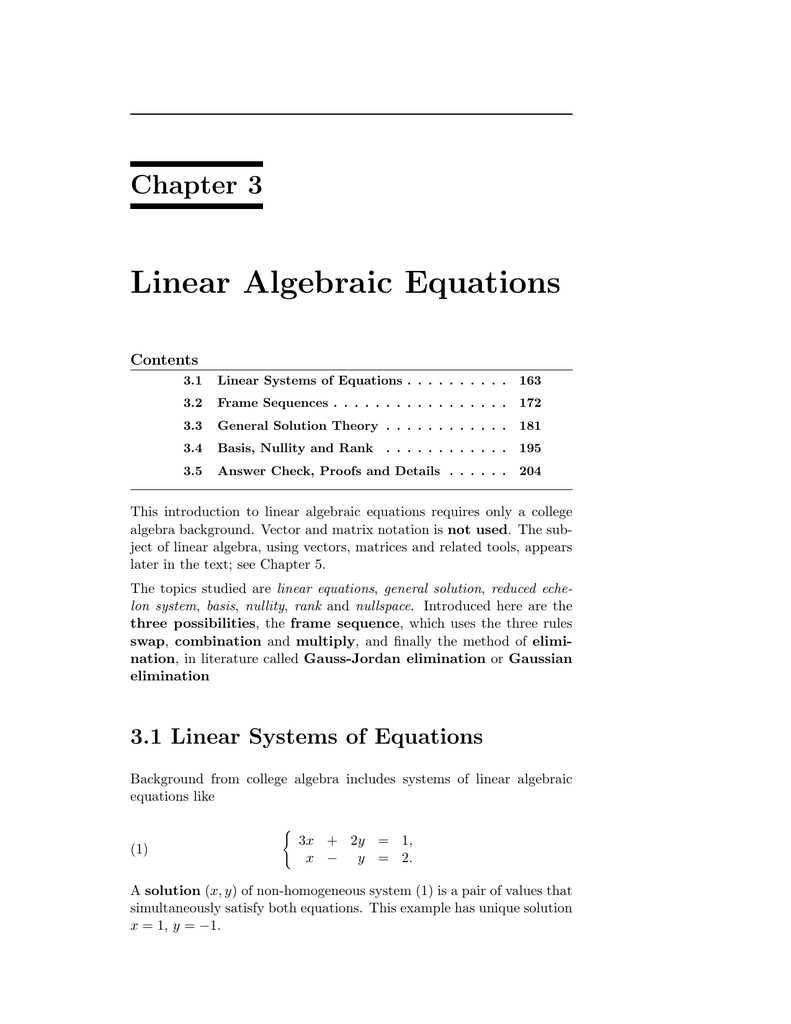
Don’t limit yourself to just one aspect of the platform. Engage with every feature offered, from practice exercises to instructional videos, to get a holistic understanding of the material. Each resource can offer a different perspective, reinforcing your comprehension and providing various ways to approach problems.
Practice Consistently
Regular practice is crucial for mastering any concept. Set aside time each day or week to work through problems systematically. Repetition helps solidify concepts in your memory and makes you more confident when tackling similar questions in the future.
By being proactive and thorough in your approach, you can effectively harness the platform’s full potential, leading to a deeper understanding and greater success in your studies.