
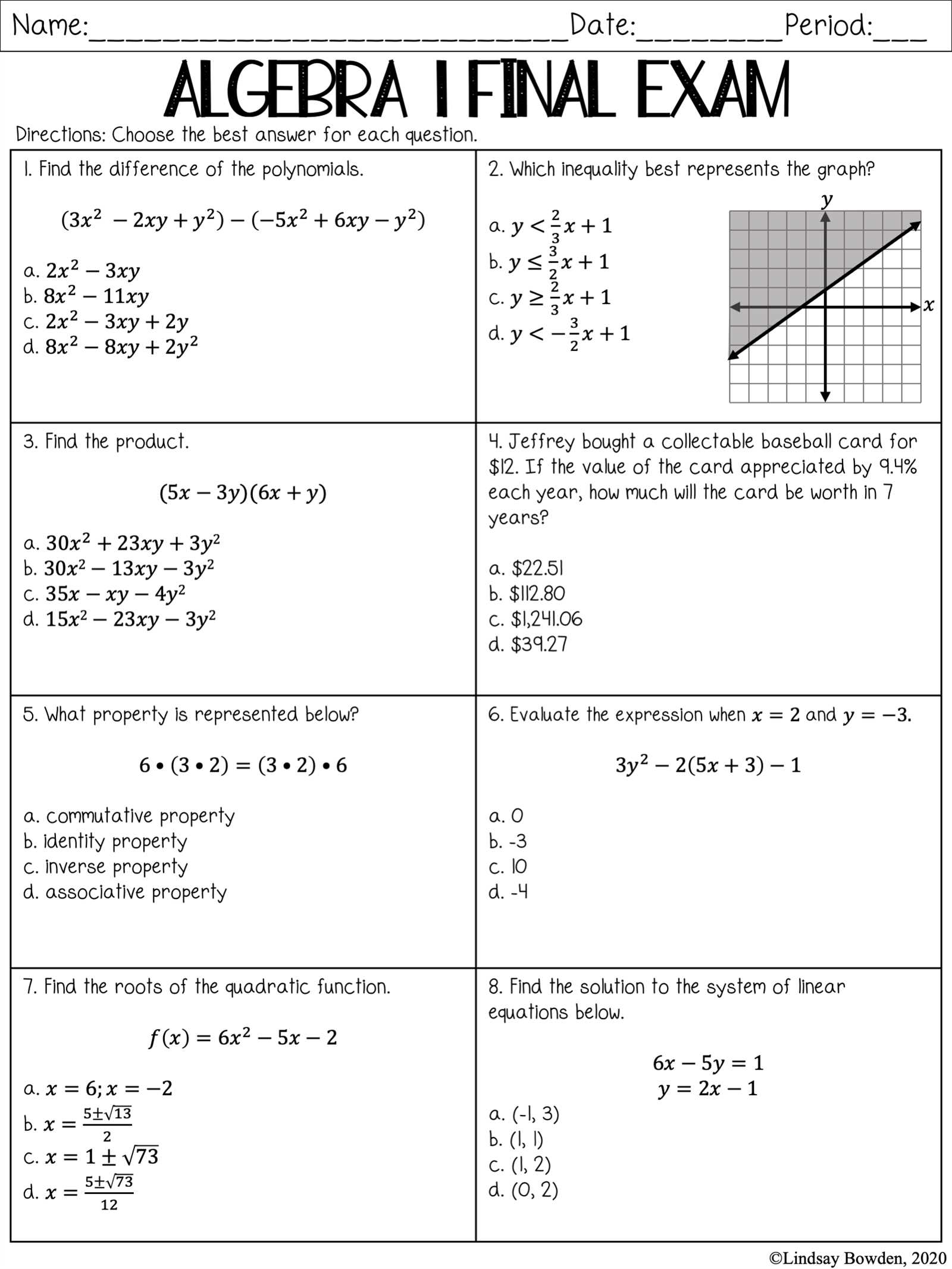
Preparing for an important exam can feel overwhelming, but with the right approach, you can tackle it with confidence. The key to success lies in understanding the material thoroughly and applying problem-solving techniques effectively. Whether you are revisiting challenging concepts or reviewing key principles, this guide will help you navigate the preparation process with clarity.
Throughout your studies, it’s essential to focus on mastering core principles and honing your skills. You’ll find that with consistent practice and a strategic study plan, even the most complex problems become more manageable. This resource provides a structured way to approach your revision, offering insights into how to break down difficult problems and improve your overall performance.
Preparation Guide for Your Upcoming Exam
Effective preparation is the cornerstone of success when approaching any challenging assessment. Organizing your study sessions, identifying key topics, and understanding the underlying principles will greatly enhance your performance. This section will help you structure your revision process, focusing on areas that demand attention and providing strategies to improve both your speed and accuracy.
Start by breaking down the material into manageable sections. Concentrate on concepts that are frequently tested and ensure that you understand the fundamental principles behind them. Using a variety of resources such as practice problems, study guides, and review sheets can help reinforce your knowledge. Consistent practice is crucial, as it not only familiarizes you with the types of questions you will encounter but also builds your confidence.
As you prepare, don’t forget to review common problem-solving techniques. Whether it’s manipulating equations, interpreting graphs, or applying formulas, mastering these skills will save you time during the exam. Time management is another essential aspect of your preparation. Allocate enough time to each topic, but leave room for relaxation and breaks to avoid burnout.
Key Concepts to Review for Your Exam
To perform well on any assessment, it’s crucial to focus on the fundamental concepts that form the foundation of the subject. These are the areas that tend to be the most heavily tested and often require a deeper understanding. Reviewing these core principles will ensure that you are well-prepared to solve a variety of problems and answer questions confidently.
Start with equations and their transformations. Whether it’s solving linear equations, working with quadratics, or handling exponential expressions, mastering these techniques is essential. You should also review methods for simplifying expressions and factoring, as these are commonly tested skills. Being able to identify patterns and apply the appropriate methods will help you work through problems efficiently.
Another important area is understanding how to analyze and interpret graphs and functions. This includes recognizing different types of graphs, understanding how changes in equations affect their shapes, and being able to solve problems based on graphical data. These skills are crucial for answering a wide range of questions related to visual representation and mathematical analysis.
How to Solve Equations Effectively
Solving equations is a critical skill that forms the basis of many mathematical challenges. By mastering the appropriate methods, you can confidently tackle a wide range of problems. This section will guide you through the process of solving various types of equations, focusing on the techniques that will help you work more efficiently and accurately.
Basic Steps for Solving Equations

When solving any equation, it’s important to follow a systematic approach. Here are the key steps:
- Isolate the variable: The goal is to get the unknown on one side of the equation by using inverse operations like addition, subtraction, multiplication, and division.
- Combine like terms: Simplify both sides of the equation by adding or subtracting terms that are similar in nature.
- Apply inverse operations: Reverse the operations that have been applied to the variable. For example, if a variable is multiplied by a number, divide both sides by that number to isolate the variable.
Advanced Techniques for More Complex Equations
For more complicated equations, you may need to use additional methods. These include:
- Factoring: If an equation can be factored, set each factor equal to zero and solve for the variable.
- Quadratic Formula: For equations involving quadratics, use the quadratic formula to find the solutions.
- Substitution and Elimination: These methods are useful for solving systems of equations by eliminating one of the variables or substituting one equation into another.
With practice, these techniques will become second nature, allowing you to solve even the most challenging equations with ease.
Common Mistakes to Avoid During Your Exam
When approaching a challenging exam, it’s easy to make small but significant errors that can impact your performance. By recognizing and addressing common pitfalls, you can improve your chances of success. This section highlights frequent mistakes that many students make and provides tips on how to avoid them to ensure a smoother testing experience.
One of the most common errors is misinterpreting the question. It’s easy to overlook small details, especially under time pressure. Always read each question carefully and make sure you understand what is being asked before attempting to solve it. Double-checking the wording can save you from misapplying methods or using the wrong formula.
Another frequent mistake is skipping steps in calculations. While it might seem efficient to skip intermediate steps, doing so can lead to small errors that compound over time. Always show your work, even if it seems unnecessary–this will help you catch mistakes and ensure that you haven’t missed anything important.
Rushing through the test is also a mistake many students make, especially when time is tight. It’s tempting to move quickly through easier questions, but hurrying can cause careless errors. Take the time to check your answers, even if you’re confident about them.
Lastly, not managing time properly can result in leaving questions unanswered or insufficiently explored. Ensure that you allocate time to each section and leave room to review your work before submitting the exam. Prioritizing difficult questions and returning to simpler ones later can help you manage your time more effectively.
Tips for Efficient Study Sessions
To maximize your preparation and improve retention, it’s important to approach study sessions with a focused and organized strategy. By setting clear goals and following structured methods, you can optimize your study time and reduce the feeling of overwhelm. This section provides key strategies that will help you make the most of your study sessions and improve your overall performance.
Create a Study Schedule
One of the most effective ways to stay on track is by planning your study time in advance. Break down the material into manageable chunks and set specific goals for each session. Allocate more time to difficult topics and ensure that you review regularly to reinforce what you’ve learned. A well-organized schedule can help you stay disciplined and prevent last-minute cramming.
Active Learning Techniques
Instead of passively reading through notes, engage in active learning techniques to deepen your understanding. Practice solving problems, explain concepts aloud, or use flashcards to test your knowledge. Working through problems and applying concepts will help solidify your understanding and make it easier to recall information during your assessment.
Additionally, try studying in focused intervals, such as using the Pomodoro technique, where you study for 25 minutes and then take a 5-minute break. This method helps maintain concentration and prevents burnout during long study sessions.
Understanding Word Problems in Mathematics
Word problems can be some of the most challenging aspects of any exam, as they require both comprehension and the application of mathematical concepts. Rather than just solving equations, these problems ask you to translate real-world situations into mathematical expressions. This section will help you understand how to approach and solve these problems efficiently, focusing on key strategies that simplify complex scenarios.
Breaking Down the Problem
The first step in solving any word problem is to carefully read and understand the information provided. Look for important data, keywords, and phrases that can guide you in forming an equation. Identify the unknowns and determine what the problem is asking you to find. Once you know what you’re solving for, it becomes easier to set up the correct approach.
Setting Up Equations
After breaking down the problem, the next step is to translate the word problem into a mathematical equation. This may involve using variables to represent unknown values, setting up proportions, or applying formulas related to the context of the problem. Pay attention to units and make sure they match across the equation. This step is crucial for solving the problem correctly and efficiently.
As you practice, you will improve your ability to quickly identify relevant information and translate it into solvable equations, making word problems less intimidating and easier to tackle.
Practice Problems to Boost Confidence
One of the best ways to build confidence before any assessment is through consistent practice. By solving a variety of problems, you can strengthen your understanding of key concepts and become more comfortable with different question types. This section offers a set of practice problems designed to challenge your skills and improve your readiness for the exam.
Problem Set 1
The following problems cover a range of topics that are commonly tested. Take your time to solve each one, and try to work through them without referring to notes. Afterward, check your solutions to identify areas that need further review.
| Problem | Solution |
|---|---|
| Solve for x: 3x + 5 = 20 | x = 5 |
| Simplify: (4x – 3) + (2x + 6) | 6x + 3 |
| Solve for y: 2y – 4 = 16 | y = 10 |
| Factor: x² – 5x + 6 | (x – 2)(x – 3) |
Problem Set 2
In this set, we focus on more complex problems. Attempt to break them down step by step, and remember that persistence is key to mastering difficult topics.
| Problem | Solution |
|---|---|
| Solve for x: 2x² + 3x – 5 = 0 | x = -5/2, 1 |
| Simplify: (2x² + 3x) – (x² – 4x) | x² + 7x |
| Solve for z: 4z – 3 = 2z + 5 | z = 4 |
| Expand: (x + 3)(x – 4) | x² – x – 12 |
By regularly solving problems like these, you will not only improve your skills but also build the confidence needed to tackle even the most difficult questions with ease.
Strategies for Time Management During Exam
Effective time management during an exam can significantly impact your performance. By strategically allocating time to each section and staying organized, you can avoid feeling rushed and ensure that you complete all the tasks to the best of your ability. This section provides valuable tips on how to manage your time efficiently, reduce stress, and maximize your chances of success during the test.
Prioritize the Questions
When the exam begins, take a moment to skim through all the questions. Identify the ones that seem easier and those that may take longer. Prioritize them based on your strengths and the time required to solve each one.
- Start with familiar questions: Begin with the problems that you are confident in. This will help build momentum and boost your confidence.
- Leave difficult questions for later: If you encounter a challenging question, don’t spend too much time on it. Move on and come back to it once you’ve finished the rest of the test.
Set Time Limits for Each Section
To ensure you complete the exam on time, assign a specific time limit to each section or question. Stick to these limits as closely as possible to avoid spending too much time on one section and running out of time for others.
- Divide the total exam time: If the exam is 90 minutes long and contains 3 sections, allocate 30 minutes to each section.
- Adjust as needed: If you finish early in one section, use the extra time for more difficult questions in another section.
By following these strategies, you can stay focused, avoid panic, and manage your time more effectively throughout the exam, increasing your chances of success.
How to Interpret Graphs in Mathematics
Understanding graphs is essential for interpreting data and solving mathematical problems. These visual representations allow you to quickly grasp relationships between variables, identify trends, and make predictions. This section will guide you on how to analyze graphs effectively, focusing on key features such as intercepts, slopes, and shapes of curves.
Key Features to Identify
When interpreting a graph, it’s important to first identify the key features that will help you understand the relationship between the variables. Look for the following elements:
- Intercepts: The points where the graph crosses the axes (x-intercepts and y-intercepts) are often critical in solving equations.
- Slopes: The slope indicates the rate of change between the variables, which can be either positive, negative, or zero.
- Curvature: The shape of the graph (whether it’s linear, quadratic, etc.) gives insight into the type of relationship between the variables.
Example Graphs and Their Interpretation
Below is an example of different types of graphs and how to interpret their key features:
| Graph Type | Key Features |
|---|---|
| Linear Graph | The graph is a straight line, with a constant slope, indicating a direct proportionality between variables. |
| Quadratic Graph | The graph is a parabola. The vertex represents the maximum or minimum value, and the axis of symmetry divides the graph into two equal halves. |
| Cubic Graph | The graph has an S-shape. It may have more than one turning point, indicating changes in direction and rate of growth. |
| Exponential Graph | The graph shows rapid growth or decay. It has a horizontal asymptote, representing a limit that the graph approaches but never touches. |
By identifying these key features in a graph, you can better understand the mathematical relationships and solve problems more effectively.
Essential Formulas to Memorize
Mastering fundamental equations is essential for solving a wide range of mathematical problems. These key formulas provide the framework for understanding relationships between variables and enable you to approach various tasks with clarity. By committing these expressions to memory, you can work more efficiently and with greater confidence, ensuring a deeper understanding of the material.
Key Formulas for Linear Relationships
Linear equations are foundational in mathematics, representing constant rates of change. Here are some critical formulas to remember:
- Slope-Intercept Form: y = mx + b
This equation describes a straight line, where m represents the slope and b is the y-intercept. - Point-Slope Form: y – y₁ = m(x – x₁)
This form is useful when you know a specific point (x₁, y₁) on the line and the slope m. - Distance Formula: d = √((x₂ – x₁)² + (y₂ – y₁)²)
This formula calculates the distance between two points (x₁, y₁) and (x₂, y₂).
Key Formulas for Quadratic Relationships

Quadratic equations describe parabolic curves and are crucial for solving many problems in geometry and physics. The following formulas are essential:
- Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a
This formula solves quadratic equations in the form ax² + bx + c = 0, where a, b, and c are constants. - Vertex Form: y = a(x – h)² + k
In this equation, the vertex of the parabola is located at (h, k), with a determining its direction and shape. - Standard Form: y = ax² + bx + c
This is the general form for quadratic functions, useful for identifying properties such as roots and the direction of the parabola.
Regularly practicing with these formulas will help strengthen your understanding and ability to solve problems swiftly. As you familiarize yourself with these essential tools, you’ll be well-equipped to tackle a variety of mathematical challenges.
Importance of Showing Work in Solutions

Demonstrating the steps taken to solve a problem is a crucial part of the problem-solving process. Not only does it help ensure accuracy, but it also allows you to identify errors more easily and track the logic behind your solution. By showing all steps, you create a clear and transparent path for others (or yourself) to follow, ensuring the integrity of the final result. This approach promotes understanding and can serve as a useful reference for reviewing or explaining your work.
Benefits of Showing Work
Here are some reasons why documenting your process is essential:
- Improves Clarity: Writing down every step in a problem allows you to organize your thoughts and makes the solution process clear and easy to follow.
- Prevents Mistakes: When you document your work, you reduce the risk of skipping crucial steps or making errors. It acts as a safeguard for your reasoning.
- Helps with Understanding: By showing your process, you’re more likely to gain a deeper understanding of the problem and how each step contributes to the final solution.
- Provides Evidence: If you’re required to review or justify your work, showing the steps offers tangible proof of how you arrived at your answer.
How to Effectively Show Your Work

It’s not enough to simply write down random calculations. Properly formatted and clear documentation ensures that others can easily follow along with your thought process. Here are some tips for effective problem documentation:
| Step | What to Include |
|---|---|
| 1. Identify the problem | Read the question carefully and write down what is being asked. Highlight key information. |
| 2. Choose a method | Decide on the appropriate approach or formula to solve the problem. |
| 3. Solve step by step | Break down the problem into smaller steps. Clearly write each calculation or transformation. |
| 4. Check the result | Once you reach an answer, review your steps to confirm the solution is correct. |
By following these guidelines and consistently showing your work, you can enhance both your problem-solving abilities and your ability to communicate complex solutions effectively.
What to Expect in Your Exam

Preparing for a test can be overwhelming, especially when you’re unsure of what to expect. However, understanding the structure and content of your upcoming assessment can help you focus your study efforts. Typically, exams will test your ability to apply various techniques, solve problems, and demonstrate a deep understanding of the material. Knowing what types of questions to expect will help you approach the exam with confidence.
Types of Questions You May Encounter
There are several types of questions that are commonly featured in assessments of this nature:
- Multiple Choice: These questions will provide several possible answers, and you’ll need to choose the correct one based on your knowledge and calculations.
- Short Answer: You will need to provide a brief explanation or show the solution to a specific problem.
- Long Form Problems: These require a detailed process, where you show your steps to solve a more complex issue. They are often worth more points.
- Graphing: You may be asked to interpret or create graphs that represent specific mathematical concepts.
Key Concepts to Review
To ensure you’re well-prepared, focus on the following topics:
- Equations and Inequalities: Be sure to understand how to solve linear equations, quadratic equations, and inequalities.
- Functions: Review the properties of different types of functions and how to manipulate them.
- Exponents and Radicals: Know how to simplify expressions with exponents and radicals, and be familiar with their properties.
- Polynomials: Practice factoring, expanding, and simplifying polynomial expressions.
- Rational Expressions: Understand how to simplify, multiply, and divide rational expressions.
By focusing on these areas and practicing problem-solving, you’ll be ready to tackle your exam with ease and clarity. Remember to stay calm and take your time during the test to carefully review each question before answering.
How to Tackle Complex Number Problems
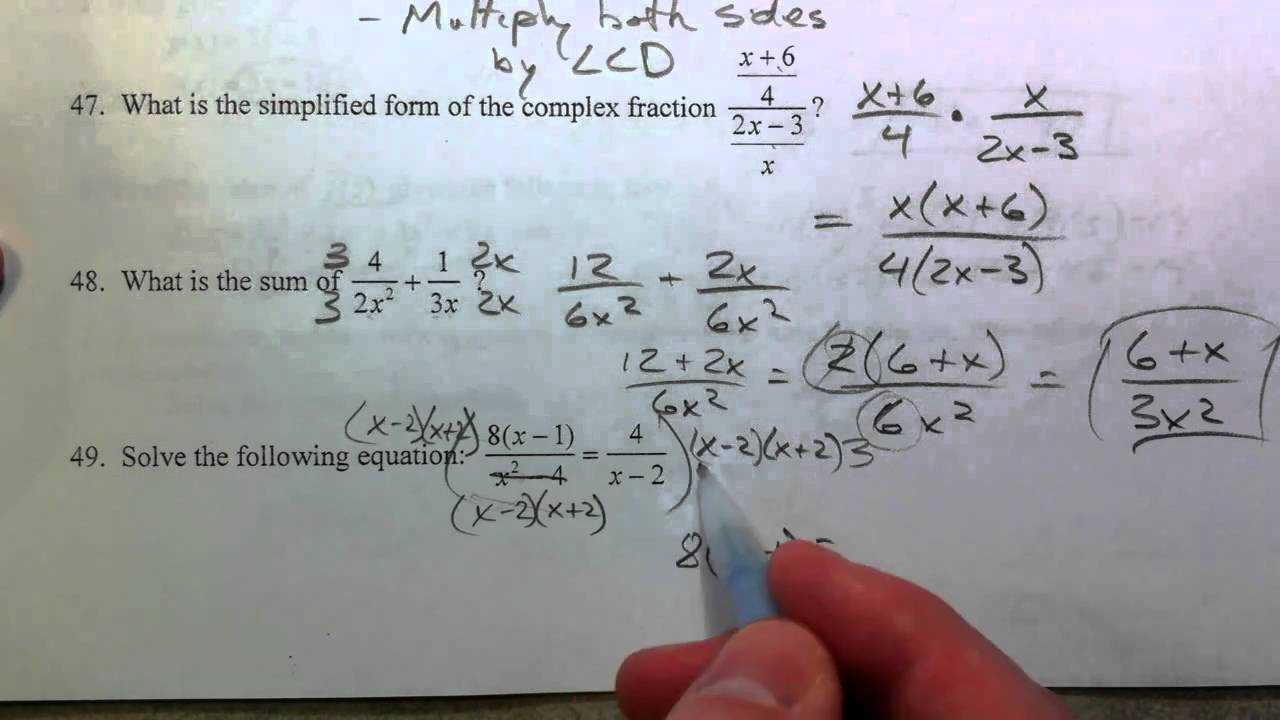
Dealing with problems involving complex numbers can seem intimidating at first, but once you understand the basic concepts, they become much easier to handle. Complex numbers consist of a real part and an imaginary part, and mastering their operations is key to solving related problems effectively. The goal is to break down the problem into simpler components and apply the appropriate mathematical rules for addition, subtraction, multiplication, and division.
Key Concepts to Master
Before diving into problems, make sure you’re familiar with these fundamental concepts:
- Imaginary Unit: The imaginary unit, represented as i, is defined as i = √-1.
- Real and Imaginary Parts: A complex number is typically written as a + bi, where a is the real part and bi is the imaginary part.
- Conjugates: The conjugate of a complex number a + bi is a – bi. This concept is especially important when simplifying complex fractions.
Steps to Solve Complex Number Problems
Follow these steps when tackling problems involving complex numbers:
- Addition and Subtraction: To add or subtract complex numbers, combine like terms–add or subtract the real parts and the imaginary parts separately. For example: (3 + 2i) + (4 – 5i) = 7 – 3i.
- Multiplication: To multiply complex numbers, use the distributive property and simplify the result. Remember that i² = -1 and replace any occurrences of i² with -1. For example: (3 + 2i)(4 – 5i) = 12 – 15i + 8i – 10i² = 12 – 7i + 10 = 22 – 7i.
- Division: To divide complex numbers, multiply both the numerator and the denominator by the conjugate of the denominator. This eliminates the imaginary part from the denominator. For example: (3 + 2i) / (4 – i) becomes (3 + 2i)(4 + i) / (4 – i)(4 + i).
With these strategies and a bit of practice, you can confidently approach and solve any problem involving complex numbers.
Algebra 2 Midterm: Tips for Success
Preparing for an important exam can often feel overwhelming, but with the right strategies and mindset, you can tackle even the most challenging topics with confidence. Focusing on key concepts, practicing problem-solving techniques, and staying organized can make a significant difference in your performance. In this section, we’ll explore practical tips that will help you succeed in your upcoming assessment.
One of the most important aspects of preparation is to make sure you understand the foundational principles before moving on to more complex topics. This will allow you to approach problems with a clear framework in mind. Take time to review the core concepts and work through practice problems to reinforce your understanding.
Additionally, don’t overlook the importance of managing your time effectively during your review sessions. Setting aside dedicated blocks of time each day for focused study, while also allowing time for breaks, can help maintain your energy and prevent burnout. With consistent practice and a solid plan, you’ll be well-prepared when the day of the test arrives.
Resources for Algebra 2 Review
Having access to the right tools and materials can make all the difference when preparing for a challenging exam. Whether you are revisiting fundamental concepts or reinforcing advanced techniques, the right resources can help clarify difficult topics and improve problem-solving skills. In this section, we will explore some valuable tools that can aid in your review process and ensure you’re ready for any challenge that comes your way.
Online platforms, such as video tutorials and interactive problem solvers, provide a flexible way to review at your own pace. Websites like Khan Academy and Coursera offer free lessons that cover everything from basic principles to advanced techniques, helping you build a solid understanding. Additionally, forums and discussion boards provide opportunities to ask questions and engage with peers or instructors who can offer further insights.
For those who prefer hands-on practice, printable worksheets and practice exams are excellent for reinforcing what you’ve learned. Many educational websites offer downloadable resources that simulate real exam conditions, allowing you to test your knowledge under time constraints. These practice materials will help you gauge your progress and identify areas where you need additional focus.
How to Check Your Answers Effectively
Ensuring the accuracy of your solutions is a crucial step in any problem-solving process. After completing your work, reviewing your results carefully can help you identify mistakes and verify that your approach was correct. By using specific techniques and methods, you can increase your confidence in the solutions you’ve derived and avoid common errors that could impact your final score.
1. Break Down Each Step
One of the most effective ways to verify your solutions is by reviewing each step methodically. Going through your work step by step allows you to spot where you might have made an error and ensures that the logical progression is intact.
2. Use Alternative Methods

Whenever possible, try using different techniques to arrive at the same solution. If you approached the problem algebraically, consider using graphical methods, or if you solved numerically, check your results by estimation. This will help confirm the validity of your answer from different angles.
3. Compare With Known Formulas

Another way to validate your answers is by comparing your results to established formulas and known solutions. If your answer is significantly different from the expected result, it may signal an error in your process.
4. Double-Check Your Calculations
- Recheck any arithmetic operations, especially multiplication, division, or factoring steps, as they are common areas for mistakes.
- Verify signs (positive and negative) to ensure they align with the context of the problem.
- Reevaluate any substitutions made in your equations to ensure accuracy.
5. Use Technology
- Online calculators and graphing tools can be used to check your answers, providing quick feedback on whether the solutions are reasonable.
- Many software programs, such as Wolfram Alpha or Desmos, can solve problems and compare results with your calculations.
Staying Calm During the Test
Maintaining composure during an important assessment is essential for performing at your best. Stress and anxiety can impair your ability to think clearly and solve problems effectively. By using specific strategies to manage nerves, you can approach the test with confidence and clarity.
1. Prepare Thoroughly in Advance
One of the best ways to reduce anxiety is by being well-prepared. Familiarize yourself with the material and practice different types of problems. The more confident you are in your knowledge, the less likely you are to feel overwhelmed during the test.
2. Take Deep Breaths and Stay Focused
If you feel nervous during the test, pause for a moment to take deep, slow breaths. This simple technique helps lower stress and allows you to refocus your mind. Staying calm will help you think more clearly and avoid rushing through questions.
3. Manage Your Time Wisely
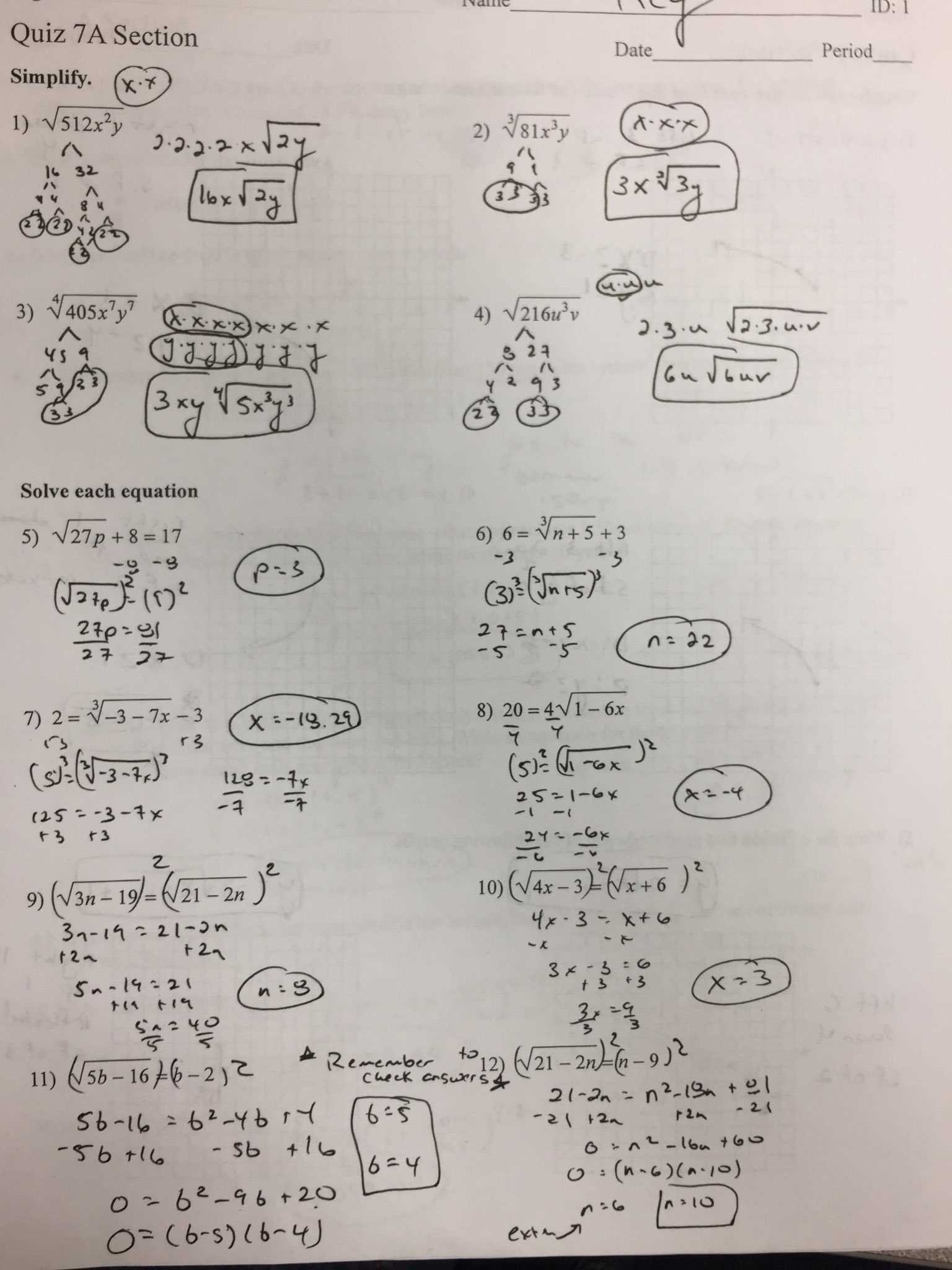
Time pressure is one of the most common stressors during assessments. Plan your time carefully, allocating enough moments to address each section of the test. If you get stuck on a difficult problem, move on and return to it later–this will prevent you from wasting precious time and getting frustrated.
4. Trust Your Instincts
Don’t second-guess yourself too much. Trust your initial instincts and the methods you’ve practiced. Overthinking can lead to confusion and mistakes. If you’re unsure about an answer, eliminate obviously incorrect options to increase your chances of selecting the right one.
5. Keep a Positive Mindset
It’s essential to stay positive. Even if you encounter a challenging problem, remind yourself that you are capable of solving it. A positive attitude can boost your confidence and help you approach the test with a calm and clear mind.