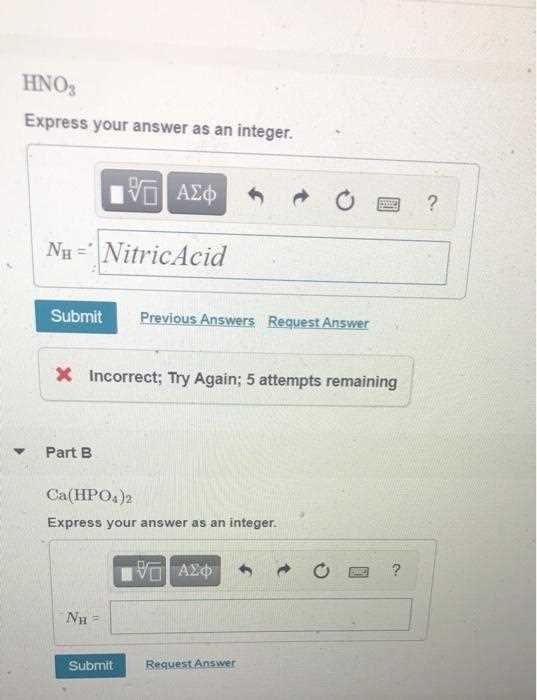
In scientific fields, providing precise numerical results is crucial for clear communication and effective problem-solving. Whether conducting experiments or analyzing data, using whole numbers when appropriate can prevent misunderstandings and lead to more reliable outcomes.
When calculations require simplification or rounding, it’s essential to follow established guidelines to ensure the results are both accurate and relevant. This approach is particularly important in fields like physics and biology, where exact figures often dictate the success of an experiment or process.
Accurate numerical representation not only enhances clarity but also facilitates comparison and consistency across different scientific studies. Applying the correct methods can streamline calculations, ensuring that the final outcome aligns with the practical needs of the task at hand.
Following these practices helps avoid errors and makes the scientific work more accessible, creating a solid foundation for further analysis or discovery.
Expressing Answers as Whole Numbers in Scientific Calculations
In scientific calculations, presenting results as whole numbers is often necessary for simplicity and clarity. This practice ensures that outcomes are easily understood and applied in further studies or experiments. By avoiding complex fractions, numerical data becomes more accessible for comparison and interpretation.
In many instances, such results reflect the practical limits of measurement tools or the expected accuracy of the calculation. For example, in laboratory settings, using rounded figures can help avoid overcomplicating reports or analysis, focusing instead on the most relevant data for the given context.
Moreover, rounding to the nearest whole number or performing other similar adjustments is common in certain processes, especially when dealing with large datasets or when precision beyond a certain point is unnecessary. This approach ensures that the final figures are not only correct but also appropriate for real-world applications and further calculations.
Importance of Whole Number Results in Scientific Work
In scientific calculations, providing results as whole numbers is essential for clarity and consistency. It allows for easier interpretation of data and ensures that conclusions are based on the most relevant figures. This approach also minimizes confusion, particularly when dealing with large datasets or when performing repeated experiments.
Whole numbers are often used in practical applications where precision beyond a certain point is unnecessary. For instance, when calculating quantities of substances for reactions or preparing solutions, results are typically rounded to the nearest whole number to match the limitations of the measuring instruments and the scale of the task.
Using whole numbers in scientific reporting simplifies communication between researchers, making data more accessible and comparable. This practice also helps to maintain consistency in experimental results and ensures that findings can be applied effectively across various contexts without unnecessary complexity.
Why Accuracy Matters in Scientific Calculations
In scientific work, precision is essential to obtaining reliable and meaningful results. Even small errors in calculations can lead to significant discrepancies in conclusions, affecting the validity of experiments and the application of findings. Ensuring accuracy in every step of the process is crucial for maintaining the integrity of the data and the overall reliability of the work.
Impact on Experimental Outcomes
Accurate calculations directly influence the outcome of scientific experiments. In fields like physics, biology, and engineering, even a minor miscalculation can result in incorrect assumptions, leading to flawed hypotheses or incorrect conclusions. Correct numerical results are necessary to ensure that theories are tested properly and that experiments yield reproducible, valid results.
Ensuring Consistency Across Studies
Accuracy also plays a crucial role in ensuring that research findings are consistent and comparable across different studies. When experiments are repeated or results are shared among researchers, precise measurements and calculations are essential for cross-validation. This consistency enables the scientific community to build on existing knowledge and drive further advancements.
Common Mistakes in Scientific Calculations
Errors in mathematical procedures are common when performing scientific calculations, often leading to inaccurate results or misinterpretations. These mistakes can occur at various stages, from data collection to the final computation. Even a small oversight can distort findings and impact the overall validity of an experiment or analysis.
One frequent mistake is failing to round numbers correctly, which can introduce inconsistencies. Another common issue is neglecting significant figures, which can affect the precision of the outcome. Misapplying formulas or using incorrect units also leads to errors, often resulting in outcomes that are far from the expected values.
Additionally, overlooking basic mathematical principles such as proper order of operations can lead to miscalculation of values. It’s essential to follow established protocols and check calculations carefully to minimize the risk of these common pitfalls.
When to Round Your Scientific Results
Rounding is an essential step in scientific calculations, but it must be done carefully to avoid introducing errors. Knowing when to round ensures that the results remain accurate and meaningful while avoiding unnecessary complexity. In most cases, rounding is necessary when the precision of the measurement or calculation exceeds the limits of the instruments used or when exact values are not required for practical purposes.
Guidelines for Rounding
Typically, results are rounded based on the number of significant figures in the initial measurements. The general rule is to match the number of significant digits in the final result to the least precise measurement used in the calculation. This ensures that the final number is not artificially more accurate than the data supports.
Examples of Rounding in Practice
Here are some common scenarios where rounding is applied in scientific calculations:
| Scenario | Before Rounding | After Rounding |
|---|---|---|
| Measured quantity | 5.6789 | 5.68 (to 2 decimal places) |
| Calculated value | 0.004567 | 0.005 (rounded to 2 significant figures) |
| Concentration of a solution | 0.00012345 | 0.00012 (rounded to 3 significant figures) |
These examples demonstrate how rounding helps to simplify results while ensuring they remain within the appropriate level of precision for practical use.
Understanding Significant Figures in Scientific Calculations
Significant figures play a crucial role in ensuring the accuracy and reliability of numerical results in scientific work. They reflect the precision of measurements and calculations, indicating how closely a value is determined. Proper use of significant figures ensures that no false sense of accuracy is conveyed and that the data aligns with the limitations of the measurement tools used.
How to Identify Significant Figures
In general, significant figures include all non-zero digits, any zeros between non-zero digits, and trailing zeros in a decimal number. For example, in the number 0.00450, the digits 4, 5, and the trailing zero are considered significant. However, leading zeros are not counted, as they only serve to place the decimal point correctly.
Rounding and Its Role in Significant Figures
When performing calculations, rounding may be required to ensure the result reflects the appropriate number of significant figures. For instance, multiplying or dividing numbers mandates that the result should have the same number of significant figures as the number with the least amount. This practice maintains the accuracy of the data without overstating its precision.
How to Avoid Fractional Results in Scientific Calculations
In many scientific situations, dealing with fractional values can introduce unnecessary complexity. Converting fractions to whole numbers or applying rounding rules helps simplify calculations and ensures that the results remain practical for real-world applications. Avoiding fractional results is often necessary when precise measurements or simplified reporting are required.
Here are some strategies to avoid fractional outcomes:
- Round numbers at appropriate steps in the calculation to ensure the results align with the required precision.
- Use appropriate conversion factors to ensure the units work out to whole numbers when possible.
- Apply estimation techniques when dealing with large sets of data to reduce the need for precise fractions.
- In cases of repeated measurements, use averaging methods that naturally yield whole number results if the data allows.
By applying these methods, calculations can be kept straightforward, and results will be easier to interpret and apply, especially in practical tasks like measurements and solutions preparation.
The Role of Scientific Notation in Scientific Calculations
Scientific notation is a vital tool for simplifying the expression of very large or very small numbers. It helps streamline calculations by representing numbers in a more compact and manageable form. This method is especially useful in fields where dealing with extreme values is common, such as in physics, biology, and environmental science.
Benefits of Using Scientific Notation
There are several advantages to using scientific notation in scientific work:
- It simplifies complex calculations by reducing the number of zeros needed in large or small values.
- It helps maintain precision when working with quantities that vary significantly in scale.
- It makes it easier to perform operations such as multiplication and division, as exponents can be added or subtracted.
How to Apply Scientific Notation
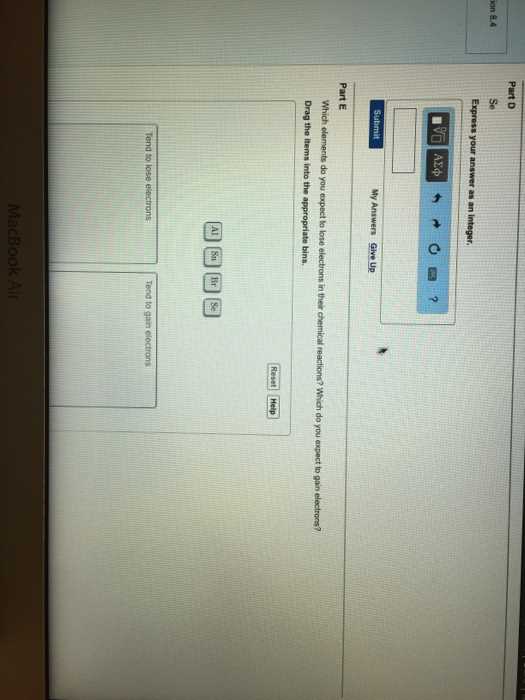
To use scientific notation effectively, follow these steps:
- Write the number as a product of a decimal between 1 and 10 and a power of 10.
- Ensure that the exponent reflects how many places the decimal has been moved to convert the number into this standard form.
- For very small numbers, use a negative exponent to indicate a value less than one.
Scientific notation makes it easier to handle large datasets and perform calculations efficiently without losing accuracy in measurement or computation.
Rounding Rules for Chemical Calculations
Rounding plays a critical role in ensuring that scientific results remain consistent with the precision of the measurements used. In various scientific disciplines, especially when working with numerical data, it is important to follow specific rules for rounding values to avoid overstating precision and to reflect the accuracy of the instruments and methods used. Proper rounding is essential for maintaining the reliability of experimental data and ensuring that calculations align with accepted scientific standards.
Basic Rounding Guidelines
When rounding values in chemical calculations, the following guidelines are generally followed:
- For addition and subtraction, the result should be rounded to the same number of decimal places as the measurement with the fewest decimal places.
- For multiplication and division, the result should be rounded to the same number of significant figures as the measurement with the fewest significant figures.
- When rounding a number with a digit 5 or greater, round up the last digit; otherwise, round down.
Examples of Rounding in Practice
Here are some examples of rounding rules applied in different situations:
- If the sum of two numbers is 4.758 and 1.2, the result should be rounded to 1 decimal place: 5.0.
- For a multiplication result of 3.205 × 4.2, the result should be rounded to 2 significant figures: 13.
By following these rules, calculations remain consistent with the precision of the initial measurements, ensuring reliable results that are both accurate and practical for further use.
Handling Complex Chemical Equations
Working with intricate equations often requires careful attention to ensure that each component is properly balanced and that the calculations reflect the correct proportions of reactants and products. In many cases, these equations involve multiple steps and require systematic methods to simplify and solve. Understanding how to manage these calculations effectively is crucial for obtaining reliable results in scientific work.
Here are some steps to manage and solve complex equations:
- Ensure all components are properly balanced before starting calculations. Each element must appear on both sides of the equation in equal amounts.
- Break down the equation into smaller, more manageable parts. Tackle one element or molecule at a time to avoid confusion.
- Use stoichiometric coefficients to ensure that the correct proportions of reactants and products are maintained throughout the process.
- In cases of simultaneous reactions, apply techniques such as substitution or elimination to solve for unknowns.
By following these guidelines, complex calculations can be approached methodically, minimizing errors and ensuring the accuracy of the final results.
Common Approaches to Simplification
In many cases, simplifying equations can make calculations easier and reduce the likelihood of mistakes. Here are some strategies to consider:
- Identify and cancel out common terms when possible to simplify the equation.
- Use approximations for constants or values that are difficult to measure directly, as long as they do not compromise the integrity of the result.
- When possible, perform dimensional analysis to ensure the consistency of units throughout the equation.
By applying these methods, handling complex equations becomes a more straightforward process, allowing for efficient and accurate problem-solving in scientific calculations.
Practical Examples of Whole Number Results
In scientific work, certain calculations are expected to yield whole numbers to simplify interpretation and application. These results often arise in scenarios where measurements or quantities are standardized or inherently fit into whole number values. Understanding how to approach such calculations can make it easier to handle real-world situations, where exact fractions or decimals might not be necessary or practical.
Example 1: Molar Ratios in Reactions
In a chemical reaction, the ratio of reactants to products can often be represented by whole numbers. For instance, when balancing a reaction, stoichiometric coefficients are determined in such a way that the amounts of substances involved are in whole-number multiples. This is important for ensuring that no fraction of a molecule is used in practical scenarios.
- For example, the reaction of hydrogen and oxygen to form water is often represented as 2H2 + O2 → 2H2O. Here, the number of molecules on both sides is balanced with whole numbers.
- In real applications, such as making a solution, these whole-number ratios ensure that the correct amounts of each substance are mixed together without fractions.
Example 2: Mass Relationships in Simple Compounds
When working with simple compounds, the mass of elements involved in a reaction is often expected to be in whole numbers after calculations. For instance, the molar mass of carbon dioxide (CO2) is calculated by adding the atomic masses of carbon and oxygen, both of which are whole numbers.
- If carbon weighs approximately 12 grams and oxygen weighs about 16 grams, the total molar mass of CO2 is 44 grams, a whole number that can be used directly in laboratory calculations.
- This simplifies the process of determining the mass of reactants and products in a given chemical process.
These examples demonstrate how simplifying to whole numbers ensures that results are practical, easier to understand, and directly applicable in real-world scientific tasks.
Using Moles and Avogadro’s Number
In scientific calculations, particularly in the field of material science and molecular studies, moles provide a bridge between the atomic scale and practical quantities. By using a fundamental constant, it becomes possible to relate microscopic measurements of atoms and molecules to macroscopic amounts of matter that can be measured in a laboratory. This relationship allows scientists to work with tangible amounts of substances while maintaining precision at the molecular level.
The Role of Moles in Calculations
The concept of the mole is central to understanding how to scale from atomic or molecular levels to the quantities of material handled in experiments. By knowing the number of particles per mole, scientists can determine how much of a substance is needed to achieve a particular reaction, create a solution, or measure a substance’s mass accurately.
For example, one mole of any substance contains approximately 6.022 x 1023 particles (atoms, molecules, or ions), a quantity known as Avogadro’s number. This allows scientists to calculate the mass of a sample based on its molecular weight and the number of moles in the sample.
Example Calculation Using Avogadro’s Number
Let’s consider an example where we need to calculate the number of molecules in a 2-gram sample of water. The molar mass of water (H2O) is approximately 18 grams per mole, and using Avogadro’s number, we can calculate the number of molecules present in the sample.
| Step | Calculation | Result |
|---|---|---|
| 1. Find the number of moles in 2 grams of H2O | 2 grams ÷ 18 g/mol | 0.111 moles |
| 2. Calculate the number of molecules in 0.111 moles | 0.111 moles × 6.022 x 1023 molecules/mole | 6.68 x 1022 molecules |
This calculation shows how moles and Avogadro’s number allow for the conversion of mass into the number of molecules, bridging the gap between the atomic and macroscopic worlds.
Converting Units for Integer Results
In scientific work, converting units correctly is essential for achieving precise and meaningful results. Whether it involves transforming measurements from one system to another or adjusting units within the same system, understanding how to apply unit conversions is key to ensuring that the results are both accurate and usable. This process becomes particularly important when the final outcome needs to be a whole number for clarity or practicality in experiments and calculations.
Unit conversions typically involve multiplying or dividing by a conversion factor, which allows for the direct translation between different units of measurement. However, when the goal is to achieve a whole number in the result, special attention must be paid to factors such as the precision of the conversion and the scaling of quantities. These details are especially relevant when dealing with measurements that involve quantities of material, such as mass, volume, or the number of particles.
Example of Unit Conversion in Mass
Consider a situation where the mass of a substance is given in milligrams, but the desired result is in grams. The conversion factor between milligrams and grams is 1 gram = 1000 milligrams. To convert a mass of 2500 milligrams to grams, divide by 1000:
- 2500 milligrams ÷ 1000 = 2.5 grams
In this case, the result is a decimal. If the goal is to express the mass as a whole number, one might round the result to 3 grams. However, when precision is required, keeping track of significant figures and proper rounding is crucial to maintain the integrity of the calculation.
Converting Volume for Practical Use
In another example, converting between liters and milliliters may require adjustments to ensure the final result aligns with practical requirements. If you need to convert 1500 milliliters into liters:
- 1500 milliliters ÷ 1000 = 1.5 liters
In many cases, rounding this to 2 liters might be necessary for ease of use in a laboratory setting, but it is important to understand the implications of such rounding on the accuracy of experimental outcomes.
Tools and Calculators for Accurate Results
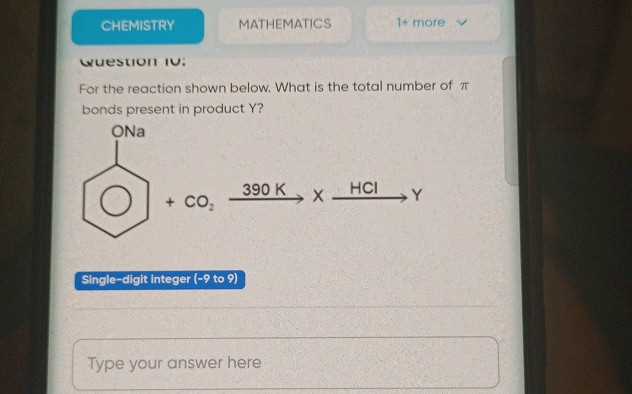
Accurate calculations are crucial in scientific work, and modern tools and calculators have made it easier than ever to achieve reliable outcomes. Whether performing complex conversions, determining molar masses, or adjusting for significant figures, using the right tools can help ensure that all measurements and calculations are precise. These tools help reduce human error and provide a systematic approach to obtaining results that are both correct and consistent.
From handheld calculators designed for scientific use to specialized online tools, there are a variety of resources available for those working in laboratory settings or conducting research. Many of these calculators include built-in functions for unit conversions, stoichiometric calculations, and error analysis, making them indispensable for everyday tasks in the field. Using these resources not only saves time but also enhances the accuracy of the data collected.
In addition to basic calculators, there are also advanced software tools that cater to specific needs, such as molecular weight determination, pH calculations, or reaction yield predictions. These digital resources often provide step-by-step solutions and can handle more complex mathematical operations, offering a level of precision that manual calculations might miss.
Understanding Precision in Chemical Data
In scientific research, particularly in laboratory environments, the accuracy of measurements and the consistency of data are paramount. Precision refers to the degree of reproducibility or consistency of a set of measurements under unchanged conditions. A higher degree of precision means that repeated measurements will yield similar results, even if they are not necessarily accurate. This is an important concept when dealing with large amounts of data or conducting experiments where small variations can significantly affect outcomes.
Importance of Consistency in Measurements
When performing experiments, precision is crucial for comparing results across different trials. Even slight inconsistencies can introduce errors that skew the interpretation of the data. This is particularly true when analyzing concentrations, reaction rates, or thermodynamic properties, where small deviations can alter the conclusions drawn from the experiment.
Factors Affecting Precision
Several factors contribute to the precision of measurements in a laboratory setting. These include the quality of the instruments used, the skill of the person conducting the measurements, and the stability of the environment. For example, fluctuations in temperature, pressure, or humidity can affect the outcome of sensitive measurements. Ensuring that all variables are controlled as much as possible is key to obtaining precise data.
High precision is especially important when working with highly sensitive chemical reactions or molecular structures. For these studies, even the smallest errors can lead to incorrect conclusions. As such, understanding how to measure and record data with precision is a fundamental skill in scientific practice.
Common Units in Chemistry and Their Integers
In scientific practice, especially in laboratory settings, measurements are expressed using various units, each corresponding to a particular physical quantity. These units provide a standardized way of representing quantities like mass, volume, temperature, and concentration. Some units are particularly important in chemical calculations and often require whole number values to maintain accuracy and simplicity, ensuring consistency in results.
For example, when working with gases, the amount of substance is typically measured in moles, a unit that allows scientists to relate the number of particles to macroscopic amounts of material. This number is often represented by an exact integer value, such as Avogadro’s number, which defines the number of atoms or molecules in one mole of any substance.
Common units like liters for volume, grams for mass, and moles for the amount of substance are essential in many calculations. These units form the basis for chemical formulas, balancing equations, and determining reaction yields. It is important to keep these units in mind when working with data, as rounding off or approximating certain values could introduce significant errors, especially when dealing with large quantities or highly sensitive measurements.
How Integer Results Simplify Chemical Work
Simplifying calculations in scientific work can lead to more efficient and accurate outcomes. In many cases, working with whole numbers rather than fractional values makes the entire process faster and less prone to errors. When the results of a calculation can be expressed as whole numbers, the focus shifts from complex fraction handling to straightforward application, improving both clarity and precision in chemical processes.
Here are a few key reasons why whole-number results are advantageous in laboratory work:
- Reduced Complexity: Avoiding fractions and decimals simplifies calculations, particularly when working with large datasets or performing repetitive tasks.
- Clear Communication: Using whole numbers enhances communication between team members and ensures that everyone is interpreting results consistently.
- Minimized Error Margin: Whole numbers often result in fewer rounding errors, which is especially important when measuring and mixing chemical substances.
- Faster Processing: When results are rounded to the nearest whole number, calculations can be completed more quickly, which is beneficial in time-sensitive research environments.
In practice, ensuring that outcomes are simplified to whole numbers where possible not only saves time but also enhances the overall reliability of the results in chemical experiments. Whether it’s calculating quantities for reactions, determining concentrations, or converting between units, the use of whole-number results provides a clearer path to accuracy.
Reviewing the Importance of Integer Results
The use of whole numbers in scientific calculations provides several advantages, particularly when dealing with precise measurements and ensuring consistency across various experiments. By focusing on clear, whole-number outcomes, professionals in the field can avoid unnecessary complexity and potential errors that arise from fractional values. This approach streamlines calculations, making data easier to interpret and apply in practical settings.
Key benefits of using whole numbers in scientific work include:
- Simplicity: Whole-number results reduce the need for continuous adjustments or conversions, allowing for quicker analysis and decision-making.
- Precision: Minimizing the use of decimals or fractions reduces the chances of introducing errors during calculation, enhancing the overall reliability of results.
- Consistency: When measurements and outcomes are consistently expressed as whole numbers, it ensures that data across multiple experiments or processes align with one another.
- Efficiency: Streamlined calculations and simplified results lead to more efficient workflow, especially in environments where speed and accuracy are critical.
In sum, the importance of using whole numbers lies not only in simplifying the process but also in providing a higher degree of reliability and clarity, especially when precise measurements are essential for successful outcomes. This approach is indispensable in laboratories, research settings, and industry applications, where accurate and reproducible results are paramount.