
Understanding the fundamental principles of mathematics is crucial for students aiming to build a strong foundation in problem-solving. In this section, we delve into the essential skills required to tackle a wide variety of equations and expressions. The focus is on simplifying complex problems and providing a clear approach to understanding solutions.
Each exercise is designed to help learners develop logical thinking and enhance their ability to approach challenges systematically. By studying the comprehensive explanations provided for each problem, students can gradually improve their mathematical proficiency and gain confidence in their abilities. Whether for homework, class practice, or test preparation, the solutions presented serve as a guide to mastering the content.
Prentice Hall Gold Algebra 1 Overview
This educational resource offers a structured approach to learning the essential techniques and methods necessary for solving mathematical problems. It is designed to guide students through a series of progressively more complex tasks, allowing them to build their understanding step by step. The material covers a wide range of topics, each crucial for developing a deep and comprehensive grasp of mathematical reasoning.
Structured Learning for Success
By breaking down each concept into manageable segments, the curriculum ensures that learners can follow along at their own pace. The explanations are clear, with ample examples provided to illustrate key ideas. Interactive exercises further reinforce the material, allowing students to practice what they have learned and test their skills.
Benefits of This Educational Approach
One of the key advantages of this method is its ability to cater to diverse learning styles. Whether students prefer visual aids, step-by-step instructions, or hands-on practice, the material accommodates a variety of preferences. This approach ensures that learners not only memorize formulas but also develop a deep understanding of the underlying principles that govern mathematical operations.
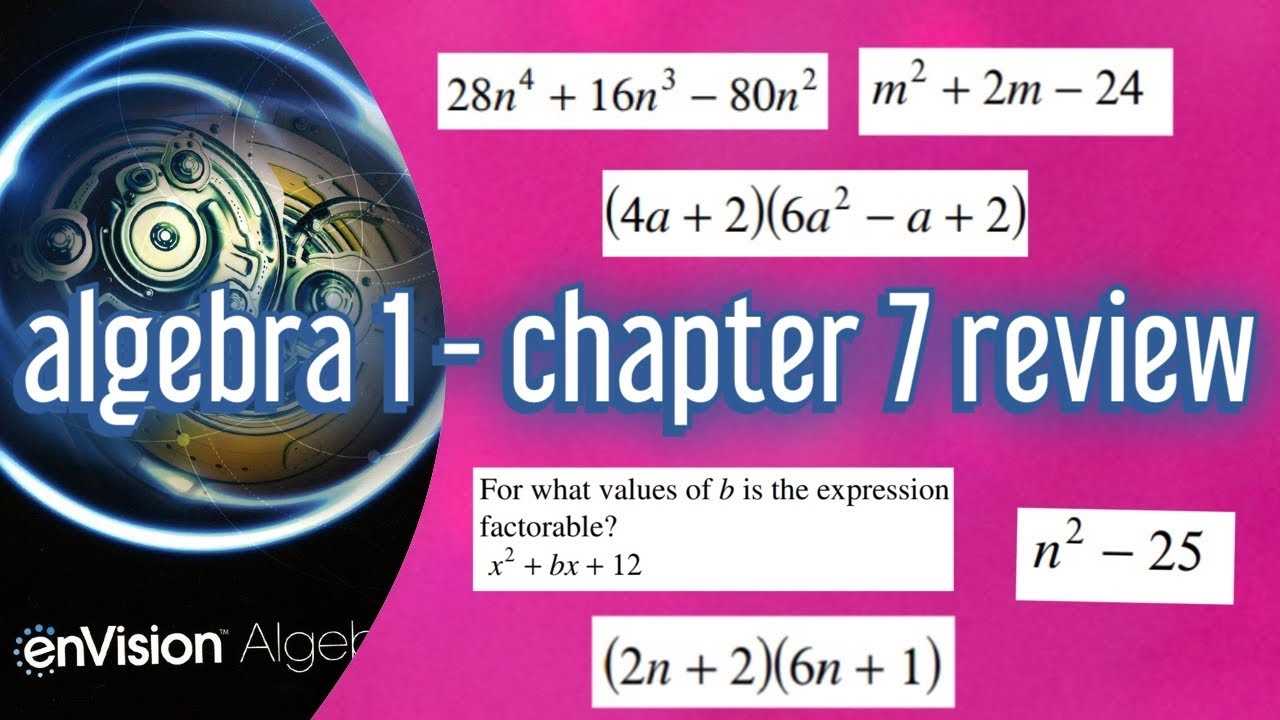
Importance of Chapter 7 in Algebra
The seventh section of this mathematics curriculum plays a pivotal role in shaping a student’s understanding of more advanced topics. It introduces concepts that build upon earlier lessons and provide a foundation for future studies. Mastery of these principles is essential for progressing in the subject and applying mathematical reasoning to real-world problems.
- Provides critical problem-solving techniques.
- Strengthens understanding of core mathematical concepts.
- Prepares students for more complex topics and applications.
In this section, learners explore foundational skills that are integral to tackling more sophisticated equations. The methods introduced here not only support academic growth but also foster practical skills for everyday use. Without a solid grasp of these essential topics, students may struggle to succeed in higher-level mathematical courses.
How to Use the Answer Key Effectively
Using a solution guide can greatly enhance your learning process, but it’s important to approach it with a strategic mindset. Simply looking at the solutions without understanding the reasoning behind them won’t help you fully grasp the material. Instead, take the time to analyze each solution and break down the steps involved in arriving at the correct answer.
| Step | Action | Purpose |
|---|---|---|
| 1 | Attempt the problem on your own first | Ensure independent thinking and problem-solving skills |
| 2 | Compare your solution with the provided one | Identify any mistakes or differences in your approach |
| 3 | Analyze the steps in the solution | Understand the reasoning behind each decision and method |
| 4 | Review any concepts you struggled with | Reinforce areas where you need additional practice |
| 5 | Apply the learned methods to new problems | Solidify your understanding and improve retention |
By following these steps, you will not only verify your work but also deepen your comprehension of the material. This approach ensures that you’re not just memorizing answers, but developing a stronger mathematical intuition and problem-solving ability.
Understanding the Key Concepts in Chapter 7
This section covers essential ideas that lay the groundwork for more advanced mathematical topics. Mastering these concepts is crucial for progressing in your studies, as they introduce new methods of problem-solving and help strengthen your understanding of the underlying principles. These fundamental skills are applicable not only in future lessons but also in real-world scenarios where logical thinking is required.
One of the key ideas in this section is equation manipulation. By learning how to manipulate expressions and solve for unknowns, students gain a deeper understanding of the relationships between variables. This process is vital for simplifying complex problems and finding solutions in a structured way.
Additionally, graphing techniques are explored, allowing students to visualize mathematical relationships. This skill helps in interpreting data, solving systems of equations, and analyzing trends, all of which are important for future coursework and practical applications.
Step-by-Step Solutions in Algebra 1
Breaking down complex problems into manageable steps is an essential approach for mastering mathematical techniques. A structured solution process allows students to focus on each individual part of the problem, ensuring they fully understand the reasoning behind each step. This method not only helps in solving specific exercises but also builds a strong foundation for approaching more advanced topics in mathematics.
Each solution follows a clear, logical progression. Starting with the initial equation, students are guided through the process of simplifying terms, applying operations, and solving for unknowns. By working through the steps methodically, learners can track their progress and identify where mistakes may occur, improving their problem-solving skills.
Common Mistakes and How to Avoid Them
While solving mathematical problems, it’s easy to fall into certain traps that can lead to incorrect results. Recognizing these frequent errors and learning how to avoid them is crucial for improving accuracy and efficiency. By understanding where mistakes commonly occur, students can be more mindful of their approach and develop stronger problem-solving skills.
- Misapplying Operations: Failing to properly distribute terms or forgetting to apply operations in the correct order often leads to errors. Always double-check your work to ensure each step follows logically from the previous one.
- Sign Errors: Miscalculating signs, especially when dealing with negative numbers, is a frequent issue. Take extra care when adding, subtracting, or multiplying negative values.
- Skipping Steps: Rushing through a solution can lead to skipped steps or incomplete work. Always work methodically through each part of the problem to ensure nothing is overlooked.
- Incorrect Substitution: Using incorrect values or substituting variables improperly can result in incorrect answers. Verify that each variable is substituted correctly before continuing.
- Overlooking Units: When working with real-world problems, it’s important to keep track of units of measurement. Always ensure that units are consistent throughout the calculation.
By being aware of these common mistakes, students can take proactive steps to avoid them and improve their overall mathematical proficiency. Regular practice and attention to detail will help in developing strong problem-solving habits and reducing errors over time.
Algebra 1 Tips for Students
Mastering mathematics can be challenging, but with the right strategies, students can develop a stronger understanding and confidence in their abilities. A few practical tips can make a significant difference when it comes to approaching problems efficiently and avoiding common pitfalls. Consistent practice, patience, and attention to detail are key to success in any mathematical course.
Effective Study Habits
- Practice Regularly: Consistent practice is essential for reinforcing concepts and developing problem-solving skills. Set aside dedicated time each day to work through practice problems.
- Break Down Complex Problems: Divide complicated tasks into smaller, manageable steps. This makes it easier to identify the correct approach and avoid feeling overwhelmed.
- Review Mistakes: When you make errors, take the time to understand why the solution didn’t work. Reviewing mistakes helps in avoiding them in the future and improves your learning process.
Time Management and Focus
- Prioritize Difficult Topics: Spend more time on the topics that challenge you the most. Don’t hesitate to seek extra help or resources to fully understand these areas.
- Avoid Multitasking: Focus solely on one problem at a time to ensure clarity and accuracy in your solutions. Multitasking can lead to careless mistakes.
- Stay Organized: Keep track of notes, assignments, and deadlines. An organized study routine allows you to stay on top of your work and reduces last-minute stress.
By applying these tips, students can enhance their mathematical abilities and build a solid foundation for more advanced topics. Remember, persistence is key, and improvement comes with regular effort and careful practice.
Exploring Key Formulas in Chapter 7
This section introduces several crucial mathematical formulas that are fundamental for solving a variety of problems. Understanding and mastering these formulas is essential for progressing in more complex topics and developing effective problem-solving strategies. By applying these formulas correctly, students can simplify their approach to solving equations and improve accuracy in their work.
One of the key formulas in this section involves working with linear equations, where the goal is to manipulate the variables in a consistent way to find their values. Another important concept is quadratic relationships, which require a different set of formulas for solving equations involving squared terms. These formulas are not just theoretical; they are designed to help students apply mathematical principles in real-world situations.
Mastering these formulas will not only help you in exams but also provide a solid foundation for tackling more advanced mathematical concepts in future courses.
Benefits of Using a Detailed Answer Key
Utilizing a comprehensive solution guide offers numerous advantages for students working through mathematical exercises. A detailed guide not only provides the correct answers but also walks through the reasoning behind each step, enabling learners to understand the process and improve their problem-solving skills. This approach fosters a deeper comprehension of mathematical concepts, helping students avoid common mistakes and solidify their grasp on the material.
One key benefit is the ability to learn from mistakes. When students compare their solutions with the step-by-step explanations, they can identify where they went wrong and adjust their methods. Additionally, a well-structured guide serves as a valuable tool for reinforcing concepts, as it offers clear examples and shows how to apply different techniques in various scenarios.
Furthermore, using such a resource promotes self-study and independence. By reviewing solutions independently, students can practice critical thinking and become more confident in their ability to solve similar problems in the future. This enhances both their learning experience and long-term retention of the material.
Practice Problems and Their Solutions
Working through practice problems is one of the most effective ways to reinforce mathematical concepts and build confidence in problem-solving skills. This section presents a set of problems designed to help students apply what they’ve learned and gain a deeper understanding of key principles. Each problem is followed by a detailed solution, showing the step-by-step process required to reach the correct answer.
Example Problem 1
Solve the following equation for x:
4x + 7 = 19
Solution
To solve for x, follow these steps:
- Subtract 7 from both sides: 4x = 12
- Divide both sides by 4: x = 3
The solution is x = 3.
Example Problem 2

Solve the quadratic equation:
x² – 5x + 6 = 0
Solution
To solve this quadratic equation, use factoring:
- Factor the equation: (x – 2)(x – 3) = 0
- Set each factor equal to zero: x – 2 = 0 or x – 3 = 0
- Solve for x: x = 2 or x = 3
The solutions are x = 2 and x = 3.
Additional Practice Problems

| Problem | Solution |
|---|---|
| 5x – 3 = 12 | x = 3 |
| x² + 4x + 3 = 0 | x = -1, x = -3 |
| 2(x + 4) = 14 | x = 3 |
By regularly practicing problems like these, students can enhance their understanding and gain the skills needed to tackle more complex challenges with ease.
Key Terms to Remember in Chapter 7
As you progress through this section, it’s crucial to familiarize yourself with several important terms that form the foundation of the concepts explored. Understanding these terms will not only help you tackle specific problems more effectively but also provide clarity in applying techniques across a variety of mathematical scenarios. Below, we outline the key terms that are essential for mastering this material.
1. Linear Equation
A linear equation represents a relationship between two variables where the highest exponent of the variable is 1. These equations are typically written in the form ax + b = c, where a, b, and c are constants, and x is the variable.
2. Quadratic Equation
A quadratic equation is an equation of degree 2, meaning the highest power of the variable is squared. It is generally expressed as ax² + bx + c = 0, where a, b, and c are constants. Solving these equations often involves factoring, completing the square, or using the quadratic formula.
3. Slope

The slope of a line describes how steep the line is. It is calculated as the change in the vertical direction divided by the change in the horizontal direction between two points on the line. The formula is m = (y₂ – y₁) / (x₂ – x₁), where m represents the slope and (x₁, y₁) and (x₂, y₂) are two points on the line.
4. Intercept
The intercept refers to the point where a line crosses the axis of a graph. There are two types: the y-intercept (where the line crosses the vertical axis) and the x-intercept (where the line crosses the horizontal axis). Identifying intercepts is crucial for graphing linear equations.
By remembering and understanding these terms, you’ll be better equipped to navigate through the problems and concepts in this section with confidence and clarity.
Visual Aids to Support Learning Algebra
Using visual tools can significantly enhance understanding and retention of mathematical concepts. These resources help learners translate abstract ideas into more tangible forms, making complex topics easier to grasp. By incorporating diagrams, graphs, and charts, students can visualize relationships, patterns, and solutions, which supports both problem-solving and conceptual learning.
For instance, graphical representations of equations can clarify how different variables interact. Additionally, tables can be used to organize data, offering a clear view of trends and relationships. Furthermore, visual models like number lines and geometric shapes provide a concrete foundation for abstract concepts, aiding students in connecting theory with practical examples.
Whether it’s plotting a line on a graph or using a chart to compare values, visual aids play a crucial role in making mathematical learning more accessible. They not only support comprehension but also encourage active engagement and critical thinking, making them invaluable tools for mastering complex material.
How Algebra 1 Prepares for Advanced Topics
Foundational concepts learned in earlier courses lay the groundwork for more complex mathematical topics in higher education. A solid understanding of fundamental operations, equations, and expressions provides the tools needed to tackle more challenging problems in fields such as calculus, statistics, and beyond. Mastering these basic principles ensures that students can navigate abstract concepts with ease and confidence as they progress.
Key skills developed in this stage, such as solving linear and quadratic equations, understanding functions, and interpreting graphs, are directly applicable to higher-level topics. These concepts serve as building blocks for more advanced subjects, enabling learners to understand patterns, structures, and relationships in more intricate mathematical systems.
To give an example of how these foundational principles support more complex areas, consider the following table that compares basic topics with their applications in advanced areas:
| Basic Topic | Advanced Topic Application |
|---|---|
| Linear equations | Calculus: Derivatives and slope analysis |
| Quadratic equations | Pre-calculus: Solving polynomial functions |
| Graphing functions | Statistics: Data analysis and function fitting |
| Systems of equations | Linear Algebra: Solving matrix equations |
By mastering the fundamental principles in this stage, students are equipped to handle more advanced topics with greater ease, setting them up for success in their academic journey.
Using Online Resources for Extra Help
In today’s digital age, there is an abundance of online resources available to assist students who need extra support with their studies. These platforms provide a wide range of materials, from instructional videos and tutorials to interactive practice problems and forums for peer discussions. Utilizing these resources can greatly enhance understanding and provide additional practice for mastering difficult concepts.
For students struggling with specific topics, websites offering step-by-step explanations and visual aids can be particularly helpful. These platforms often break down complex material into simpler, more digestible pieces, allowing learners to grasp key ideas at their own pace. Interactive tools such as virtual tutors, quizzes, and simulations are also valuable for reinforcing learning and testing comprehension.
Additionally, online forums and study groups can foster a collaborative learning environment where students can ask questions and discuss challenging problems with peers or instructors. This can create a sense of community and reduce the feeling of isolation that often accompanies individual study.
By combining these resources with traditional learning methods, students can build a well-rounded approach to mastering difficult material and overcome obstacles more effectively.
Time Management for Algebra Homework
Effectively managing time when tackling homework is essential for student success, especially when the material becomes more challenging. Planning and prioritizing tasks can help ensure that students complete assignments on time without feeling overwhelmed. Developing a good time management strategy can reduce stress and enhance understanding of the content.
Tips for Effective Time Management
- Set Specific Goals: Break down the homework into smaller, manageable tasks. Set clear, realistic goals for each study session.
- Use a Timer: Work in focused intervals (e.g., 25-30 minutes) followed by short breaks to maintain focus and avoid burnout.
- Prioritize Difficult Tasks: Start with the most challenging problems, as they require more concentration and energy.
- Eliminate Distractions: Find a quiet space to work, and turn off phone notifications or other distractions during study sessions.
- Review and Reflect: At the end of each session, review the work completed and assess your progress before taking a break or moving to the next task.
Staying Consistent
Time management is about consistency. Make a schedule that includes dedicated study periods and stick to it. Regular practice will build a routine and help make studying more efficient over time. Whether you prefer working early in the morning or late at night, find what works best for you and maintain that schedule to improve productivity and retention.
Preparing for Algebra 1 Tests and Exams
Preparing for a major exam or test requires a structured approach to ensure mastery of the material. It’s essential to focus not only on memorization but also on understanding the underlying concepts. By developing effective study habits and strategies, students can confidently approach their assessments and perform at their best.
Study Strategies for Success
- Start Early: Begin reviewing the material well in advance. Cramming the night before is less effective than consistent, early preparation.
- Understand Key Concepts: Make sure you understand the core ideas and how they connect with each other, rather than simply memorizing formulas or procedures.
- Practice Regularly: Solve practice problems to reinforce your knowledge and identify areas that need more attention. Regular practice will help you improve both speed and accuracy.
- Use Study Aids: Take advantage of study guides, past tests, and other resources to familiarize yourself with the format of the exam and the types of questions you’ll encounter.
- Review Mistakes: Review incorrect problems to understand your mistakes. This will help you avoid similar errors on the test.
Test-Taking Tips

- Manage Your Time: During the exam, allocate time to each section based on its weight. Don’t spend too much time on one question.
- Read Instructions Carefully: Before starting each section, take a moment to read through the instructions thoroughly to avoid mistakes.
- Stay Calm and Focused: If you encounter a challenging question, stay calm and move on to the next one. You can always come back to it later.
- Double-Check Your Work: If time permits, go back through your answers to ensure that you didn’t overlook any mistakes.
By incorporating these strategies and techniques, students can approach their exams with confidence, leading to better outcomes and a deeper understanding of the material.