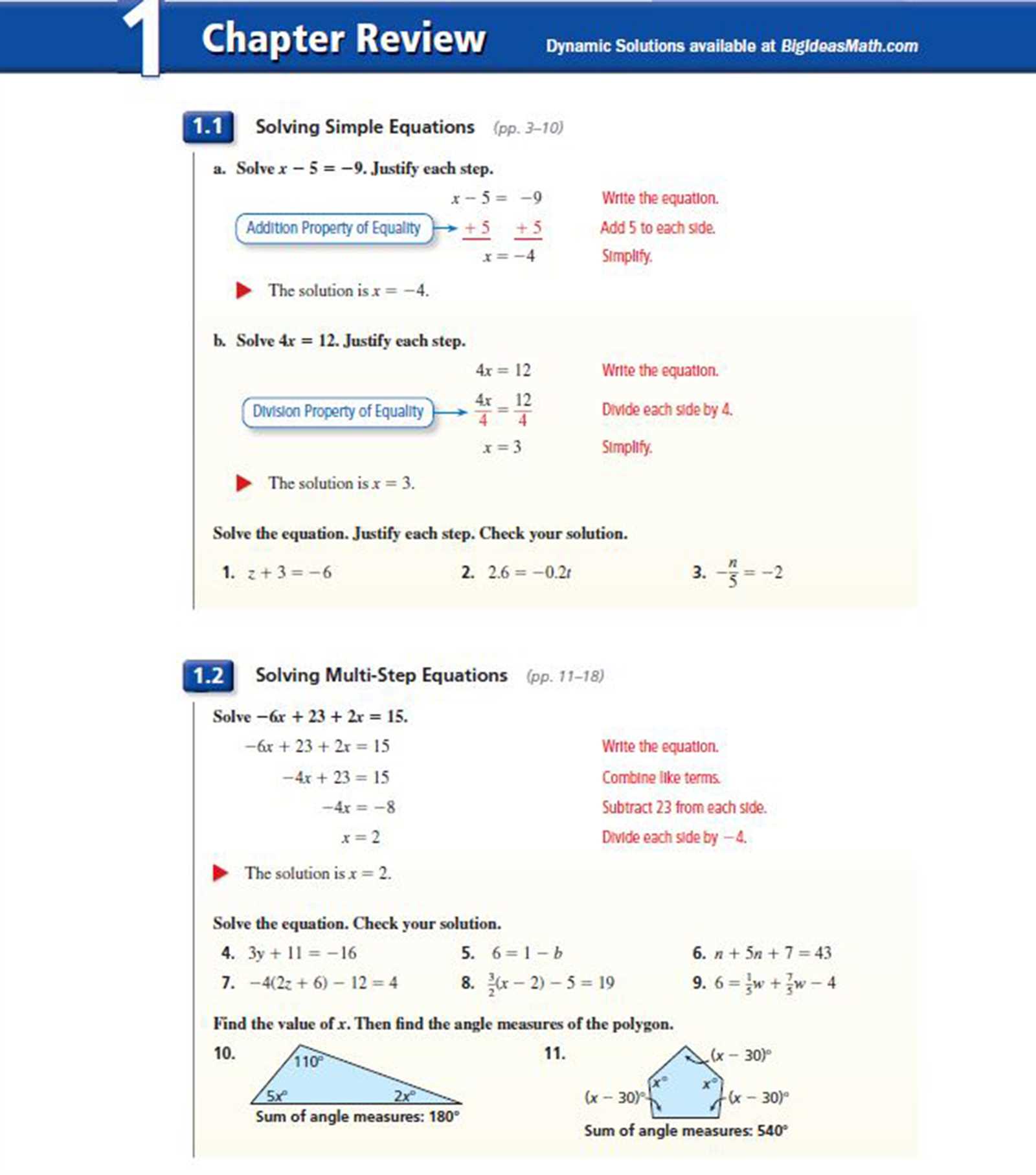
Mastering the fundamentals of mathematical operations is crucial for building a strong foundation in solving various types of problems. In this section, we explore key topics that form the backbone of early math education, providing a solid understanding of core techniques and methods.
Through a combination of clear explanations and practical examples, we aim to help learners grasp essential skills. By breaking down each concept, students can gain confidence and tackle more complex problems with ease. Understanding how to approach each problem logically and systematically will improve accuracy and speed in problem-solving.
Whether you’re revisiting foundational material or diving into new topics, this guide is designed to offer clarity and support. Developing strong problem-solving abilities ensures success in more advanced mathematical studies and applications.
Algebra 1 Chapter 1 Review Answers
In this section, we will explore the essential problem-solving techniques that form the foundation of early mathematical studies. By breaking down key operations and offering step-by-step guidance, we aim to strengthen understanding and build confidence in tackling related exercises.
Here, you will find various practice problems with detailed solutions. The goal is to help you understand each concept fully, ensuring you can apply these methods effectively in different contexts. The following table highlights common types of problems and their solutions:
| Problem Type | Example | Solution |
|---|---|---|
| Simple Equations | x + 5 = 12 | x = 7 |
| Linear Expressions | 2x – 3 = 7 | x = 5 |
| Word Problems | Twice a number plus 4 is 18. What is the number? | x = 7 |
| Fractions | 1/2x = 3 | x = 6 |
By reviewing these examples, you can strengthen your problem-solving skills and prepare for more complex challenges in future lessons. Each solution is designed to reinforce key strategies that will help you succeed in similar exercises.
Understanding the Basics of Algebra
In this section, we focus on the fundamental concepts that are essential for tackling various mathematical problems. A solid grasp of these foundational principles is crucial for progressing to more advanced topics and developing problem-solving abilities.
The basic building blocks include variables, constants, and operations that allow for the creation and manipulation of expressions and equations. Understanding how these elements interact will provide a strong foundation for solving a wide range of problems. Below is a table outlining some of the core elements of these concepts:
| Concept | Definition | Example |
|---|---|---|
| Variable | A symbol, often a letter, representing an unknown value. | x = 5 |
| Constant | A fixed value that does not change. | 3, -7 |
| Expression | A combination of variables, constants, and operations. | 2x + 3 |
| Equation | A mathematical statement where two expressions are equal. | x + 4 = 7 |
By understanding how to work with these elements, students can confidently approach a variety of mathematical challenges. Mastery of these basics sets the stage for more complex problem-solving techniques that will be explored in future lessons.
Key Concepts in Chapter 1
This section covers the fundamental ideas and methods that form the basis for solving a variety of mathematical problems. Understanding these core concepts will provide the necessary tools for successfully working through equations and expressions in subsequent lessons.
Essential Operations and Their Functions
The first step in tackling mathematical problems is becoming comfortable with the basic operations such as addition, subtraction, multiplication, and division. These operations are combined to create expressions and solve for unknown variables. Below is a table that illustrates how each operation is applied:
| Operation | Example | Result |
|---|---|---|
| Addition | 3 + 5 | 8 |
| Subtraction | 7 – 4 | 3 |
| Multiplication | 2 × 6 | 12 |
| Division | 10 ÷ 2 | 5 |
Understanding Variables and Expressions
Another key concept is working with variables and expressions. Variables represent unknown values that need to be determined, while expressions are combinations of variables and constants connected by operations. The following table highlights examples of simple expressions:
| Expression | Example | Explanation |
|---|---|---|
| Linear Expression | 2x + 3 | Expression involving a variable and constant |
| Simple Equation | x + 4 = 7 | Equation that represents a balance between two expressions |
| Fractional Expression | 1/2x | Expression involving a fraction and a variable |
Mastering these concepts is essential for understanding how to manipulate equations and solve for unknowns in more complex problems.
Solving Linear Equations Step by Step
Solving linear equations involves isolating the unknown variable to find its value. This process requires applying a set of straightforward steps, using inverse operations to simplify the equation. By carefully following the correct order of operations, it becomes easier to determine the value of the variable.
Understanding the Process
The goal of solving an equation is to get the variable on one side of the equation while keeping the constants on the other. This is done by performing equivalent operations on both sides, ensuring the equation remains balanced. Here’s an example to illustrate:
| Equation | Step 1 | Step 2 | Solution |
|---|---|---|---|
| x + 5 = 12 | Subtract 5 from both sides | x = 7 | x = 7 |
Applying Operations to More Complex Equations
For equations involving more than one term with the variable, you can apply the same method. Start by simplifying both sides and then solve for the unknown. Here’s another example:
| Equation | Step 1 | Step 2 | Solution |
|---|---|---|---|
| 3x – 4 = 11 | Add 4 to both sides | 3x = 15 | x = 5 |
By consistently applying these steps, even more complex problems become manageable. Understanding and practicing these techniques is essential for gaining proficiency in solving equations.
Understanding Variables and Constants
In mathematics, working with variables and constants is essential for forming and solving equations. Variables represent unknown values that we seek to determine, while constants are fixed numbers that remain unchanged throughout the problem-solving process. Both play a crucial role in creating mathematical expressions and equations.
Variables are typically represented by letters, such as “x” or “y,” and their values can vary depending on the context of the problem. Constants, on the other hand, are specific numbers, like 3 or -7, that do not change. These elements come together in expressions, where the variable interacts with the constant through mathematical operations.
Understanding the difference between variables and constants helps in manipulating equations, isolating unknowns, and ultimately solving problems efficiently.
Identifying Expressions and Equations
Recognizing the difference between expressions and equations is a fundamental skill in mathematics. Both involve variables and constants, but their structure and purpose are distinct. Expressions represent combinations of numbers, variables, and operations, while equations set two such expressions equal to each other.
In an expression, there is no equality sign. It simply shows a relationship between variables and constants, which can be simplified or evaluated. On the other hand, an equation shows that two expressions are equal, requiring a solution for the unknown variable. Understanding this difference allows for a clear approach when solving problems.
Distinguishing between these two forms is the first step in mastering problem-solving techniques and building confidence in handling mathematical challenges.
Working with Simplification Techniques
Simplification is an essential technique used to make mathematical expressions more manageable. By applying specific rules and strategies, complex expressions can be reduced to simpler forms, making it easier to solve for unknowns or analyze relationships. This process helps to eliminate unnecessary complexity and enhances clarity when working through problems.
Combining Like Terms
One of the most common simplification methods is combining like terms. Like terms are terms that have the same variable raised to the same power. For instance, in the expression 3x + 5x, both terms involve the variable “x” and can be combined to form a simpler expression:
| Expression | Simplification | Result |
|---|---|---|
| 3x + 5x | Combine like terms | 8x |
Distributive Property
Another important simplification method is the distributive property, which allows you to remove parentheses by multiplying each term inside by the factor outside. For example, in the expression 2(3x + 4), you can distribute the 2 to both terms inside the parentheses:
| Expression | Distributive Property | Result |
|---|---|---|
| 2(3x + 4) | Distribute the 2 | 6x + 8 |
Mastering these techniques is key to efficiently simplifying and solving expressions. The more familiar you become with these methods, the quicker and more accurately you will handle mathematical problems.
Common Mistakes in Algebra 1
When working with mathematical expressions and equations, it’s easy to make certain errors that can lead to incorrect solutions. Recognizing these common mistakes can help prevent confusion and improve accuracy. Many of these mistakes stem from misunderstanding basic concepts or overlooking important steps in problem-solving.
Forgetting to Apply the Correct Operations
One of the most frequent mistakes occurs when the correct operations are not applied consistently. For example, when solving equations, it’s crucial to apply inverse operations to isolate the variable. Skipping steps or reversing the order of operations can lead to incorrect results. Below is an example of a common error:
| Equation | Incorrect Step | Correct Step |
|---|---|---|
| x + 4 = 10 | Subtract 10 from both sides | Subtract 4 from both sides |
Misunderstanding Negative Signs
Another common mistake involves handling negative signs incorrectly. This can occur when subtracting or multiplying terms with negative values. For instance, in expressions involving two negative numbers, students may mistakenly think the result is negative when it is positive. Here’s an example of how this mistake might appear:
| Expression | Incorrect Calculation | Correct Calculation |
|---|---|---|
| -3 × -4 | -12 | +12 |
Avoiding these errors requires careful attention to detail and a solid understanding of fundamental operations. By practicing the correct methods, you can improve your problem-solving skills and achieve more accurate results.
Graphing Solutions to Equations

Graphing is an effective method to visually represent solutions to mathematical problems. By plotting points on a coordinate plane, one can see how the values of variables relate to one another. This approach provides a clearer understanding of the relationship between the equation and its solutions, allowing you to identify patterns and trends.
When graphing the solution to an equation, each solution corresponds to a point on the graph. For linear equations, this typically results in a straight line, where the variable values meet certain conditions set by the equation. The x- and y-values are plotted as coordinates, and the graph displays how they interact.
To graph a solution, begin by selecting values for the variable and plotting the corresponding points on a graph. Once enough points are plotted, draw a line or curve through them to represent the equation. This visual representation helps in understanding how changing one variable affects the other.
Practice Problems with Solutions
Working through practice problems is a great way to reinforce understanding and improve problem-solving skills. By solving a variety of exercises, learners can test their knowledge, identify areas of difficulty, and apply learned concepts. Below are some example problems followed by step-by-step solutions to help clarify the process.
Problem 1
Solve for x: 2x + 5 = 15
Solution: To solve this equation, first subtract 5 from both sides:
| Step | Equation |
|---|---|
| Subtract 5 from both sides | 2x = 10 |
| Divide both sides by 2 | x = 5 |
Problem 2
Solve for y: 3y – 4 = 8
Solution: First, add 4 to both sides:
| Step | Equation |
|---|---|
| Add 4 to both sides | 3y = 12 |
| Divide both sides by 3 | y = 4 |
By practicing problems like these, learners can build confidence in their ability to solve equations accurately and efficiently.
How to Approach Word Problems
Word problems can often seem daunting at first, but with the right approach, they become much more manageable. The key to solving these types of problems is to break down the information step by step and translate it into mathematical expressions. Here are some effective strategies to help you tackle word problems more confidently:
- Read the Problem Carefully: Understand the scenario being described and identify the key pieces of information provided.
- Identify What You Need to Find: Determine what the problem is asking you to solve for, whether it’s a specific variable or an unknown quantity.
- Translate Words into Mathematical Expressions: Convert the given information into an equation or inequality. Look for words that indicate operations (such as “sum,” “difference,” or “product”).
- Choose a Strategy: Decide on the best method to solve the problem, whether it involves solving an equation, drawing a diagram, or using logical reasoning.
- Check Your Work: Once you’ve solved the problem, revisit the original question to ensure your solution makes sense and is reasonable.
By following these steps, you’ll be able to approach word problems systematically and improve your ability to solve them correctly and efficiently.
Solving Systems of Equations
When dealing with multiple equations that involve two or more variables, the goal is to find values for these variables that satisfy all the equations simultaneously. This is known as solving a system of equations. There are several methods to approach such problems, and choosing the right one depends on the specific system you’re working with. Below are the common methods used to solve systems of equations.
Method 1: Substitution
The substitution method involves solving one equation for one variable and then substituting this expression into the other equation. This eliminates one of the variables, making the system easier to solve.
- Choose one of the equations and solve for one variable.
- Substitute this expression into the other equation.
- Solve for the remaining variable.
- Substitute the value of the variable back into the original equation to find the other variable.
Method 2: Elimination
The elimination method involves adding or subtracting the equations in such a way that one of the variables is eliminated. This method is especially useful when the coefficients of one of the variables are the same or opposites in both equations.
- Multiply one or both equations to align the coefficients of one variable.
- Add or subtract the equations to eliminate one variable.
- Solve for the remaining variable.
- Substitute the value of the variable into one of the original equations to find the other variable.
Both methods will lead to the solution, but the choice of method may vary depending on the specific system you are solving.
Understanding Inverse Operations
Inverse operations are fundamental tools that help to reverse the effect of a mathematical operation. They are crucial when solving equations, as they allow us to isolate variables and simplify expressions. Inverse operations “undo” each other, providing a way to solve for unknowns by eliminating terms systematically. This section will explore how these operations work and how to apply them in solving problems.
Key Pairs of Inverse Operations
Each operation has a corresponding inverse. Here are the most common pairs:
- Addition and Subtraction: The inverse of addition is subtraction. For example, if you add 5 to a number, you can reverse it by subtracting 5.
- Multiplication and Division: The inverse of multiplication is division. If you multiply a number by 3, you can reverse it by dividing by 3.
- Exponentiation and Root Extraction: Raising a number to a power (exponentiation) and extracting a root are inverse operations. For instance, squaring a number can be undone by taking its square root.
Using Inverse Operations to Solve Equations
Inverse operations are often used to isolate variables in equations. Here’s how they help:
- Start with the equation that includes the unknown variable.
- Identify the operation being performed on the variable.
- Apply the inverse operation to both sides of the equation to simplify it.
- Repeat this process until the variable is isolated.
By applying inverse operations step by step, you can systematically solve equations and find the value of unknown variables.
Using Substitution in Algebra
Substitution is a powerful technique used to solve systems of equations by replacing one variable with an expression that relates to another. This method simplifies the process by reducing the number of variables in one equation, making it easier to solve. Substitution is especially useful when one equation can be solved for a single variable, allowing it to be substituted into the other equation(s) in the system.
The key idea behind substitution is to isolate one variable in one of the equations and then substitute that expression into the other equation. This results in a simpler equation with only one variable, which can then be solved directly. Once that variable is found, the value can be substituted back into one of the original equations to find the value of the other variable.
Steps for Using Substitution
Follow these steps to solve a system using substitution:
- Step 1: Solve one equation for one variable (either x or y).
- Step 2: Substitute this expression into the other equation.
- Step 3: Solve the resulting equation for the remaining variable.
- Step 4: Substitute the value of the solved variable back into the original equation to find the other variable.
Substitution can be particularly effective when dealing with linear systems or equations where one of the variables is easy to isolate. By following these steps, you can systematically work through problems and find solutions with ease.
Why Order of Operations Matters
The order in which mathematical operations are performed is crucial to obtaining the correct result. Without a clear structure, the same expression could be interpreted in multiple ways, leading to different answers. To avoid confusion and ensure consistency, there are established rules that govern how operations should be carried out.
The main principle behind the order of operations is that certain calculations need to be done before others. This ensures that every equation is solved in a standardized way, making it easier to communicate and verify results. Following the proper sequence prevents errors and ensures that everyone solving the problem arrives at the same answer.
Common Operations and Their Order
The typical order in which operations are performed is represented by the acronym PEMDAS, which stands for:
- Parentheses – Operations inside parentheses are done first.
- Exponents – Powers an
Exploring Absolute Value Problems
Understanding how to solve problems involving the distance from zero is an essential skill in mathematics. These problems focus on the concept of magnitude, which is always positive, regardless of the direction or sign of the number. This allows for the expression of both positive and negative values in a simplified, uniform way.
Absolute value problems are commonly used to represent situations where only the size of a quantity matters, not its direction. Whether dealing with financial data, temperature changes, or distances, this concept helps to eliminate any negative signs and focus on the numerical magnitude of values.
Solving Absolute Value Equations
To solve an equation involving absolute value, we need to consider both possible outcomes: the positive and negative solutions. Here’s a general approach:
- First, isolate the absolute value expression on one side of the equation.
- Next, split the equation into two cases: one where the value inside the absolute value is positive and another where it is negative.
- Finally, solve each case separately and combine the solutions to find all possible answers.
Example Problem
Let’s solve the equation: |x – 3| = 7
- Case 1: x – 3 = 7 ⟶ x = 10
- Case 2: x – 3 = -7 ⟶ x = -4
Thus, the solutions to the equation are x = 10 and x = -4.
By understanding and practicing absolute value problems, you’ll be better equipped to handle a variety of real-world scenarios where magnitude plays a crucial role in the outcome.
Reviewing Fractional Equations
Working with equations involving fractions can initially seem challenging, but once you understand the steps involved, they become much more manageable. These equations often include variables in the denominator and require specific techniques to solve. The goal is to eliminate the fractions and simplify the equation, making it easier to isolate the variable and solve for its value.
To solve fractional equations, one of the most effective methods is multiplying both sides of the equation by the least common denominator (LCD). This step helps to eliminate the fractions and allows you to work with a simpler equation. Let’s break down the process with a few examples.
Step-by-Step Guide
- Step 1: Identify the fractions and their denominators.
- Step 2: Find the least common denominator (LCD) of all the denominators in the equation.
- Step 3: Multiply both sides of the equation by the LCD to eliminate the fractions.
- Step 4: Simplify the resulting equation and solve for the variable.
Example Problem
Let’s solve the equation: 1/x + 2/3 = 4
- Step 1: The denominators are x and 3.
- Step 2: The least common denominator (LCD) is 3x.
- Step 3: Multiply both sides of the equation by 3x>:
3x * (1/x) + 3x * (2/3) = 3x * 4 - Step 4: Simplify the equation:
3 + 2x = 12x - Step 5: Solve for x>:
3 = 10x, thus x = 3/10.
By following these steps, we can successfully solve fractional equations and avoid the complexity of dealing with fractions directly throughout the process. Practice and understanding the proper steps are key to mastering these types of problems.
Preparing for Upcoming Topics
As you advance in your studies, it’s important to lay a strong foundation for the next set of concepts you will encounter. Preparing for upcoming material involves understanding the key ideas that will build upon what you’ve already learned. This approach ensures that when more complex topics arise, you’ll be ready to tackle them with confidence.
In this stage, focus on refining your skills and reinforcing the basics. Practicing essential techniques and ensuring that you’re comfortable with core principles is crucial for making the transition to more advanced material seamless. Let’s explore some strategies to help you stay ahead and maximize your learning potential.
Focus on Core Concepts
- Review key operations: Make sure you are comfortable with fundamental mathematical operations, as these will serve as the building blocks for more complex problems.
- Understand the language: Familiarize yourself with the terminology and notation that is often used in upcoming lessons. This will help you grasp new concepts more easily.
- Practice problem-solving: Work through a variety of problems to gain fluency in applying techniques to different situations. Consistent practice is key to mastering the material.
Strategies for Effective Learning
- Stay organized: Keep track of formulas, rules, and methods you’ve learned. This will serve as a quick reference when needed.
- Ask questions: If you’re unsure about certain concepts, seek clarification. Understanding early can save you time later on.
- Work with others: Collaborating with peers can provide different perspectives on solving problems and enhance your understanding.
By focusing on these strategies, you’ll be better equipped to tackle new challenges and move forward with ease. The key is consistent practice, clarity of concepts, and preparing yourself mentally for the material ahead.