As the time approaches for your upcoming challenge in mathematical reasoning, it’s essential to focus on the critical concepts and methods that will help you succeed. This section will guide you through a strategic review of the most important areas, ensuring you are well-prepared to tackle various problems confidently and efficiently.
The key to excelling lies in mastering fundamental principles, understanding complex relationships between figures, and solving problems with precision. This guide is designed to provide a structured approach to help you enhance your problem-solving abilities and solidify your understanding of the material.
By revisiting the core topics and practicing essential techniques, you will gain the necessary skills to perform well. The next steps will focus on reinforcing knowledge, identifying areas of weakness, and offering effective strategies for approaching different types of questions.
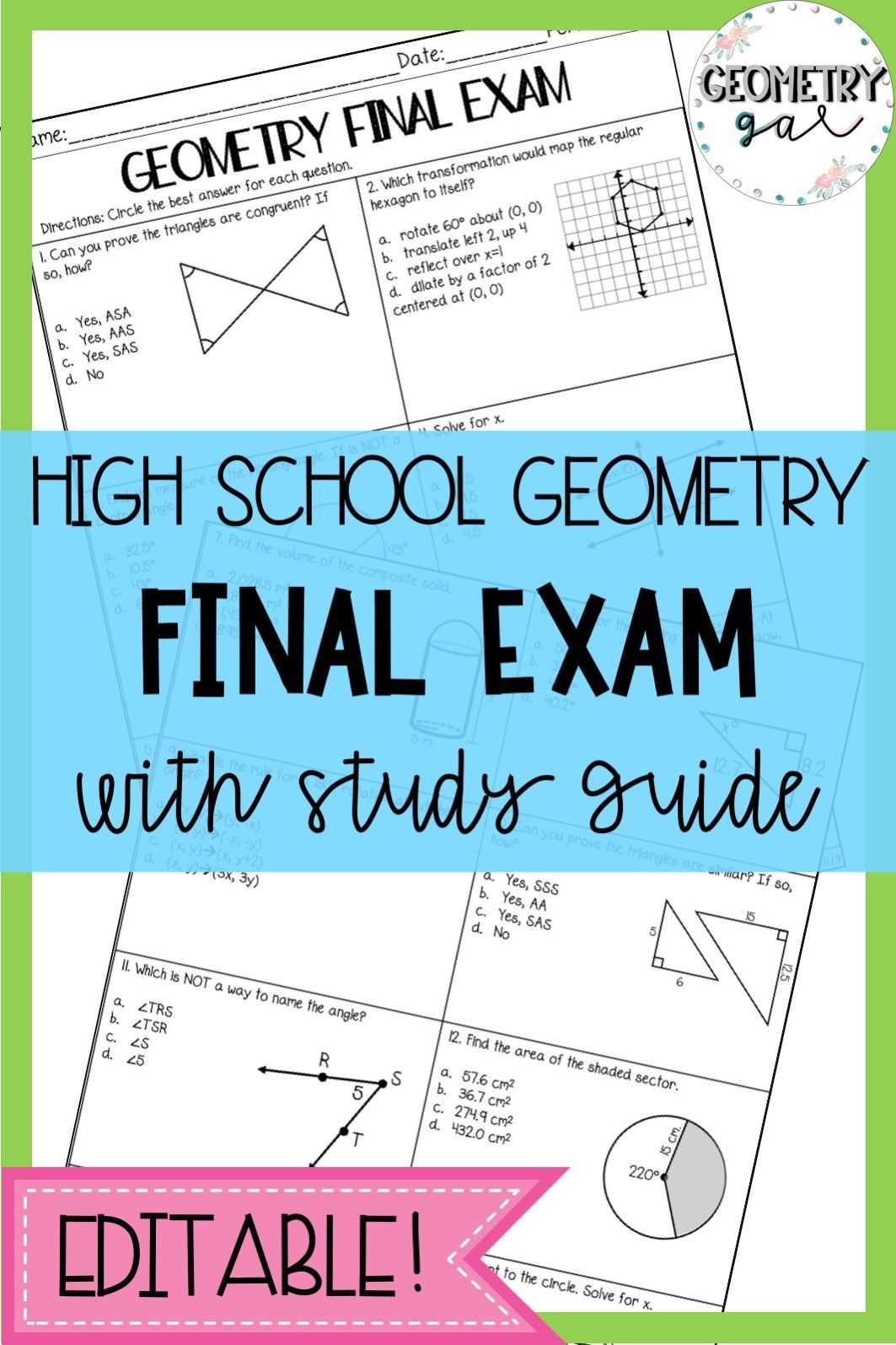
Geometry Final Exam Review Packet
Preparing for an important assessment requires a systematic approach to consolidate your understanding of key topics. A comprehensive study guide helps focus your efforts on the most crucial areas, ensuring you’re well-equipped to handle various problems and scenarios with ease.
This guide covers essential mathematical concepts, from basic principles to more complex problem-solving techniques. By breaking down each section into manageable chunks, it enables you to address each topic effectively, reinforcing your knowledge and boosting your confidence.
To maximize your success, this collection of exercises and explanations provides targeted practice, allowing you to familiarize yourself with typical question formats. Whether you need to revisit basic formulas or refine your reasoning skills, this material offers a well-rounded approach to preparation.
Understanding Key Geometry Concepts
Mastering fundamental principles is crucial for success in any mathematical discipline. A deep understanding of core concepts such as shapes, angles, and spatial relationships forms the foundation for solving complex problems. By focusing on these essential elements, you can approach questions with clarity and precision.
Start by revisiting basic elements like points, lines, and planes. These building blocks are used to define more advanced shapes and structures. Additionally, an in-depth grasp of the properties of triangles, quadrilaterals, and circles will allow you to solve a wide range of problems effectively.
Understanding formulas for areas, volumes, and perimeters is also key to solving real-world problems. While some of these may seem straightforward, knowing when and how to apply them correctly is essential for accurate results. Practice using these formulas in different contexts to solidify your understanding.
As you progress, comprehending proofs becomes increasingly important. This skill allows you to logically connect various elements and demonstrate the validity of your reasoning. By refining your ability to create structured arguments, you will strengthen your overall problem-solving ability.
Essential Formulas for Geometry Exams
One of the most effective ways to succeed in any mathematical assessment is by mastering the key formulas that govern shapes, sizes, and spatial relationships. These formulas serve as tools to calculate areas, volumes, and other important properties, helping you solve problems efficiently and accurately.
Area and Perimeter Formulas
Understanding the formulas for finding areas and perimeters of various shapes is essential. For instance, the area of a rectangle is calculated by multiplying length by width, while the area of a triangle is half the base times the height. Similarly, the perimeter of a polygon is the sum of the lengths of its sides. These basic formulas are foundational for many types of questions.
Volume and Surface Area Formulas
For three-dimensional shapes, it’s important to know how to calculate volume and surface area. For example, the volume of a sphere is given by the formula V = (4/3)πr³, and the surface area of a cylinder is A = 2πr(h + r). These formulas will help you determine the size and surface characteristics of objects in space, which is crucial for solving many types of problems.
Practice Problems for Geometry Mastery
Practicing a variety of problems is crucial to mastering mathematical concepts and sharpening problem-solving skills. The more you engage with different types of challenges, the more confident and capable you’ll become when approaching new tasks. This section provides a set of problems designed to reinforce your understanding of key principles and enhance your ability to apply them effectively.
Below are a few problems that cover a range of topics and difficulty levels. Work through each one carefully, paying attention to the methods used to arrive at the solution.
| Problem | Solution |
|---|---|
| Find the area of a rectangle with length 8 cm and width 5 cm. | Area = length × width = 8 × 5 = 40 cm² |
| What is the volume of a cylinder with a radius of 3 cm and height of 7 cm? | Volume = πr²h = 3.14 × 3² × 7 = 197.82 cm³ |
| Find the perimeter of a triangle with sides 6 cm, 8 cm, and 10 cm. | Perimeter = 6 + 8 + 10 = 24 cm |
| What is the area of a circle with radius 4 cm? | Area = πr² = 3.14 × 4² = 50.24 cm² |
By regularly solving problems like these, you’ll improve your speed and accuracy, enabling you to tackle even more complex questions with confidence.
Common Mistakes to Avoid in Geometry
While working through mathematical problems, it’s easy to make small errors that can lead to incorrect answers. These mistakes often arise from misunderstandings of basic principles, miscalculations, or overlooking key details. Identifying and avoiding these common pitfalls is essential for improving accuracy and boosting performance.
One frequent error is misapplying formulas. It’s important to know which formula is relevant for each specific problem and to use it correctly. For example, confusing the area of a rectangle with the perimeter can lead to significant mistakes in calculations.
Another mistake is not fully reading the problem before attempting to solve it. Skipping over key information, such as given values or specific instructions, can result in solving for the wrong quantity. Always take time to understand the question thoroughly before jumping into the solution.
Lastly, neglecting to check your work is a common misstep. Even after solving a problem, reviewing your calculations and reasoning is crucial. Double-checking your steps ensures that no errors have slipped through and helps you catch any inconsistencies before finalizing your answer.
Time Management Tips for Geometry Exams
Effective time management is crucial when preparing for any challenging assessment. Organizing your study sessions, allocating time wisely during the test, and staying focused can greatly improve your performance. By following specific strategies, you can ensure that you address all necessary areas without rushing or feeling overwhelmed.
During Study Sessions
When preparing for the test, it’s important to prioritize your time and focus on key areas. Here are some tips to help you maximize your study time:
- Set specific goals: Break your study time into manageable chunks and focus on one topic at a time.
- Use timed practice sessions: Simulate test conditions by timing yourself while working through practice problems.
- Review regularly: Spend short sessions reviewing key formulas and concepts to reinforce your knowledge.
- Identify weak areas: Focus more on challenging topics or areas where you feel less confident.
During the Test
Once you’re in the testing environment, managing time efficiently becomes essential. Here are some strategies to help you stay on track:
- Start with easy questions: Answer the simpler questions first to build confidence and secure easy points.
- Allocate time for each section: Divide your total test time by the number of sections or questions, and stick to it.
- Don’t get stuck: If a question is taking too long, move on and come back to it later.
- Leave time to review: Always reserve a few minutes at the end to check your answers for mistakes.
Reviewing Key Theorems and Proofs
Understanding theorems and the logical reasoning behind them is a critical aspect of mastering mathematical principles. Proofs allow you to demonstrate the validity of these theorems through structured arguments. A strong grasp of both will not only help you solve problems but also give you the tools to approach complex scenarios with confidence.
Key Theorems to Remember
There are several essential theorems that form the foundation of many problems. Familiarizing yourself with these theorems and knowing how to apply them in different contexts is crucial for success. Here are a few fundamental ones to keep in mind:
- Pythagorean Theorem: For any right triangle, a² + b² = c², where c is the hypotenuse.
- Congruence Theorems: SAS, ASA, and SSS theorems help prove the congruence of triangles.
- Properties of Parallel Lines: Angles formed by a transversal cutting parallel lines are key for solving problems involving parallelism and angle relationships.
- Angle Sum Theorem: The sum of the angles in any triangle equals 180°.
Understanding Proofs
Proofs provide a logical framework for demonstrating the truth of geometric statements. Whether proving the congruence of shapes or validating angle relationships, each proof follows a step-by-step process. The most effective way to understand proofs is by practicing how to construct them:
- Start with known facts: Use theorems, definitions, and given information as the basis for your proof.
- Follow logical steps: Build your argument in a clear, methodical way, making sure each step logically follows from the previous one.
- Conclude with the result: Finish the proof by restating the conclusion, ensuring that all steps lead to the desired outcome.
Tips for Problem Solving in Mathematics
Approaching mathematical challenges with the right strategies can greatly improve your ability to find solutions. Developing effective problem-solving techniques helps you break down complex scenarios and apply appropriate methods. With a clear approach, you can handle a variety of problems with confidence and accuracy.
Start with a clear understanding of the problem: Carefully read through the question, ensuring you understand all the given information. Identify what is being asked and which elements of the problem are critical for finding a solution.
Draw diagrams and label important elements: Visualizing the problem is often the key to unlocking the solution. Drawing diagrams, labeling key points, and marking measurements can make it easier to see relationships and apply formulas.
Use known formulas and relationships: Apply relevant mathematical principles that you are familiar with, such as area and perimeter formulas or properties of angles and shapes. Don’t hesitate to recall previous examples or patterns you’ve learned.
Break the problem into smaller parts: If the problem seems overwhelming, divide it into manageable sections. Solve each part step by step, and eventually, you’ll arrive at the complete solution.
Check your work: After solving the problem, review each step to ensure no mistakes were made. Verifying your calculations and logical steps helps catch errors and solidifies your solution.
How to Tackle Word Problems Effectively
Word problems often seem daunting due to the need to translate verbal descriptions into mathematical expressions. However, by breaking them down into manageable steps, you can turn complex situations into solvable challenges. The key to success is understanding the structure of the problem and applying the right techniques to find the solution.
Read the problem carefully: Begin by thoroughly reading the entire question. Pay attention to the details, such as units of measurement and key information, which will guide your solution process.
Identify what is being asked: Understand the main goal of the problem. Are you asked to find a length, an area, or a relationship between different quantities? Knowing what you need to solve for is the first step in choosing the right approach.
Translate the words into mathematical expressions: Once you have a clear understanding of the problem, convert the written information into symbols, equations, or diagrams. This helps you visualize the relationships between variables and simplifies the process of finding a solution.
Organize the information: Write down all the known values, and clearly label the unknowns. This will make it easier to set up the necessary calculations and identify any additional steps needed to reach the answer.
Work step by step: Solve the problem methodically, ensuring that each step logically follows from the previous one. Avoid rushing, as each stage builds on the last to ensure an accurate solution.
Check your solution: Once you’ve solved the problem, review your work. Ensure that the solution makes sense in the context of the question and verify all calculations for accuracy.
Understanding Diagrams and Figures
Diagrams and figures play a crucial role in solving mathematical problems, as they visually represent relationships between different elements. Understanding how to interpret these visual aids is key to correctly applying principles and arriving at the right answers. Recognizing key features, angles, and measurements in a diagram will guide you through the problem-solving process.
Here are some strategies for understanding and using diagrams effectively:
- Identify known information: Look for labeled points, angles, and lines. These often contain the values or relationships that are essential for solving the problem.
- Recognize shapes and their properties: Identify whether the figure represents common shapes like triangles, circles, or quadrilaterals. Each shape has specific properties that can be applied to find unknown values.
- Understand the relationships between elements: Pay attention to how different parts of the figure relate to one another, such as parallel lines, perpendicular lines, or congruent angles.
- Look for symmetry: Many problems involve symmetrical figures. Identifying lines of symmetry can simplify the process of solving for unknown values.
- Use auxiliary lines: In some cases, drawing additional lines or segments within the diagram can help reveal hidden relationships and make the solution more straightforward.
By carefully studying the diagram and focusing on these aspects, you can build a solid foundation for solving the problem and applying the correct mathematical techniques. The visual representation provides a helpful roadmap for organizing your thoughts and calculations.
Strategies for Multiple Choice Questions
When tackling multiple-choice questions, it’s essential to apply a strategic approach to maximize your chances of selecting the correct answer. These types of questions often present several possible solutions, and understanding how to efficiently analyze each option can save time and reduce errors. By breaking down each question methodically, you can eliminate unlikely choices and confidently determine the right answer.
Here are some key strategies to apply when faced with multiple-choice problems:
| Strategy | Description |
|---|---|
| Eliminate clearly incorrect answers | Start by reviewing all options and immediately rule out answers that are obviously incorrect. This narrows your choices and increases the odds of guessing correctly if you’re unsure. |
| Check for common distractors | Many multiple-choice questions include answers that are designed to confuse, often with small errors. Carefully analyze each option to spot these common mistakes. |
| Use estimation | If the question involves calculations, try estimating the answer to quickly gauge which choices are reasonable. This technique can help you avoid wasting time on complex computations. |
| Look for keywords in the question | Pay attention to specific wording in the question, such as “always,” “never,” or “most likely,” as these can give clues about the correct answer. The right choice often aligns with the phrasing of the question. |
| Work backward from the options | If you’re unsure, try substituting the answer choices into the problem and check which one fits the given conditions. This process can often help you identify the right solution. |
By incorporating these strategies into your approach, you can increase your efficiency and accuracy when answering multiple-choice questions. Each step helps you focus on the key elements of the question, making it easier to narrow down your options and confidently select the correct response.
How to Solve Equations in Mathematics
Solving equations in mathematical problems requires a step-by-step approach to isolate unknowns and find the values that satisfy the given conditions. Whether working with basic algebraic expressions or more complex relationships, following a systematic method will help ensure accurate results. It’s crucial to understand the structure of the equation and apply the appropriate mathematical techniques to solve it.
Key Steps to Solve Mathematical Equations
- Identify the variables: Start by recognizing the unknowns in the equation. These are typically represented by letters like x or y, and your goal is to find their values.
- Isolate the variable: Use algebraic operations such as addition, subtraction, multiplication, and division to get the variable on one side of the equation. This makes it easier to solve for it.
- Simplify the equation: Combine like terms and reduce the equation as much as possible. Simplification helps in eliminating unnecessary components and brings you closer to the solution.
- Apply inverse operations: If the variable is being added, subtract it; if it’s being multiplied, divide by the same number. Use the inverse operations to reverse the effects and isolate the variable.
- Check your solution: Once you’ve found a solution, substitute the value of the variable back into the original equation to verify that it satisfies the equation. This ensures your work is correct.
Common Mistakes to Avoid
- Not following the order of operations: Always remember the proper order (PEMDAS – Parentheses, Exponents, Multiplication, Division, Addition, Subtraction) when simplifying the equation.
- Forgetting to simplify both sides: Ensure that you simplify both sides of the equation equally during each step to avoid errors in your solution.
- Skipping the verification step: Failing to check your solution can lead to overlooked mistakes, which can result in incorrect answers.
By following these steps and avoiding common pitfalls, you can efficiently solve mathematical equations and confidently arrive at the correct solutions. Practice is key to mastering these techniques and becoming proficient at solving a wide range of problems.
Essential Vocabulary You Should Know
Mastering key terms and concepts is essential to solving mathematical problems efficiently. A strong grasp of the right vocabulary not only aids in understanding the principles behind each problem but also helps when interpreting questions and instructions. Here are some crucial terms that every student should familiarize themselves with to navigate mathematical challenges with confidence.
Key Terms in Mathematics
| Term | Definition |
|---|---|
| Angle | The space between two intersecting lines measured in degrees. |
| Perimeter | The total distance around the boundary of a shape or figure. |
| Area | The amount of space within the boundary of a shape, usually measured in square units. |
| Radius | The distance from the center of a circle to any point on its circumference. |
| Diameter | The longest distance across a circle, passing through its center. |
| Hypotenuse | The longest side of a right triangle, opposite the right angle. |
| Congruent | Figures or shapes that have the same size and shape. |
| Parallel | Lines or planes that never meet and are equidistant from each other. |
Additional Terms to Remember
| Term | Definition |
|---|---|
| Symmetry | A balanced arrangement of parts on either side of a line or around a point. |
| Vertical angles | Two opposite angles formed by two intersecting lines, which are always equal. |
| Complementary angles | Two angles that add up to 90 degrees. |
| Supplementary angles | Two angles that add up to 180 degrees. |
| Transversal | A line that crosses two or more other lines, often creating angles. |
Understanding these terms will help you approach problems more effectively and interpret questions with greater ease. Be sure to regularly review these vocabulary words as they form the foundation of many mathematical concepts and problem-solving techniques.
Focus Areas for Test Preparation
When preparing for a significant assessment in mathematics, identifying the most crucial topics is essential for effective study. Focusing on key concepts will ensure that your time is used wisely, allowing you to confidently tackle the most common problem types and challenges. By honing in on these critical areas, you’ll improve your problem-solving skills and build a stronger foundation for answering complex questions.
| Topic | Why It’s Important |
|---|---|
| Basic Shapes and Properties | Understanding the fundamental properties of shapes, such as circles, triangles, and quadrilaterals, is key to solving many problems. You’ll need to know their characteristics, such as side lengths, angles, and relationships between different elements. |
| Angles and Their Relationships | Angles appear in a variety of problems, from determining missing angles in a figure to solving for unknown variables. Understanding complementary, supplementary, and vertical angles is crucial for accurate calculations. |
| Area and Perimeter Formulas | These fundamental formulas are essential for solving problems related to the measurement of surfaces and boundaries. You should be able to apply them to various figures, including complex shapes that require decomposition into simpler forms. |
| Volume and Surface Area of Solids | Calculating the volume and surface area of three-dimensional objects is a common task. Mastering the formulas for spheres, cones, cylinders, and other solids is crucial for handling word problems efficiently. |
| Transformations (Translation, Rotation, Reflection) | Understanding how shapes move and change under translation, rotation, and reflection is key for recognizing symmetries and solving geometric puzzles. These skills are vital for visualizing problems and finding solutions. |
| Pythagorean Theorem | This theorem is essential for solving right-angled triangle problems. Being able to apply it to find missing sides of triangles will help with numerous geometry questions. |
| Trigonometric Ratios | For right triangles, the use of sine, cosine, and tangent ratios is a powerful tool for solving problems involving angles and sides. Familiarity with these concepts is necessary for tackling more advanced questions. |
By prioritizing these areas, you’ll not only strengthen your understanding of core concepts but also increase your speed and accuracy during the assessment. Regular practice in these focus areas will help solidify your knowledge and ensure you’re well-prepared for whatever challenges arise on test day.
Study Techniques That Work
Effective preparation requires not only understanding the material but also utilizing study strategies that enhance memory retention and problem-solving skills. By adopting techniques that promote active engagement and consistent practice, you can improve your understanding and boost your confidence. These methods are designed to help you organize your study time, focus on key concepts, and tackle challenging problems with ease.
1. Active Recall – This technique involves actively testing your knowledge rather than passively reviewing notes. For example, after reading through a section, close your book and try to recall the main ideas or formulas. This helps strengthen memory and improve long-term retention.
2. Practice Problems – Regularly solving problems is one of the most effective ways to reinforce learning. By working through a variety of problems, you not only test your knowledge but also learn how to apply theories and concepts to different scenarios.
3. Spaced Repetition – Instead of cramming all at once, break your study sessions into smaller chunks and revisit the material periodically. This technique helps to cement knowledge and prevent it from fading away after a short period of time.
4. Concept Mapping – Visualizing relationships between concepts is an excellent way to organize information. Creating diagrams or flowcharts can help you see how different topics are interconnected, making it easier to understand and remember complex ideas.
5. Study Groups – Collaborating with peers can provide new perspectives and help fill gaps in your understanding. Explaining concepts to others also reinforces your own knowledge, making it a valuable part of your preparation.
6. Break Down Difficult Concepts – When encountering challenging material, break it into smaller, manageable parts. Focus on understanding each piece before trying to tackle the entire problem. This method prevents feeling overwhelmed and builds confidence step by step.
7. Teach What You Learn – One of the best ways to solidify your understanding is to teach the material to someone else. Explaining complex topics in simple terms helps identify any gaps in your knowledge and deepens your understanding.
By incorporating these proven study techniques into your routine, you can maximize your preparation and approach any challenge with confidence. Consistency and smart strategies are the keys to mastering the material and achieving success.
Important Topics to Review
To effectively prepare for any assessment in this subject, it’s essential to focus on the most significant areas that encompass key concepts and problem-solving strategies. By understanding and mastering these topics, you’ll be better equipped to tackle a variety of questions and scenarios. Below are the primary topics you should prioritize in your preparation.
Key Areas to Focus On

- Angles and Their Properties – Understanding types of angles, such as complementary, supplementary, vertical, and adjacent angles, is critical. Learn how to solve problems that involve angle relationships in various shapes and configurations.
- Triangles and Their Properties – Be sure to review the properties of different triangles, including equilateral, isosceles, and scalene. Master the Pythagorean theorem, as well as methods to calculate side lengths and angles using trigonometry.
- Circles – Focus on the relationships between the radius, diameter, circumference, and area. Be prepared to solve problems involving arcs, chords, tangents, and sector areas.
- Coordinate Systems – Review how to plot points and graph equations on the coordinate plane. Understand the concepts of slope, distance, and midpoint formulas for solving problems involving lines and geometric shapes in the coordinate plane.
- Polygons – Understand the properties of regular and irregular polygons, such as quadrilaterals, pentagons, and hexagons. Know how to calculate area and perimeter for various polygon types.
Advanced Topics for Deeper Understanding
- Solid Figures – Review the properties and formulas for calculating surface area and volume for three-dimensional shapes such as cubes, spheres, cones, and cylinders.
- Transformations – Study the effects of transformations like translation, rotation, reflection, and dilation on geometric figures. Be able to apply these transformations to solve problems and prove congruence or similarity.
- Congruence and Similarity – Understand the criteria for triangle congruence (SSS, SAS, ASA, AAS) and the properties of similar triangles. Know how to use these principles to solve real-world problems involving scaling and proportionality.
- Proofs and Theorems – Focus on key theorems such as the triangle sum theorem, the properties of parallel lines, and theorems related to circles and angles. Be familiar with writing logical proofs and justifying each step based on established rules.
Mastering these topics will not only ensure you’re prepared for a variety of questions but will also help you approach more complex problems with confidence and clarity. Regular practice and a solid understanding of these key concepts are essential to success.
How to Stay Calm During the Exam
Managing stress and maintaining composure during a test is crucial to perform at your best. By employing certain techniques, you can reduce anxiety and approach each question with a clear, focused mind. The key is to prepare both mentally and physically before and during the assessment. Here are some effective strategies to stay calm and perform well under pressure.
- Practice Deep Breathing – Slow, deep breaths can help calm your nervous system and reduce anxiety. Before you begin, take a few deep breaths, inhaling through your nose and exhaling through your mouth. This simple exercise can lower stress levels and keep you focused.
- Read Instructions Carefully – Taking time to thoroughly understand the instructions prevents mistakes caused by rushing. Reading each question carefully allows you to plan your approach and avoid unnecessary stress.
- Start with What You Know – Begin with the questions that seem easiest to you. This will help build confidence and create a positive momentum that can carry you through the more challenging parts of the test.
- Stay Positive – Avoid negative self-talk. Remind yourself that you are prepared and capable. A positive mindset can significantly boost your performance and help reduce feelings of panic.
- Take Breaks When Needed – If you feel overwhelmed, take a short mental break. Close your eyes for a few seconds, stretch, or shake out your hands. A brief break can refresh your mind and reduce stress.
- Focus on One Question at a Time – Avoid overthinking the entire test. Concentrate on answering one question at a time and tackle them methodically. This focused approach reduces the feeling of being overwhelmed.
- Use Time Wisely – Allocate time for each section or question. If you get stuck, move on to the next one and return later. Proper time management ensures you don’t spend too much time on any single part, keeping your anxiety in check.
By incorporating these techniques, you’ll be able to manage stress more effectively and approach the assessment with a calm, focused mindset. Preparation, relaxation, and positive thinking are your best tools for performing at your best under pressure.