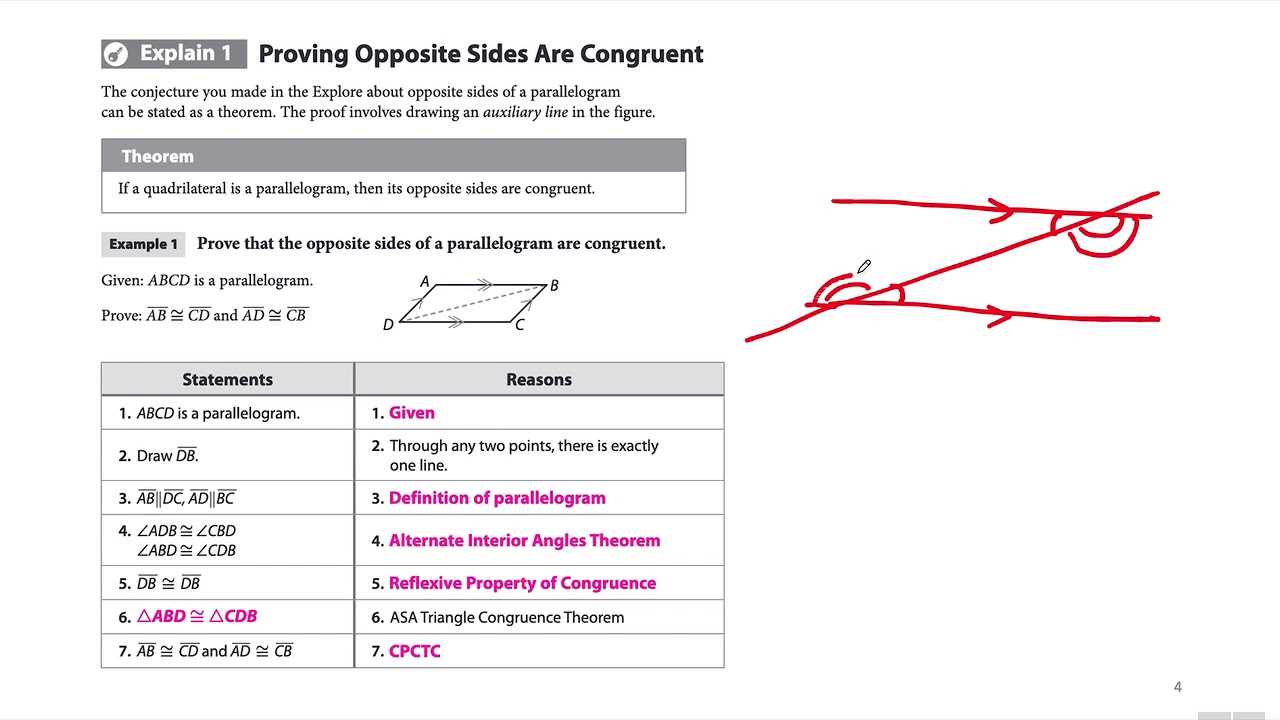
In the world of geometry, certain shapes exhibit unique features that distinguish them from others. Some of these figures possess specific characteristics that help identify them easily, especially when analyzing their sides, angles, and other defining elements. Recognizing these traits is essential for solving related mathematical problems and understanding the shape’s behavior in various contexts.
When exploring quadrilaterals, one encounters figures with parallel sides, equal angles, and other specific relationships between their parts. These traits allow for a deeper understanding of how such figures function in geometric proofs and calculations. Whether you are working on area or angle problems, grasping these details is crucial for tackling related tasks successfully.
Through this exploration, we will break down key features, providing clarity on how to approach calculations, proofs, and real-life applications. Understanding these aspects will sharpen your ability to identify and work with these shapes confidently.
Properties of Parallelograms Answers

Understanding the characteristics of certain quadrilaterals is essential for solving geometric problems effectively. These shapes exhibit distinct relationships between their sides, angles, and diagonals, which can be leveraged in various mathematical contexts. By recognizing these key aspects, one can easily apply the relevant formulas and theorems to calculate area, perimeter, or solve for missing measurements.
Here are some key insights that help in identifying and solving problems related to such figures:
- Opposite Sides: In these shapes, opposite sides are always parallel and equal in length. This basic feature plays a significant role when determining the shape’s area or perimeter.
- Opposite Angles: The angles formed by opposite sides are congruent, meaning they share the same measure. This property is useful when working with angle-based calculations.
- Diagonals: The diagonals of these shapes do not necessarily bisect each other at right angles, but they always divide the shape into two triangles of equal area.
- Sum of Angles: The sum of the interior angles of these figures is always 360 degrees. This principle helps in angle-related problems.
- Area Formula: To calculate the area, simply multiply the base by the height. This formula is effective for all figures with parallel sides.
By focusing on these key characteristics, students and practitioners can apply logical reasoning to answer various questions and solve problems accurately. Recognizing the interrelationships between angles, sides, and diagonals simplifies the approach to complex geometric tasks.
Defining a Parallelogram in Geometry
In geometry, certain quadrilaterals stand out due to their distinct characteristics. These shapes are defined by their sides and angles, which follow specific rules that differentiate them from other types of polygons. Understanding the definition and structure of such shapes is essential for solving a wide range of problems in both theoretical and practical contexts.
Key Features of These Quadrilaterals
Several key elements define the shape, and knowing them helps in both identification and calculation:
- Parallel Sides: The most important feature is that both pairs of opposite sides are parallel to each other. This means that the sides never intersect, regardless of how far they are extended.
- Equal Opposite Sides: In addition to being parallel, opposite sides are also equal in length, which is a crucial aspect when solving for unknown distances or areas.
- Angle Relationships: The angles formed between adjacent sides are supplementary, meaning their sum is always 180 degrees.
Application and Identification
Recognizing this figure in geometry often involves checking the above characteristics. If a quadrilateral has parallel and equal opposite sides, with supplementary adjacent angles, it can be confidently classified as this type of quadrilateral. These properties are frequently applied in problems related to geometry, such as calculating areas or solving for unknown values based on given dimensions.
Key Characteristics of Parallelograms
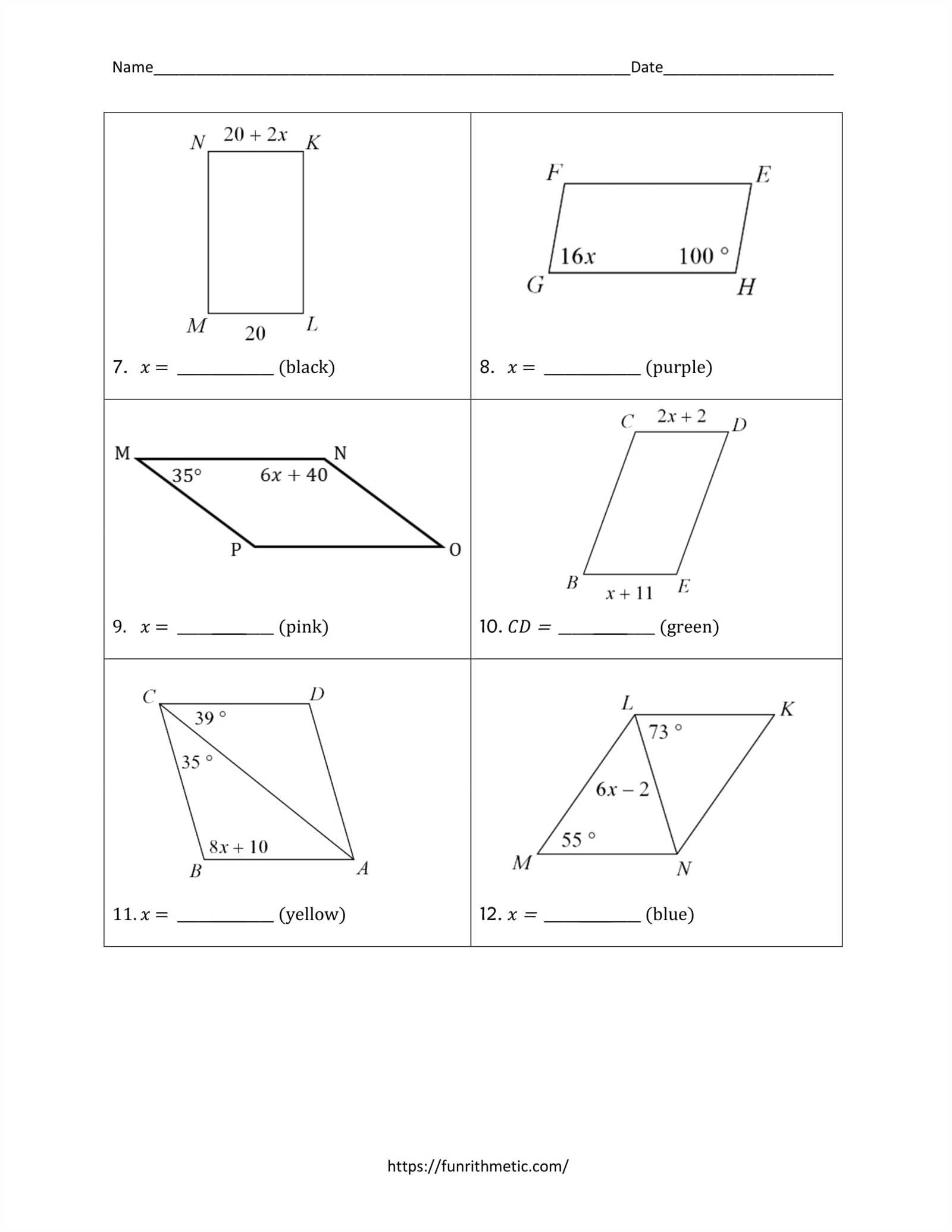
Quadrilaterals with specific geometric features form an important category in the study of shapes. These characteristics set them apart from other four-sided figures and provide a foundation for solving various mathematical problems. Understanding these essential traits is crucial for accurately applying formulas and principles in geometry.
Fundamental Traits of These Quadrilaterals
The following traits define the structure and behavior of these shapes:
- Opposite Sides Are Equal: Each pair of opposite sides not only runs parallel but also maintains the same length. This ensures balance in the overall shape and is a vital factor in geometric calculations.
- Adjacent Angles Are Supplementary: The angles formed between adjacent sides always add up to 180 degrees, which is a critical property when working with angle-based problems.
- Diagonals Divide the Shape: The diagonals intersect each other, splitting the shape into two congruent triangles. However, they do not necessarily bisect each other at right angles.
- Angle Pairing: Opposite angles in these shapes are always congruent, meaning they have equal measurements, another factor that aids in solving for unknown angles.
Why These Traits Matter
These fundamental characteristics provide both a method of identification and a toolkit for solving related geometric problems. Whether determining area, finding missing sides, or proving the equality of angles, the unique combination of these traits offers clear strategies for tackling various challenges.
Angle Relationships in Parallelograms
The relationships between angles in certain quadrilaterals play a crucial role in geometry. These connections help identify specific angle measures and provide valuable insights into the shape’s symmetry and structure. By understanding these relationships, it becomes easier to calculate unknown angles and solve various geometric problems.
Adjacent Angles Are Supplementary

One key principle in these shapes is that adjacent angles always add up to 180 degrees. This means that if the measure of one angle is known, the measure of the adjacent angle can be easily determined by subtracting it from 180. This rule simplifies angle-related calculations and helps verify the consistency of angle measures within the shape.
Opposite Angles Are Congruent
Another important characteristic is that opposite angles are always equal. No matter how the shape is oriented, each pair of opposite angles will have the same measure. This symmetry is a helpful tool in geometric proofs and calculations, as it reduces the number of variables to consider when working with angles.
By applying these fundamental angle relationships, solving for missing angles becomes a straightforward process, helping to confirm the validity of various geometric properties.
Opposite Sides and Angles Equality
In certain quadrilaterals, the symmetry of opposite sides and angles is a fundamental characteristic. This equality creates balance within the shape, making it easier to identify and solve for unknown measurements. Understanding these relationships is key to working with such figures in geometric problems.
Equal Opposite Sides
One of the most important traits of these shapes is that opposite sides are both parallel and equal in length. This means that if you know the length of one side, you can easily deduce the length of its opposite side. This property is especially useful when calculating area or perimeter, as it ensures that all sides are accounted for consistently.
Equal Opposite Angles
In addition to equal sides, opposite angles in these shapes are always congruent. This means that each pair of angles that are opposite to one another will have the same measure. This symmetry helps simplify angle-related problems and serves as a foundational concept when proving geometric theorems.
The equality of opposite sides and angles provides a reliable framework for solving problems related to these figures, ensuring consistent results in calculations and proofs.
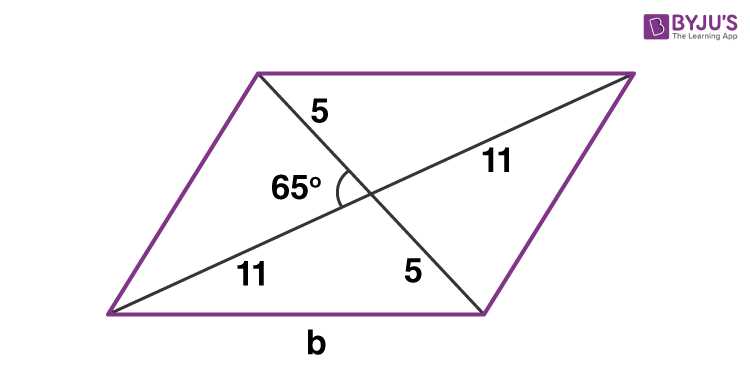
Diagonals of Parallelograms Explained
The diagonals of certain quadrilaterals offer important insights into the structure of the shape. These line segments, drawn between opposite corners, play a key role in understanding the properties and relationships within the figure. Exploring how these diagonals behave allows for more accurate calculations and geometric reasoning.
Intersection and Symmetry
One notable feature of the diagonals is that they always intersect at a point. However, unlike in some shapes where diagonals bisect at right angles, in these figures, the diagonals simply divide the shape into two triangles of equal area. This symmetry ensures that each half of the shape mirrors the other, which can be useful when solving for unknown values or proving geometric properties.
Diagonals Are Not Necessarily Equal
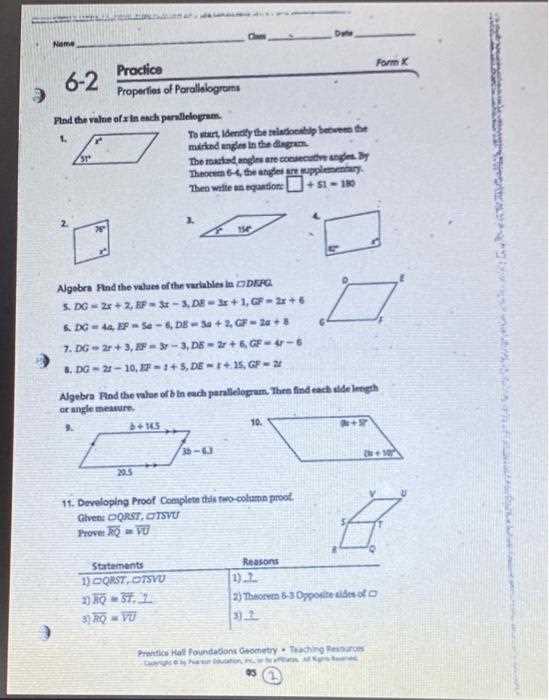
Although the diagonals intersect at a single point, they are not always of equal length. The lengths of the diagonals can vary depending on the specific dimensions of the shape. This characteristic distinguishes these figures from others, where diagonals are often congruent.
Understanding the behavior of the diagonals is essential for tackling complex geometric problems, such as calculating area, finding angles, or working with transformations. By recognizing how they divide the shape and relate to other elements, one can make more informed calculations and conclusions in geometric tasks.
Area Calculation of a Parallelogram
Finding the area of certain quadrilaterals is a fundamental task in geometry. The area represents the total space enclosed within the boundaries of the figure. The formula for calculating the area of these shapes is straightforward, but it requires understanding the relationship between the base and height of the shape.
Formula for Area
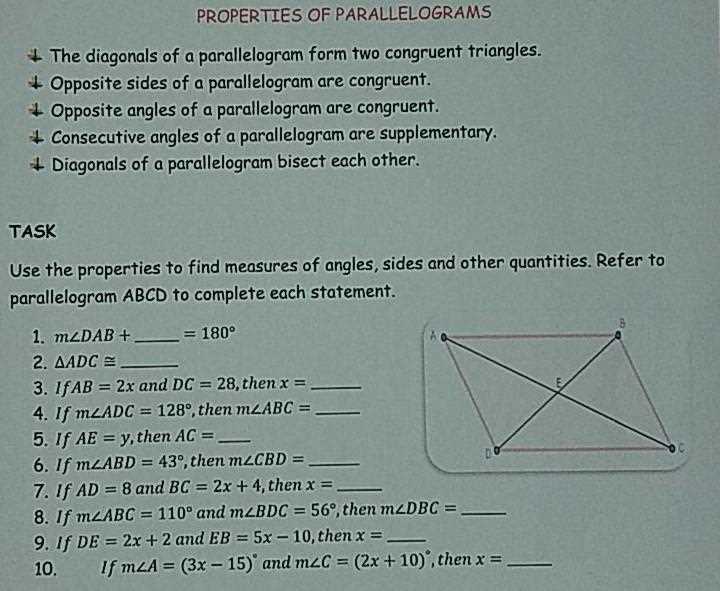
The area of this type of quadrilateral can be calculated using the following formula:
- Area = base × height
Where:
- Base: The length of one of the sides, typically the bottom side, which is used as the reference length for the calculation.
- Height: The perpendicular distance from the base to the opposite side. This is the shortest distance between the two parallel sides.
Key Considerations
It is important to note that the height must be measured perpendicular to the base. This ensures that the area calculation is accurate. If the height is not given directly, it may need to be derived using other geometric properties or trigonometric relationships, depending on the given information.
Once the base and height are identified, applying the formula becomes a simple task. This calculation is particularly useful when working with geometry problems involving transformations, area comparisons, or spatial reasoning.
How to Prove a Figure is a Parallelogram
To establish that a quadrilateral has specific properties, several geometric tests can be applied. These tests verify the necessary conditions that define the shape and help distinguish it from other figures. By using logical reasoning and mathematical principles, one can confirm if a given shape satisfies the criteria to be categorized as this particular type of four-sided figure.
Using Opposite Sides
One common method to prove that a figure is this type of quadrilateral is by showing that both pairs of opposite sides are parallel. If both sets of opposite sides are parallel, the figure can be concluded to have the desired characteristics. This method is simple and effective, particularly when parallel lines can be identified clearly.
Using Opposite Sides and Angles
Another way to prove a quadrilateral is this shape is to demonstrate that opposite sides are not only parallel but also congruent in length. Additionally, proving that opposite angles are equal further solidifies the classification of the shape. If both conditions are met, the figure must be the type of quadrilateral in question.
Using Diagonal Properties
Finally, analyzing the diagonals can be a helpful approach. If the diagonals bisect each other at their midpoint, this is another indicator that the shape exhibits the necessary qualities. Diagonal bisecting ensures that each half of the shape mirrors the other, which is a hallmark of the desired geometric figure.
Through these various methods, it becomes possible to rigorously prove that a quadrilateral possesses the defining characteristics required to be classified as this type of shape.
Applications of Parallelograms in Real Life
The unique characteristics of certain four-sided figures make them highly useful in various real-world applications. From engineering to design, these shapes are integral to many structures and objects we encounter daily. Their ability to maintain parallelism and symmetry under different conditions provides both functionality and aesthetic value in numerous fields.
Architectural and Structural Design
In architecture and civil engineering, these shapes are often used in the design of buildings, bridges, and other structures. The ability to create stable and durable forms is essential, and the parallel sides offer increased support. Many roofs, windows, and door frames use this shape for aesthetic appeal as well as practicality, ensuring stability in design.
Design and Manufacturing
In the manufacturing of everyday items such as tables, shelves, and even certain mechanical components, these figures help in creating uniform, sturdy structures. The symmetrical nature of the shape allows for efficient material distribution, making products not only more durable but also cost-effective in terms of material usage.
Navigation and Mapping
In the field of navigation, particularly in map design, this shape is often utilized. Grid-based maps, for example, use similar structures to divide space in an organized manner. The even spacing and alignment allow for accurate measurements and clear representation of geographical areas.
From construction projects to the design of simple everyday items, the use of these shapes provides both functional and visual benefits. Their widespread application demonstrates their importance in achieving efficiency, stability, and balance in various sectors.
Properties of Parallelograms in Coordinate Geometry
In coordinate geometry, the study of certain four-sided shapes is enhanced by utilizing the coordinate plane. By assigning coordinates to the vertices of a figure, one can easily calculate distances, slopes, and other geometric properties that help confirm the shape’s classification. This approach not only simplifies problem-solving but also provides a clear, algebraic method for verifying key characteristics.
Determining Slope and Parallelism
One of the primary ways to analyze these shapes in coordinate geometry is by examining the slopes of opposite sides. If the slopes of two sides are equal, it confirms that the sides are parallel. This method is especially useful in determining whether a quadrilateral exhibits the necessary symmetry for being this type of shape. The slope formula, m = (y₂ – y₁) / (x₂ – x₁), can be applied to the coordinates of each pair of opposite sides to check for parallelism.
Checking for Congruent Opposite Sides
Another important characteristic in coordinate geometry is verifying that opposite sides have equal length. By using the distance formula, d = √[(x₂ – x₁)² + (y₂ – y₁)²], one can calculate the length of each side of the quadrilateral. If both pairs of opposite sides are congruent, this further supports the claim that the figure meets the conditions for being this type of geometric shape.
Through these methods, coordinate geometry allows for a more straightforward and systematic way to prove whether a quadrilateral possesses the necessary features, offering a deeper understanding of its structure and behavior.
Symmetry and Reflection in Parallelograms
In the study of geometric shapes, symmetry and reflection are key aspects that provide insight into their structure and properties. These concepts allow us to understand how a figure behaves when manipulated or mirrored along a specific axis. For some four-sided figures, symmetry plays a crucial role in determining balance and visual harmony, while reflections reveal how the shape can be folded or flipped across certain lines.
Reflection Symmetry
Reflection symmetry occurs when a shape can be divided into two identical halves by a line of symmetry. In the case of a specific type of quadrilateral, this symmetry often reveals itself in the alignment of opposite sides and angles. However, unlike certain shapes such as squares or rectangles, this figure does not always have a line of symmetry through the center.
Symmetry in Opposite Sides
The symmetry of opposite sides plays a significant role in maintaining the balance and structure of this type of shape. The two pairs of opposite sides are parallel and congruent, contributing to the overall uniformity of the figure. This inherent symmetry allows the shape to exhibit properties such as balanced diagonals and equal opposite angles.
| Property | Symmetry Type |
|---|---|
| Opposite Sides | Parallel and Congruent |
| Opposite Angles | Equal |
| Diagonals | Bisect Each Other |
Through these reflections and symmetric properties, the shape’s geometry reveals a deeper understanding of its overall structure. Symmetry ensures that these figures maintain their form under various transformations, whether mirrored or rotated.
Special Types of Parallelograms
In geometry, certain quadrilaterals have unique features that set them apart from other four-sided shapes. These variations arise from specific relationships between their sides and angles, and they exhibit distinctive characteristics that make them important in various geometric contexts. Understanding these specialized types helps to explore how a figure can adapt to different constraints while still maintaining fundamental structural qualities.
Among these, there are several notable categories that are often encountered in geometric problems, each with its own set of defining traits. These specialized shapes share some common features, but they also differ in key aspects that make them stand out. Here are some of the most important types:
- Rectangle: A quadrilateral with all angles equal to 90 degrees. It is a special type of shape where opposite sides are equal in length and parallel, but all angles are right angles.
- Rhombus: A quadrilateral with all sides of equal length. Unlike a square, the angles are not necessarily 90 degrees, but the opposite angles remain congruent.
- Square: A special case of both a rectangle and a rhombus, where all sides are equal, and all angles are 90 degrees.
- Rhomboid: A quadrilateral where opposite sides are parallel and unequal in length, and the angles are oblique (not 90 degrees), but opposite angles are equal.
These variations are useful in solving many types of geometric problems, as each type of figure brings unique properties that can simplify calculations and reasoning. Whether working with right angles, equal sides, or diagonal relationships, understanding these special shapes allows for more efficient solutions in both theoretical and practical applications.
Area vs Perimeter of Parallelograms
When working with quadrilaterals, two fundamental measurements are often of interest: the area and the perimeter. Both are essential in determining the size and boundary of a figure, but they are calculated differently and serve distinct purposes. While the area measures the space enclosed by the figure, the perimeter focuses on the total length of its sides. These concepts help us understand and compare geometric shapes in a variety of real-world applications.
For any quadrilateral, including those with parallel opposite sides, the area and perimeter can be computed using specific formulas, each focusing on different aspects of the shape’s structure.
- Area Calculation: The area of a quadrilateral is calculated by multiplying the base by the height. The base is any side of the shape, and the height is the perpendicular distance from that base to the opposite side. This gives the total space enclosed by the figure.
- Perimeter Calculation: The perimeter is found by adding the lengths of all four sides. Since opposite sides are equal in length, this calculation simplifies to twice the sum of one pair of opposite sides.
Both area and perimeter are crucial for practical purposes such as construction, design, and various forms of measurement in engineering and architecture. While the area gives an understanding of how much space a figure occupies, the perimeter informs us about the total distance around it. Each serves as a different but complementary tool in the study of geometry.
Why Diagonals Do Not Bisect Each Other
In certain quadrilaterals, the diagonals intersect, but they do not always split each other into two equal parts. The reason for this lies in the geometric properties and the angle relationships of the sides and angles of the shape. While some types of quadrilaterals exhibit bisected diagonals, this is not universally true for all quadrilateral forms.
The key factor that prevents the diagonals from bisecting each other is the lack of symmetry in some shapes. For example, in a general quadrilateral with no special conditions, the diagonals typically do not meet at their midpoints. This is due to the unequal lengths and angles between opposite sides, which do not allow the diagonals to divide each other evenly.
In specific quadrilaterals, such as rectangles or rhombuses, the diagonals are either perpendicular or bisect each other because of symmetry and equal opposite sides. However, in more irregular shapes, the absence of symmetry results in the diagonals crossing at different angles, thus failing to bisect each other.
Understanding Parallelogram Proofs
In geometry, demonstrating the validity of certain shapes involves establishing relationships between their sides, angles, and diagonals. This process requires logical reasoning and evidence to confirm whether a given figure meets the necessary conditions to be classified as a specific type of quadrilateral. When it comes to proving the properties of a quadrilateral, several approaches and strategies can be applied to show that certain criteria are met.
Steps to Prove a Quadrilateral is a Specific Shape
To prove that a figure is a specific quadrilateral, one must focus on particular characteristics such as side lengths, angle measurements, or diagonal intersections. Below is a table outlining some common methods used in proofs.
| Method | Explanation |
|---|---|
| Side Parallelism | If both pairs of opposite sides are parallel, the shape can be classified as a quadrilateral with parallel opposite sides. |
| Equal Opposite Sides | If opposite sides are equal in length, this can serve as a proof of symmetry, helping identify specific types of quadrilaterals. |
| Angle Relationships | Demonstrating that opposite angles are equal or adjacent angles are supplementary can verify a quadrilateral’s specific type. |
Using Coordinates in Proofs
In some cases, the use of a coordinate system can simplify the proof process. By plotting the vertices of a figure and calculating distances and slopes, one can easily determine whether the shape meets the required conditions for a specific type of quadrilateral.
Common Mistakes in Parallelogram Problems
When working with quadrilaterals that exhibit specific geometric relationships, it is easy to make certain miscalculations or assumptions. Many people encounter challenges when applying properties such as parallelism, angle measures, and side lengths. Understanding where these mistakes often occur can help prevent errors and lead to more accurate solutions.
One of the most frequent errors occurs when assuming that a shape automatically fits the criteria for a specific category of quadrilateral based on only one or two characteristics. This can lead to misclassifications or overlooked details in calculations. Let’s examine some of the most common pitfalls encountered in solving these problems.
Incorrect Assumptions About Angles
Another common mistake is misinterpreting the angle relationships within a figure. For instance, assuming that all angles are equal simply because they are adjacent or opposite to one another can lead to incorrect conclusions. While opposite angles may indeed be equal, this is not always true in every scenario.
Ignoring Symmetry
Failing to account for symmetry can also lead to errors. In some cases, diagonal lengths or the angles between them might suggest that symmetry exists, but it is important to check the conditions carefully. For example, even if the sides of a shape are equal, without checking if the diagonals bisect each other correctly, one may miss crucial aspects of the problem.
Forgetting to Use Coordinates
In problems involving coordinates, one common mistake is neglecting to properly calculate the slopes and distances between vertices. Without this information, it’s difficult to verify whether the sides of a quadrilateral are indeed parallel or equal in length. Accurate coordinate geometry can eliminate assumptions and provide more reliable results.
By carefully avoiding these errors and applying logical steps in geometric problems, one can improve accuracy and gain a better understanding of the relationships in various quadrilaterals.