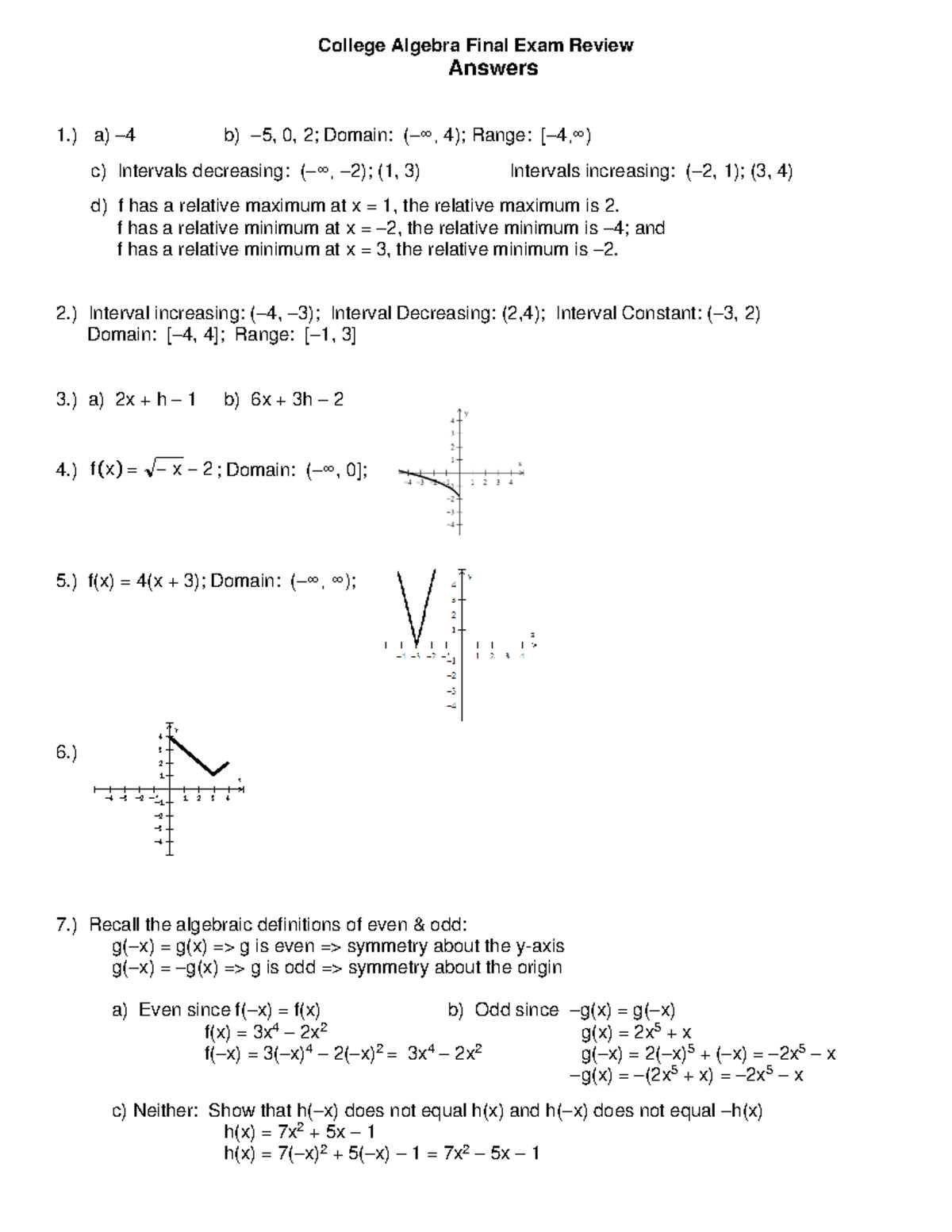
Achieving success in mathematics requires a deep understanding of essential principles and an ability to solve problems efficiently. As you approach the upcoming challenge, it’s important to reinforce your knowledge and strengthen your skills through targeted practice. This section provides comprehensive solutions to help you navigate through various problem types with clarity and confidence.
By working through key mathematical topics and honing your problem-solving abilities, you will develop the tools necessary to tackle even the most complex tasks. Understanding each concept thoroughly will enable you to approach questions methodically, ensuring that you make fewer mistakes and increase your overall performance.
Consistent practice and strategic preparation are the best ways to ensure you are fully prepared when it’s time to demonstrate your knowledge.
Comprehensive Guide to Math Assessment
Preparing for a major mathematics assessment involves more than just memorizing formulas. It requires a thorough understanding of foundational principles and the ability to apply them in various problem-solving scenarios. This guide provides a structured approach to help you review essential topics and sharpen your skills in preparation for the test.
Throughout this section, we will break down the key concepts you’ll need to master. From equations to graphing techniques, every topic is addressed with clear explanations and practical examples. By focusing on the reasoning behind each solution, you will improve your problem-solving techniques and gain confidence in tackling similar questions.
Consistent practice and a strategic approach are crucial in maximizing your preparation and achieving your desired results. Each section is designed to guide you step by step, so you can tackle any question with precision and ease.
Understanding Key Math Concepts
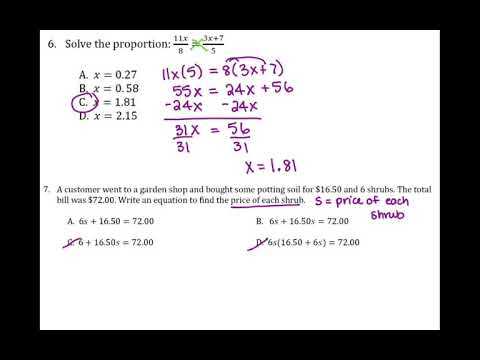
To excel in mathematics, it’s essential to have a strong grasp of the core principles that form the foundation of problem-solving. These fundamental concepts allow you to approach various types of problems with confidence and clarity. In this section, we’ll explore the most important ideas that you need to understand in order to succeed.
Each concept plays a critical role in helping you solve equations, manipulate expressions, and apply different strategies. By breaking down each topic into manageable parts, you’ll be able to understand the reasoning behind every step and recognize patterns that will make solving future problems easier.
Focusing on the underlying principles rather than just memorizing steps will give you a deeper understanding of how and why mathematical operations work the way they do. With consistent practice, these concepts will become second nature and enable you to approach any challenge with ease.
What to Expect on the Test
Preparing for a major assessment requires knowing not just the content but also what format the questions will take. Understanding the structure and types of problems you will encounter can help reduce anxiety and improve your performance. In this section, we will highlight the typical types of questions you might face and the strategies for tackling them effectively.
Common Question Formats

In most cases, you will be asked to solve equations, simplify expressions, or graph various functions. Some problems may require you to interpret word problems and translate them into mathematical expressions. Familiarizing yourself with different question types will ensure you’re prepared to approach each one confidently.
Time Management Tips
Efficiently managing your time is crucial during the assessment. Allocate enough time for each section, and avoid spending too long on any single problem. Staying calm and focused will help you complete the test within the allotted time while minimizing errors.
Common Topics in Math Reviews
When preparing for a major mathematics assessment, there are several recurring themes and concepts that are typically covered. These topics are foundational to many questions you will encounter, and mastering them will provide a strong basis for your success. This section highlights the most common subjects that are frequently emphasized in review materials.
Equations and Inequalities
One of the most common areas you’ll encounter involves solving various types of equations, including linear equations, systems of equations, and inequalities. Understanding how to manipulate variables and apply different methods, such as substitution or elimination, will help you tackle these problems effectively.
Graphing and Functions
Another important topic is graphing, particularly linear and quadratic functions. You will need to understand how to plot points on a coordinate plane and interpret the graphs of various equations. Mastering the relationship between equations and their graphical representations is essential for solving real-world problems and understanding mathematical behavior.
Step-by-Step Solutions for Practice Problems
Approaching complex problems in a logical and structured way is essential for understanding and mastering key mathematical concepts. Breaking down each problem into smaller, more manageable steps allows you to track your progress and ensures that no important detail is overlooked. This section provides a series of practice problems with clear, step-by-step instructions to help you gain confidence and proficiency.
Here’s an example of how to approach a problem, breaking down the solution process:
| Step | Action | Explanation |
|---|---|---|
| 1 | Write the equation | Start by clearly writing down the given equation to ensure you have all the necessary information. |
| 2 | Move variables to one side | Use addition or subtraction to isolate the variables on one side of the equation. |
| 3 | Simplify terms | Combine like terms and simplify both sides of the equation, if applicable. |
| 4 | Isolate the variable | Perform operations like division or multiplication to solve for the variable. |
| 5 | Check your solution | Substitute your solution back into the original equation to verify it is correct. |
By practicing this approach with various problems, you will become more comfortable with identifying key steps and applying the right methods to reach accurate solutions.
Tips for Solving Algebraic Equations
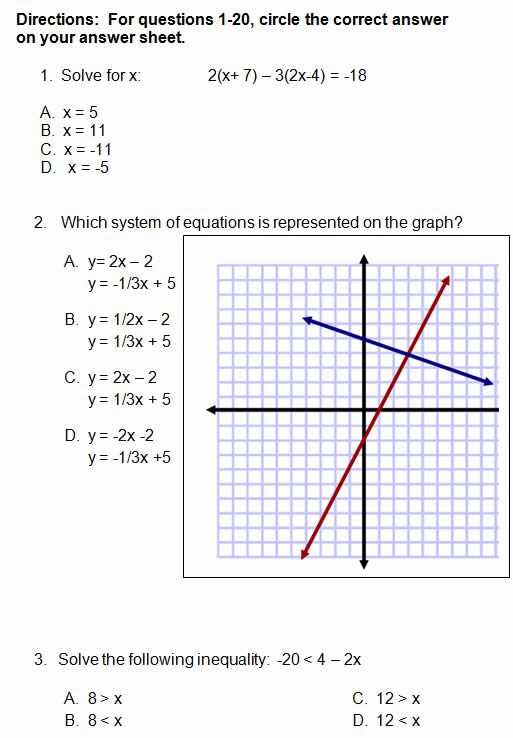
When solving mathematical equations, understanding the key techniques and strategies can make all the difference. Effective problem-solving requires a clear approach and the ability to manipulate expressions efficiently. In this section, we will discuss valuable tips that can help you approach and solve equations with greater ease and accuracy.
- Understand the structure: Always start by analyzing the equation and identifying the components–variables, constants, and operations. This will give you a clearer idea of how to proceed.
- Isolate the variable: Your goal is to get the variable on one side of the equation. Use addition, subtraction, multiplication, or division to move terms around.
- Perform inverse operations: To simplify equations, use inverse operations to eliminate terms or balance both sides of the equation.
- Simplify step-by-step: Break down complex expressions by simplifying each part. Combining like terms and factoring can make the process smoother.
- Check for consistency: Always double-check your work by substituting the solution back into the original equation to ensure it’s correct.
By following these steps and consistently practicing, you’ll develop a stronger understanding of how to handle different types of equations and solve them more efficiently.
Reviewing Linear Equations and Inequalities
Understanding how to solve linear equations and inequalities is essential for building a strong foundation in mathematics. These types of problems are common in many mathematical applications and often form the basis for more complex concepts. By mastering the techniques for solving and graphing these problems, you can simplify and approach them with confidence.
Linear equations involve variables raised only to the first power and can be solved through simple arithmetic operations. Inequalities, on the other hand, describe relationships between quantities that are not equal but follow certain limits. Whether dealing with equations or inequalities, the goal is to isolate the variable while maintaining balance on both sides of the expression.
Key steps for solving linear equations:
- Identify the equation type and isolate the variable by performing the inverse operation on both sides.
- Simplify the equation by combining like terms and reducing fractions if necessary.
- Check the solution by substituting the value of the variable back into the original equation.
For inequalities:
- Follow the same process as for equations, but remember that multiplying or dividing by a negative number reverses the inequality sign.
- Graph the solution on a number line to clearly represent the range of possible values.
Mastering these methods will help you confidently tackle linear equations and inequalities in various problem-solving scenarios. Practice and consistency are key to becoming proficient in these concepts.
Mastering Functions and Graphing Skills
Understanding how to work with functions and graph equations is crucial for tackling a wide range of mathematical problems. Mastering these skills not only enhances your ability to visualize relationships between variables but also provides a deeper understanding of how equations behave in different contexts. Whether you are dealing with linear, quadratic, or more complex functions, knowing how to graph and interpret them is key to solving problems effectively.
Functions represent relationships between two sets of values, where each input has one corresponding output. Graphing these relationships on a coordinate plane allows you to visualize how changes in one variable affect the other. As you become more comfortable with graphing techniques, you’ll gain a greater understanding of how to interpret slopes, intercepts, and other important features of a graph.
Tips for mastering functions and graphing:
- Know the function type: Identify whether you’re working with a linear, quadratic, or another type of function. This will determine the graph’s shape and behavior.
- Plot key points: Start by plotting important points such as intercepts and other points that satisfy the equation.
- Draw the graph: Use a ruler or plotting tool to connect the points smoothly. For linear functions, the graph will be a straight line; for others, it may curve.
- Understand the slope and intercepts: The slope indicates the rate of change, while the y-intercept shows where the graph crosses the y-axis. Both are crucial for understanding the behavior of the graph.
Graphing practice:
- Start with simple equations and plot them to understand the relationship between the variables.
- Use graphing tools or software to visualize more complex functions, allowing you to better interpret the graph.
- Practice drawing graphs by hand to strengthen your understanding of key features such as slopes and intercepts.
By consistently practicing these techniques, you will develop a solid foundation for solving problems involving functions and graphing, making complex concepts more accessible and easier to manage.
Factoring Techniques You Should Know
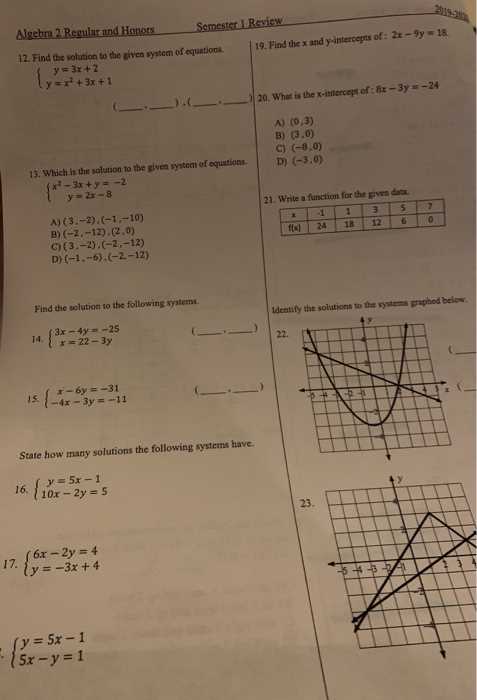
Factoring is a fundamental skill in mathematics that allows you to break down expressions into simpler components. Mastering different factoring methods can make complex problems more manageable and help you solve equations more efficiently. Whether you’re dealing with quadratic expressions or polynomials, knowing the right technique is key to finding solutions quickly.
In this section, we will explore some of the most common factoring methods that will make your work easier and more accurate. These techniques are essential for simplifying equations and solving problems involving variables and constants.
Common factoring methods:
- Factoring out the greatest common factor (GCF): Always start by looking for the GCF of all terms in the expression. This step can simplify the problem significantly.
- Factoring by grouping: For expressions with four terms, try grouping them in pairs. Factor each pair and then look for a common binomial factor.
- Factoring trinomials: For quadratics in the form of ax² + bx + c, find two numbers that multiply to ac and add to b. This method works best for factoring trinomials.
- Difference of squares: When you have two perfect squares separated by subtraction, apply the formula (a² – b²) = (a + b)(a – b) to factor it easily.
- Sum or difference of cubes: Recognize when you have cubes of numbers, and use the special formulas for factoring (a³ + b³) = (a + b)(a² – ab + b²) and (a³ – b³) = (a – b)(a² + ab + b²) .
By applying these factoring methods, you can efficiently simplify expressions and solve a variety of problems. Practice is key, as the more you work with different expressions, the more intuitive these methods will become.
Solving Word Problems Effectively
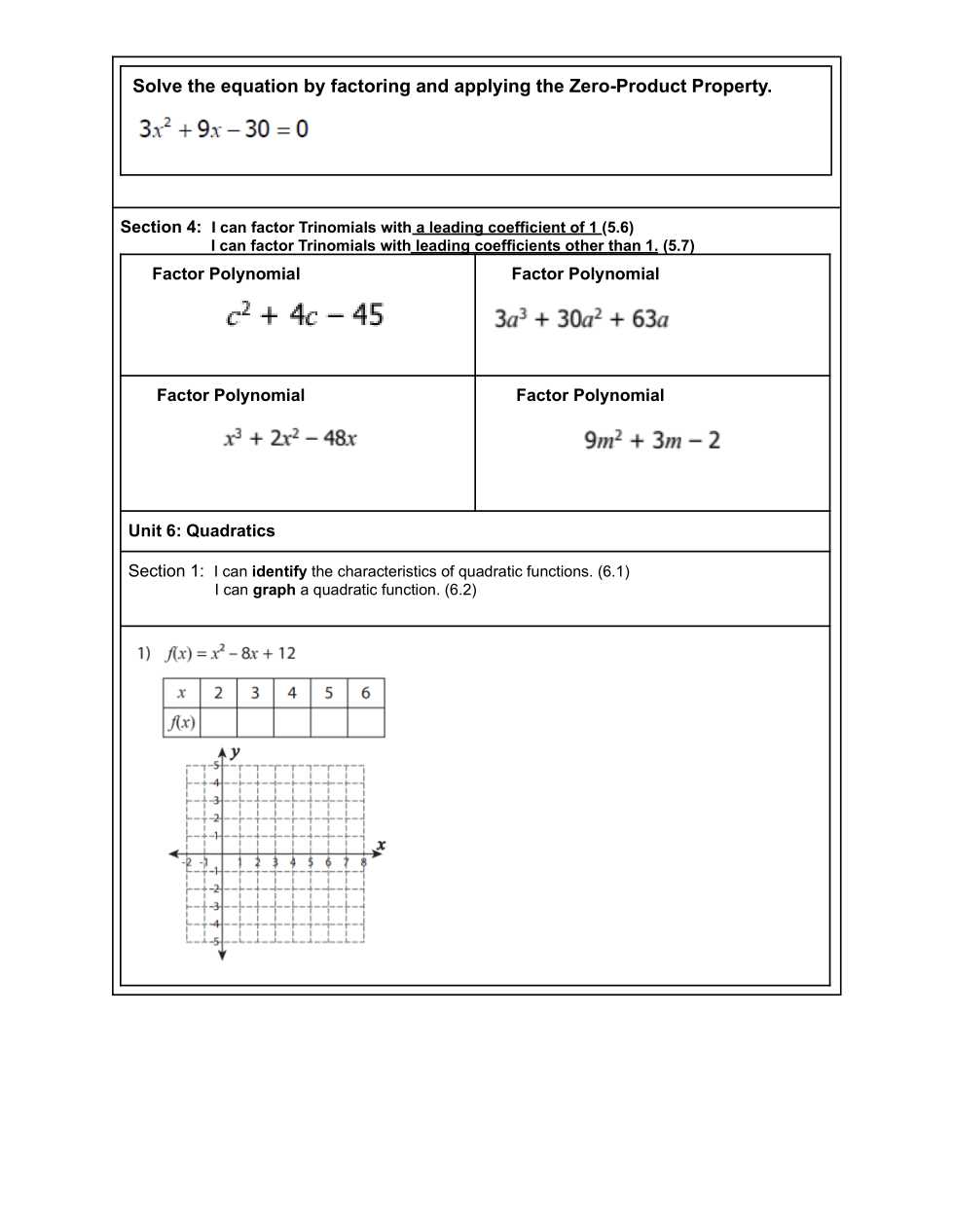
Word problems are an essential part of mathematics, requiring you to apply mathematical concepts to real-world situations. The key to solving these problems efficiently is breaking them down step by step, interpreting the information correctly, and translating it into a mathematical equation. While word problems can seem overwhelming, mastering the process can significantly improve your ability to solve complex questions.
The first step in approaching a word problem is to understand what the problem is asking. Carefully read the text to identify the variables, constants, and relationships between different elements. Once you’ve broken down the information, the next step is to create an equation that models the situation. From there, you can solve the equation and interpret the results in the context of the problem.
Steps for solving word problems:
- Read the problem carefully: Understand what the question is asking. Identify known quantities and what needs to be found.
- Identify variables: Assign a variable to represent the unknown quantity in the problem.
- Translate the words into equations: Look for key phrases such as “total,” “difference,” “product,” or “sum” to form an equation that models the scenario.
- Solve the equation: Use appropriate mathematical methods to solve the equation you’ve set up.
- Check your solution: Once you’ve solved the equation, make sure to review the context of the problem and ensure your answer makes sense.
Example of solving a word problem:
| Problem | Solution |
|---|---|
| A car travels 60 miles per hour for 2 hours. How far does it travel? | To find the distance, multiply speed by time. 60 miles/hour × 2 hours = 120 miles. |
By following these steps and practicing regularly, you’ll become more confident and efficient in solving word problems. The more you practice, the easier it becomes to recognize patterns and approaches to tackle different types of word problems.
Exploring Systems of Equations
Systems of equations involve solving multiple equations simultaneously to find the values of variables that satisfy all equations in the system. These problems are encountered when dealing with real-world scenarios that involve more than one constraint or condition. The process of solving a system requires determining where two or more equations intersect, which represents the common solution for the variables involved.
There are several methods to solve systems of equations, each offering different advantages depending on the situation. The most common methods are graphing, substitution, and elimination. Each approach provides a way to find the solution set, which may include one solution, no solution, or infinitely many solutions depending on the system.
Graphing Method
Graphing involves plotting each equation on a coordinate plane. The point where the lines intersect represents the solution to the system. This method is most useful when dealing with simple equations, but may be less effective when precision is needed.
Substitution and Elimination Methods
For more complex systems, substitution and elimination are often more efficient. The substitution method involves solving one equation for one variable and substituting that expression into the other equation. The elimination method focuses on adding or subtracting equations to eliminate one of the variables, making it easier to solve for the remaining variable.
By mastering these methods, you can tackle a wide variety of problems involving systems of equations. Each method has its strengths, and with practice, you’ll be able to choose the most effective approach depending on the problem at hand.
Understanding Quadratic Equations
Quadratic equations are polynomial expressions that involve a variable raised to the power of two. These equations often appear in problems related to motion, area, and optimization. Solving them can lead to finding the values of variables that satisfy the given conditions. There are several methods available to solve these types of equations, each with its own advantages depending on the situation.
One key to understanding quadratic equations is recognizing their general form, which is typically written as:
- ax² + bx + c = 0
Where ‘a’, ‘b’, and ‘c’ are constants, and ‘x’ is the variable to be solved. Depending on the values of ‘a’, ‘b’, and ‘c’, a quadratic equation can have zero, one, or two solutions.
Methods for Solving Quadratic Equations

There are three main methods used to solve quadratic equations:
- Factoring: This method involves rewriting the quadratic equation as a product of two binomials. It is effective when the equation can be factored easily.
- Quadratic Formula: This formula is a universal tool for solving any quadratic equation, given as:
- x = (-b ± √(b² – 4ac)) / 2a
Discriminant and Solution Types
The discriminant, found within the quadratic formula (b² – 4ac), plays a crucial role in determining the nature of the solutions:
- If the discriminant is positive, the equation has two real solutions.
- If the discriminant is zero, the equation has exactly one real solution.
- If the discriminant is negative, the equation has no real solutions, but two complex solutions.
By mastering these techniques and understanding the discriminant, you can confidently solve any quadratic equation and determine the nature of its solutions.
Working with Rational Expressions
Rational expressions are mathematical expressions that involve fractions with polynomials in both the numerator and the denominator. These expressions can be simplified, added, subtracted, multiplied, and divided, much like regular fractions. However, working with them requires an understanding of specific rules and techniques to handle the variables and operations properly.
The first step in working with rational expressions is to simplify them. This often involves factoring both the numerator and denominator to identify common factors. Once these factors are canceled out, the expression becomes simpler and easier to manipulate. It’s essential to remember that the denominator cannot be zero, as division by zero is undefined.
Another key concept when dealing with rational expressions is finding the least common denominator (LCD). This is necessary when adding or subtracting rational expressions with different denominators. The LCD is the smallest expression that both denominators can divide into evenly, allowing the fractions to be combined.
Multiplying and dividing rational expressions is more straightforward, as it involves multiplying the numerators and denominators directly. However, like any fraction multiplication, it’s important to simplify the result by canceling common factors between the numerator and the denominator.
By mastering the manipulation of rational expressions, you can efficiently solve a wide range of problems involving fractions with polynomials. Understanding how to simplify, combine, and transform these expressions is an important skill in many areas of mathematics.
Examining Exponents and Polynomials
Exponents and polynomials are foundational concepts in mathematics that are essential for understanding higher-level topics. Exponents indicate how many times a base number is multiplied by itself, while polynomials are algebraic expressions consisting of variables and coefficients, combined using addition, subtraction, and multiplication. Both are crucial when it comes to simplifying and solving various mathematical problems.
Understanding Exponents
Exponents represent repeated multiplication. For example, the expression 23 means multiplying 2 by itself three times (2 × 2 × 2 = 8). The rules for exponents, such as the product rule, quotient rule, and power rule, help simplify calculations involving powers of numbers and variables. These rules allow for more efficient manipulation of expressions that include exponents.
Working with Polynomials

Polynomials are expressions that consist of terms connected by addition or subtraction. Each term includes a variable raised to an exponent and is multiplied by a coefficient. For example, the expression 3x2 + 2x – 5 is a polynomial. The process of adding, subtracting, and multiplying polynomials involves combining like terms and applying distribution rules. When multiplying polynomials, the distributive property is used to expand expressions, ensuring each term is accounted for.
Both exponents and polynomials require practice to master, but with the proper strategies, handling these expressions becomes manageable. Whether simplifying complex expressions or solving equations, these concepts form the basis for many other areas in mathematics.
Reviewing Radical Expressions and Roots

Radical expressions and roots play a significant role in mathematics, especially when solving equations and simplifying complex expressions. A radical expression involves roots, which represent the inverse operation of exponentiation. Understanding how to work with roots and simplify radical expressions is crucial for mastering more advanced mathematical concepts.
At the core of radical expressions is the concept of roots. A root is a number that, when multiplied by itself a certain number of times, yields the original value. The square root, cube root, and higher-order roots are common types. For example, the square root of 9 is 3 because 3 × 3 equals 9. Similarly, the cube root of 8 is 2 because 2 × 2 × 2 equals 8.
Simplifying Radical Expressions
Simplifying radical expressions involves breaking down complex roots into simpler forms. This often requires factoring out perfect squares or cubes and applying the properties of exponents. For example, to simplify √50, we recognize that 50 = 25 × 2, and thus √50 = √(25 × 2) = 5√2.
Operations with Radicals
When working with radical expressions, performing operations such as addition, subtraction, multiplication, and division requires careful attention to the terms involved. For instance, when adding or subtracting radicals, the radicands (the numbers under the radical sign) must be the same. If they are not, simplification or further manipulation may be necessary to combine them effectively.
| Expression | Simplified Form |
|---|---|
| √18 | 3√2 |
| √32 | 4√2 |
| √72 | 6√2 |
By mastering the rules and techniques of working with radical expressions and roots, one can simplify and solve equations more efficiently. This skill is essential for success in many areas of mathematics, from basic problem-solving to more advanced topics involving functions and equations.
Prepping for Word Problems
Word problems are a critical aspect of mathematics that test your ability to translate real-life scenarios into mathematical equations. Mastering these types of problems requires a systematic approach to break down the information, identify key variables, and apply the correct operations to find a solution. Preparation is key to handling word problems with confidence and accuracy.
When tackling word problems, the first step is to carefully read and understand the problem’s context. Often, the challenge lies in recognizing what information is essential and how to relate it to mathematical concepts. To ensure a smooth problem-solving process, follow these important steps:
- Read the Problem Thoroughly: Take your time to fully understand what the problem is asking. Identify keywords that will help you recognize the operations needed (e.g., “sum,” “difference,” “product,” “quotient”).
- Identify Variables: Assign variables to unknown quantities. This will make it easier to form an equation and solve the problem.
- Write an Equation: Based on the information provided, set up an equation that represents the problem. This equation is the foundation for solving it.
- Solve the Equation: Use appropriate mathematical techniques to solve the equation step by step.
- Check Your Solution: Once you have a solution, go back to the problem to ensure that your answer makes sense in the context of the real-world scenario.
To further prepare for word problems, it’s important to practice with a variety of problem types, ranging from basic arithmetic to more complex situations. Here are some common problem types to focus on:
- Distance, Rate, and Time Problems: These problems involve calculating travel time, speed, or distance, and are often solved using the formula Distance = Rate × Time.
- Mixture Problems: These require you to work with different substances or solutions and find the resulting concentration or amount after mixing them.
- Work Problems: These problems involve rates of work, such as how long it takes for one or more people to complete a task when working together.
- Age Problems: These problems focus on the relationship between ages at different points in time and are solved by setting up equations that express the relationship.
By practicing regularly and following a structured approach, you can improve your skills in solving word problems and feel more confident when faced with these challenges in mathematical assessments.
Strategies for Time Management During the Test
Effective time management is crucial for performing well on any assessment. The ability to allocate sufficient time to each section of the test can make the difference between finishing on time and rushing through questions. Developing a strategic approach to managing your time will help reduce stress and allow you to approach each problem with focus and clarity.
Here are some helpful strategies to ensure that you manage your time wisely during the test:
- Familiarize Yourself with the Test Format: Before starting, take a moment to quickly scan the test. Understanding the structure–whether it’s multiple-choice, short-answer, or long-form problems–will allow you to better allocate your time to each section.
- Prioritize the Easier Questions: Begin by answering questions you feel most confident about. This will help you build momentum and ensure that you collect points quickly. Leave more challenging questions for later.
- Time Each Section: Divide the total time by the number of sections or problems. This will give you a rough idea of how long you should spend on each part. Stick to this limit to prevent spending too much time on any one question.
- Set Time Limits for Each Question: For longer problems, set a personal time limit. If you haven’t found a solution within that time, move on and revisit it later. This will ensure you don’t get stuck and waste valuable time.
- Skip and Return to Difficult Questions: If you encounter a question that’s taking too long, skip it and continue to others. You can always return to it once the easier questions are completed.
In addition to these strategies, consider the following tips for maintaining focus and efficiency throughout the test:
- Stay Calm: Anxiety can lead to poor time management. If you feel stressed, take a deep breath and refocus. Staying calm will help you think more clearly and work more efficiently.
- Practice Time Management in Advance: Before the test, practice with timed mock tests. This will help you get comfortable with pacing and identify areas where you need to improve.
- Keep Track of Time: Keep an eye on the clock but don’t obsess over it. Regularly check your time to ensure you’re staying on track without getting distracted.
By using these strategies, you can approach your test with greater confidence and effectively manage your time, ensuring that you complete all sections within the allotted time and maximize your potential score.
How to Avoid Common Mistakes
When preparing for and taking assessments, it’s easy to fall into certain pitfalls that can lead to avoidable errors. These mistakes can often be traced back to a lack of focus, misinterpretation of questions, or rushed answers. Being aware of common errors and adopting strategies to prevent them can make a significant difference in your performance.
Here are some tips to help you avoid common mistakes during your test:
- Read Questions Carefully: One of the most common mistakes is misunderstanding what the question is asking. Always take a moment to read the question thoroughly before jumping into an answer. Pay attention to words like “not,” “all,” or “except,” as they can change the meaning of the problem.
- Double-Check Your Work: It’s easy to make simple errors while solving problems, especially under time pressure. Before moving on to the next question, quickly review your solution to ensure there are no calculation or logical mistakes.
- Watch for Sign Errors: Negative signs are often overlooked. A small mistake like misplacing a minus sign can lead to incorrect results. Pay extra attention when working with negative numbers, especially in equations or when multiplying/dividing.
- Don’t Rush: Time management is important, but rushing through questions can lead to careless mistakes. Take your time to carefully solve each problem, ensuring you understand the steps involved. If you feel rushed, take a deep breath and refocus.
- Check Units and Labels: When dealing with word problems or equations involving measurements, make sure that you’re using the correct units. Converting between units, such as from centimeters to meters, can be a source of error if not done properly.
- Stay Organized: Keep your work neat and well-organized. Jumbled or unclear work can lead to confusion and mistakes when reviewing or when working with complex problems.
By staying aware of these common mistakes and taking the time to double-check your work, you can minimize errors and increase your confidence as you work through problems. The more you practice these habits, the less likely you are to make preventable mistakes during any assessment.