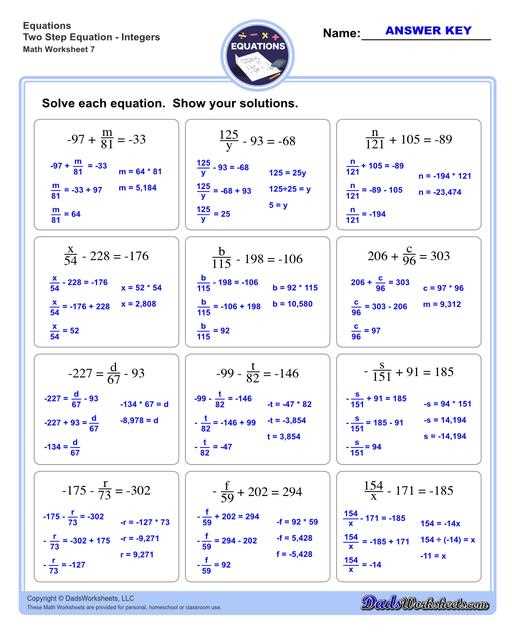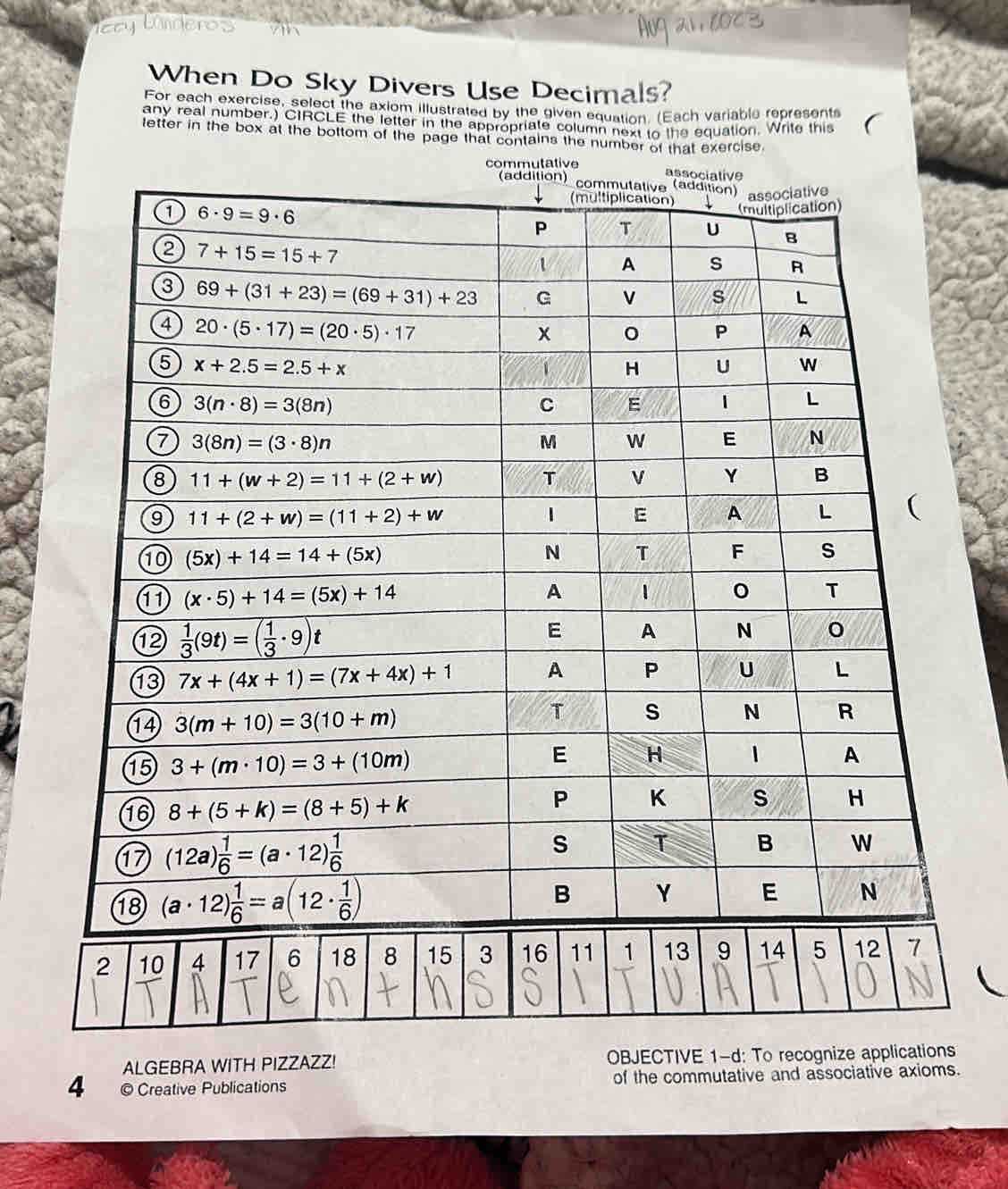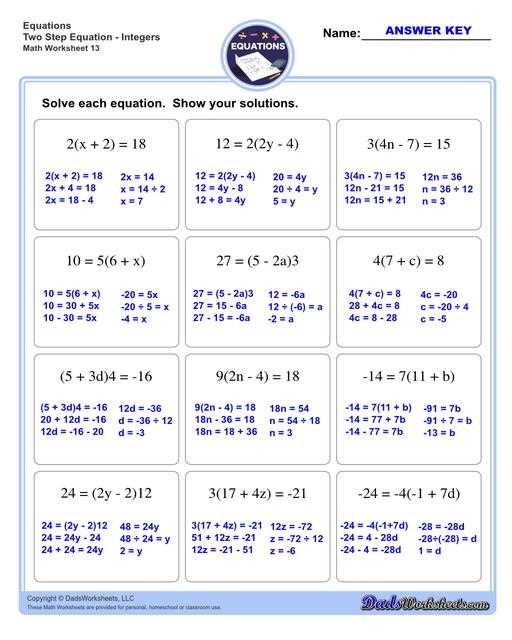
Mathematical challenges often provide a unique opportunity to develop critical thinking and logical reasoning. One specific section focuses on enhancing these skills by presenting engaging and thought-provoking exercises. By working through these tasks, learners can uncover new strategies for approaching numerical puzzles.
In this section, the emphasis is placed on exploring patterns and applying innovative solutions to seemingly complex scenarios. Understanding these concepts not only aids in completing the exercises but also builds a foundation for tackling similar problems in the future.
Through careful analysis and systematic approaches, students can navigate the intricacies of each task. Key strategies involve breaking problems into smaller steps and recognizing recurring themes, which simplify the process and make learning enjoyable and effective.
Understanding the Exercises on Page 91
The problems presented on this specific page are designed to enhance analytical thinking and reinforce key principles in mathematical reasoning. By engaging with these tasks, learners can refine their approach to solving structured problems and explore innovative techniques for reaching solutions.
Breaking Down Complex Scenarios
Each task on this page requires careful consideration and a methodical approach. By dissecting the components of a problem, students can identify critical elements and determine the best path to a solution. This process not only simplifies the challenge but also builds confidence in problem-solving abilities.
Recognizing Patterns and Connections
Many exercises rely on the ability to spot recurring themes or sequences. Recognizing these patterns is essential for efficient problem resolution. By paying close attention to details, learners can uncover hidden connections and apply them to solve even the most intricate challenges effectively.
Key Concepts Explained for Better Clarity
To solve any mathematical challenge effectively, it’s essential to first grasp the fundamental principles at play. By breaking down key ideas, learners can gain a deeper understanding and approach problems with greater confidence. This section highlights the core concepts that are crucial for mastering the exercises presented.
Understanding Core Principles
At the heart of many problems lie a few fundamental rules that guide the solution process. Recognizing these principles allows students to frame their thinking in a structured way, ensuring they can apply the correct methods when tackling more complex problems. This approach minimizes confusion and helps sharpen problem-solving skills.
Building Strong Problem-Solving Foundations
Once the basic concepts are clear, students can build upon them by developing a logical sequence of steps for solving more intricate tasks. Creating a foundation based on these core ideas makes it easier to approach any new challenge. Mastery of these basics not only boosts confidence but also speeds up the process of finding solutions.
Breaking Down Complex Algebra Problems
Challenging equations often appear overwhelming at first glance, but a systematic approach can simplify even the most intricate tasks. By analyzing each component step by step, learners can transform complicated problems into manageable parts, making the process more straightforward and effective.
Decomposing Problems into Smaller Steps
Large tasks often consist of smaller, interconnected pieces. By isolating these segments, students can focus on solving one element at a time. This method reduces complexity and allows for a clearer understanding of how each piece contributes to the overall solution.
Identifying Key Relationships
Many problems are built upon relationships between variables and operations. Recognizing these connections helps in forming logical sequences to solve them. By prioritizing clarity and organization, learners can uncover efficient paths to their solutions.
Strategies to Solve Tricky Equations
When facing complex numerical challenges, adopting effective strategies can make the process smoother and more logical. By employing targeted techniques, learners can approach even the most intricate tasks with confidence and clarity. This section outlines key methods to simplify and solve difficult equations efficiently.
Prioritizing Key Operations
Breaking a problem into distinct steps allows for a better focus on essential operations. Identifying which calculations to perform first ensures accuracy and reduces the likelihood of errors. A step-by-step approach is particularly useful for equations involving multiple variables.
Using Visual Aids
Tables and charts can serve as powerful tools for organizing data and tracking progress. They help in identifying patterns and simplifying the representation of complex relationships. Below is an example of how a table can assist in solving equations:
| Step | Action | Result | ||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | Isolate key variables | Simplified equation | ||||||||||||||||||
| 2 | Perform primary operations | Intermediate solution |
| Skills Developed | Benefits to Critical Thinking |
|---|---|
| Logical Reasoning | Enhances decision-making and analysis |
| Pattern Recognition | Improves understanding of complex concepts |
| Strategic Planning | Helps organize thoughts and anticipate outcomes |
Applying Math Concepts to Real Life
Mathematical principles are not confined to the classroom; they serve as powerful tools for solving everyday problems. By using numbers, patterns, and logic, individuals can make more informed decisions in various areas of life, from budgeting to planning a trip or even cooking. Applying these concepts practically can simplify complex situations and enhance problem-solving skills.
Real-world applications of mathematical thinking can be seen in many areas. For example, when shopping, individuals use basic arithmetic to calculate discounts, compare prices, or figure out total costs. Similarly, when organizing a project, understanding proportions or ratios helps allocate resources effectively. These skills go beyond theoretical exercises, providing individuals with the ability to make practical decisions quickly and accurately.
| Real-Life Application | Mathematical Concept Used |
|---|---|
| Budgeting | Arithmetic and percentages |
| Cooking | Fractions and ratios |
| Home Improvement | Geometry and measurement |
| Travel Planning | Time calculations and distance |
These examples demonstrate how mathematical thinking can be applied to improve decision-making and efficiency in daily tasks. Whether you are managing personal finances or undertaking a DIY project, the ability to analyze and solve problems using basic math concepts offers significant benefits in real-life situations.
Common Pitfalls in Algebraic Solutions
When solving mathematical equations, it’s easy to fall into certain traps that can lead to incorrect results. These common mistakes often stem from overlooked details or misapplied rules, which can complicate the process unnecessarily. Recognizing these pitfalls is essential for improving accuracy and efficiency when approaching complex problems.
One frequent error is neglecting to properly distribute terms, especially when dealing with parentheses. For example, failing to multiply each term inside parentheses by the number outside can cause significant mistakes. Another common mistake involves improper handling of negative signs, which often leads to sign errors when adding or subtracting values.
Misinterpreting Operations
Confusing the order of operations can cause calculations to go awry. The established order–parentheses, exponents, multiplication and division, addition and subtraction (PEMDAS)–should always be followed to ensure correct results. Skipping steps or rearranging operations can lead to incorrect answers, especially in more intricate equations.
Overlooking Simplification Opportunities
Many solutions can be simplified before reaching the final answer. However, students often overlook opportunities to reduce expressions early in the process. Whether it’s factoring common terms, simplifying fractions, or canceling out like terms, simplifying intermediate steps can save time and reduce the chances of errors.
Key Takeaways: Avoiding these common mistakes requires careful attention to detail and a systematic approach to problem-solving. By practicing and reviewing each step thoroughly, you can reduce the likelihood of making these errors in future calculations.
Tools to Improve Problem-Solving Speed
Enhancing problem-solving speed is crucial when tackling mathematical challenges. Whether you’re working under time constraints or simply looking to improve efficiency, certain strategies and tools can help streamline the process. By adopting a systematic approach and using the right resources, it becomes easier to solve problems more quickly and accurately.
One effective method is mastering mental math techniques, which allow you to bypass lengthy calculations and reach answers faster. This includes practicing basic operations like addition, subtraction, multiplication, and division without relying on a calculator. Additionally, understanding shortcuts such as multiplying by 10 or dividing by fractions can significantly save time during problem-solving.
Using Technology for Speed
There are numerous apps and tools designed to accelerate problem-solving by providing quick feedback and suggestions. Online calculators, equation solvers, and step-by-step guides are valuable resources for learning how to solve problems faster. These tools not only assist with checking answers but also highlight areas where time can be saved by simplifying steps or applying specific strategies.
Practice Makes Perfect
Regular practice is another essential tool for improving speed. The more you work through problems, the more familiar you become with common patterns and solutions. This familiarity allows you to identify shortcuts and recognize familiar steps, reducing the time it takes to work through each problem.
By incorporating these techniques and tools into your problem-solving routine, you can sharpen your skills and enhance your ability to solve problems swiftly and efficiently.
Learning from Answer Key Explanations
One of the most effective ways to improve problem-solving skills is by reviewing detailed explanations provided in answer keys. These explanations offer step-by-step guidance that helps learners understand the process behind each solution. Instead of simply memorizing answers, taking the time to analyze the reasoning behind each step fosters a deeper understanding of the concepts and enhances overall problem-solving abilities.
Understanding the Process
Rather than just looking at the final result, focusing on the process that leads to it is crucial for mastering the material. Here’s why examining the explanations is beneficial:
- Step-by-step breakdown: Each solution is broken down into smaller, manageable parts, making it easier to follow and replicate the process.
- Identifying common techniques: Answer keys often highlight common problem-solving methods, such as factoring, simplifying expressions, or applying formulas. Recognizing these patterns can help you apply the right approach in future problems.
- Learning from mistakes: If an error is made in a solution, the explanation often shows how to correct it, helping you avoid the same mistakes in the future.
Benefits for Retention and Confidence
Revisiting and studying the explanations reinforces your memory and builds confidence. As you internalize the methods used in solving problems, you’ll become more comfortable tackling new challenges. Over time, this strengthens both your conceptual knowledge and your ability to solve problems efficiently.
By learning from the detailed explanations provided in answer keys, you can bridge the gap between theory and practice, allowing for continuous growth and improvement in problem-solving skills.
Making Sense of Complex Number Patterns
Understanding patterns in numbers that involve real and imaginary components can seem challenging at first, but it becomes more manageable once you recognize the recurring relationships. By identifying these patterns, one can predict and analyze future outcomes, making it easier to solve related problems. Breaking down complex numbers into their real and imaginary parts and observing how they interact can reveal underlying structures that simplify solutions.
Identifying Key Patterns
When working with complex numbers, the key to understanding their patterns is recognizing how their components behave. Here are some common patterns to look for:
- Repetition of powers: When raising complex numbers to increasing powers, certain values repeat after a certain number of iterations. Identifying these cycles helps predict results without recalculating each time.
- Symmetry: Many complex numbers exhibit symmetry when plotted on the complex plane. These symmetrical properties can help you predict the behavior of related numbers or simplify calculations.
- Magnitude and phase patterns: The magnitude and angle (phase) of complex numbers often follow predictable paths when transformed, making it easier to calculate the results of operations like multiplication or division.
Using Patterns to Solve Problems
Recognizing and applying these patterns can significantly speed up solving problems involving complex numbers. Once you are familiar with the behavior of numbers in different operations, you can quickly calculate powers, roots, and other transformations without needing to go through every step. This knowledge enables a deeper understanding and more efficient handling of problems involving complex numbers.
By observing and understanding the patterns in complex number behavior, you can approach problems more strategically and improve both accuracy and speed in solving them.
How to Approach Challenging Math Questions
When faced with difficult mathematical problems, it’s essential to break down the question into manageable steps. Instead of getting overwhelmed, focus on understanding the structure of the problem and applying logical strategies to find a solution. A systematic approach not only simplifies complex questions but also builds confidence in tackling future challenges.
Step-by-Step Breakdown
Start by carefully reading the problem to understand what is being asked. Identify the given information and the unknowns, then determine the most appropriate method to solve it. Here are a few steps to consider:
- Read the problem multiple times: Understanding every part of the question ensures you don’t miss important details.
- Organize your work: Clearly lay out all given information and separate each part of the question.
- Identify patterns: Look for similarities with problems you’ve solved before or recognize recurring strategies that may help.
Stay Calm and Persistent
It’s easy to get frustrated when a problem seems too challenging. However, maintaining patience is crucial. If you’re stuck, take a step back and approach the problem from a different angle. Sometimes, revisiting simpler related concepts can provide insight into more complex questions. Don’t hesitate to ask for help or search for alternative methods to solve the problem.
By staying organized and persistent, you’ll find that even the toughest problems become more manageable.
The Role of Fun in Learning Mathematics
Incorporating enjoyment into the learning process can significantly improve engagement and understanding of complex concepts. When students find joy in solving mathematical problems, they are more likely to remain motivated and eager to tackle new challenges. By turning problem-solving into a rewarding experience, learners can deepen their comprehension while having fun.
Benefits of a Fun Approach
Engaging students in enjoyable activities related to mathematical tasks can lead to numerous positive outcomes:
- Increased Retention: When learning is enjoyable, the material is more likely to be retained. Fun activities make concepts stick in students’ minds longer.
- Enhanced Creativity: Approaching problems in creative ways helps learners think outside the box and discover novel solutions.
- Reduced Stress: Fun learning environments can decrease the pressure often associated with challenging subjects, leading to a more relaxed mindset.
Making Math Enjoyable
Incorporating games, puzzles, and interactive activities into lessons is a great way to make learning mathematics more enjoyable. These activities encourage exploration and critical thinking in a low-pressure environment. For example, using riddles or competitive challenges related to problem-solving can make even the most complex tasks feel more like a fun game than a test of skill.
By transforming the learning experience into something enjoyable, students can build a positive relationship with mathematics, which can lead to a deeper understanding and greater success in the subject.