Algebra 2 Core Connections Answers and Solutions

When it comes to mastering higher-level mathematics, it’s essential to have a strong grasp of fundamental principles and problem-solving strategies. Navigating through complex equations and functions requires more than just rote memorization–it demands a deep understanding of concepts and the ability to apply them effectively. This section is dedicated to providing structured support for those seeking to enhance their mathematical skills in these advanced areas.

From solving systems of equations to exploring the intricacies of polynomial expressions, practical strategies and detailed solutions are offered to guide learners at each step. Whether you’re tackling challenging functions, inequalities, or word problems, having access to clear explanations can significantly improve your ability to tackle these topics confidently.

As you progress through various types of problems, it becomes increasingly important to recognize patterns and develop efficient methods for tackling each challenge. With the right tools and strategies, any complex topic can become manageable, empowering learners to not only find solutions but also build a stronger foundation for future success.

Algebra 2 Core Connections Answers Guide

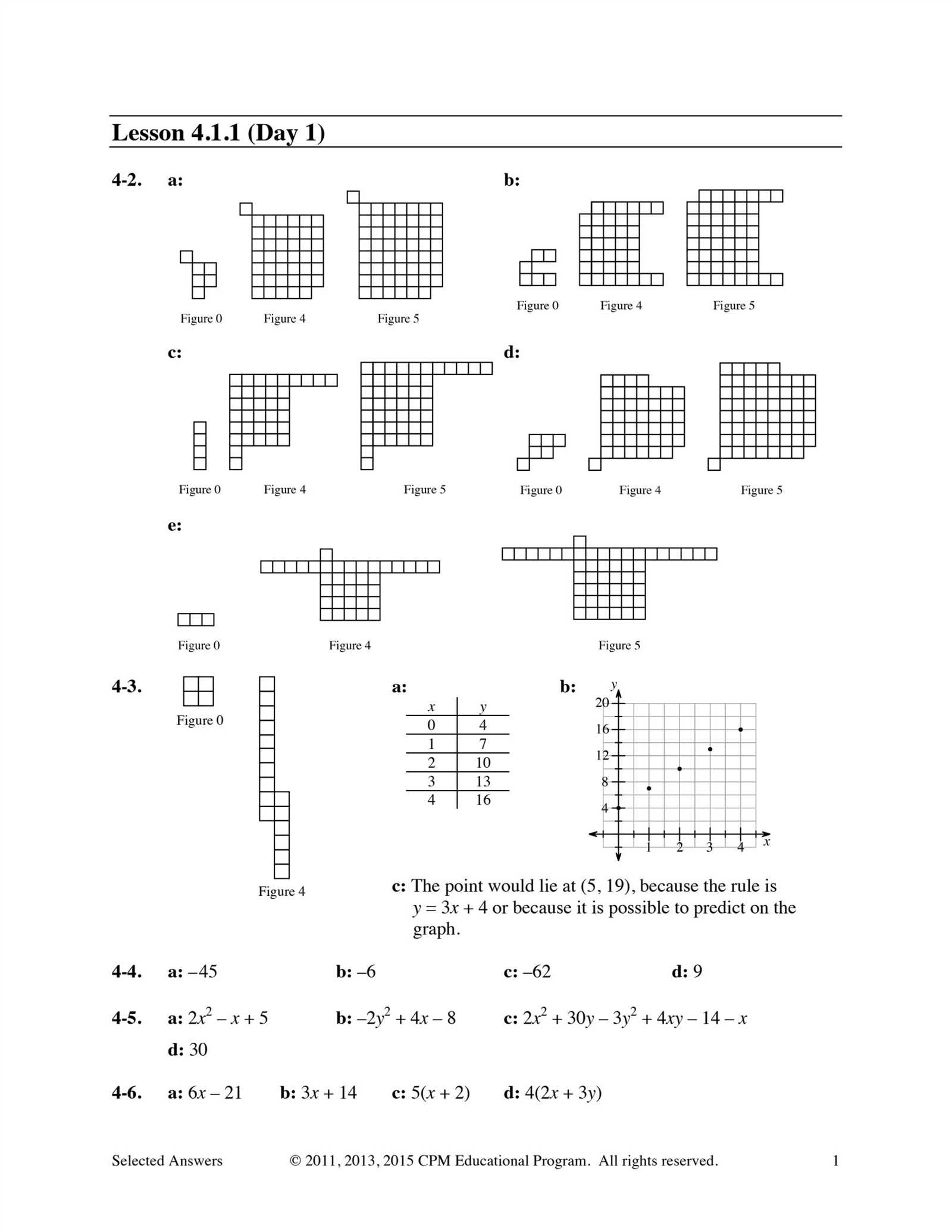
This guide is designed to help learners navigate through complex mathematical problems, providing clear and concise explanations at each stage. Understanding how to approach difficult problems is key to mastering advanced concepts. With step-by-step instructions and detailed walkthroughs, this section ensures that every essential topic is covered, enabling learners to build confidence in their skills.

From solving intricate equations to interpreting graphical data, each section breaks down challenging material into manageable steps. The focus is not only on finding solutions but also on understanding the underlying principles that govern each method. By following this approach, you can develop a deeper understanding and improve your ability to tackle future mathematical challenges.

By applying these techniques, students will learn to approach problems logically, recognize patterns, and refine their problem-solving strategies. This guide serves as an invaluable resource for anyone looking to strengthen their mathematical foundation and excel in advanced problem-solving tasks.

Understanding Algebra 2 Core Concepts

Mastering advanced mathematical topics requires a solid foundation in key principles and an ability to apply them in various scenarios. In this section, we explore essential mathematical ideas that are central to higher-level problem solving. Understanding these concepts not only helps in solving specific problems but also in developing a broader mathematical mindset that can be applied across different areas.

Key Topics to Focus On

Among the most important concepts are solving equations, working with functions, and understanding relationships between variables. These ideas serve as building blocks for more complex problems. By thoroughly grasping how equations are structured and how they can be manipulated, you will gain the confidence needed to handle more challenging material as you progress.

Building a Strong Foundation

It’s essential to be comfortable with both theoretical and practical aspects of these concepts. This includes not only knowing the methods for solving specific types of problems but also understanding why these methods work. By developing a deep understanding of these fundamentals, students are better equipped to approach increasingly complex problems with greater ease and accuracy.

Step-by-Step Solutions for Algebra Problems

Breaking down complex mathematical challenges into smaller, more manageable steps is key to mastering advanced topics. This section focuses on providing clear, step-by-step guidance for solving common types of problems, ensuring that learners can follow a logical sequence to find solutions with ease. Each step is explained in detail, allowing you to see how each part of the problem leads to the next, making the process more understandable.

Solving Equations

To tackle equations efficiently, follow these steps:

Identify the type of equation (linear, quadratic, etc.).
Isolate the variable on one side of the equation.
Apply appropriate operations (addition, subtraction, multiplication, division) to simplify the equation.
Check your solution by substituting it back into the original equation.

Working with Functions

When dealing with functions, the following approach is effective:

Define the function and identify its domain and range.
Substitute known values for variables to evaluate the function.
Graph the function to visualize its behavior and relationships.
Analyze key features such as intercepts, slopes, and asymptotes.

By following a structured approach to each type of problem, learners will develop stronger problem-solving skills, enabling them to tackle even the most complex equations with confidence.

How to Solve Systems of Equations

Solving a system of equations involves finding values for the variables that satisfy all the equations simultaneously. This process can be approached in different ways, depending on the type and complexity of the system. Understanding these methods allows you to select the most efficient strategy for each problem.

Methods for Solving Systems

There are several common methods to solve systems of equations:

Substitution Method: Solve one equation for one variable, then substitute that expression into the other equation.
Elimination Method: Add or subtract the equations to eliminate one of the variables, then solve for the remaining variable.
Graphing Method: Graph both equations on the same coordinate plane and identify the point where they intersect, which gives the solution.

Steps to Solve Using Substitution

To solve a system using substitution, follow these steps:

Choose one equation and solve for one variable in terms of the other.
Substitute this expression into the second equation to create a single-variable equation.
Solve the new equation for the remaining variable.
Substitute the value back into the first equation to find the value of the other variable.

Steps to Solve Using Elimination

For the elimination method, follow these steps:

Align the equations so that like terms are in the same columns.
Multiply one or both equations if necessary to get the coefficients of one variable to be opposites.
Add or subtract the equations to eliminate one variable.
Solve for the remaining variable, then substitute back into one of the original equations to find the other variable.

By mastering these methods, you can confidently solve systems of equations and apply these techniques to more advanced problems.

Mastering Polynomial Functions in Algebra 2

Polynomial functions are fundamental to advanced mathematics, and mastering them is key to understanding a wide range of mathematical topics. These functions involve variables raised to various powers and are essential for modeling real-world scenarios, solving equations, and analyzing patterns. Grasping the core principles behind polynomials allows you to solve more complex problems and explore their behavior in various contexts.

Understanding Polynomial Structure

Polynomial functions are composed of terms that include a variable raised to a non-negative integer exponent, multiplied by a coefficient. The degree of the polynomial is determined by the highest exponent in the expression. To master these functions, it’s crucial to understand how each term contributes to the overall behavior of the function and how the degree affects the graph of the polynomial.

Key Techniques for Working with Polynomials

There are several important techniques to focus on when working with polynomials:

Factoring: Breaking down a polynomial into simpler components that can be solved or analyzed more easily.
Graphing: Understanding how the degree and coefficients affect the shape and intercepts of the polynomial’s graph.
Solving Polynomial Equations: Using methods like synthetic division, long division, and the Rational Root Theorem to find solutions.

By becoming proficient in these techniques, you will be able to solve polynomial equations, graph polynomials, and analyze their properties with confidence.

Strategies for Solving Quadratic Equations

Quadratic equations are a fundamental part of advanced mathematics, and knowing how to solve them efficiently is crucial. These equations often arise in various contexts, such as physics, engineering, and economics, making it important to understand different strategies for finding their solutions. There are several methods to approach solving these types of equations, and each one is useful depending on the specific problem you’re facing.

Common Methods for Solving Quadratics

The following methods are commonly used to solve quadratic equations:

Method	Description	When to Use
Factoring	Express the quadratic as a product of two binomials, then set each factor equal to zero.	When the quadratic easily factors into simple terms.
Quadratic Formula	Use the formula x = (-b ± √(b² – 4ac)) / 2a to find the roots.	When factoring is difficult or impossible, or to find exact solutions.
Completing the Square	Rearrange the equation and add a constant to form a perfect square trinomial.	When the equation does not factor easily or for understanding the vertex form.

Choosing the Right Strategy

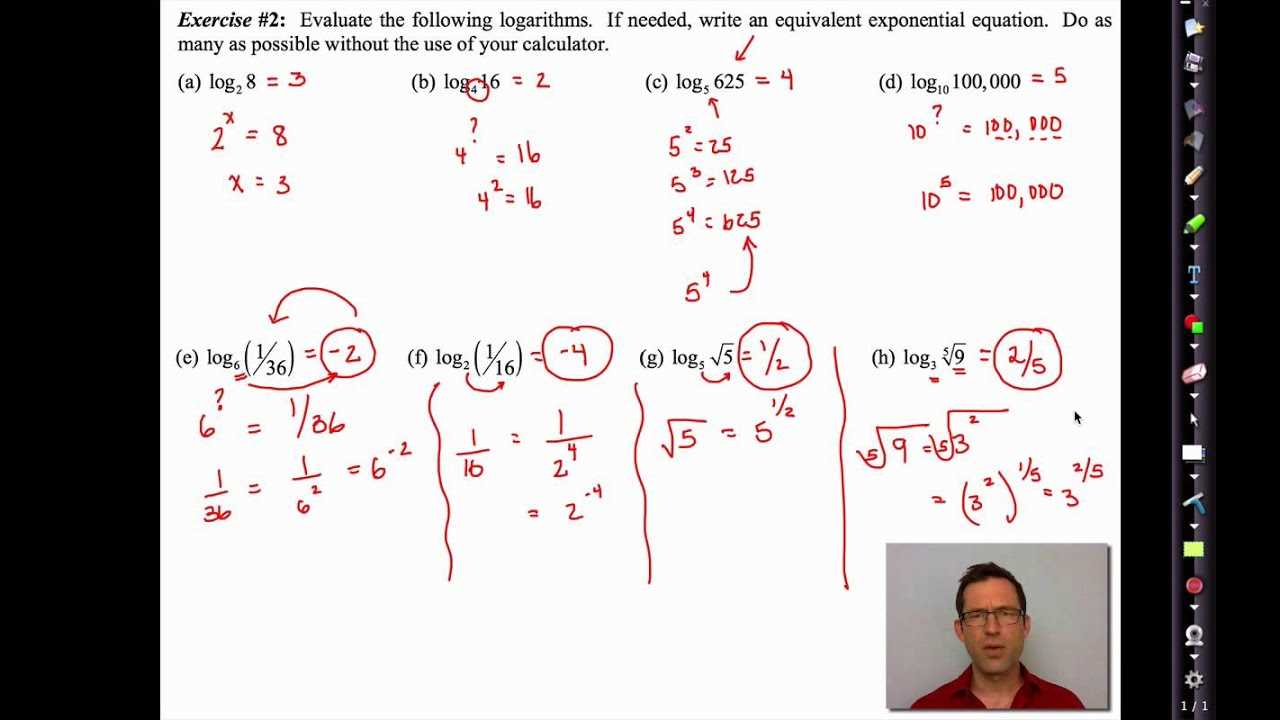
Choosing the right method depends on the structure of the equation and the context of the problem. For simple quadratics, factoring is often the fastest and easiest way to find the solution. If the equation does not factor easily, the quadratic formula provides a reliable method for finding the roots. Completing the square is especially useful when you need to convert the equation into vertex form for graphing or analysis.

By practicing these strategies, you will be able to approach any quadratic equation with confidence and choose the best method for solving it efficiently.

Exploring Rational Expressions and Equations

Rational expressions and equations involve the ratio of two polynomials, and they are an essential part of advanced mathematics. Understanding how to manipulate and solve these expressions is crucial for tackling more complex problems in various fields, such as engineering, physics, and economics. This section focuses on how to simplify, solve, and analyze rational expressions, as well as the techniques for dealing with rational equations effectively.

Simplifying Rational Expressions

To simplify rational expressions, the goal is to reduce the expression to its simplest form. This can be achieved by factoring both the numerator and the denominator and canceling out common factors. For example:

Factor both the numerator and denominator.
Identify and cancel any common factors.
Ensure the expression is in its simplest form, without any factors that can be further reduced.

Solving Rational Equations

When solving equations that contain rational expressions, the process typically involves eliminating the denominators. To do this, multiply both sides of the equation by the least common denominator (LCD). Here are the steps:

Identify the denominators in the equation.
Multiply both sides of the equation by the LCD to eliminate the denominators.
Simplify and solve the resulting equation as a regular polynomial equation.
Check for extraneous solutions by substituting back into the original equation.

By mastering these techniques, you can effectively simplify complex rational expressions and solve equations involving fractions, making it easier to approach higher-level mathematical concepts.

Working with Exponents and Radicals

Understanding exponents and radicals is essential for simplifying expressions and solving equations in advanced mathematics. These concepts are closely related, as exponents represent repeated multiplication, while radicals involve the inverse process of finding roots. Mastery of these topics allows for greater flexibility in solving problems and manipulating mathematical expressions more efficiently.

Exponent Rules and Properties

Exponents follow a set of rules that simplify operations involving powers. These rules help to manage multiplication, division, and powers of powers, among other operations:

Product Rule: When multiplying terms with the same base, add the exponents. For example, a^m × aⁿ = a^m+n.
Power Rule: When raising a power to another power, multiply the exponents. For example, (a^m)ⁿ = a^m×n.
Quotient Rule: When dividing terms with the same base, subtract the exponents. For example, a^m ÷ aⁿ = a^m-n.

Working with Radicals

Radicals are the inverse of exponents and are often used to express roots. Understanding how to simplify and manipulate radical expressions is key to solving many types of equations:

Square Roots: The square root of a number is the value that, when multiplied by itself, gives the original number. For example, √a × √a = a.
Cube Roots and Higher: The cube root of a number is the value that, when cubed, gives the original number. This concept can be extended to higher roots as well.
Rationalizing the Denominator: This process involves eliminating radicals from the denominator of a fraction by multiplying both the numerator and denominator by an appropriate expression.

By applying these rules and techniques, you can simplify complex expressions, solve equations involving exponents and roots, and gain a deeper understanding of their interrelationships.

Tips for Solving Word Problems in Algebra

Word problems are often seen as one of the more challenging aspects of mathematics. They require the ability to translate real-world situations into mathematical expressions and solve them using the concepts you’ve learned. Developing a structured approach to these problems can make them easier to tackle and ensure you don’t miss important details in the process.

Understanding the Problem

Before jumping into the calculations, it’s essential to first understand what the problem is asking. Break down the information and identify the key variables. Here are some steps to follow:

Read carefully: Read the problem more than once to ensure you fully grasp the scenario.
Identify what is given: Highlight or note down all the known information provided in the problem.
Determine what is unknown: Understand what the problem is asking you to find and define those variables.

Formulating the Equation

Once you have a clear understanding of the problem, the next step is to set up an equation that represents the situation. Here are some tips for doing so:

Assign variables: Use letters like x, y, or z to represent the unknown values in the problem.
Translate words into math: Convert phrases such as “total amount,” “difference,” or “product” into mathematical operations like addition, subtraction, or multiplication.
Check for units: Ensure that all quantities are in the same unit of measurement before proceeding.

Solving and Verifying the Solution

Once you’ve formed your equation, you can proceed to solve it using appropriate techniques. After finding the solution, it’s crucial to verify your answer to make sure it makes sense within the context of the problem:

Use the correct methods: Whether it’s solving for a variable, simplifying expressions, or factoring, apply the right techniques based on the equation.
Check your answer: Substitute your solution back into the original problem to see if it satisfies the given conditions.
Ensure completeness: Double-check that you’ve answered all parts of the problem.

By following these tips and practicing regularly, you’ll improve your ability to solve word problems and gain a deeper understanding of how mathematical concepts apply to real-world situations.

Understanding Functions and Their Graphs

Functions are fundamental concepts in mathematics, representing relationships between inputs and outputs. A function takes an input, processes it, and produces an output, which can be graphed to visually represent these relationships. Understanding how to interpret and manipulate functions is key to solving many types of mathematical problems and analyzing real-world phenomena.

Key Properties of Functions

There are several key properties to consider when working with functions. These properties help define the behavior and characteristics of the function in question:

Domain: The set of all possible input values for which the function is defined.
Range: The set of all possible output values that the function can produce.
Continuity: Whether the function has any breaks or gaps in its graph.
Increasing or Decreasing: Describes whether the function’s graph moves upward or downward as you move from left to right.

Graphing Functions

Graphing is a powerful tool to visualize the behavior of functions. Here are the basic steps for graphing a function:

Plot Points: Choose a set of input values (x-values), calculate the corresponding output values (y-values), and plot these points on the graph.
Connect the Points: Draw a smooth curve or line through the points. The shape of this curve depends on the type of function.
Analyze the Graph: Identify key features, such as intercepts, asymptotes, and symmetry, which provide valuable information about the function’s behavior.

Example: Linear Function Graph

Consider a simple linear function:

x	y = 2x + 1
-2	-3
0	1
2	5

By plotting these points on a graph, you would observe a straight line, indicating a linear relationship between x and y.

Understanding functions and their graphs allows for a deeper comprehension of mathematical concepts, and it is an essential skill for higher-level mathematics and various real-world applications. By practicing graphing different types of functions, you can develop a solid foundation for analyzing more complex relationships.

Factoring Methods and Techniques Explained

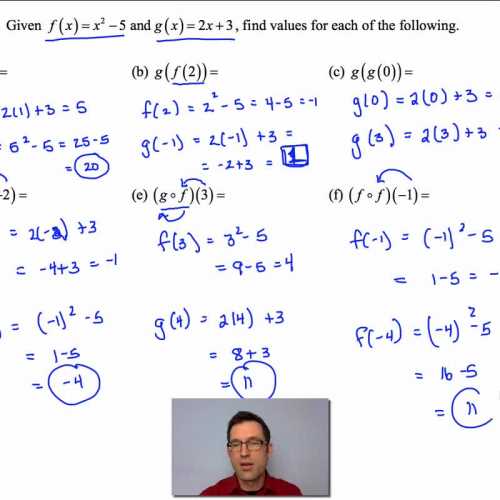
Factoring is a powerful technique used to simplify and solve polynomial expressions. It involves breaking down complex expressions into simpler components, making them easier to manipulate or solve. By factoring, you can often identify solutions to equations, simplify calculations, and reveal underlying relationships between terms.

Common Factoring Methods

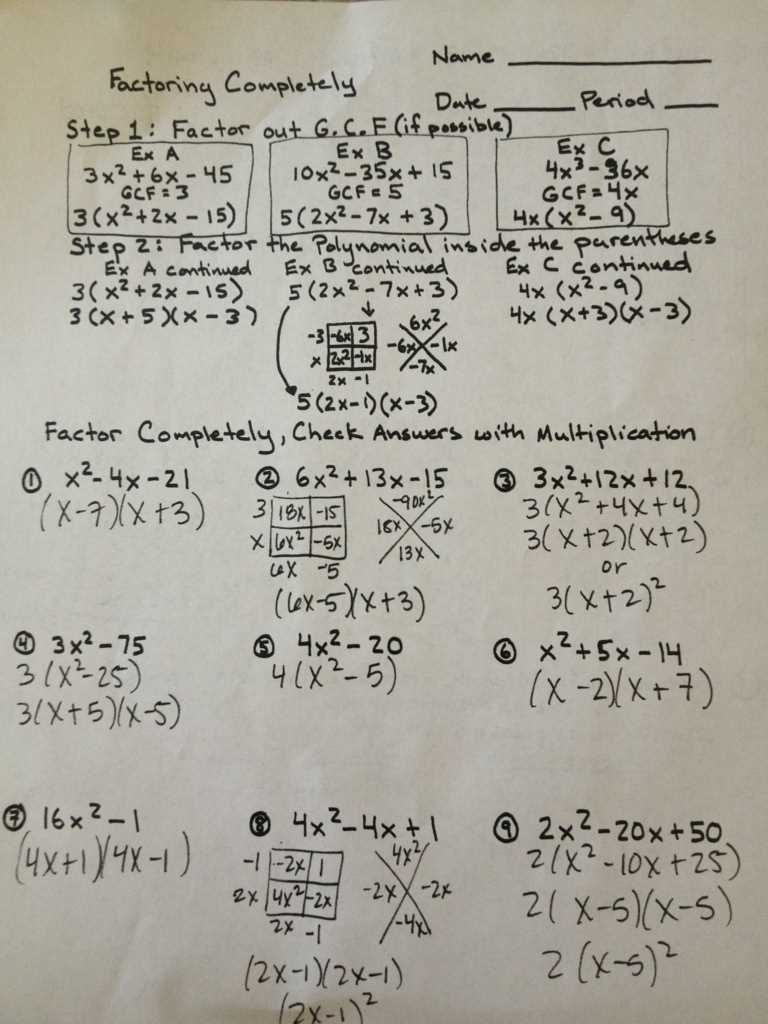
There are several methods used for factoring polynomials, each suited to different types of expressions. Understanding when and how to use each method is crucial for mastering this skill:

Greatest Common Factor (GCF): This method involves factoring out the largest factor common to all terms in the expression. It’s often the first step in simplifying any polynomial.
Factoring by Grouping: This technique is used when an expression has four terms. You group the terms in pairs, factor each group, and then factor out the common binomial factor.
Difference of Squares: This method applies when you have a binomial expression where both terms are perfect squares and separated by subtraction. It factors into the form (a – b)(a + b).
Trinomial Factoring: This method is used for quadratics or other trinomials. It involves finding two numbers that multiply to give the product of the first and last terms and add to give the middle term.

Step-by-Step Factoring Process

Regardless of the method, factoring follows a general approach. Here’s how to factor a polynomial using the appropriate technique:

Step 1: Look for the greatest common factor and factor it out if possible.
Step 2: If there are four terms, consider grouping them into pairs and factoring each pair separately.
Step 3: For trinomials, identify the two numbers that multiply to the product of the first and last terms and add up to the middle term.
Step 4: Apply the appropriate method based on the structure of the polynomial.
Step 5: Double-check the result by multiplying the factored expression back out to ensure it matches the original polynomial.

Mastering these factoring techniques is essential for solving a wide variety of mathematical problems. With practice, you’ll be able to factor polynomials quickly and efficiently, allowing you to solve equations, simplify expressions, and understand the structure of mathematical relationships more clearly.

Solving Inequalities with Algebraic Methods

Solving inequalities involves finding the set of values that satisfy a given condition, represented as a mathematical inequality. These problems are similar to equations but instead of finding a single value, you seek a range of possible solutions. By applying various algebraic techniques, inequalities can be simplified and solved to reveal the solution set, which can then be expressed in interval or set notation.

Basic Steps in Solving Inequalities

Solving inequalities follows a similar process to solving equations, but with important differences, especially when it comes to multiplying or dividing by negative numbers. The basic steps include:

Step 1: Simplify the inequality by combining like terms and moving constants to one side.
Step 2: Isolate the variable by performing the appropriate algebraic operations.
Step 3: Pay close attention when multiplying or dividing by negative numbers, as this will reverse the inequality sign.
Step 4: Express the solution in a suitable form, either as a number line, interval notation, or set notation.

Types of Inequalities and Methods

Different types of inequalities require specific methods for solving:

Linear Inequalities: These are solved similarly to linear equations, but the solution is typically expressed as a range of values.
Quadratic Inequalities: These can be solved by first finding the roots of the corresponding quadratic equation and then testing intervals to determine which ones satisfy the inequality.
Rational Inequalities: These inequalities involve rational expressions and are solved by finding the critical points and testing the sign of the expression in each interval.

Understanding these methods is key to mastering inequalities and solving real-world problems where boundaries or constraints are involved. With practice, algebraic techniques will help in tackling even the most complex inequality problems.

Utilizing Graphing Calculators for Algebra 2

Graphing calculators are powerful tools that help students visualize and analyze mathematical functions, making complex problems more accessible. By plotting graphs and providing numerical solutions, these calculators simplify tasks that would otherwise require tedious calculations. In courses that involve higher-level equations, such as quadratic or polynomial functions, graphing calculators are essential for efficiently solving and understanding the relationships between variables.

Benefits of Using a Graphing Calculator

Graphing calculators offer several advantages that enhance the learning experience:

Visualization: Students can quickly see the graph of an equation, making it easier to understand the behavior of the function, including intercepts, slopes, and asymptotes.
Accuracy: These calculators provide precise solutions for problems that involve complex calculations or multiple steps, reducing the risk of human error.
Efficiency: By automating tedious tasks, graphing calculators save time and allow students to focus on the conceptual aspects of problem-solving.
Interactive Learning: Many calculators allow users to zoom in and out of graphs or adjust parameters, which fosters a more interactive approach to exploring mathematical concepts.

How to Use a Graphing Calculator Effectively

To get the most out of a graphing calculator, it’s important to understand its key features and functions:

Function	Description
Graphing Functions	Plot equations and visualize their graphs to understand their behavior and key characteristics.
Solve Equations	Use the calculator’s built-in solvers to find the roots or solutions to equations.
Analyze Graphs	Find points of intersection, maxima, minima, and other key values from the graph.
Zoom Features	Adjust the viewing window to focus on specific areas of the graph for a detailed analysis.

By incorporating these tools into daily study routines, students can develop a deeper understanding of mathematical concepts and improve their problem-solving skills. Graphing calculators not only simplify calculations but also foster a more intuitive grasp of abstract mathematical ideas.

Key Algebraic Identities You Should Know

Mathematical identities play a critical role in simplifying expressions and solving problems efficiently. These fundamental formulas allow you to manipulate and transform equations, making them essential tools for higher-level problem-solving. Understanding and applying these identities can streamline calculations, eliminate complex steps, and help you identify patterns in mathematical problems.

Common Identities for Simplification

There are several key identities that are widely used to simplify and solve equations. These include:

Difference of Squares: This identity expresses the product of two binomials as the difference between their squares. It is written as (a + b)(a – b) = a² – b².
Perfect Square Trinomial: This identity represents the square of a binomial. It is written as (a + b)² = a² + 2ab + b² and (a – b)² = a² – 2ab + b².
Sum and Difference of Cubes: These identities help to factor cubic expressions. They are written as a³ + b³ = (a + b)(a² – ab + b²) and a³ – b³ = (a – b)(a² + ab + b²).

Advanced Identities for Complex Problems

As you advance in mathematical studies, more complex identities become useful for handling intricate expressions:

Binomial Theorem: This identity helps expand expressions of the form (a + b)ⁿ. It states that (a + b)ⁿ = aⁿ + n(aⁿ⁻¹)b + … + bⁿ, where “n” is a positive integer.
Quadratic Formula: Used to find the solutions of a quadratic equation ax² + bx + c = 0. The solutions are given by x = (-b ± √(b² – 4ac)) / 2a.
Sum of Geometric Series: For a geometric sequence, the sum of the first “n” terms is given by S = a(1 – rⁿ) / (1 – r), where “a” is the first term and “r” is the common ratio.

These identities form the backbone of many advanced mathematical techniques and are indispensable when solving complex problems. By mastering these formulas, you’ll be able to approach problems with greater confidence and efficiency.

Applying Algebra 2 Concepts to Real Life

Mathematical concepts learned in advanced courses are not just theoretical; they have practical applications in everyday life. By understanding how to manipulate equations, functions, and variables, individuals can make informed decisions in fields ranging from finance to engineering. These skills are invaluable for problem-solving in real-world scenarios, providing solutions to challenges faced in various professions and personal situations.

Practical Applications in Finance

One of the most prominent areas where advanced mathematical concepts are applied is in managing finances. For example, understanding how to solve equations and work with expressions allows individuals to calculate interest rates, loan payments, and investment growth over time. Key concepts, such as exponential growth and functions, are often used to determine how money will accumulate in savings accounts or how loans will be paid off over time. By applying these mathematical techniques, you can better plan your financial future and make more informed decisions about your personal budget.

Engineering and Technology

In engineering, mathematical principles are used to design structures, systems, and technologies that affect our daily lives. For instance, understanding how to solve systems of equations is essential when working with electrical circuits, optimizing supply chains, or calculating the necessary materials for construction projects. The ability to model real-world problems using mathematical formulas helps engineers ensure that their designs are safe, efficient, and effective.

Whether you’re making decisions about personal finances or working on a professional project, the mathematical concepts learned in advanced courses provide essential tools for success. By recognizing the relevance of these skills in various industries, you can apply your knowledge to solve everyday problems more effectively.

Common Mistakes to Avoid in Algebra 2

When studying advanced mathematical concepts, it’s easy to fall into certain traps that can lead to errors. Many students make similar mistakes while working through equations, simplifying expressions, or solving problems. Recognizing and avoiding these pitfalls is crucial for mastering the material and ensuring accuracy. Below are some of the most common mistakes encountered in higher-level math, along with tips for avoiding them.

Incorrectly Solving Equations

One of the most frequent errors in math is mishandling equations. Whether it’s linear, quadratic, or rational, equations require careful attention to detail. Common mistakes include:

Forgetting to apply the distributive property: Always ensure that you distribute terms properly across parentheses when simplifying or solving an equation.
Sign errors: Pay close attention to negative signs, especially when working with subtraction or division.
Dividing by zero: Never divide an expression by zero, as it leads to undefined results.

Misunderstanding Functions and Their Properties

Functions are fundamental in advanced mathematics, but their properties can sometimes be misunderstood. Some typical mistakes include:

Confusing function notation: Make sure to distinguish between the variable and the function itself, especially when solving for unknowns.
Overlooking domain and range: Always check the domain and range of a function to avoid invalid inputs or outputs.
Incorrectly interpreting transformations: When shifting, stretching, or reflecting graphs, double-check your transformations to ensure they align with the problem’s instructions.

By being aware of these common mistakes and taking the time to check your work, you can significantly improve your understanding and performance in mathematics. Practice and attention to detail will help you avoid these errors and approach problems with greater confidence.