
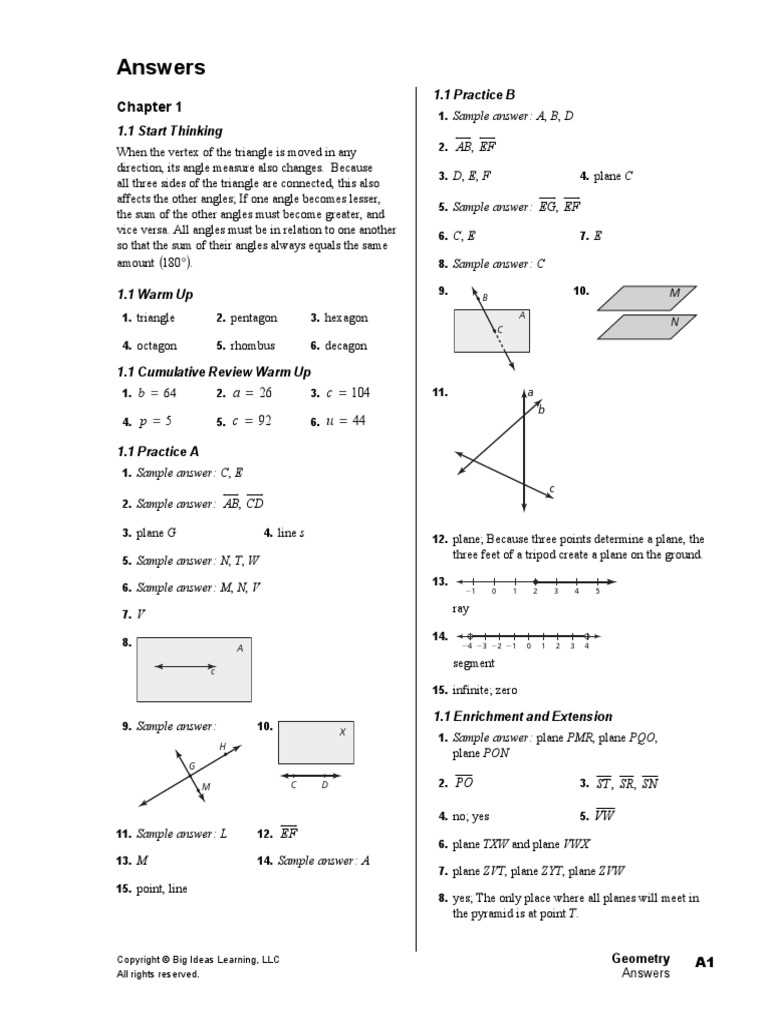
When it comes to mastering complex subjects, having the right resources at your disposal can make all the difference. Comprehensive solution guides provide a valuable tool for reinforcing understanding and correcting mistakes. These resources allow learners to see detailed solutions, helping them grasp difficult concepts and approaches with ease.
With a well-structured guide, students can not only verify their results but also understand the logic behind each step. This approach promotes deeper learning, offering insights into the problem-solving process and allowing for better retention of key techniques. Whether you’re reviewing material or practicing new exercises, these solutions serve as an essential support system in any educational journey.
By incorporating these helpful resources into your study routine, you can tackle challenges with confidence and continue progressing in your studies. They are designed to simplify learning, reduce frustration, and boost performance, all while ensuring a clearer understanding of the subject matter.
Big Ideas Math Green Answer Key Overview
This section provides a detailed examination of a comprehensive resource designed to enhance the learning experience for students. With step-by-step solutions and clear explanations, it serves as an essential tool for those seeking to improve their understanding of complex topics. By breaking down each problem, this guide helps learners see the logic behind each solution, making it easier to absorb and apply key concepts.
How the Resource Supports Learning
Rather than simply providing answers, this resource offers an in-depth breakdown of each step involved in solving a problem. This method ensures that learners don’t just memorize the solutions but truly understand the process. The guide emphasizes problem-solving strategies and provides opportunities for practice, allowing students to approach future tasks with confidence.
Who Can Benefit from This Resource
This resource is ideal for students at various levels who need extra support in understanding challenging concepts. Whether used as a review tool or as a primary source for learning, it caters to different learning styles by providing both visual and written explanations. In addition, teachers can use it to reinforce classroom lessons, ensuring that all students have the tools they need to succeed.
How to Use the Answer Key
Using a solution guide effectively can greatly improve your understanding of complex topics and help you correct mistakes. This section will explain how to make the most of the resource by following a structured approach to enhance learning. By actively engaging with the material, you can reinforce concepts and identify areas that need further attention.
Here are the steps to make the most of the guide:
- Review the Problem First: Always attempt to solve the problem on your own before referring to the guide. This will allow you to evaluate your understanding and identify gaps.
- Follow the Step-by-Step Solutions: When you consult the guide, follow each step carefully. This will help you understand the logic behind each solution and improve your problem-solving skills.
- Check for Mistakes: After comparing your solution with the guide’s steps, analyze any differences. Identify where you made errors and review those concepts.
- Apply What You Learned: Use the insights gained from the solution process to attempt similar problems. This will help reinforce the knowledge and improve your proficiency.
By following these steps, you can ensure that you use the resource in a way that promotes long-term learning and understanding. Rather than just checking your answers, the guide should be used as a tool to deepen your grasp of the material and build confidence in your abilities.
Understanding Key Concepts in Math
Grasping essential principles is vital to mastering any subject, particularly those involving complex calculations and problem-solving. A solid understanding of these core ideas allows students to tackle a wide range of problems confidently. This section will explore how focusing on the underlying concepts helps build a strong foundation for advanced learning and practical application.
Core Areas of Focus
When working through problems, it is crucial to focus on specific areas that frequently form the basis of more complicated topics. Below is a table that highlights some of the key concepts that should be prioritized in the learning process:
| Concept | Importance | Application |
|---|---|---|
| Algebraic Expressions | Helps in understanding relationships between variables | Used in solving equations and inequalities |
| Fractions and Ratios | Essential for comparing quantities and proportional reasoning | Common in real-world problems, such as cooking or budgeting |
| Geometry Basics | Foundation for understanding shapes, areas, and volumes | Applicable in design, architecture, and navigation |
Mastering Concepts Through Practice
To fully understand these concepts, continuous practice is key. By solving a variety of problems that apply these core principles, students can strengthen their understanding and build connections between ideas. Over time, this consistent effort leads to greater confidence and better results in tackling even the most challenging problems.
Step-by-Step Problem Solving Guide
Breaking down a problem into manageable steps is an effective way to find solutions efficiently. This method ensures that learners understand the process and develop the skills needed to tackle future challenges. By following a structured approach, you can solve problems logically and methodically, avoiding confusion and errors.
Below is a guide to help you solve problems step by step. This method can be applied to various types of exercises, from simple calculations to more complex challenges:
| Step | Action | Purpose |
|---|---|---|
| 1 | Understand the Problem | Read the problem carefully to determine what is being asked. Identify the knowns and unknowns. |
| 2 | Plan Your Approach | Decide which methods or formulas will help solve the problem based on the information provided. |
| 3 | Carry Out the Plan | Execute the necessary steps, ensuring each calculation or operation is performed correctly. |
| 4 | Review Your Work | Double-check the calculations and ensure the solution makes sense within the context of the problem. |
By following this guide, you can systematically approach any problem and increase your chances of reaching an accurate and confident solution. This method not only enhances problem-solving skills but also builds a deeper understanding of the principles involved.
Benefits of Using the Answer Key
Utilizing a comprehensive solution guide offers several advantages to students seeking to strengthen their understanding of complex concepts. Rather than merely providing solutions, this resource serves as an effective tool for reinforcing learning, correcting mistakes, and improving problem-solving abilities. It encourages students to engage with the material at a deeper level, promoting greater retention and mastery of the subject matter.
Enhanced Understanding of Concepts
One of the primary benefits of using a solution guide is the opportunity to gain a clearer understanding of the steps involved in problem-solving. By examining each step, learners can see the logic behind the solutions and better grasp the underlying principles. This process helps solidify their knowledge and enhances their ability to approach similar problems in the future.
Improved Accuracy and Confidence
Another significant advantage is the ability to identify and correct mistakes. By comparing your own work to the detailed solutions provided, you can pinpoint where errors may have occurred and understand how to fix them. This not only boosts accuracy but also increases confidence, allowing students to tackle more complex challenges with greater assurance.
Incorporating a solution guide into your study routine provides a structured way to track progress, identify areas of improvement, and reinforce key concepts, making it an invaluable resource for mastering the material.
Common Mistakes to Avoid
When working through complex problems, it’s easy to make errors that can hinder progress and lead to confusion. Understanding and recognizing common mistakes helps prevent these pitfalls, ensuring a more effective learning process. Being aware of typical challenges allows students to focus on improving their approach and mastering the material.
Skipping Steps in the Process
One of the most frequent errors is skipping crucial steps in problem-solving. Rushing through a solution can lead to missing important details or making incorrect assumptions. It’s essential to follow each step carefully, ensuring that no part of the problem is overlooked. Taking the time to work through the problem methodically enhances accuracy and prevents unnecessary mistakes.
Misinterpreting the Problem
Another common mistake is misunderstanding the problem itself. This can happen if key information is missed or misread. Always read the problem thoroughly and underline or highlight the important details to ensure that the correct approach is applied. Clear comprehension of the problem’s requirements is vital for selecting the appropriate method and reaching the right solution.
Avoiding these common mistakes is crucial for developing strong problem-solving skills. By paying attention to detail, following the solution process step by step, and fully understanding the problem at hand, students can improve their accuracy and performance over time.
How the Answer Key Enhances Learning
A solution guide serves as an invaluable resource for students seeking to deepen their understanding of difficult topics. It goes beyond providing final answers, offering insights into the process behind each solution. By analyzing how problems are solved, students can improve their reasoning, discover alternative methods, and apply their knowledge more effectively in future tasks.
Key Benefits of Using the Guide
- Clarifies Problem-Solving Techniques: The guide offers step-by-step explanations, helping students learn the strategies behind solving complex problems.
- Improves Comprehension: By studying each stage of the solution process, learners can connect theory with practical application, reinforcing their understanding.
- Encourages Self-Assessment: Comparing personal attempts with the guide allows students to evaluate their strengths and weaknesses, facilitating focused improvement.
- Boosts Confidence: Regularly reviewing correct solutions builds confidence, empowering students to tackle more challenging problems independently.
How It Supports Long-Term Retention
Learning from mistakes is a powerful tool for retention, and a solution guide helps students identify errors and understand where they went wrong. Repeated exposure to correct methods ensures that students not only memorize solutions but truly grasp the underlying principles, leading to greater long-term mastery of the material.
Accessing the Math Resources
Having easy access to relevant learning materials is essential for students looking to strengthen their skills. Comprehensive resources provide a structured path for exploring complex topics, offering everything from practice exercises to step-by-step solutions. These tools are designed to support both independent study and classroom learning, making it easier for students to find the help they need at every stage of their educational journey.
Where to Find the Learning Materials
Accessing the necessary resources is straightforward. These materials are often available online through official platforms, textbooks, or companion websites. Some common methods for obtaining these resources include:
- Official Website: Many educational platforms host digital copies of textbooks, exercises, and guides that are easily downloadable or accessible via subscription.
- Teacher and School Access: Educators and institutions may provide students with login credentials or printouts that grant access to specific learning tools.
- Online Educational Platforms: Websites dedicated to academic support often offer supplementary materials, including video tutorials and interactive problem sets.
Maximizing the Use of Available Resources
To make the most out of these resources, students should focus on consistency. Regularly engaging with the provided materials enhances retention and helps in mastering the concepts being taught. Additionally, students can combine these resources with real-world applications or peer discussions to deepen their understanding and keep the learning process dynamic and effective.
Improving Skills with Solution Guides
Using a comprehensive solution guide plays a significant role in strengthening problem-solving abilities. It not only provides final answers but also outlines the reasoning behind each step, offering insights into how to approach various challenges. By regularly consulting such guides, students can identify gaps in their understanding and improve their technique, making it easier to solve more complex problems over time.
Steps to Enhance Skills

- Study the Process: Don’t just look at the final solution–focus on understanding the steps leading up to it. This reinforces problem-solving techniques and helps build a stronger foundation.
- Compare Solutions: After attempting problems independently, compare your solutions with those provided in the guide. This allows you to identify errors, learn from them, and improve your approach.
- Practice Regularly: Consistent practice is essential for honing skills. The more problems you solve, the better equipped you will be to tackle new challenges confidently.
- Understand Mistakes: When mistakes occur, use the solution guide to figure out where the error happened. Understanding your mistakes helps avoid them in the future.
Long-Term Benefits
Utilizing a solution guide not only improves immediate problem-solving skills but also fosters a deeper understanding of underlying concepts. This holistic approach to learning ensures long-term retention, helping students apply their knowledge effectively in future studies or real-life scenarios.
How to Check Your Solutions
Verifying your solutions is a crucial step in the problem-solving process. By carefully reviewing your work, you ensure accuracy and identify any mistakes you may have overlooked during the initial attempt. This practice not only boosts confidence in your results but also helps you refine your approach for future problems.
Steps to Verify Your Solutions

Follow these steps to effectively check your work:
- Review Each Step: Go through your calculations and logic step by step. Ensure that each action follows logically from the previous one and that no steps have been skipped.
- Check for Consistency: Make sure that your results are consistent with the problem’s requirements and that your interpretation of the question is correct.
- Use a Different Method: If possible, try solving the problem using an alternative approach or method. If both methods yield the same result, this is a strong indicator that the solution is correct.
- Compare with a Solution Guide: Compare your final result with a trusted solution guide to verify if your answer matches the expected outcome.
Additional Tips for Effective Checking
| Tip | Description |
|---|---|
| Work Backwards | If applicable, try starting from the final answer and working your way back to the original problem to check for errors. |
| Double-Check Units | Ensure that all units are consistent and correctly used throughout the problem, especially in complex calculations involving measurements. |
| Take Breaks | Returning to the problem after a short break can help you see errors you might have missed when you were focused for too long. |
By following these steps and tips, you can be confident that your solutions are correct and thoroughly verified, leading to a deeper understanding and better results in your studies.
Why Accuracy is Crucial in Mathematics
Precision plays a pivotal role in solving problems correctly and efficiently. In subjects that involve numerical calculations or logical reasoning, even a small mistake can lead to incorrect results, which may carry over to subsequent steps. Ensuring that every action is accurate not only helps in obtaining the right solution but also strengthens understanding and reinforces reliable problem-solving techniques.
Impact of Mistakes on Results
When errors occur, they can distort the final outcome and lead to confusion. The consequences of inaccurate calculations can range from simple misunderstandings to more significant setbacks, especially when dealing with complex problems. Some possible effects of mistakes include:
- Misinterpretation of the Problem: An error in the initial steps can skew the entire approach, resulting in a solution that does not align with the question.
- Compounded Errors: Mistakes in earlier calculations can lead to a cascade of incorrect steps, making it increasingly difficult to identify the original problem.
- Wasted Time: Time spent on incorrect solutions can delay progress and lead to frustration, especially when the error goes unnoticed.
Building Strong Skills Through Precision
By focusing on accuracy, learners develop a deeper understanding of the concepts at hand. This careful attention helps students identify patterns, recognize potential pitfalls, and build confidence in their abilities. In addition, maintaining precision in problem-solving helps prevent misunderstandings and encourages more effective learning overall.
How to Learn from Your Mistakes
Making errors is an inevitable part of the learning process, but the key to growth lies in how we respond to them. Instead of being discouraged, it is essential to treat mistakes as valuable learning opportunities. By analyzing where things went wrong and understanding why an error occurred, we can improve our skills and enhance our problem-solving abilities over time.
One effective approach is to review each mistake thoroughly. Ask yourself questions like, “What did I overlook?” or “Which step caused confusion?” This reflection can help pinpoint the source of the problem and guide you toward the correct method. Additionally, taking time to reattempt the problem, applying what you’ve learned, will reinforce new concepts and build confidence.
Another key aspect is seeking feedback from trusted resources, whether from teachers, peers, or solution guides. Learning from others’ insights can provide different perspectives on how to approach the same challenge, leading to deeper understanding and greater mastery.
Mastering Concepts Using the Solution Guide

Achieving a strong understanding of concepts requires more than just solving problems; it involves a deeper engagement with the material. One powerful tool in the learning process is a solution guide, which provides clear explanations and step-by-step solutions to complex problems. By using this resource strategically, learners can develop a solid grasp of important principles and reinforce their knowledge.
Steps to Effectively Use the Solution Guide
To make the most of a solution guide, follow these practical steps:
- Understand the Process: Don’t just look at the final answer. Focus on understanding the method used to arrive at the solution.
- Identify Key Steps: Break down the solution into individual steps. This helps in recognizing patterns and applying them to other problems.
- Compare Your Approach: Compare your solution with the one provided in the guide. Identify any discrepancies and analyze why your method might have been different.
- Practice Without Assistance: After reviewing the solution, attempt similar problems on your own to reinforce your learning and ensure retention.
Benefits of Using a Solution Guide
Utilizing a solution guide offers several advantages for learners, including:
- Clarifying Doubts: It provides answers to any questions or confusion you might have during problem-solving.
- Building Confidence: Seeing how solutions are structured boosts confidence and enables better performance in similar challenges.
- Reinforcing Concepts: By following detailed steps, you internalize fundamental concepts that are vital for future success.
Tips for Effective Problem Solving
Solving complex problems requires a thoughtful and methodical approach. While it may seem challenging at first, breaking down the task into manageable steps can make a significant difference. By using effective strategies, you can improve your problem-solving skills and gain a deeper understanding of the underlying concepts.
Key Strategies for Success
Here are some proven techniques to help you approach problems more effectively:
- Analyze the Problem: Before jumping into a solution, take a moment to fully understand the question. Identify the key information and what is being asked.
- Break It Down: Divide the problem into smaller, simpler parts. This makes the overall task more approachable and easier to tackle step by step.
- Look for Patterns: Often, problems are based on familiar patterns or rules. Recognizing these patterns can help you find a more efficient solution.
- Use Logical Reasoning: Approach the problem with a clear, logical thought process. Avoid guessing or making assumptions without a solid foundation.
- Check Your Work: After solving the problem, revisit each step to ensure accuracy. Double-check your calculations and reasoning to catch any mistakes.
Improving Through Practice
The more you practice solving problems, the more proficient you become. Consistent practice allows you to refine your approach and develop a deeper understanding of the material. Here are a few tips for enhancing your problem-solving skills:
- Practice Regularly: Set aside time to solve problems daily. The more you practice, the better you’ll get at recognizing patterns and applying strategies effectively.
- Learn from Mistakes: Mistakes are an essential part of learning. Analyze where you went wrong and use that experience to improve in the future.
- Ask for Help When Needed: Don’t hesitate to seek guidance from others if you’re stuck. A fresh perspective can often lead to breakthroughs in understanding.
Strategies for Tackling Complex Problems
When faced with challenging tasks, having a solid approach can make a world of difference. Rather than feeling overwhelmed, focusing on systematic techniques can help break down a seemingly difficult problem into smaller, more manageable parts. By mastering a few key strategies, you can approach even the most complex problems with confidence and clarity.
The following strategies are effective for navigating intricate challenges, whether they involve detailed calculations, conceptual understanding, or logical reasoning:
- Identify the Core Issue: Before diving into the solution, take a step back and determine the exact nature of the problem. Isolate the key factors that must be addressed.
- Work Backwards: Start from the end goal and reverse-engineer the process. This can often highlight the necessary steps and clarify the path to the solution.
- Divide and Conquer: Break the problem down into smaller, more digestible segments. Solve each segment independently before putting them all together to form the complete answer.
- Use Visual Aids: Sometimes a diagram, chart, or graph can help visualize the problem. This can make abstract concepts easier to understand and provide insight into possible solutions.
- Take a Step-by-Step Approach: Don’t rush. Tackle each part of the problem one step at a time, ensuring you fully understand each phase before moving on to the next.
With these strategies in mind, complex problems become less intimidating. Practice and patience are key as you refine your problem-solving skills, ultimately building greater confidence in your ability to handle intricate challenges.
Using the Answer Key for Review
Reviewing solutions is an essential part of reinforcing understanding and mastering any topic. After working through a set of problems, revisiting the correct solutions helps identify any gaps in knowledge and areas for improvement. Using a solution guide effectively can lead to deeper insights and more efficient learning.
Here are some strategies for leveraging the solution guide to enhance your review process:
- Compare Your Solutions: Carefully compare your answers with the provided solutions. Look for any discrepancies and identify the steps where you made mistakes or took shortcuts.
- Understand the Process: Focus not just on the final result, but on the methods used to arrive at the answer. Understanding the reasoning behind each step is crucial for mastering the material.
- Identify Patterns: As you review multiple solutions, look for recurring strategies or methods that appear in different problems. Recognizing these patterns can make solving similar problems faster and easier in the future.
- Clarify Concepts: If there are concepts that remain unclear after reviewing the solutions, take time to revisit your notes, textbooks, or online resources to clarify them. Sometimes a brief review can solidify your understanding.
Common Pitfalls to Avoid
- Relying Too Heavily on the Guide: While the guide is a helpful tool, avoid using it too early or too frequently. Try solving problems independently before checking the solution.
- Skipping Steps: Ensure that you don’t just glance at the final answer. Reviewing each step carefully ensures you fully understand the process involved.
Incorporating these strategies into your study routine will not only improve your problem-solving skills but also strengthen your grasp on key concepts. Consistent and thoughtful use of the solution guide turns it into a powerful tool for continuous learning.
Aligning Lessons with Solution Guide Insights
Effective teaching requires integrating learning materials with clear, structured solutions to enhance understanding and application. By aligning lessons with detailed solution guides, educators can create a direct link between theory and practical problem-solving. This alignment allows students to better grasp concepts and see the step-by-step process used to reach conclusions, reinforcing their ability to apply knowledge independently.
When planning lessons, it’s important to ensure that the instructional content mirrors the strategies and steps found in the solution guide. This allows students to see how each lesson builds towards solving real-world problems, while also providing a clear reference point for review and practice.
Example of Aligning a Lesson with Solution Steps
Consider a problem-solving exercise where students are asked to solve for unknown variables. Here’s how to align the lesson content with the solution guide:
| Step | Lesson Focus | Solution Guide Insight |
|---|---|---|
| Step 1 | Introduce the concept of isolating the variable. | Solution guide demonstrates isolating the variable using basic algebraic operations. |
| Step 2 | Show how to simplify the equation. | The guide details how to combine like terms and reduce the equation to a simpler form. |
| Step 3 | Provide techniques for solving for the variable. | Solution guide walks through solving the simplified equation and finding the value of the variable. |
By reviewing the lesson alongside the solution steps, educators can ensure a coherent teaching approach that strengthens students’ problem-solving skills and prepares them for future challenges.
Improving Confidence in Math Studies
Building confidence in solving mathematical problems is crucial for long-term success in any area of study. Students often struggle with complex concepts and feel discouraged when they cannot immediately grasp new material. However, consistent practice, guidance, and a solid understanding of foundational principles can significantly improve both comprehension and self-assurance in this subject. Through structured problem-solving methods and clear explanations, learners can break down difficult challenges and build a more confident approach to their studies.
Practical Strategies for Building Confidence
- Start with the Basics: Reinforce fundamental skills and concepts to ensure a solid foundation. Mastering the basics leads to greater ease in understanding more advanced topics.
- Consistent Practice: Regularly solving problems, even simple ones, can boost confidence by helping students recognize patterns and improve their problem-solving speed.
- Positive Reinforcement: Celebrating small successes can have a huge impact on motivation. Recognizing progress, even with minor victories, fosters a growth mindset.
- Collaborate with Peers: Group study sessions and collaborative problem-solving can provide different perspectives and encourage mutual support.
Tracking Progress for Continued Improvement
Keeping track of personal progress is an effective way to build confidence. Regular self-assessment allows students to identify areas of strength and areas needing improvement. By understanding where they excel, they can focus efforts on areas that require further attention. Over time, this cycle of self-evaluation and growth helps build a more positive relationship with the subject.