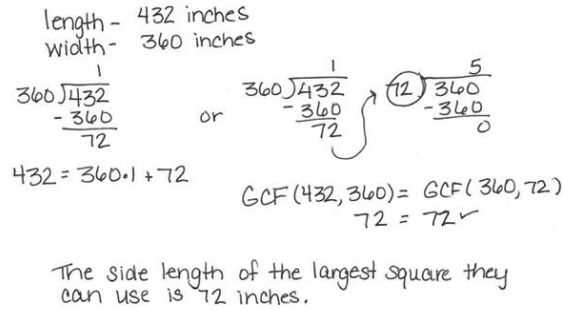
In this section, we explore essential topics and provide clear explanations to help you grasp important mathematical concepts. Our goal is to guide you through complex problems with ease, offering practical strategies to improve your understanding. Whether you’re preparing for exams or working through assignments, this guide is designed to support your learning journey.
From foundational principles to advanced techniques, we break down each problem type and show you how to solve them step by step. You’ll find comprehensive solutions that explain not just the answer, but the reasoning behind each step. This approach ensures that you build a solid foundation and gain the confidence needed to tackle similar challenges independently.
By focusing on key strategies, tips, and common pitfalls, you’ll be better prepared to approach any topic with a deeper understanding. The solutions provided will serve as a valuable resource for reinforcing your knowledge and mastering the material.
Secondary Math 2 Module 6 Answers
This section provides comprehensive guidance for solving various mathematical problems typically encountered in the second-level curriculum. It offers a step-by-step breakdown of common challenges and their solutions, helping students gain confidence and clarity in their problem-solving abilities. You will find explanations for each step, ensuring that the reasoning behind each answer is clearly understood.
Key Concepts Covered
- Understanding linear equations and their solutions
- Graphing points and interpreting data
- Solving inequalities and interpreting results
- Working with systems of equations
- Applying mathematical principles to real-world problems
How to Approach Problem Solving
- Start by carefully reading the problem to identify key information.
- Choose the appropriate method to solve, whether it’s substitution, elimination, or graphing.
- Apply the method step by step, checking each calculation along the way.
- Revisit the question and ensure your solution makes sense in the given context.
By following these guidelines, students can enhance their problem-solving skills and become more proficient in tackling complex mathematical challenges.
Overview of Module 6 Concepts
This section provides a broad understanding of the key concepts covered in the current level of study. It focuses on essential mathematical principles and techniques that help in solving various types of problems. Students will explore topics ranging from solving equations to interpreting graphical data, each crucial for mastering more advanced topics.
Core Topics to Explore
- Solving linear equations and inequalities
- Graphing and analyzing functions
- Working with systems of equations
- Exploring polynomial expressions
- Understanding the principles of proportional reasoning
Skills to Develop
- Mastering equation manipulation for solving problems
- Developing graphing techniques for visualizing data
- Applying mathematical models to real-world scenarios
- Interpreting and analyzing results accurately
By the end of this section, students should feel confident in applying these concepts to a wide range of problems, enhancing both their theoretical understanding and practical problem-solving abilities.
Key Formulas for Module 6
In this section, we will highlight the essential formulas that are crucial for solving a wide variety of problems. These mathematical expressions serve as the foundation for much of the work in this area, allowing students to approach problems with a systematic and efficient method. Knowing and understanding these formulas is key to mastering the material and achieving accuracy in solutions.
Important Equations to Remember
- Linear Equations: y = mx + b (Slope-intercept form)
- Quadratic Equations: ax² + bx + c = 0 (Standard form)
- Exponential Growth/Decay: y = a(1 ± r)ᵗ
- Systems of Equations: ax + by = c and dx + ey = f
Useful Mathematical Relationships
- Distance Formula: d = √((x₂ – x₁)² + (y₂ – y₁)²)
- Pythagorean Theorem: a² + b² = c²
- Slope Formula: m = (y₂ – y₁) / (x₂ – x₁)
Mastering these formulas will allow students to approach problems with greater confidence and accuracy. Each formula plays a significant role in simplifying complex tasks and ensuring correct solutions across a range of mathematical challenges.
Step-by-Step Solution Process
Breaking down complex problems into smaller, manageable steps is essential for understanding and solving them effectively. This approach allows students to focus on one part of the problem at a time, ensuring clarity and reducing the chance of mistakes. By following a systematic method, each stage of the problem is addressed logically, leading to the correct solution.
First, it is important to thoroughly read and understand the problem. Identify key variables, constants, and the desired outcome. Once you have a clear picture of the problem, decide on the most appropriate method or formula to apply. Next, solve the equation or work through the steps, checking calculations along the way. Finally, review the result to ensure it aligns with the original question and makes sense within the given context.
By following these steps, students can develop a structured approach to solving problems that is both efficient and accurate. This process builds confidence and ensures consistent success in tackling even the most challenging tasks.
Understanding the Problem Types
To solve any problem effectively, it’s crucial to first recognize the type of problem you’re dealing with. Each type has its own unique characteristics, requiring specific strategies and methods. By identifying the problem type early, you can choose the right approach and avoid unnecessary complications.
Common Problem Types
- Linear Equations: These involve finding the value of a variable that satisfies an equation with a straight-line graph.
- Quadratic Equations: Problems that involve equations of the form ax² + bx + c = 0, often requiring factoring or the quadratic formula.
- Word Problems: Real-life scenarios that require translating words into mathematical expressions and solving accordingly.
- Systems of Equations: Problems where multiple equations must be solved simultaneously to find the values of the variables.
- Inequalities: Involves finding the range of values for a variable that satisfies a given inequality.
Key Strategies for Each Problem Type
- For linear equations: Use substitution or graphing techniques to find the solution.
- For quadratic equations: Apply factoring, completing the square, or use the quadratic formula to find roots.
- For word problems: Translate the description into mathematical equations and solve as usual.
- For systems of equations: Use substitution, elimination, or matrix methods to find the solution.
- For inequalities: Solve algebraically, remembering to reverse the inequality sign when multiplying or dividing by negative numbers.
By recognizing the problem type and applying the appropriate solution method, students can tackle each challenge with greater efficiency and confidence.
Common Mistakes in Module 6
When working through complex mathematical problems, students often make certain errors that can lead to incorrect results. These mistakes typically arise from misunderstandings of the concepts or from rushing through steps without double-checking the work. Recognizing and addressing these common pitfalls is key to improving accuracy and building confidence in solving problems.
One frequent error occurs when students misinterpret the problem, leading to the wrong approach or formula. Another mistake is neglecting to simplify equations properly, which can result in more complicated work down the line. Students may also overlook small details, such as sign changes or incorrect order of operations, which can completely alter the outcome of a problem.
Being mindful of these mistakes and taking the time to carefully review each step can prevent many issues. Developing a habit of verifying calculations and double-checking the reasoning behind each decision will help ensure more reliable results and a deeper understanding of the material.
Solving Linear Equations in Module 6
Linear equations are a fundamental part of solving algebraic problems. They involve finding the value of a variable that satisfies a given equation. The solution process generally focuses on isolating the variable on one side of the equation, allowing you to determine its value. Understanding how to approach these equations step-by-step is essential for success in this area.
The basic steps for solving a linear equation include simplifying both sides, using inverse operations to move terms, and performing operations to isolate the variable. It’s important to maintain balance throughout the process, ensuring that every operation done on one side is also done on the other. Once the variable is isolated, you can solve for its value.
Example: Consider the equation 2x + 5 = 15. To solve for x, subtract 5 from both sides to get 2x = 10, then divide both sides by 2 to find x = 5. These basic principles apply to all linear equations, whether they are simple or more complex.
By following these steps carefully and practicing with various types of problems, students can become more proficient in solving linear equations and apply these skills to other areas of algebraic problem-solving.
Graphing Solutions and Coordinates
Graphing is an essential technique for visualizing solutions to equations and understanding the relationship between variables. By plotting solutions on a coordinate plane, you can observe how the values of variables change relative to one another. This method allows for a clearer, more intuitive understanding of algebraic concepts and is a key skill in solving equations involving two variables.
Each solution of an equation can be represented as a point on the graph, with the x-coordinate corresponding to one variable and the y-coordinate representing the other. For example, the solution to the equation y = 2x + 3 can be plotted by substituting different values for x and calculating the corresponding y values.
Example: For the equation y = 2x + 3, if x = 1, then y = 5. The point (1, 5) can be plotted on the coordinate plane. By repeating this process for several values of x, a straight line can be drawn, representing all possible solutions to the equation.
Graphing not only helps with visualizing individual solutions but also provides insight into how different equations behave and intersect. This skill is vital for solving systems of equations and understanding the geometry behind algebraic relationships.
Applications of Module 6 in Real Life
The concepts learned in this section have many practical applications that extend far beyond the classroom. From budgeting and shopping to engineering and technology, understanding the relationships between variables helps to make informed decisions in everyday life. These skills are essential for solving real-world problems and can be applied in a variety of fields and situations.
Financial Planning and Budgeting
One of the most direct applications of algebraic principles is in managing personal finances. Whether you are calculating expenses, planning savings, or determining loan payments, these mathematical techniques provide the foundation for making informed financial choices. For instance, solving equations can help determine how much you need to save each month to reach a specific goal, or how changes in interest rates will affect your total loan repayment.
Engineering and Technology
In fields such as engineering and computer science, solving equations and graphing solutions are crucial for designing structures, creating algorithms, and optimizing systems. Engineers use mathematical models to predict behavior, calculate loads, and ensure safety in construction projects. Similarly, in technology, algorithms that process data rely heavily on these skills to achieve efficient results.
By applying the techniques from this section, individuals can better understand and navigate challenges in a wide range of contexts, making these skills invaluable in both professional and personal life.
Important Theorems to Remember
In solving algebraic problems, certain theorems are crucial to understanding and applying key concepts effectively. These fundamental principles form the backbone of many mathematical operations and provide a foundation for solving complex equations. By familiarizing yourself with these theorems, you can simplify and expedite problem-solving processes.
One of the most important theorems is the Distributive Property, which states that for any numbers a, b, and c, the expression a(b + c) = ab + ac holds true. This property is essential for expanding expressions and simplifying equations. Another key theorem is the Zero Product Property, which asserts that if the product of two factors equals zero, at least one of the factors must be zero. This is particularly useful when solving quadratic equations and other polynomial problems.
Additionally, the Commutative and Associative Properties are crucial for manipulating algebraic expressions. The Commutative Property states that a + b = b + a and ab = ba, while the Associative Property indicates that (a + b) + c = a + (b + c) and (ab) * c = a * (bc). These theorems allow for flexibility in how equations are approached and solved.
By mastering these key theorems, you can confidently tackle a wide variety of algebraic problems, ensuring a more efficient and accurate problem-solving experience.
Tips for Mastering Module 6 Topics
Successfully mastering the concepts of this section requires a combination of consistent practice, understanding core principles, and applying strategies that reinforce your learning. These approaches help to simplify complex topics and build a solid foundation for solving various problems. Whether you’re working through equations, graphing, or exploring theorems, these tips can make a significant difference in your understanding and performance.
Practice Regularly and Step-by-Step
One of the most effective ways to master these topics is through consistent practice. Break down each problem into manageable steps and focus on understanding the process behind each calculation. It’s important not just to memorize formulas but to comprehend how and why they work. This deeper understanding will help you apply the concepts more effectively in different scenarios.
Review Key Concepts and Theorems
Familiarizing yourself with essential theorems and key properties is vital. For example, understanding the distributive property or the zero-product property can simplify many problems. Review these principles regularly, and ensure that you know when and how to use them. Creating flashcards or summary notes for quick reference can be particularly helpful in reinforcing these concepts.
By dedicating time to practice and reinforcing key ideas, you’ll develop a stronger grasp of the material. Don’t rush through the topics; take the time to understand each step and approach problems from different angles to build confidence and mastery.
Example Problems with Detailed Solutions
Working through example problems is one of the best ways to grasp complex concepts and improve problem-solving skills. By following step-by-step solutions, you can see how to apply the theoretical knowledge to actual problems. This approach not only reinforces understanding but also builds confidence in tackling similar challenges independently.
Example 1: Solving a Linear Equation
Let’s consider the equation 3x – 7 = 11. To solve for x, we begin by isolating the variable on one side:
- First, add 7 to both sides: 3x = 18.
- Next, divide both sides by 3: x = 6.
The solution is x = 6. By following these simple steps, we can solve linear equations efficiently.
Example 2: Graphing a Linear Equation
Consider the equation y = 2x + 4. To graph this equation, follow these steps:
- Start by plotting the y-intercept, which is 4 (the point (0, 4)).
- Then, use the slope, which is 2. For every 1 unit you move to the right on the x-axis, move 2 units up on the y-axis. Plot the point (1, 6) and continue this pattern.
- Finally, connect the points to form a straight line.
This process helps visualize the relationship between the variables and aids in solving real-world problems that involve linear relationships.
How to Check Your Answers
After solving a problem, it’s essential to verify your results to ensure accuracy. This process not only helps you catch mistakes but also solidifies your understanding of the concepts. There are several effective strategies to check your work, and applying these can lead to better outcomes in problem-solving tasks.
1. Substitute the Solution Back into the Original Problem
One of the easiest ways to check your answer is to substitute it back into the original equation or expression. If both sides of the equation are equal, then your solution is correct. For example, if you solved for x in the equation 3x – 5 = 10 and found x = 5, substitute it back:
- 3(5) – 5 = 10
- 15 – 5 = 10
- 10 = 10
Since both sides are equal, the solution is correct.
2. Use a Different Method
Sometimes, it’s helpful to solve the same problem using a different approach to ensure consistency in your results. For instance, if you used substitution to solve an equation, try graphing the equation to see if the solution matches. Alternatively, check your work by using a calculator or another tool to confirm your answer.
3. Check the Reasoning Behind Each Step
Review the steps you took to solve the problem. Did you follow the correct procedure? Were there any shortcuts or assumptions made that could lead to errors? By retracing your steps and thinking critically, you can often spot mistakes that might not be immediately obvious.
Study Strategies for Module 6 Success
To master the concepts and perform well, it’s essential to approach your studies with a focused and organized strategy. Understanding the key ideas, practicing consistently, and reviewing material regularly can significantly boost your performance. Using effective study techniques can make a substantial difference in your learning experience and retention of the material.
1. Break Down Complex Concepts
When faced with challenging topics, it’s important to break them down into smaller, more manageable parts. Take the time to understand each step or principle before moving on to the next. By doing so, you ensure that you build a strong foundation and avoid confusion later on. Don’t rush through difficult sections – take your time to grasp the material fully.
2. Practice Regularly with Sample Problems
Consistent practice is key to mastering any subject. Working through example problems and exercises allows you to apply what you’ve learned and identify any gaps in your understanding. Start with simpler problems to reinforce basic skills, then gradually tackle more complex ones. The more you practice, the more confident you’ll become.
3. Collaborate with Peers
Studying with classmates or friends can be incredibly beneficial. Group study sessions provide opportunities to discuss difficult concepts, ask questions, and share different perspectives. Explaining a concept to someone else can also help solidify your own understanding.
4. Create a Study Schedule
Develop a study plan that breaks down your tasks into manageable time blocks. By setting aside specific times each day to focus on different topics, you can ensure steady progress and prevent last-minute cramming. Stick to your schedule and prioritize the areas where you need the most improvement.
5. Review and Reinforce Key Concepts
Regular review is crucial for retaining what you’ve learned. Even if you understand a concept initially, revisiting it after a few days or weeks helps reinforce the material in your long-term memory. Consider summarizing key points in your own words, creating flashcards, or teaching the material to someone else to further cement your knowledge.
Challenges Students Face in Module 6
Students often encounter various difficulties while studying complex concepts, and this particular set of topics is no exception. Whether it’s understanding intricate formulas, solving equations, or interpreting graphs, many learners struggle to fully grasp the material. Identifying these challenges early and seeking strategies to overcome them is crucial for success.
1. Difficulty in Solving Complex Equations
One common challenge is mastering the techniques needed to solve more advanced equations. These problems often involve multiple steps and require a clear understanding of algebraic principles. Mistakes in early steps can lead to incorrect solutions, making it difficult to progress further.
2. Misunderstanding Graphs and Coordinates
Another frequent obstacle is interpreting and plotting graphs. Understanding the relationship between coordinates and how they are used to represent equations can be tricky for many students. Inaccurate graphing or confusion about the axes can lead to incorrect analysis and conclusions.
| Challenge | Impact on Learning | Possible Solution |
|---|---|---|
| Difficulty with equations | Leads to incorrect solutions and confusion | Practice breaking down each step, review basic concepts |
| Misunderstanding graphs | Incorrect graph interpretation affects problem-solving | Regularly practice graphing, seek visual aids and explanations |
| Poor time management | Increases stress and leads to rushed solutions | Develop a structured study plan and allow ample time for practice |
By recognizing these challenges and addressing them with the right strategies, students can improve their understanding and overcome obstacles. Focusing on consistent practice and seeking help when needed are essential steps to success.
Practical Exercises for Practice
To master any topic, consistent practice is essential. Engaging in various exercises allows students to reinforce their knowledge and build a deeper understanding of concepts. Whether it’s solving equations, interpreting data, or graphing, practical exercises offer the hands-on experience needed to solidify learning.
1. Solving Linear Equations
Linear equations are fundamental in many areas of study. By practicing solving different types of linear equations, students can strengthen their problem-solving skills and gain confidence. These exercises help students familiarize themselves with different methods for isolating variables and finding solutions.
2. Graphing Coordinate Points
Graphing coordinates is another crucial skill that enhances spatial reasoning. By plotting various points on a coordinate plane, students can better understand how equations translate into visual representations. This skill is essential for interpreting functions and relationships between variables.
| Exercise Type | Description | Recommended Practice |
|---|---|---|
| Solving Linear Equations | Practice isolating variables and finding solutions | Start with simple equations and gradually increase difficulty |
| Graphing Coordinates | Plot points on a coordinate plane and interpret their meaning | Use graphing tools or draw on paper for better visualization |
| Word Problems | Apply equations to real-world scenarios | Work through problems step-by-step, focusing on key information |
Incorporating these exercises into regular study sessions will help solidify foundational skills and prepare students for more advanced topics. By focusing on a variety of exercises, learners can approach problems from different angles and improve their overall understanding.
How to Review and Prepare for Exams

Effective exam preparation involves more than just memorizing facts; it requires a structured approach to consolidate knowledge, build confidence, and ensure success. The key to successful review lies in identifying critical concepts, practicing problem-solving techniques, and reviewing mistakes to avoid repeating them. By focusing on a few essential strategies, students can enhance their understanding and improve their performance on the exam.
1. Start Early – Begin your review well before the exam date. Spreading out study sessions allows for better retention and understanding. Cramming at the last minute is rarely effective and can cause unnecessary stress.
2. Focus on Key Topics – Identify the most important concepts or areas where you struggle the most. Concentrate your efforts on these topics to ensure a deep understanding. Pay attention to any areas highlighted by your instructor or practice exams.
3. Use Practice Problems – Solving problems similar to those expected on the exam is one of the most effective ways to prepare. Practice will help you apply theoretical knowledge to practical situations and develop problem-solving strategies.
Study Techniques
- Active Recall: Test yourself regularly on key concepts without looking at notes.
- Spaced Repetition: Review material at increasing intervals to improve long-term retention.
- Group Study: Collaborate with peers to discuss difficult concepts and solve problems together.
4. Review Mistakes – When practicing problems or completing assignments, take time to review any mistakes you made. Understanding why an answer is incorrect will help you avoid similar errors on the exam.
5. Time Management – During your review, create a study schedule to allocate enough time to each subject. Prioritize tasks based on importance and difficulty. On exam day, ensure you manage your time wisely so you can complete all sections.
6. Stay Positive and Rested – A calm and rested mind performs better than a stressed one. Get enough sleep before the exam, and try to stay positive about your preparation. Positive thinking boosts confidence and reduces exam anxiety.
By following these strategies, you will be well-prepared to face the exam with confidence and a clear mind, giving you the best chance for success.
Additional Resources for Further Study
Expanding your understanding beyond textbooks and class materials can significantly enhance your learning experience. There are numerous tools and resources available to help reinforce key concepts and provide deeper insights into the subject matter. These resources range from online platforms and interactive tools to textbooks and study guides, offering a variety of learning styles to suit different needs.
Online Learning Platforms
- Khan Academy: Offers free lessons and exercises on a wide range of topics with clear explanations and practice opportunities.
- Coursera: Provides courses from top universities and institutions, including free and paid options on advanced topics.
- EdX: Another online platform offering courses on various subjects, including comprehensive study materials and resources from renowned universities.
Books and Study Guides
- Principles of Algebra: A helpful textbook that covers foundational concepts in algebra and equations.
- Schaum’s Outlines: A series of study guides that provide solved problems, examples, and step-by-step explanations on a variety of topics.
- Practice Workbook: Find workbooks dedicated to problem-solving practice, which are excellent for reinforcing learned concepts.
Utilizing these resources can be especially useful when you’re preparing for exams or struggling with certain topics. Combining different types of learning tools–whether through online tutorials, self-paced courses, or detailed guides–ensures a well-rounded understanding and helps you tackle any difficulties you may encounter.