
In this section, we focus on reinforcing the essential mathematical principles that are pivotal to progressing in higher-level coursework. By understanding and applying these foundational concepts, students can build confidence and skill in their problem-solving abilities. This guide aims to simplify complex topics and provide clarity on how to navigate through challenging exercises.
To succeed in mathematics, it’s crucial to approach each problem methodically, breaking down equations and expressions step by step. With clear explanations and strategic solutions, this guide will assist in identifying common difficulties and offer practical techniques to overcome them. Whether you are reviewing material or preparing for future exams, these insights will sharpen your mathematical reasoning.
By practicing regularly and mastering the fundamentals, you’ll be well-equipped to tackle more advanced problems with ease. The goal is not just to complete exercises but to understand the underlying logic and structure behind them. A solid grasp of these key topics will serve as a strong base for future studies.
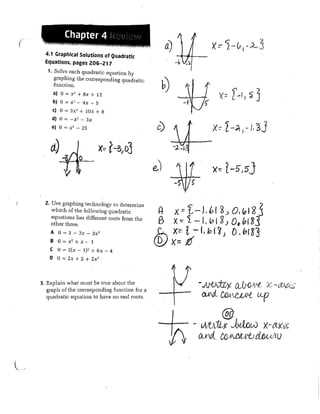
Algebra 1 Chapter 4 Test Answer Key
In this section, we provide a comprehensive guide to the solutions for the exercises in this particular unit. Each problem is broken down into manageable steps, designed to help clarify the methods used to arrive at the correct results. By understanding the reasoning behind each solution, students can enhance their ability to tackle similar challenges in the future.
Detailed Solutions and Explanations

To fully grasp the techniques involved, it’s important to follow through each solution with careful attention to detail. For each exercise, we outline the necessary steps to isolate variables, simplify expressions, and apply appropriate mathematical rules. These explanations serve not only to correct mistakes but also to strengthen understanding for future exercises.
How to Review and Improve
Reviewing solutions is a crucial part of the learning process. After working through the problems, students should revisit each step to confirm their understanding. Identifying any mistakes made along the way provides an opportunity for growth and improvement. With practice, this methodical approach will lead to greater confidence and proficiency in handling similar problems.
Overview of Chapter 4 Topics

This section introduces the key mathematical concepts that form the foundation of the current unit. The focus is on developing skills in solving complex equations and understanding various problem-solving strategies. The material covered will empower students to approach mathematical challenges with more confidence and precision.
Main Concepts Covered
Throughout this unit, students will encounter a variety of techniques and problem types designed to enhance their mathematical reasoning. Topics include working with different forms of equations, simplifying expressions, and applying logical methods to find solutions. The ability to understand these concepts will play a crucial role in advancing through the course.
Concepts Breakdown

| Topic | Description |
|---|---|
| Solving Linear Equations | Techniques for finding the value of variables in simple equations |
| Graphing Functions | Understanding the visual representation of mathematical expressions |
| Working with Polynomials | Operations and simplification of polynomial expressions |
| Factoring Expressions | Methods for breaking down complex expressions into simpler factors |
Key Concepts in Algebra 1
In this section, we explore the fundamental principles that are crucial for mastering mathematical reasoning. The topics covered lay the groundwork for solving equations, manipulating expressions, and understanding the relationships between variables. Grasping these core ideas is essential for further mathematical progression and success in subsequent courses.
Understanding Equations and Expressions
One of the most important aspects of this unit is developing the ability to work with equations and expressions. Solving equations involves isolating variables and applying appropriate mathematical operations. Simplifying expressions allows for easier manipulation and solving of more complex problems. These skills are necessary for both academic success and real-world problem solving.
Graphing and Interpreting Functions
Graphing functions provides a visual representation of mathematical relationships. Interpreting graphs helps in understanding how variables interact and change. Learning to plot points, draw lines, and interpret curves will give students a deeper insight into mathematical models and their practical applications. This skill is foundational for analyzing data and solving real-world problems.
How to Approach Chapter 4 Problems

Successfully solving mathematical problems requires a strategic approach. By breaking down each problem into manageable steps and focusing on key techniques, you can avoid common pitfalls and improve your problem-solving efficiency. In this section, we’ll discuss practical strategies for tackling challenges effectively and with confidence.
Step-by-Step Problem Solving
When faced with a complex problem, it’s essential to approach it in a systematic way. Follow these steps to help guide you through the process:
- Read the problem carefully: Understand the given information before attempting to solve it.
- Identify the variables: Determine what you are solving for and what unknowns need to be addressed.
- Apply the correct operations: Use the appropriate mathematical rules to manipulate the equation or expression.
- Check your work: Once you’ve arrived at a solution, verify it by substituting the result back into the original problem.
Common Strategies for Success
Here are some additional strategies to enhance your problem-solving skills:
- Look for patterns: Identifying repeating structures or relationships between variables can simplify complex problems.
- Work with similar problems: Practicing similar exercises helps reinforce concepts and makes it easier to recognize familiar techniques.
- Break problems into smaller parts: Divide larger challenges into smaller, more manageable sections to reduce confusion and minimize mistakes.
Understanding Equations in Chapter 4
This section focuses on the essential principles behind solving equations. By mastering these foundational concepts, students will gain the ability to approach more complex problems with confidence. Understanding how to isolate variables, apply operations correctly, and interpret the results is key to mastering mathematical challenges in this unit.
Key Techniques for Solving Equations
Solving equations involves using systematic steps to manipulate and simplify expressions. It’s important to understand how different operations affect the equality of an equation. By learning the most effective methods for isolating unknowns, students can easily find solutions to a wide range of problems.
Common Types of Equations
There are several types of equations that students will encounter, each requiring a specific approach to solve. Below is a table summarizing some of the most common equations and their solving methods:
| Equation Type | Method of Solving |
|---|---|
| Linear Equations | Simplify and isolate the variable by performing inverse operations |
| Quadratic Equations | Factor or apply the quadratic formula to find the solutions |
| Systems of Equations | Use substitution or elimination to solve for variables |
| Rational Equations | Eliminate fractions by multiplying both sides by the least common denominator |
Step-by-Step Solutions for Each Problem

Breaking down each problem into manageable steps is crucial for understanding the solution process. By following a clear and logical sequence, you can ensure accuracy and build a deeper understanding of the methods used to reach the final result. This approach will make even the most complex problems easier to solve.
Steps to Solve Problems Efficiently
For each type of problem, follow these general steps to guide you through the solution:
- Identify the given information: Carefully read through the problem and extract all known values.
- Set up the equation: Translate the word problem or expression into a mathematical equation, ensuring that all components are correctly represented.
- Perform operations: Apply the appropriate mathematical operations, such as addition, subtraction, multiplication, or division, to simplify the equation.
- Isolate the variable: Use inverse operations to isolate the variable on one side of the equation.
- Check your solution: Substitute the found value back into the original equation to verify the result.
Example Problem Breakdown
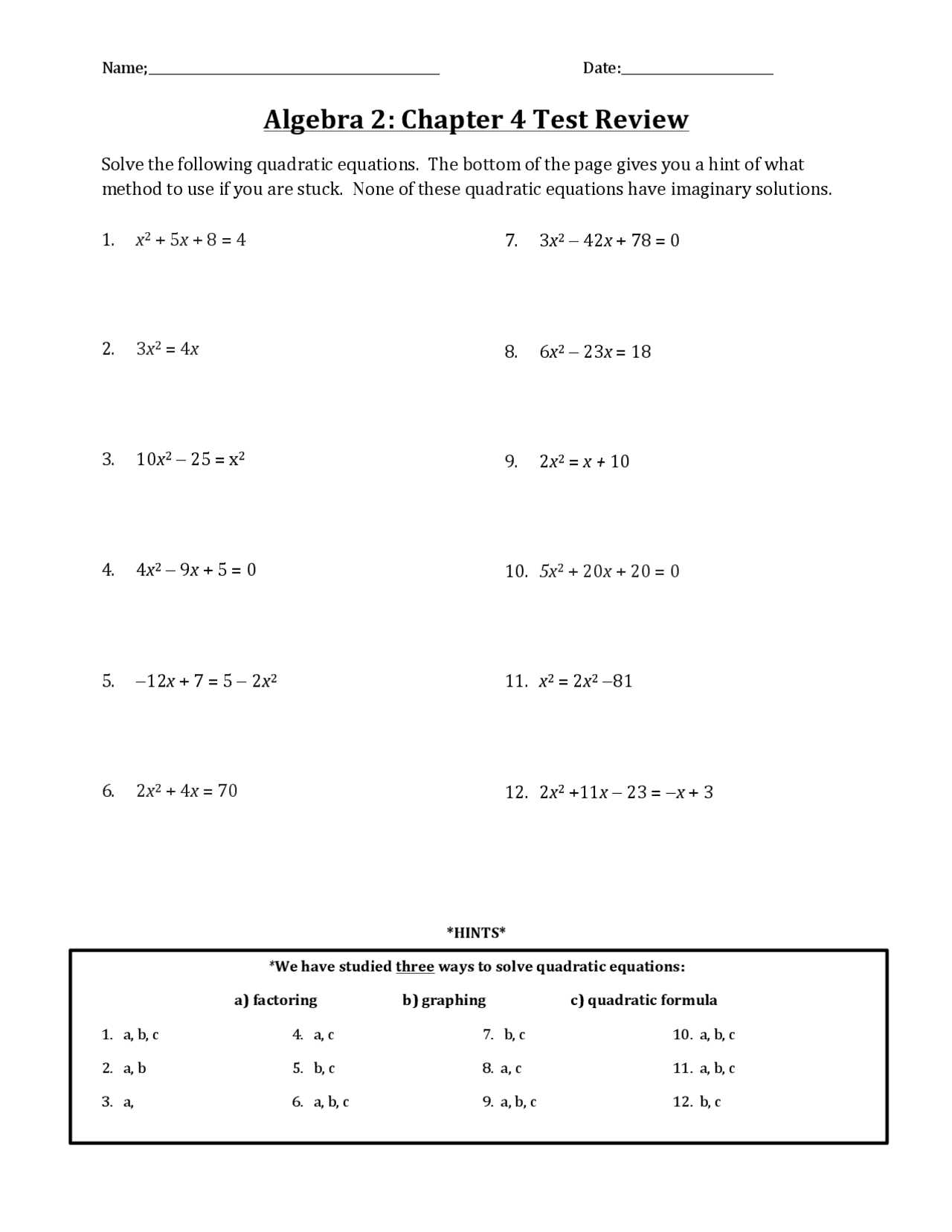
Let’s take a look at how these steps are applied to a typical problem:
- Problem: Solve for x: 3x + 5 = 20
- Step 1: Subtract 5 from both sides: 3x = 15
- Step 2: Divide both sides by 3: x = 5
- Step 3: Check the solution by substituting x = 5 back into the original equation: 3(5) + 5 = 20, which is correct.
Common Mistakes in Chapter 4 Tests
Even the most well-prepared students can make errors when solving mathematical problems. Understanding the common pitfalls can help avoid unnecessary mistakes and improve performance. In this section, we will highlight frequent errors that often arise during problem-solving and provide tips on how to prevent them.
Typical Errors and How to Avoid Them
- Misinterpreting the problem: One of the most common mistakes is not fully understanding the problem. Always read the question carefully and identify all given information before attempting a solution.
- Incorrectly applying operations: Using the wrong operation can lead to an incorrect solution. Be sure to follow the correct order of operations (PEMDAS) and check each step as you go.
- Not simplifying expressions: Forgetting to simplify intermediate expressions can result in a more complex problem. Always reduce expressions to their simplest form before moving on to the next step.
- Skipping the check: Failing to check your work is a major cause of errors. After solving, always substitute your result back into the original equation to verify its correctness.
How to Avoid These Mistakes

- Double-check each step: Before moving to the next step, review your calculations to make sure everything is correct.
- Work systematically: Break down each problem into smaller steps to ensure no part is overlooked.
- Practice regularly: The more you practice solving problems, the better you’ll become at recognizing potential mistakes and avoiding them.
- Ask for clarification: If a problem seems unclear, don’t hesitate to ask for help to ensure you’re on the r
Tips for Solving Algebraic Expressions
When working with mathematical expressions, it’s crucial to follow a structured approach. These expressions often contain variables and constants that require specific methods to simplify or solve. By applying the right strategies, you can tackle these problems efficiently and accurately.
Essential Strategies for Simplification
- Identify like terms: Combine terms that have the same variable raised to the same power. This will simplify the expression and make it easier to solve.
- Use the distributive property: When multiplying a number by a group of terms inside parentheses, apply the distributive property to remove parentheses and simplify the expression.
- Pay attention to signs: Properly managing positive and negative signs is essential. Always double-check when adding or subtracting terms to avoid sign errors.
- Factor when possible: If the expression can be factored, it can often be simplified further. Look for common factors or use factoring techniques to break down complex expressions.
Common Techniques for Solving
- Isolate the variable: When solving for a variable, move all terms involving that variable to one side of the equation using inverse operations.
- Simplify both sides: Before solving, simplify both sides of the equation as much as possible to make the calculations easier.
- Check your work: After obtaining a solution, substitute the result back into the original equation to ensure your answer is correct.
Practice Problems to Master Chapter 4
To truly grasp the concepts introduced in this section, consistent practice is essential. By working through various problems, you will strengthen your skills and become more confident in solving similar questions. These exercises are designed to help you apply the techniques you’ve learned and reinforce your understanding of the material.
Regularly practicing different types of problems ensures that you can approach any challenge with the right strategy. It also allows you to identify areas where you might need further review. Here are some practice problems to help you master the concepts covered in this section:
- Solve for x: 5x – 3 = 17
- Simplify the expression: 3(2x + 4) – 5x
- Factor the expression: x^2 + 5x + 6
- Find the solution to the system of equations:
- 2x + 3y = 12
- x – y = 1
- Evaluate the expression for x = 2: 4x^2 + 3x – 7
By solving these problems, you will improve your ability to handle similar tasks and strengthen your understanding of the methods required. Be sure to check your work and revisit any problem areas to ensure complete comprehension.
How to Check Your Answers Effectively
Reviewing your work is an essential part of problem-solving, allowing you to catch any mistakes and ensure accuracy. Taking the time to verify each step can help you identify where things went wrong and improve your overall understanding. In this section, we will explore strategies for checking your results thoroughly.
Steps for Verifying Solutions
- Substitute back into the original equation: After solving, substitute your solution back into the original expression or equation to see if it holds true. If both sides are equal, the solution is correct.
- Double-check your calculations: Review every step carefully. Look for any arithmetic mistakes, misapplied operations, or skipped steps that may have led to an incorrect answer.
- Check for consistency: If your solution involves multiple variables or steps, ensure that each part is consistent and that you haven’t overlooked any details.
Tips for Improving Accuracy
- Work methodically: Keep a systematic approach when solving problems. This reduces the likelihood of missing important details or making careless mistakes.
- Take breaks if needed: If you feel fatigued, take a short break and return with a fresh perspective. Fatigue can lead to overlooked errors.
- Use a different method: If you’re unsure about your solution, try approaching the problem from a different angle. This can help confirm whether the answer is accurate.
Explaining Graphs and Functions in Chapter 4
Understanding the relationship between variables is crucial when working with mathematical problems. Graphs and functions provide a visual representation of these relationships, making it easier to analyze and solve complex problems. In this section, we will explore how to interpret and create graphs and how functions can help us understand variable interactions.
Graphing Functions
Graphs allow us to visualize how one quantity affects another. Each point on a graph represents a solution to an equation, with the x-axis typically showing the independent variable and the y-axis representing the dependent variable. By plotting these points, you can identify patterns, trends, and key features like intercepts and slopes.
- Identify key points: Start by plotting the known points of the function. These could be points where the function intersects the axes or other critical points given in the problem.
- Determine the slope: The slope represents how much the dependent variable changes for each unit change in the independent variable. A steeper slope indicates a faster change.
- Use graphing tools: Graphing calculators or software can make the process faster and more accurate, especially for complex functions.
Understanding Functions
Functions define the relationship between two variables where each input corresponds to exactly one output. Understanding how to work with functions involves recognizing how different inputs will produce different results, and how these outputs are related in a meaningful way.
- Recognize function notation: A function is often written as f(x), where f is the name of the function and x is the input. This helps to differentiate between various functions and their corresponding results.
- Graph functions accurately: When graphing functions, make sure to plot enough points to clearly show the pattern or trend. Look for behaviors like increasing, decreasing, or constant values over the range of inputs.
- Analyze function transformations: Functions can be shifted, stretched, or reflected, which affects their graphs. Identifying these transformations helps in interpreting and solving problems more efficiently.
Test-Taking Strategies for Algebra 1
Effective strategies can make a significant difference when approaching any type of assessment. The goal is to manage time wisely, minimize errors, and ensure that every question is addressed with care. By preparing thoughtfully and applying the right techniques during the exam, you can maximize your chances of success.
One of the most important aspects of test-taking is reading each problem thoroughly before attempting a solution. Ensure that you fully understand the question and identify the key information before diving into calculations. Take a moment to break down complex questions into manageable parts to avoid feeling overwhelmed.
During the exam, time management is crucial. Start with the questions that seem easiest to you, as they will build your confidence and ensure you accumulate points early on. Save the more challenging problems for later, giving yourself enough time to think through them carefully.
Finally, reviewing your work before submitting it is essential. If time allows, go over your solutions to check for any mistakes or missed steps. This final review can help catch simple errors that may have been overlooked during the initial process.
Why Chapter 4 is Crucial for Algebra 1

The concepts covered in this section are foundational to mastering more advanced topics in mathematics. Understanding how different operations and relationships work together prepares you for future challenges, whether you’re solving for unknowns, working with functions, or graphing data. Without a solid grasp of these core ideas, progressing through later material becomes more difficult, and solving problems becomes more complex.
Building Problem-Solving Skills
In this part of the course, you learn how to approach and solve a variety of problems that require logical reasoning and structured thinking. These skills are not just essential for mathematics but are also useful in real-life situations, such as budgeting, planning, or troubleshooting. Developing these techniques early ensures that you can handle more sophisticated concepts down the road.
Establishing Strong Mathematical Foundations
The tools and methods introduced here lay the groundwork for everything that comes next. Whether you’re tackling equations, functions, or inequalities, the fundamental understanding gained in this section serves as a stepping stone to more intricate concepts. Mastering this material allows you to move forward with confidence and success in subsequent topics.
Real-World Applications of Algebra Concepts
The concepts you learn in mathematics are not confined to the classroom; they are applicable in numerous real-life scenarios. From personal finance to engineering, understanding how variables and equations work can be extremely beneficial. By applying these mathematical principles, you can solve everyday problems, make informed decisions, and even predict future outcomes in a variety of fields.
Finance and Budgeting
One of the most practical applications of these concepts is in managing finances. Whether you’re calculating interest rates on loans, planning a budget, or saving for a goal, understanding how to manipulate variables helps you make more informed financial decisions. For example, using equations to determine monthly payments on a car loan or figuring out how much you need to save each month to reach a financial target involves skills learned in this area.
Engineering and Technology

In fields like engineering, technology, and science, understanding relationships between variables is crucial. Whether you’re designing a building, creating a software program, or calculating the necessary materials for a project, mathematical principles are used to ensure everything fits together as planned. By grasping the fundamentals of equations and functions, professionals can model complex systems and predict how different variables interact in real-world applications.
Improving Your Algebra 1 Skills
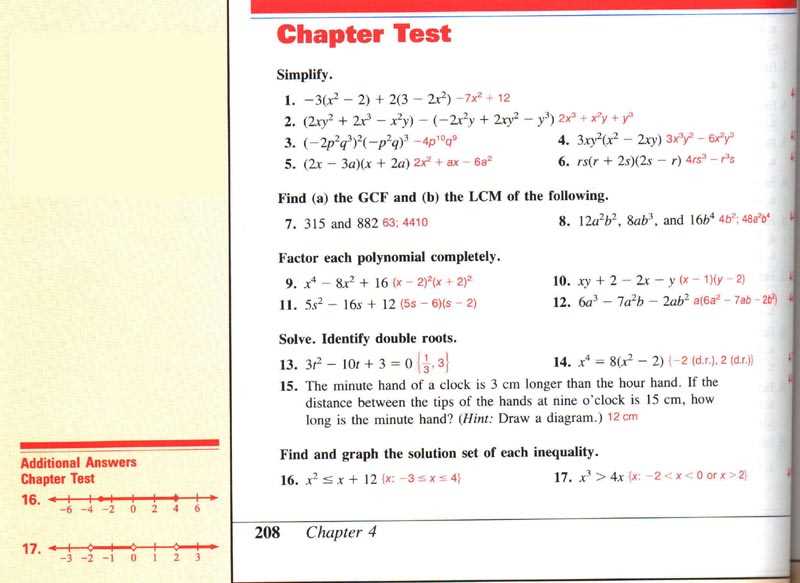
Mastering mathematical concepts requires practice, patience, and a clear strategy. The more you engage with different types of problems, the more confident you’ll become in applying mathematical methods. By identifying areas where you struggle and focusing your efforts on them, you can significantly improve your problem-solving abilities and build a strong foundation for future topics.
Practice Regularly and Consistently
Consistent practice is key to improving your skills. Set aside dedicated time each day or week to solve problems, review concepts, and reinforce what you’ve learned. The more problems you tackle, the more familiar you become with different approaches and solutions, making it easier to understand complex ideas. Start with simpler exercises and gradually work your way up to more difficult challenges.
Seek Help When Necessary
Don’t hesitate to ask for help when needed. Whether it’s from a teacher, tutor, or peer, clarifying your doubts can prevent confusion from building up. Sometimes, a different explanation or perspective can help you see a concept in a new light. Additionally, online resources and practice problems can provide additional support and insight into areas where you need improvement.
Resources to Help You Study Chapter 4
Studying effectively requires the right set of tools and resources. Whether you prefer learning through videos, reading materials, or interactive platforms, there are numerous resources available to reinforce your understanding of mathematical concepts. Utilizing a combination of textbooks, online tutorials, and practice exercises can help you grasp difficult ideas and improve your problem-solving skills.
One of the most beneficial resources is your course textbook, which provides a structured approach to the topics covered. Additionally, many educational websites offer free tutorials and explanations that break down concepts step-by-step. Interactive platforms such as math apps or online problem solvers also provide hands-on practice to apply what you’ve learned.
Don’t forget to use study groups or forums where you can discuss problems and solutions with others. This collaborative learning approach often brings new insights and alternative methods for solving problems. Together, these resources can enhance your comprehension and boost your confidence as you prepare for assessments.
What to Do After Completing the Test
Once you have finished your assessment, it’s important to take a few key steps to ensure that you’ve done your best and are prepared for the next challenge. First, take a moment to review your work and verify that you’ve answered all questions to the best of your ability. It’s common to overlook small mistakes or misinterpretations during the initial attempt, so a second look can often help catch these errors.
Next, ensure that your responses are clearly written and that you’ve followed all instructions provided. Double-check that your work is organized, especially if you’ve used multiple steps to solve a problem. It’s essential to have your solutions clearly outlined to avoid confusion, whether you are reviewing them yourself or submitting them for grading.
If possible, discuss the assessment with peers or your instructor to gain additional insights. This can be a valuable learning opportunity, allowing you to understand areas where you may have struggled and learn from others’ approaches. Lastly, don’t forget to reflect on what you’ve learned and identify areas where further study might be needed.
Action Benefit Review your work Helps identify mistakes and missed questions Organize your responses Ensures clarity and easy understanding Discuss with peers/instructor Provides new insights and alternative approaches Reflect on areas for improvement Guides your future study focus