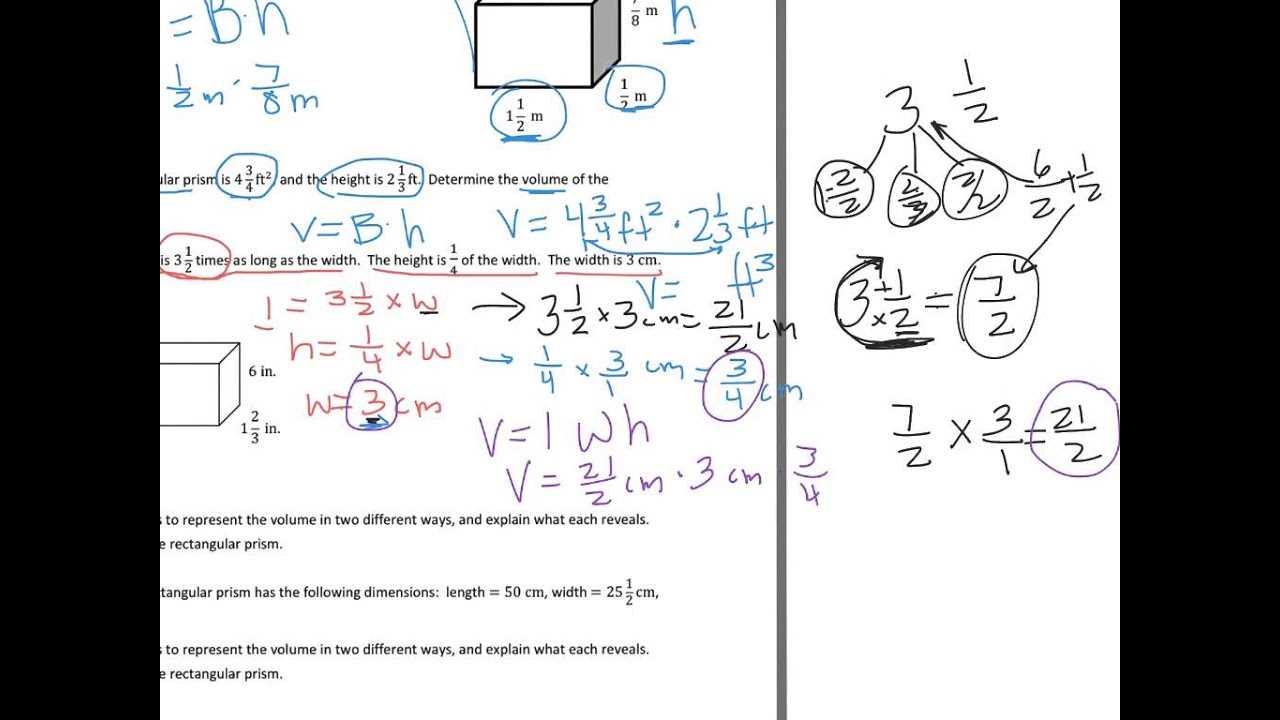
As students advance in their studies, understanding complex problem-solving strategies becomes essential. This section provides valuable resources designed to support learners in mastering key mathematical concepts. With a focus on clear, step-by-step solutions, students can improve their approach to difficult exercises and strengthen their grasp of critical topics.
By working through various exercises and analyzing detailed solutions, students can identify patterns and improve their skills in tackling more challenging problems. Each step in the process is explained in a way that enhances both comprehension and application. This guide aims to equip students with the tools necessary to excel and build confidence in their mathematical abilities.
Understanding the structure of these tasks and how they relate to real-world scenarios further enriches the learning experience. With proper guidance and practice, students will gain the confidence to approach new topics with enthusiasm and precision.
Sixth Grade Problem Solving Solutions
This section provides detailed solutions for the essential exercises students encounter while learning advanced concepts in their curriculum. It serves as a helpful tool for those looking to understand the correct methods of solving complex tasks, offering insight into step-by-step processes that can help clarify any confusion. With these resources, students can enhance their problem-solving skills and gain a deeper understanding of critical concepts.
Comprehensive Breakdown of Each Exercise
The provided solutions are broken down into manageable steps, each explained in detail. This approach allows learners to follow the logic behind each answer and replicate similar strategies when faced with new challenges. By focusing on specific techniques and examples, students can reinforce their knowledge and ensure they fully comprehend the material.
Improving Skills Through Practice and Application
By engaging with these exercises regularly, learners can strengthen their abilities and build confidence in their mathematical reasoning. These solutions not only offer the correct results but also emphasize the importance of understanding the process behind each answer, fostering a more comprehensive learning experience. This section is designed to empower students to think critically and apply their knowledge effectively across various topics.
Overview of Sixth Grade Curriculum
This section offers a comprehensive look at the essential topics covered in the sixth level of the educational program. It introduces key concepts and strategies designed to build a solid foundation for more advanced subjects. The focus is on helping students develop critical thinking skills, understand complex processes, and apply their knowledge to real-world scenarios.
Core Topics and Learning Goals
The curriculum is designed to ensure that students master the fundamental principles required for future success. Topics are chosen to gradually increase in difficulty, allowing students to build on previous knowledge. By exploring fractions, decimals, ratios, and other core concepts, learners are prepared to tackle more complex subjects in subsequent years.
Developing Problem-Solving Abilities
At this stage, the emphasis is placed on improving analytical and problem-solving skills. Students are encouraged to engage with increasingly challenging tasks, which help them to refine their ability to reason logically and work through complex scenarios. By practicing regularly, they can develop a deeper understanding of abstract concepts and enhance their confidence in solving difficult problems.
Key Topics in Module 5
This section covers the most important concepts and skills addressed in the fifth part of the curriculum. It highlights the critical areas that students need to focus on to build a strong foundation in advanced topics. By mastering these essential elements, learners will be prepared to handle more challenging tasks in future lessons.
Important Concepts
In this section, students explore several key ideas that are vital for understanding more complex mathematical operations. These include:
- Understanding fractions and their equivalent forms
- Applying decimals in real-world scenarios
- Working with ratios and proportions
- Developing strategies for comparing and ordering numbers
- Exploring the relationship between different types of numbers
Critical Skills to Master
In addition to the core concepts, there are important skills students need to develop for success. These include:
- Accurately performing calculations with fractions and decimals
- Solving real-life problems involving ratios and percentages
- Interpreting and solving word problems
- Applying logical reasoning to mathematical tasks
By practicing these skills, students will not only strengthen their understanding of the material but also become more confident in solving problems independently.
How to Use the Answer Key Effectively
Having access to solutions can be incredibly useful, but it’s important to use them in a way that enhances understanding, rather than simply providing quick fixes. To get the most out of this resource, students should approach the solutions with a mindset focused on learning and improving their problem-solving techniques.
Steps to Maximize Learning
Here are some strategies for using the solutions effectively:
- Work through problems first: Attempt each problem on your own before checking the solution. This helps identify areas where you may need additional practice.
- Understand the process: Focus on the steps taken to reach the final result, not just the answer itself. This will help reinforce your problem-solving skills.
- Compare your work: If your solution is different from the one in the resource, identify where your approach differed and adjust your method accordingly.
- Use as a guide: Treat the solution as a learning tool, not a shortcut. Review it thoroughly and try to replicate the steps on similar problems.
Benefits of Proper Use
When used correctly, solutions can provide valuable insights into efficient methods of solving problems. By taking the time to understand the steps involved, students can build a stronger grasp of the material and become more confident in their abilities. This approach ensures long-term retention and a deeper understanding of the subject matter.
Step-by-Step Solutions for Module 5
This section provides detailed, step-by-step explanations for each problem covered in the fifth unit of the curriculum. The goal is to help students understand the reasoning behind each solution, enabling them to apply similar strategies to new problems. By breaking down each task into manageable steps, students can clearly see how to approach and solve each exercise effectively.
Starting with the Basics: Each problem begins with the identification of key information and the formulation of a plan to solve it. By understanding the fundamental concepts, students can approach more complex tasks with confidence.
Step-by-Step Process: The solutions are broken down into logical stages, guiding students through each calculation or concept. These stages are designed to highlight essential techniques, ensuring that learners can follow along and replicate the steps on their own.
By carefully reviewing the steps, students will be able to see not only how to reach the correct answer but also why each step is necessary for the overall solution. This approach fosters a deeper understanding and reinforces the skills needed for problem-solving in future lessons.
Understanding Problem-Solving Strategies
Mastering problem-solving techniques is essential for successfully navigating challenging tasks. This section focuses on the different strategies students can employ to break down complex problems and find effective solutions. The goal is to provide students with a set of tools they can use to approach problems with confidence and clarity.
Key Strategies to Apply
There are several proven approaches to solving problems. By understanding and practicing these techniques, students can enhance their problem-solving skills:
| Strategy | Description | When to Use |
|---|---|---|
| Visualizing the Problem | Creating diagrams or graphs to represent the problem helps clarify relationships between elements. | When dealing with problems involving measurements, graphs, or geometric shapes. |
| Breaking Down the Problem | Dividing the problem into smaller, manageable parts makes it easier to solve step by step. | For complex problems that seem overwhelming at first glance. |
| Working Backwards | Starting from the desired outcome and reversing the steps can often lead to the solution. | When trying to solve a problem with multiple possible solutions. |
| Making an Estimate | Estimating values before solving can help determine the reasonableness of the final answer. | In problems where exact numbers are difficult to work with or when quick approximations are needed. |
Applying Strategies to Real Problems
In real-world situations, problems may involve more than one strategy. Students should practice combining techniques to find the most efficient solution. For example, combining visualization with breaking down a task into smaller parts can lead to faster, more accurate results. Over time, these strategies become second nature, allowing learners to approach any problem with a methodical and logical mindset.
Common Mistakes in Module 5 Exercises
While working through exercises, students often make similar errors that can hinder their progress. Identifying and understanding these mistakes is crucial for improvement. In this section, we will explore some of the most frequent errors students encounter in this set of problems and provide tips on how to avoid them.
Incorrect Application of Operations
A common mistake is misapplying basic operations when solving problems. For example, students might incorrectly add instead of subtracting, or mix up multiplication and division when dealing with fractions or decimals. It’s important to carefully consider the operation needed for each step.
- Confusing addition with subtraction in word problems.
- Multiplying when division is required, or vice versa.
- Forgetting to apply the correct order of operations.
Misinterpreting Word Problems
Word problems often contain extra information that can confuse students. Misinterpreting the question or overlooking key details can lead to incorrect answers. Students should focus on breaking down the problem into smaller parts and carefully identifying what is being asked.
- Overlooking units of measurement or important qualifiers in the problem.
- Misunderstanding the context of the problem, such as the relationship between different variables.
- Failing to extract the relevant information needed to solve the task.
By paying attention to these common mistakes and reviewing each problem carefully, students can avoid errors and improve their understanding of the material.
Tips for Mastering Module 5 Concepts
Mastering the core concepts in this unit requires consistent practice, understanding of key principles, and the ability to apply learned strategies to a variety of problems. In this section, we will explore practical tips to help students build a solid foundation and confidently tackle the challenges presented in this part of the curriculum.
Essential Strategies for Success
To truly grasp the material and excel in exercises, consider the following strategies:
| Strategy | Purpose | How to Implement |
|---|---|---|
| Regular Practice | Repetition helps reinforce learned concepts and improves retention. | Set aside time each day to review and solve problems related to the key topics. |
| Break Down Complex Problems | Dividing difficult tasks into smaller parts makes them more manageable. | Identify smaller steps within each problem and tackle them one at a time. |
| Use Visual Aids | Visual representations help clarify complex relationships and concepts. | Draw diagrams, charts, or number lines to help organize information and simplify calculations. |
| Understand Key Formulas | Knowing and applying essential formulas is crucial for solving problems efficiently. | Familiarize yourself with common formulas and practice using them in different scenarios. |
Review and Seek Help
If you encounter challenges, it’s important to review your work and seek guidance when necessary. Take time to re-examine each step, identify any mistakes, and ask for clarification if something isn’t clear. Discussing tricky concepts with peers or teachers can often lead to better understanding and new insights.
By applying these strategies consistently, students will gain confidence in their ability to handle the material and perform successfully in both assignments and assessments.
Practice Problems and Solutions
Engaging with practice exercises is one of the best ways to reinforce concepts and build problem-solving skills. This section provides a set of practice problems designed to challenge your understanding and application of the topics covered. Along with each problem, a detailed solution is provided to guide you through the process and ensure you understand each step.
| Problem | Solution |
|---|---|
| Solve the equation: 3x + 5 = 20 | Step 1: Subtract 5 from both sides: 3x = 15. Step 2: Divide both sides by 3: x = 5. |
| Find the perimeter of a rectangle with length 8 units and width 5 units. | Perimeter = 2(length + width). P = 2(8 + 5) = 2(13) = 26 units. |
| Calculate the area of a triangle with a base of 6 units and a height of 4 units. | Area = 1/2(base × height). A = 1/2(6 × 4) = 12 square units. |
| What is the value of 25% of 200? | 25% of 200 = 0.25 × 200 = 50. |
By practicing these problems and reviewing the provided solutions, you can gain a deeper understanding of the material and improve your ability to solve similar problems independently. It’s essential to practice regularly to ensure mastery of the skills needed for success.
How to Check Your Work
Checking your work is a critical step in the problem-solving process. It ensures accuracy and helps you identify any errors you may have overlooked during your initial attempt. In this section, we will discuss effective strategies for reviewing your solutions to ensure they are correct and well-constructed.
Step-by-Step Review Process
Begin by revisiting each step of your solution to verify that all calculations and logical steps are correct. Look for common mistakes such as simple arithmetic errors, misapplied formulas, or skipped steps. Here’s a basic checklist to follow:
- Recheck your calculations to ensure accuracy.
- Confirm that each step logically follows the previous one.
- Verify that the correct units are used throughout the solution.
Use Reverse Operations
One effective method to check your work is to apply reverse operations. This is especially useful in algebraic problems or when solving for unknown variables. For example, if you are solving for a variable, plug your solution back into the original equation to confirm that it satisfies the equation.
- Substitute your final solution back into the original problem.
- Check if both sides of the equation are equal.
- For word problems, verify that the solution makes sense in the context of the problem.
By using these techniques, you can improve the accuracy of your solutions and avoid common errors. Taking the time to thoroughly check your work will lead to greater confidence and better results.
Exploring Conceptual Understanding in Module 5
Building a deep understanding of the underlying concepts is essential for mastering the material. This section focuses on the key principles and their applications, providing insights into how to develop a stronger grasp of the topics. A conceptual understanding goes beyond memorizing formulas and procedures; it involves recognizing the relationships between ideas and seeing how they work together to solve problems.
The Importance of Grasping Core Concepts
Understanding the core ideas of a subject allows you to apply them in various contexts and situations. Instead of focusing solely on getting the correct answer, focus on the logic behind each step. Here are some tips to enhance your conceptual understanding:
- Ask “why” and “how” questions to explore the reasoning behind each step.
- Look for connections between different topics to see how they interrelate.
- Practice explaining the concepts in your own words to reinforce your understanding.
Visualizing Concepts for Better Clarity
Using visual aids can significantly improve your understanding of abstract ideas. Diagrams, graphs, and charts provide a clear representation of relationships and make complex concepts easier to comprehend. Visualizing problems can help break down complicated information into simpler, more manageable parts.
- Draw diagrams to illustrate problems and solutions.
- Create flowcharts or mind maps to organize your thoughts and see connections between concepts.
- Use graphs and tables to visualize data and relationships.
By focusing on understanding the “why” and “how” behind the content, you will build a solid foundation that supports future learning and problem-solving abilities. Understanding the big picture will enable you to tackle more advanced challenges with confidence and clarity.
Connections to Previous Math Modules
Understanding the continuity between different topics in a curriculum is essential for mastering new concepts. Each new section builds upon the knowledge and skills developed in previous lessons. In this section, we will explore how the concepts introduced in this particular unit are linked to those learned earlier, helping to reinforce your understanding and make connections across the entire learning experience.
Review of Key Concepts from Previous Lessons
The material covered earlier serves as a foundation for the current lessons, and reviewing these concepts will help strengthen your grasp of the new content. Here are some important areas to revisit:
- Understanding basic operations and their applications in problem-solving.
- Exploring fractions, decimals, and percentages as they are essential for more advanced topics.
- Recognizing patterns and relationships between numbers to help solve complex problems.
How Previous Skills Enhance Current Learning
The skills developed in earlier lessons are not just isolated concepts; they work together with new material to form a complete understanding. For example, your ability to manipulate fractions and decimals will directly help you solve more complex problems involving ratios and proportions. Likewise, your understanding of basic geometric principles will be useful when applying them to solve multi-step problems in later sections.
- Apply fraction and decimal operations to solve more complex ratios.
- Use earlier knowledge of number patterns to make sense of new problem structures.
- Review previous geometry concepts to enhance your ability to visualize and solve geometric problems.
By connecting earlier learning with the current unit, you will reinforce your problem-solving strategies and make it easier to grasp new material. Building on past knowledge allows you to approach more difficult concepts with greater confidence and understanding.
Mastering Fractions and Decimals
Understanding fractions and decimals is crucial for solving many types of problems in mathematics. These concepts form the foundation for more advanced topics and are widely used in everyday life. Mastery of fractions and decimals allows you to approach problems involving measurements, percentages, and ratios with confidence. In this section, we will discuss key strategies and techniques for mastering these essential concepts.
Key Concepts to Focus On
To truly grasp fractions and decimals, it’s important to understand their fundamental properties and how they interact with each other. Here are some key areas to focus on:
- Converting between fractions and decimals.
- Understanding equivalent fractions and their role in simplifying problems.
- Performing operations such as addition, subtraction, multiplication, and division with fractions and decimals.
- Recognizing when to use fractions or decimals in real-world applications.
Effective Strategies for Mastery

Mastery of fractions and decimals requires consistent practice and a few key strategies to help reinforce learning. Consider these helpful tips for improving your understanding:
- Practice converting fractions to decimals and decimals to fractions until the process becomes second nature.
- Work with visual aids like fraction bars or decimal grids to see the relationship between fractions and decimals.
- Break complex operations into smaller, manageable steps to avoid confusion.
- Use real-life examples, such as cooking measurements or money, to understand the practical applications of fractions and decimals.
With steady practice and a solid understanding of these core concepts, you’ll be well-equipped to tackle a wide range of problems involving fractions and decimals, both in academic settings and in everyday situations.
Real-World Applications of Module 5
The skills and concepts learned in this section have widespread applications beyond the classroom. Whether you’re managing a budget, cooking, or calculating distances, understanding the principles covered here can help solve real-world problems. This knowledge is not just theoretical; it has practical uses in daily life, making tasks more manageable and efficient.
Practical Uses in Daily Life
Here are some of the ways these concepts are applied in everyday scenarios:
- Budgeting and Finance: Understanding fractions and decimals is essential when managing personal finances. Whether you’re dividing a bill, calculating discounts, or tracking expenses, these skills help you make accurate calculations and decisions.
- Cooking and Measurements: Recipes often require adjusting ingredients based on the number of servings. Converting between fractions and decimals is key when increasing or decreasing portion sizes.
- Shopping and Discounts: When shopping, being able to calculate percentages, like sales tax or discounts, is essential. This helps you know exactly how much you’ll pay for an item after a price reduction or added tax.
- Travel and Distance: Estimating time, speed, and distance is another example of real-world use. Whether you’re planning a trip or calculating travel time, fractions and decimals help you make accurate calculations.
Applications in Professional Fields
In addition to daily life, these concepts are also critical in many professional fields. Here are a few examples:
- Engineering: Engineers often work with measurements and calculations that require converting between fractions and decimals, especially when working with blueprints or designing structures.
- Construction: Construction projects rely on precise measurements, and the ability to work with fractions and decimals is crucial when cutting materials or estimating project costs.
- Healthcare: Medical professionals use fractions and decimals when administering medication doses based on weight or body surface area, ensuring accurate treatments.
By understanding these concepts and their real-world uses, students can better appreciate the relevance of their studies and how they can apply what they learn in meaningful ways.
Common Challenges for Grade 6 Students
As students progress through their academic journey, they often face new hurdles that require greater focus and understanding. In the sixth year of their learning, they are introduced to more complex concepts that demand critical thinking and problem-solving skills. While some students find the transition challenging, identifying these common obstacles can help them navigate through difficulties and build a stronger foundation for future learning.
Key Struggles in Learning
Here are some of the most common challenges that students often encounter:
- Conceptual Understanding: Many students struggle with grasping abstract concepts and applying them to real-life situations. This can lead to difficulties in understanding new topics that build on prior knowledge.
- Working with Fractions and Decimals: Fractions, decimals, and their conversions can be tricky for some learners. The process of adding, subtracting, multiplying, and dividing these numbers requires precision and practice.
- Problem-Solving Skills: As exercises become more complex, students may find it challenging to break down word problems into smaller, manageable parts and identify the correct approach to solve them.
- Time Management: Balancing multiple assignments and staying on top of deadlines can be difficult, especially as the workload increases. Students often need guidance in managing their time effectively.
Emotional and Psychological Barriers
In addition to academic struggles, students may face emotional and psychological barriers that hinder their learning process:
- Fear of Failure: Some students may be afraid of making mistakes, which can lead to anxiety and a reluctance to engage with new material. This fear can prevent them from asking questions or seeking help when needed.
- Low Confidence: When students struggle with understanding certain topics, they may start to doubt their abilities. This can affect their motivation to continue learning and improve.
- Distractions and Lack of Focus: With more responsibilities and distractions, students may find it hard to focus during lessons or complete assignments efficiently.
Understanding and addressing these challenges early on can help students overcome obstacles and build the confidence needed to succeed in their studies.
Using Visual Aids to Support Learning
Visual aids play a crucial role in enhancing comprehension and retention of new concepts, especially when students are grappling with more challenging material. By incorporating images, diagrams, charts, and other visual tools, learners are able to make connections between abstract ideas and concrete representations. These aids provide a visual framework that helps break down complex information, making it more accessible and easier to understand.
Types of Visual Aids
There are several types of visual tools that can be used to reinforce learning and aid in problem-solving:
- Charts and Graphs: These are ideal for visualizing numerical data and trends. Bar graphs, pie charts, and line graphs help students see relationships and patterns between different sets of data.
- Diagrams: Diagrams, such as number lines, area models, and Venn diagrams, allow students to visually organize information and see how different elements are connected.
- Visual Step-by-Step Guides: Illustrations that show each step of a process can help students understand how to approach a problem methodically. These guides are particularly useful in showing procedural methods like solving equations or applying formulas.
- Interactive Tools: Online resources and software that allow students to manipulate variables, graphs, or shapes in real time help them develop a deeper understanding of concepts through hands-on experience.
Benefits of Using Visual Aids
Integrating visuals into lessons has numerous advantages for students:
- Improved Understanding: Visual aids help bridge the gap between theoretical knowledge and real-world applications. They allow students to see how abstract concepts work in practice.
- Better Memory Retention: Information presented visually is often easier to recall. The brain tends to remember images better than words, making it easier for students to retain information over time.
- Enhanced Engagement: Visuals make lessons more dynamic and engaging, encouraging active participation from students who might otherwise feel disengaged during traditional lectures.
By incorporating visual aids into learning routines, students can better grasp difficult concepts and retain them in a way that suits their individual learning styles.
How Parents Can Support Their Child
Parents play an essential role in their child’s educational journey, especially when it comes to mastering challenging concepts. By providing the right support at home, parents can help their child build confidence and develop strong problem-solving skills. Whether through encouragement, structured study time, or collaborative learning, there are many ways to foster an environment that promotes academic growth.
Creating a Positive Learning Environment
A productive study space is key to ensuring that children are focused and engaged in their work. Here are a few ways parents can set up an ideal environment:
- Minimize Distractions: Ensure the study area is quiet, well-lit, and free from distractions such as television or mobile phones. This allows the child to focus fully on their tasks.
- Provide Necessary Materials: Make sure that all tools, like notebooks, calculators, and writing supplies, are readily available to avoid interruptions during study sessions.
- Encourage Consistent Routines: Establish a regular schedule for homework and study time. A routine helps children stay organized and manage their time effectively.
Providing Emotional and Academic Support
In addition to creating the right environment, emotional and academic support is crucial for a child’s success. Parents can offer guidance in the following ways:
- Be an Active Listener: Encourage open communication. Let your child express their struggles or frustrations, and listen without judgment. This can help them feel supported and less overwhelmed.
- Offer Positive Reinforcement: Praise effort rather than just results. Acknowledge the hard work and perseverance that go into completing assignments, which can motivate them to keep going.
- Assist Without Taking Over: Provide guidance, but avoid doing the work for them. Help your child understand the steps needed to solve a problem, rather than simply giving them the solution.
By creating a supportive atmosphere, both emotionally and academically, parents can significantly impact their child’s ability to succeed and feel confident in their learning process.
Additional Resources for Module 5
To enhance understanding and provide further practice, students and educators can benefit from a variety of external resources. These materials can support the learning process by offering alternative explanations, interactive activities, and practice opportunities. Whether it’s through online platforms, textbooks, or video tutorials, additional resources can help reinforce key concepts and ensure mastery of the content.
Here are some valuable resources that can assist in mastering the concepts covered in this section:
- Interactive Websites: Platforms offering quizzes, games, and exercises can provide a fun way to practice and apply concepts. Many websites feature adaptive learning tools that adjust to the learner’s level.
- YouTube Channels: Educational YouTube channels often break down complex ideas into easy-to-understand videos. Visual demonstrations can help clarify concepts and provide step-by-step guidance.
- Practice Worksheets: Printable worksheets that align with the topics covered can offer extra practice and allow students to work at their own pace. These worksheets often come with solutions, so learners can check their progress.
- Online Forums: Engaging in discussion forums or study groups can be a great way to clarify doubts. Peers and educators can provide additional insights and help answer questions.
- Books and Study Guides: Comprehensive study guides and reference books are excellent for deepening knowledge. Many offer detailed explanations and practice problems to solidify understanding.
Utilizing these resources can provide students with the support they need to excel and gain a deeper understanding of the material. Combining classroom learning with external tools ensures a well-rounded approach to mastering the subject matter.