
In this section, we will explore important techniques and strategies for tackling a range of problems. By understanding the underlying principles, students can confidently approach each challenge with clarity and precision.
Solving complex questions requires a combination of logical reasoning and practical application of essential rules. Familiarizing yourself with the various steps and approaches ensures success in addressing each issue efficiently.
Practice and review play a crucial role in strengthening your skills. The more familiar you become with the structure of the problems, the easier it is to recognize patterns and apply the right solutions when it matters most.
Geometry Chapter 5 Test Form 1A Solutions
In this section, we will delve into the detailed solutions for various exercises, aiming to break down each step clearly and logically. By reviewing and understanding these responses, students can develop a deeper comprehension of the principles at play and enhance their problem-solving abilities.
Approaching the Problems with Precision

Each question requires a specific method to arrive at the correct solution. By recognizing the structure of the problems and applying the appropriate strategies, you can avoid common pitfalls and achieve accuracy in your results.
Key Techniques for Effective Problem Solving
Mastering the fundamental techniques for working through geometric questions is crucial. Whether it’s identifying the right formula or organizing your calculations in an orderly fashion, these strategies will ensure a smoother process and clearer outcomes.
Understanding Geometry Chapter 5 Concepts
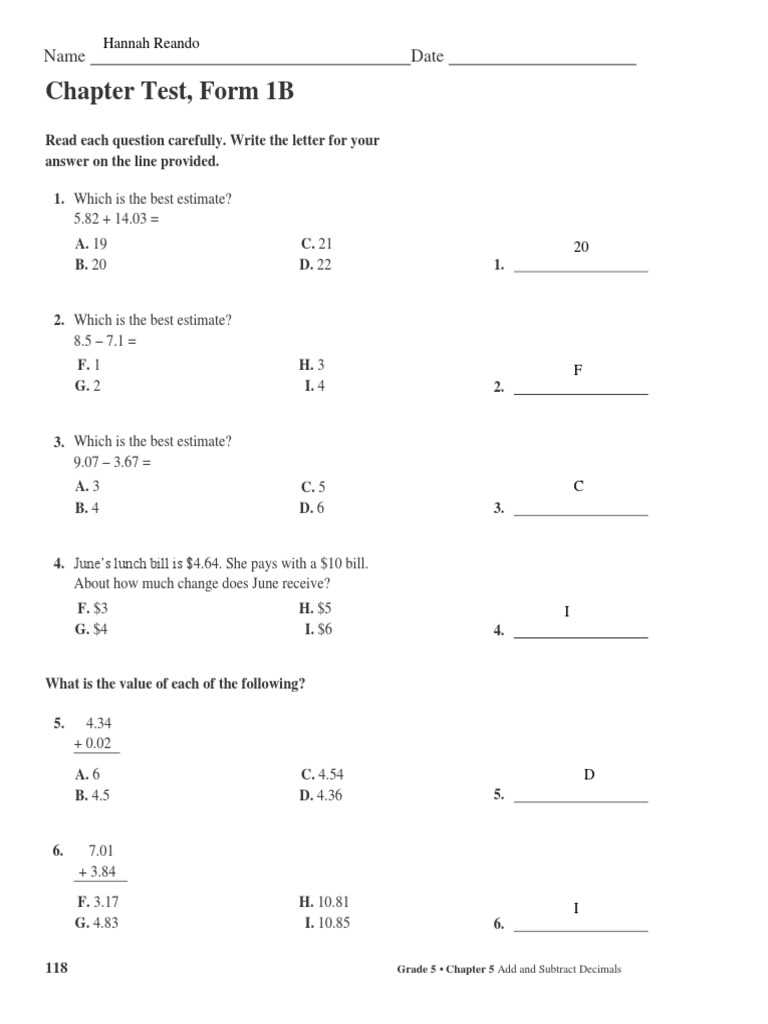
This section focuses on building a solid understanding of the essential principles and methods needed to solve the problems presented. By grasping these core ideas, you will be able to approach each challenge with greater confidence and accuracy.
Key Ideas and Techniques
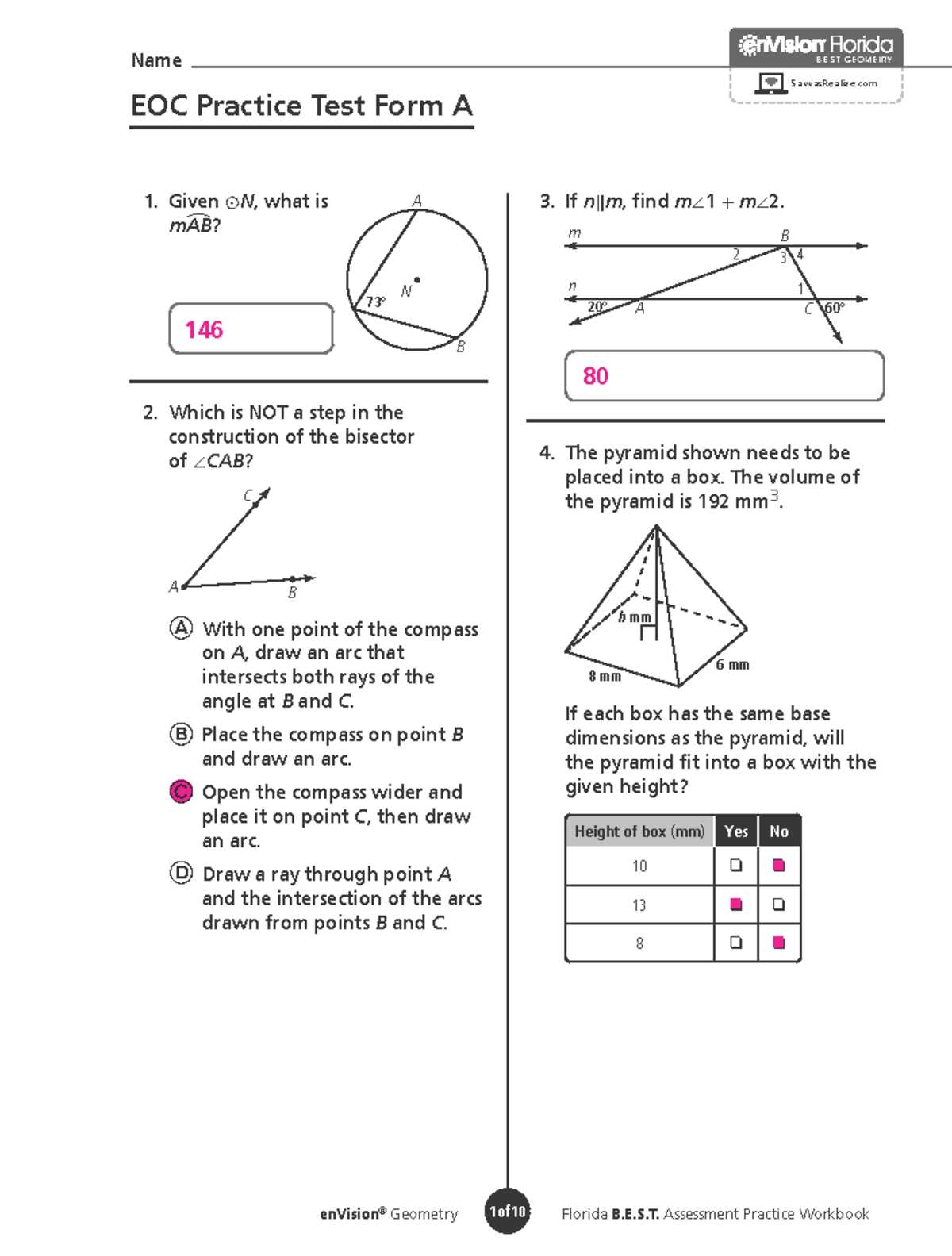
Familiarizing yourself with the core concepts will give you the tools necessary to navigate through various exercises. These ideas form the foundation for solving the problems and understanding how to manipulate geometric relationships effectively.
Important Theorems and Formulas

Several key theorems and formulas are crucial for solving questions efficiently. Knowing when and how to apply these formulas can drastically improve your ability to work through complex scenarios.
| Concept | Description | Formula/Rule |
|---|---|---|
| Angle Sum Property | The sum of angles in a triangle is always 180° | ∠A + ∠B + ∠C = 180° |
| Pythagorean Theorem | In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. | a² + b² = c² |
| Area of a Triangle | The area can be found using the base and height of the triangle. | Area = ½ × base × height |
How to Approach Form 1A Questions
When faced with a set of problems, it’s essential to approach each one systematically to ensure a clear and logical solution. Understanding the underlying concepts and breaking down each task into manageable steps will help you tackle the most complex challenges with confidence.
Step-by-Step Breakdown
Start by reading each question carefully to identify key details. Highlight important information, such as measurements or relationships between elements. Once you have a clear understanding, choose the appropriate method or formula to solve the problem. Taking time to organize your thoughts before diving into calculations can save time and reduce errors.
Check for Patterns and Relationships
Look for similarities between questions or recurring patterns in the problems. Often, the same concepts or formulas are applied in different ways, so recognizing these patterns can help you solve the problems more efficiently. By understanding how different elements interact, you can apply the most effective solution method to each situation.
Key Topics in Chapter 5 Test
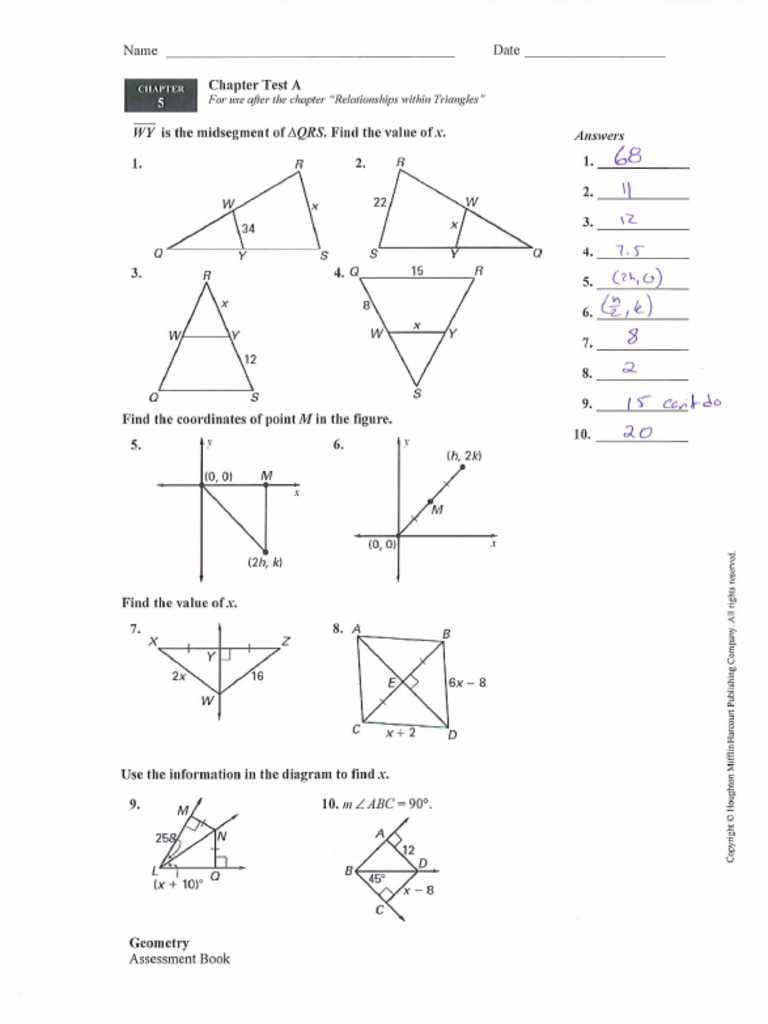
This section covers the essential concepts that are crucial for solving the problems effectively. Understanding these key areas will help you apply the right methods and formulas to tackle various challenges with ease.
Important Concepts to Focus On
In order to succeed, it’s important to be familiar with the following key ideas:
- Angles and their relationships
- Properties of different shapes
- Theorems related to parallel lines and angles
- Understanding the properties of triangles and quadrilaterals
- Calculating area and perimeter of basic figures
Common Techniques and Methods
Mastering these techniques will help you navigate through the problems efficiently:
- Using angle sum properties to solve for unknown angles
- Applying the Pythagorean theorem in right triangles
- Identifying symmetry in geometric figures
- Recognizing congruence and similarity in shapes
- Utilizing algebraic expressions to find unknown values
Step-by-Step Guide to Solutions
To solve complex problems effectively, it’s essential to break down each task into clear, manageable steps. Following a logical sequence ensures accuracy and helps avoid errors. By mastering a systematic approach, you can tackle even the most challenging exercises with confidence.
First, read the problem carefully to understand what is being asked. Identify key information such as measurements, relationships, and given conditions. This foundational step is crucial for determining the correct method to apply.
Next, select the appropriate formula or technique based on the problem’s requirements. Whether it’s using geometric properties or algebraic expressions, choosing the right approach will streamline the solution process.
Finally, carry out the calculations step by step, checking each result as you go. Ensuring every stage is completed accurately helps avoid mistakes and leads to a correct final answer.
Common Mistakes in Geometry Tests
Many students make certain errors while solving geometric problems, which can lead to incorrect results. Understanding these common mistakes can help you avoid them and improve your performance. Being aware of these pitfalls allows you to approach each question with greater care and precision.
One frequent mistake is misinterpreting the problem’s requirements. This can happen when students overlook specific conditions or fail to fully understand what is being asked. It’s important to read each question thoroughly and identify all the given information.
Another common error occurs when applying formulas incorrectly or at the wrong stage of the solution. It’s essential to know when and how to use each formula, especially in cases where there are multiple approaches to a single problem.
Additionally, not double-checking calculations or skipping intermediate steps can lead to minor mistakes that snowball into incorrect answers. Always verify each calculation to ensure consistency throughout the solution process.
Important Formulas to Remember
Mastering key formulas is crucial for solving a wide range of problems efficiently. These mathematical relationships serve as the foundation for most geometric calculations. Knowing when and how to apply each formula will greatly improve your ability to tackle questions accurately and quickly.
One essential formula is for calculating the area of a rectangle, which is found by multiplying its length by width. Similarly, the area of a triangle can be determined by multiplying the base by the height and dividing by two.
Another important formula is the Pythagorean theorem, which applies to right triangles. This formula states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. Additionally, understanding the properties of angles, such as the sum of angles in a triangle being 180°, is fundamental to solving many problems.
Tips for Efficient Problem Solving
Solving mathematical challenges can be daunting, but with the right approach, you can make the process much more manageable. By using effective strategies, you can save time and reduce the chances of making mistakes. The key is to approach each problem methodically, applying the right techniques at the right moments.
Understand the Problem Thoroughly
Before jumping into calculations, take a moment to fully grasp the details of the problem. Identify all the given information and what is being asked. Breaking down the question into smaller parts can make it easier to determine the steps needed for solving.
Apply the Right Techniques
Use relevant formulas and methods suited to the problem at hand. For example, if the problem involves finding areas or distances, use the appropriate geometric formulas. Using a logical sequence and checking each step as you go ensures that you stay on track and avoid errors.
Test Strategies for Better Accuracy
Improving accuracy during a mathematical evaluation requires more than just knowing the formulas. Developing effective strategies can help you reduce errors and approach each problem with confidence. By planning your approach and staying organized, you increase your chances of achieving precise results.
Effective Strategies to Follow
Here are some proven strategies to enhance accuracy during your practice or evaluation:
- Read each question carefully: Fully understand what is being asked before beginning any calculations.
- Highlight key information: Mark important numbers, relationships, or conditions to stay focused on the necessary details.
- Work step-by-step: Break down each problem into smaller, manageable parts to ensure you’re following the right process.
- Double-check your work: After completing each step, review your calculations to ensure no mistakes have been made.
Time Management Tips
In addition to accuracy, managing time effectively is essential. Here are some tips:
- Prioritize easier questions: Start with the questions you are most comfortable with to build confidence and save time for harder ones.
- Set time limits for each question: Allocate a specific amount of time to each problem to prevent spending too much time on one task.
- Leave difficult questions for later: If a problem is taking too long, move on and return to it after addressing the easier ones.
Breaking Down Complex Questions

When faced with challenging problems, it’s crucial to approach them in a structured way. Rather than feeling overwhelmed, breaking down the question into smaller, manageable parts can make it easier to understand and solve. This method helps to focus on the essential elements and prevents confusion during the problem-solving process.
Steps to Simplify Complex Problems

Follow these steps to effectively break down and tackle difficult questions:
- Identify key information: Extract important numbers, relationships, and conditions provided in the problem.
- Determine what is being asked: Clearly define the objective of the problem so you can focus on finding the right solution.
- Divide the problem: Split the problem into smaller sections or steps. This makes each part easier to address.
- Choose the right method: Based on the problem’s structure, decide which formula or technique to apply to each section.
Techniques for Simplification
There are several techniques that can help make complicated questions more manageable:
- Draw diagrams or sketches: Visualizing the problem can often reveal relationships and provide clarity.
- Use substitution or elimination: When working with multiple variables, simplifying equations can help break down complexity.
- Work backward: In some cases, reversing the order of operations can lead to a quicker solution.
Using Visual Aids for Geometry Problems

In many mathematical challenges, visualizing the problem can significantly simplify the process of finding a solution. Diagrams, charts, and other visual tools help to clarify relationships, angles, and shapes that might otherwise be difficult to understand through text alone. Incorporating these aids into your problem-solving strategy can improve both your speed and accuracy.
Why Visual Aids Matter
Visual aids provide a clear representation of the problem, making it easier to see connections and patterns. Here are some ways they can be helpful:
- Clarifying relationships: Diagrams can show how different elements of the problem are related to one another, which is particularly useful for visualizing angles, lengths, and positions.
- Identifying key information: By sketching the scenario, you can highlight important points, such as given measurements or points of intersection.
- Reducing errors: Seeing the problem visually helps to prevent misinterpretations and mistakes that might occur when relying solely on calculations.
Types of Visual Aids to Use
Different problems benefit from different types of visual tools. Here are some commonly used aids:
- Diagrams: Drawn representations of shapes, lines, and angles can help break down the question into manageable parts.
- Graphs: Plotting coordinates or geometric figures on a graph allows you to work through problems involving distances, slopes, and areas.
- Tables: For problems involving multiple steps or variables, organizing information in a table can make calculations and comparisons easier.
Examining Different Answer Formats
In any evaluation, the way in which a solution is presented is just as important as the steps taken to reach it. Understanding different formats for delivering solutions can ensure that answers are both clear and complete. This section explores various ways of presenting results, from numerical values to explanations, helping you identify the most appropriate format for each situation.
Types of Solution Formats
There are several ways to express solutions, each suited to different types of problems. Here are the most common formats:
- Numerical answers: In many problems, the solution is simply a number, such as a length, area, or volume. It is essential to ensure the result is presented with the correct units of measurement.
- Step-by-step solutions: Some problems require a detailed breakdown of the method used to reach the final result. This format often includes intermediate calculations and explanations.
- Diagrams and visual aids: For some questions, a visual representation is necessary to clarify the answer. This might include labeled sketches or charts that illustrate the relationships between different elements of the problem.
Presenting Complex Solutions
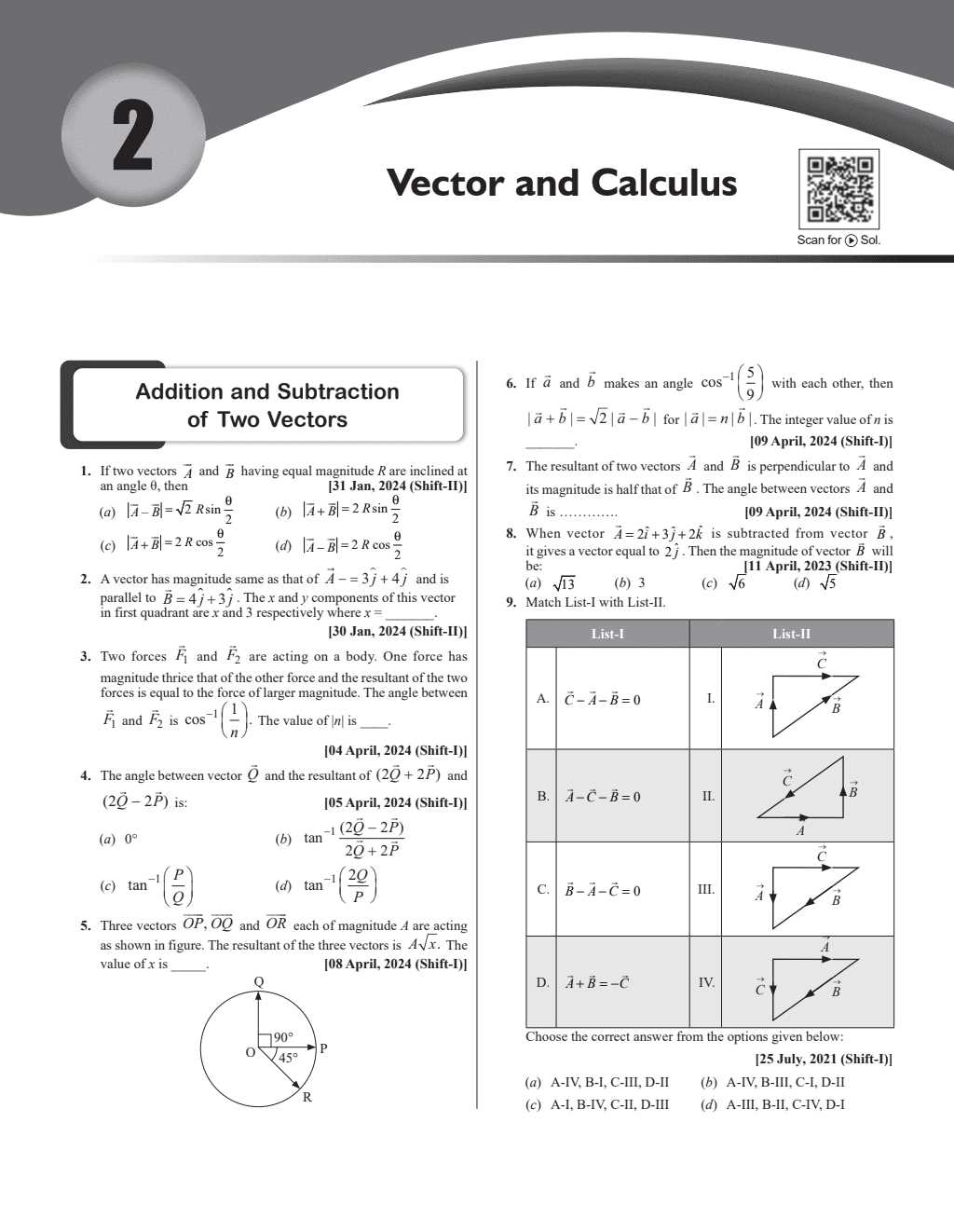
When dealing with more complex problems, solutions may require a combination of formats. In these cases, consider organizing the information clearly for easy understanding. The following table outlines different solution approaches for various problem types:
| Problem Type | Answer Format |
|---|---|
| Measurement of lengths or areas | Numerical answer with units |
| Logical or algebraic equations | Step-by-step written explanation |
| Geometric relationships or angles | Diagram with labeled elements |
Common Geometry Theorems to Know

Understanding fundamental principles is key to solving a wide range of problems. Theorems provide critical insights that simplify complex calculations and help you navigate through various scenarios. This section highlights some of the most essential theorems that form the foundation of many mathematical challenges.
These theorems are not only theoretical but practical tools that can be applied in a variety of contexts. Familiarity with them allows you to approach problems with confidence and precision, as they often serve as shortcuts to finding solutions. Below are some of the most widely used theorems to keep in mind:
- Pythagorean Theorem: This fundamental principle relates the sides of a right triangle, stating that the square of the hypotenuse is equal to the sum of the squares of the other two sides.
- Triangle Sum Theorem: The sum of the interior angles of any triangle always equals 180 degrees.
- Vertical Angles Theorem: When two lines intersect, the opposite angles (vertical angles) are always equal.
- Parallel Line Theorems: When two parallel lines are cut by a transversal, the alternate interior angles are equal, and the corresponding angles are congruent.
- Sum of Interior Angles in a Polygon: The sum of the interior angles of any polygon can be calculated using the formula (n – 2) × 180°, where n is the number of sides.
Understanding Test Instructions Thoroughly

Before diving into any assessment, it’s crucial to fully grasp the guidelines provided. These instructions outline the expectations, helping you navigate the tasks more efficiently and avoid unnecessary mistakes. Whether the questions involve multiple steps or require specific formatting, understanding the rules is the first step toward success.
In any evaluation, instructions are there to guide your approach, providing details on how to handle each section and what is required for each response. Skimming through them quickly may lead to misinterpretations, but taking the time to read carefully ensures you’re on the right track from the start.
By thoroughly understanding the guidelines, you can manage your time better, prioritize certain sections, and approach each question with the proper method. This strategy not only boosts your confidence but also increases your accuracy in completing the tasks.
Reviewing Chapter 5 Solutions
After completing an assessment, reviewing the solutions is a critical step in the learning process. This allows you to understand where mistakes were made and gain insights into areas that need improvement. A detailed review not only helps reinforce correct methods but also clarifies any misunderstandings.
When going through the results, focus on both the correct answers and any errors. Identifying patterns in mistakes can give you valuable clues on how to adjust your approach. For example, if similar errors are repeated in different problems, it may indicate a misunderstanding of a specific concept or technique.
The table below illustrates some example solutions and their corresponding explanations, which you can use as a reference during your review:
| Problem | Correct Solution | Explanation |
|---|---|---|
| Problem 1 | 45° Angle | Used the triangle sum theorem to find the missing angle in a right triangle. |
| Problem 2 | 12 cm | Calculated the length of the missing side using the Pythagorean theorem. |
| Problem 3 | 28° | Identified corresponding angles from parallel lines cut by a transversal. |
By examining each solution carefully, you’ll reinforce your understanding and develop strategies for improving your performance in future tasks.
Mastering Proof-Based Questions
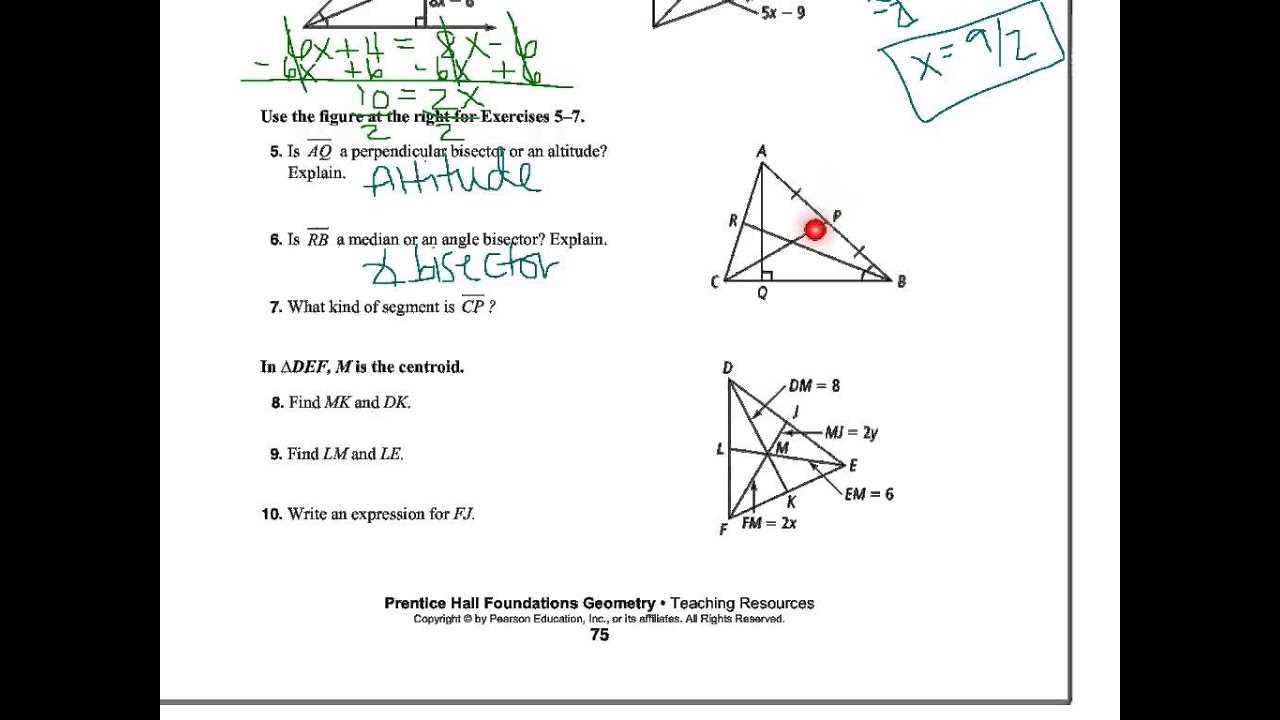
Solving proof-based questions requires a clear understanding of logical reasoning and the ability to demonstrate the relationships between different elements of a problem. These types of questions often test your ability to apply known theorems, axioms, and definitions to establish the truth of a statement. The key is to break down complex situations into smaller, manageable steps and present each step logically to build a solid case.
Understanding the Structure of Proofs
Each proof-based question typically follows a clear structure. Start by identifying what needs to be proven and what information is already provided. Carefully examine the relationships between given figures or statements. Then, determine which postulates, theorems, or properties are most applicable to the problem. From there, apply the reasoning step by step, ensuring that each statement follows logically from the previous one.
Common Approaches to Proofs
There are several common methods used in proofs, including direct proofs, proof by contradiction, and proof by induction. Understanding when and how to use each method will strengthen your ability to tackle a variety of proof-based questions effectively. For instance, when proving a statement by contradiction, you assume the opposite of what you want to prove and show that it leads to a contradiction, thereby proving your original statement is true.
By practicing these techniques and carefully following the logical flow, you can gain mastery over proof-based questions and significantly improve your problem-solving skills.
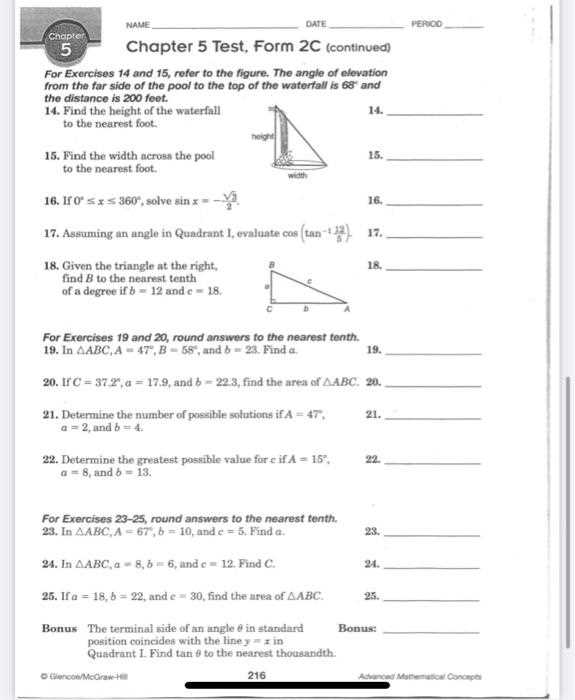
Preparing for Similar Geometry Tests
Success in similar assessments relies on a combination of strategic preparation and solid understanding of key concepts. To excel in these evaluations, it’s essential to focus on both theoretical knowledge and practical application. By identifying recurring topics and mastering the techniques required to solve problems efficiently, you can improve both speed and accuracy during the assessment.
Key Areas to Focus On
When preparing for similar challenges, prioritize areas that commonly appear in the evaluation. Here are some fundamental topics to review:
- Mathematical Properties: Understand essential rules, formulas, and postulates.
- Problem-Solving Techniques: Familiarize yourself with various strategies like deductive reasoning and visualization methods.
- Figure Interpretation: Work on reading and interpreting diagrams, as visual aids often clarify abstract concepts.
- Time Management: Practice solving problems under timed conditions to simulate the actual experience.
Effective Study Techniques
Use the following approaches to enhance your preparation:
- Practice Regularly: Consistent practice is key to building familiarity with question types.
- Work with Sample Problems: Reviewing past assessments can help you understand the structure of the questions and identify common themes.
- Study with a Group: Collaborating with peers allows for different perspectives on solving problems.
By focusing on these critical areas and implementing these study techniques, you can approach similar evaluations with confidence and perform at your best.
Resources for Additional Practice
To strengthen your understanding and improve your problem-solving skills, utilizing various learning tools is essential. Supplementary materials can provide diverse approaches to mastering key concepts, enhancing your ability to tackle similar challenges with ease. By integrating different resources into your study routine, you can refine your techniques and gain deeper insights into complex topics.
Online Platforms and Tools
There are numerous online resources available for extra practice and learning. These platforms offer interactive exercises, video tutorials, and step-by-step problem solutions:
- Khan Academy: Offers detailed lessons and practice exercises on a wide range of mathematical topics.
- Coursera: Provides online courses with interactive assignments and quizzes, perfect for mastering advanced concepts.
- Wolfram Alpha: An online tool for solving mathematical problems, providing detailed explanations and solutions.
Books and Workbooks
Traditional textbooks and workbooks are excellent for comprehensive study. They often include practice questions, examples, and detailed explanations:
- “Problem-Solving Strategies” by Arthur Engel: A great resource for practicing advanced problem-solving techniques.
- “The Art of Problem Solving” Series: Focused on building a solid foundation in critical thinking and complex problem-solving.
- Practice Workbooks: Many publishers offer workbooks specifically designed for additional exercises, which include both basic and challenging questions.
Incorporating these resources into your study routine will provide valuable opportunities for review and practice, ensuring you are fully prepared for any assessment.