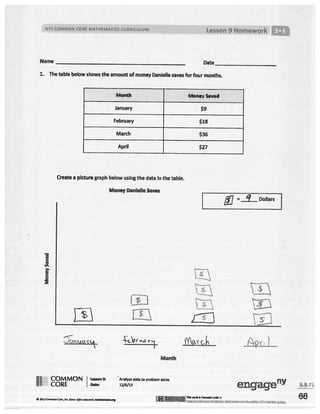
Mathematical exercises often present various problems that require logical thinking and step-by-step approaches. Whether you’re tackling abstract concepts or practical applications, understanding the methods behind each problem is key to finding the right solution. This section will guide you through some of the most common tasks and provide tips for success.
By breaking down the problem into manageable parts, you can apply foundational principles and rules to navigate through complex situations. In this guide, we will focus on the techniques and strategies that lead to clear and accurate results. With a solid grasp of these methods, you can improve your problem-solving efficiency and reduce the chances of making errors.
Understanding the core concepts is essential. Each problem is an opportunity to apply reasoning, practice calculations, and refine your approach. By consistently applying these methods, you will develop the skills to solve more challenging questions with confidence and accuracy.
Mathematical Problem Solving Overview
When faced with complex exercises that challenge your understanding of spatial relationships and logical reasoning, it’s important to approach each task methodically. This section focuses on providing a comprehensive overview of the most effective techniques for tackling various types of problems. Mastering these techniques will help you navigate through each question with clarity and precision.
The key to success lies in recognizing patterns and applying relevant principles to find the most efficient solutions. With practice, you can develop a deeper understanding of how different concepts interconnect, allowing you to solve problems faster and more accurately. Whether you’re dealing with visual puzzles, measurement calculations, or logical deductions, the strategies discussed here are designed to enhance your problem-solving abilities.
Each challenge requires a unique set of steps, but all share a common goal: to apply the right tools at the right time. By honing these strategies, you will build the skills needed to overcome even the most difficult tasks with confidence and ease.
Understanding Problem Types in Mathematical Exercises
Mathematical problems come in a variety of forms, each requiring a different approach to solve. Recognizing the type of problem you’re facing is crucial for applying the right strategies and techniques. In this section, we will explore the most common types of problems encountered in this field, providing you with the knowledge needed to tackle them effectively.
Each problem type may involve different principles, such as spatial reasoning, algebraic manipulation, or logical deductions. Understanding these differences allows you to focus on the most relevant tools and methods for solving each specific challenge. The most common categories include:
- Shape-based problems: These often require understanding the properties of different figures and calculating areas, perimeters, or angles.
- Measurement challenges: Problems that involve distances, volumes, or other measurable quantities.
- Proof-based questions: Tasks that require demonstrating relationships and properties through logical reasoning.
- Transformation and symmetry tasks: Problems where the focus is on changes in shape or position under certain rules.
- Word problems: Real-world scenarios that demand translating textual descriptions into mathematical expressions and solutions.
Each of these categories requires a unique mindset and problem-solving technique. By understanding the distinct nature of these exercises, you can choose the most effective method to approach them, increasing your chances of success and building confidence in your abilities.
Step-by-Step Solutions for Mathematical Problems
Breaking down complex tasks into manageable steps is one of the most effective ways to solve challenging problems. By following a clear, logical progression, you can simplify even the most intricate exercises and find accurate solutions. This section outlines a systematic approach for tackling various mathematical tasks, ensuring that each phase of the process is clear and straightforward.
Organizing the Problem

The first step in any problem-solving process is to carefully read the question and identify what is being asked. This helps to clarify which information is relevant and which is extraneous. Organizing the data into a structured format, such as a list or a diagram, often makes it easier to understand the relationships between different elements.
Applying the Correct Methods
Once the problem is understood, the next step is applying the appropriate methods and formulas. Whether it’s using algebraic equations, geometric principles, or logical reasoning, the key is to use the most efficient strategy for each situation. Break down the process into smaller, manageable actions, checking each step along the way to ensure that calculations and logic are correct.
Verification of Results is an important step that ensures the final solution is accurate. After completing the calculations, revisit the problem and check the results against the given conditions. If the answer makes sense in the context of the problem, you can be confident in your solution.
Common Mistakes in Mathematical Assignments
Even experienced problem-solvers can encounter obstacles when tackling challenging tasks. Often, the simplest errors can lead to incorrect solutions, but with awareness, these mistakes can be avoided. This section highlights the most frequent missteps students make when approaching mathematical exercises and offers tips on how to avoid them.
Misunderstanding the Problem Statement
One of the most common mistakes is misinterpreting the problem itself. Sometimes, key details are overlooked, or the question is not read thoroughly, leading to confusion in later steps. To prevent this, always take a moment to carefully read and re-read the task, ensuring that all conditions and requirements are understood before proceeding.
Incorrect Application of Formulas
Using the wrong formula or misapplying a correct one is another frequent issue. Whether it’s due to forgetting to adjust for units, misunderstanding the variables, or mixing up similar formulas, incorrect application can lead to errors. To avoid this, make sure that you are familiar with the relevant formulas and review them before each step, ensuring that they are correctly applied to the specific problem at hand.
In addition, skipping steps or rushing through calculations can cause simple arithmetic errors. Taking your time to perform each operation carefully can significantly improve accuracy and prevent mistakes from snowballing into bigger problems.
How to Approach Mathematical Exercises
When faced with a new set of problems, having a structured approach can make the difference between confusion and clarity. By following a logical sequence of steps, you can simplify complex tasks and increase your chances of finding the correct solution. This section outlines a strategic method to help you efficiently tackle different types of mathematical exercises.
To begin, it’s essential to break down the problem into manageable parts. Start by understanding the given information and identifying the key elements that will help in solving the task. Here’s a step-by-step guide:
- Read the problem carefully: Ensure that you fully understand the question and the requirements. Take note of any given values and what needs to be determined.
- Draw a diagram: For many tasks, visualizing the situation can help clarify relationships between elements and make the problem easier to handle.
- Identify relevant formulas: Determine which principles or equations apply to the problem at hand. Familiarity with key rules is crucial to solving tasks quickly and accurately.
- Work step-by-step: Break the problem into smaller actions. Perform calculations or logical steps one at a time, checking your work along the way.
- Verify your solution: Once you have a result, review the entire process. Ensure that your answer makes sense in the context of the problem and matches the given conditions.
By following these steps and maintaining a methodical approach, you’ll not only improve your efficiency but also build confidence in your ability to tackle complex mathematical challenges.
Tips for Effective Problem Solving
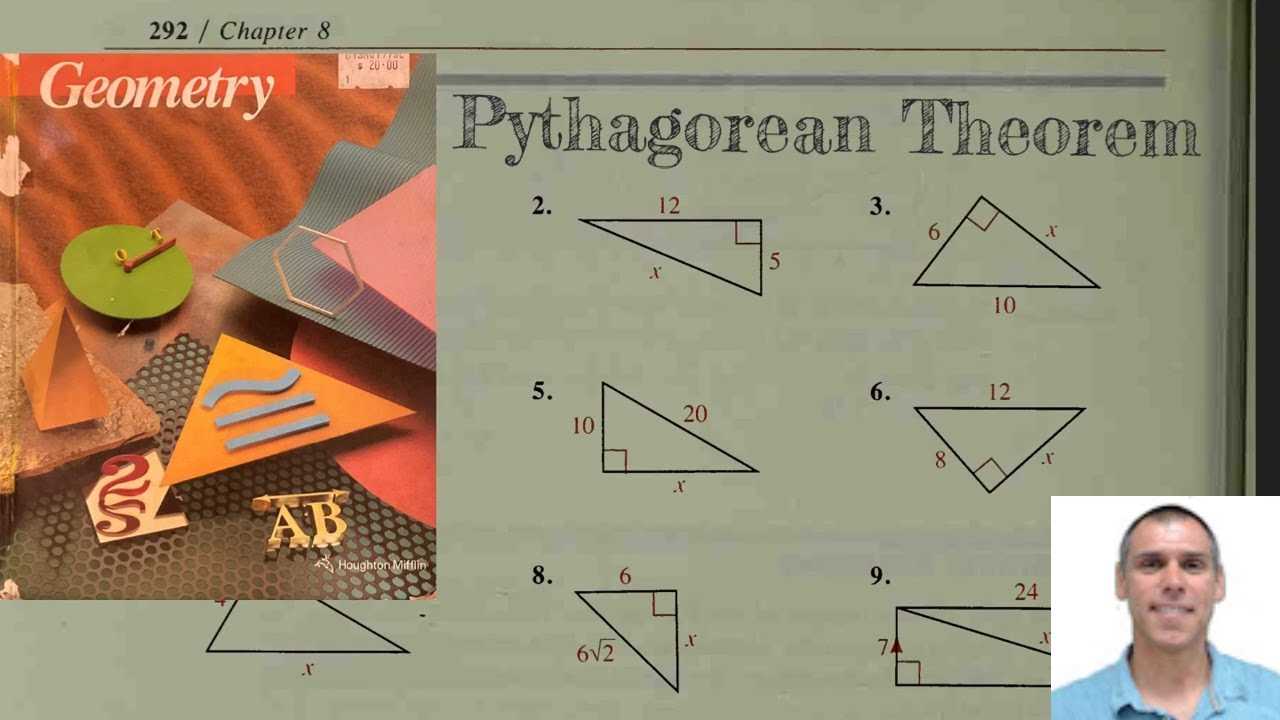
Approaching challenging tasks with the right mindset and strategies can significantly improve your ability to solve problems efficiently. By developing good habits and applying specific techniques, you can minimize errors and enhance your problem-solving skills. In this section, we provide helpful tips for tackling difficult exercises and reaching accurate solutions.
Develop a Logical Strategy
One of the most important factors in solving complex problems is having a clear, logical strategy. By following a structured approach, you can break down the task into smaller, more manageable steps. Start by reviewing the given information, organizing it in a way that makes sense, and identifying the key relationships between the elements involved.
Use Visual Aids
Drawing diagrams, creating charts, or using other visual tools can help clarify abstract concepts and relationships. Visualizing the problem allows you to better understand the spatial elements involved and can often reveal patterns or solutions that may not be immediately apparent.
| Strategy | Benefit |
|---|---|
| Breaking the problem into steps | Reduces complexity and avoids overwhelm |
| Drawing diagrams or sketches | Improves understanding of spatial relationships |
| Checking work after each step | Ensures accuracy and prevents mistakes |
| Revisiting key formulas | Clarifies the correct application of rules |
By combining these strategies and utilizing visual aids when needed, you can approach even the most complex problems with confidence and clarity, improving both your efficiency and accuracy in finding solutions.
Key Formulas for Mathematical Success
Mastering the essential formulas is crucial for tackling a wide range of mathematical problems. These formulas provide the foundational tools needed to solve tasks efficiently and accurately. Understanding when and how to apply each formula can simplify complex exercises and ensure that your solutions are correct. In this section, we will focus on some of the most important formulas that form the basis of many mathematical challenges.
Whether you’re calculating areas, solving for unknowns, or determining relationships between different elements, having these formulas at your fingertips can save time and increase precision. Below are a few key formulas to remember:
- Area of a rectangle: A = l × w (where A is the area, l is the length, and w is the width)
- Area of a triangle: A = ½ × b × h (where A is the area, b is the base, and h is the height)
- Pythagorean theorem: a² + b² = c² (where a and b are the lengths of the legs of a right triangle, and c is the hypotenuse)
- Volume of a cylinder: V = π × r² × h (where V is the volume, r is the radius, and h is the height)
- Perimeter of a circle: P = 2 × π × r (where P is the perimeter and r is the radius)
By regularly reviewing and applying these formulas, you can tackle problems with greater confidence and clarity, making your mathematical exercises more manageable and less overwhelming.
Improving Your Mathematical Skills
Enhancing your problem-solving abilities in mathematics requires dedication and practice. By developing a deeper understanding of concepts, refining techniques, and regularly applying them to different problems, you can significantly boost your skills. In this section, we will explore effective strategies to help you progress and master various mathematical tasks.
To strengthen your ability, consider the following approaches:
- Practice regularly: The more problems you solve, the more comfortable you’ll become with different types of tasks. Consistent practice builds familiarity and boosts your confidence.
- Review key concepts: Ensure that you fully understand the foundational principles. Revisiting core topics and formulas can prevent gaps in your knowledge.
- Work with examples: Studying worked-out examples helps you grasp the process involved in solving similar problems. Try to replicate the steps on your own to reinforce your understanding.
- Seek out challenges: Push yourself by attempting more difficult problems. Overcoming tougher tasks will expand your problem-solving abilities and improve your critical thinking skills.
- Collaborate with others: Working with peers or seeking help from instructors can offer new perspectives and strategies that you might not have considered.
By applying these strategies and maintaining a disciplined approach, you will gradually improve your mathematical capabilities and become more proficient at tackling increasingly complex problems.
How to Check Your Mathematical Solutions
Verifying your work is an essential part of the problem-solving process. Even after finding a solution, it is crucial to ensure that every step has been carried out correctly. By reviewing your methods and results, you can identify potential errors and confirm the accuracy of your calculations. In this section, we will discuss effective ways to check your solutions and avoid common mistakes.
Here are some strategies to ensure your solutions are correct:
- Revisit the problem statement: Before you start verifying, double-check the original question. Ensure you understand exactly what is being asked and that you’ve addressed all requirements.
- Review each step: Go through your solution one step at a time. Make sure that you followed the correct process and applied the right formulas or principles at each stage.
- Check your calculations: Mistakes in arithmetic can often be the cause of incorrect answers. Recalculate key values to confirm their accuracy.
- Look for alternative methods: If possible, try solving the problem using a different approach. This can provide an additional check on your initial solution.
- Estimate your result: Sometimes, estimating the expected range of the answer can help identify obvious errors. If your solution seems too far off from a reasonable expectation, review your steps.
By following these techniques, you can effectively catch mistakes and improve the accuracy of your solutions, ensuring that your results are reliable and well-supported.
Word Problems: Solutions Explained
Word problems are a common challenge in mathematics that require you to interpret a real-world scenario and translate it into a solvable equation or model. These types of problems test your ability to apply abstract concepts to practical situations. In this section, we will explore the step-by-step process for solving such problems and provide clear explanations for each solution.
Step-by-Step Solution Approach
To successfully solve word problems, follow these general steps:
- Read the problem carefully: Ensure that you understand the context and what the problem is asking you to find.
- Identify the known values: Highlight or write down the given information that can be used to solve the problem.
- Choose the appropriate formula or method: Based on the problem type, decide whether you need to use algebraic equations, geometric formulas, or other mathematical tools.
- Set up the equation: Translate the problem into a solvable equation using the known values and the chosen method.
- Solve the equation: Perform the necessary calculations to find the unknown value.
- Verify the solution: Check if your solution makes sense in the context of the problem and if it satisfies the original question.
Example Problem and Solution
Let’s apply these steps to a sample word problem:
Problem: A rectangular park has a length of 120 meters and a width of 80 meters. What is the area of the park?
Step 1: Identify the given information: length = 120 meters, width = 80 meters.
Step 2: The formula for the area of a rectangle is: Area = length × width.
Step 3: Apply the formula: Area = 120 meters × 80 meters = 9600 square meters.
Step 4: The area of the park is 9600 square meters.
| Step | Action | Result |
|---|---|---|
| Step 1 | Identify known values | Length = 120m, Width = 80m |
| Step 2 | Choose appropriate formula | Area = length × width |
| Step 3 | Set up and solve equation | Area = 120 × 80 = 9600 square meters |
| Step 4 | Verify solution | The area is correct and reasonable |
This simple example illustrates the process of breaking down a word problem into manageable steps. By following this approach, you can tackle a variety of real-world scenarios and apply the right mathematical methods to find the solution.
How to Master Geometry Proofs
Mastering proofs is a key skill in mathematical reasoning, and it requires a structured approach to develop logical thinking. Proofs help in demonstrating the truth of statements through a sequence of logical steps. By understanding the principles and strategies involved, anyone can improve their ability to solve and understand proofs. In this section, we will outline the essential steps and techniques for mastering this skill.
Step-by-Step Guide to Proofs
To successfully solve a proof, follow these steps:
- Understand the Given Information: Before jumping into the proof, carefully analyze the problem statement. Highlight the known facts and what needs to be proved.
- Identify the Logical Approach: Choose the appropriate type of proof for the situation. Common methods include direct proofs, proof by contradiction, and proof by induction.
- Break the Problem Into Smaller Steps: Break down the logical steps required to prove the statement. Approach the problem methodically, building from simpler steps to more complex ones.
- Use Definitions, Theorems, and Postulates: Relate the given information to relevant theorems and definitions that can be applied in the proof.
- Write Clear and Concise Justifications: As you write each step, provide the justification for why that step follows from the previous one. Use formal mathematical language and reasoning.
- Review and Revise: After completing the proof, revisit your work to ensure all steps are logically sound and the proof is complete.
Common Proof Techniques
Different types of proofs require different techniques. Here are a few common ones:
- Direct Proof: You assume the hypothesis is true and logically deduce the conclusion.
- Proof by Contradiction: Assume the opposite of what you’re trying to prove, and show that it leads to a contradiction.
- Proof by Induction: This method involves proving the base case and then showing that if the statement is true for an arbitrary case, it holds for the next case as well.
- Proof by Counterexample: This technique involves showing that a given statement is false by providing a counterexample.
By practicing these techniques and following a structured approach, you can develop strong proof skills and gain confidence in tackling even the most challenging problems.
Practice Techniques for Geometry Mastery
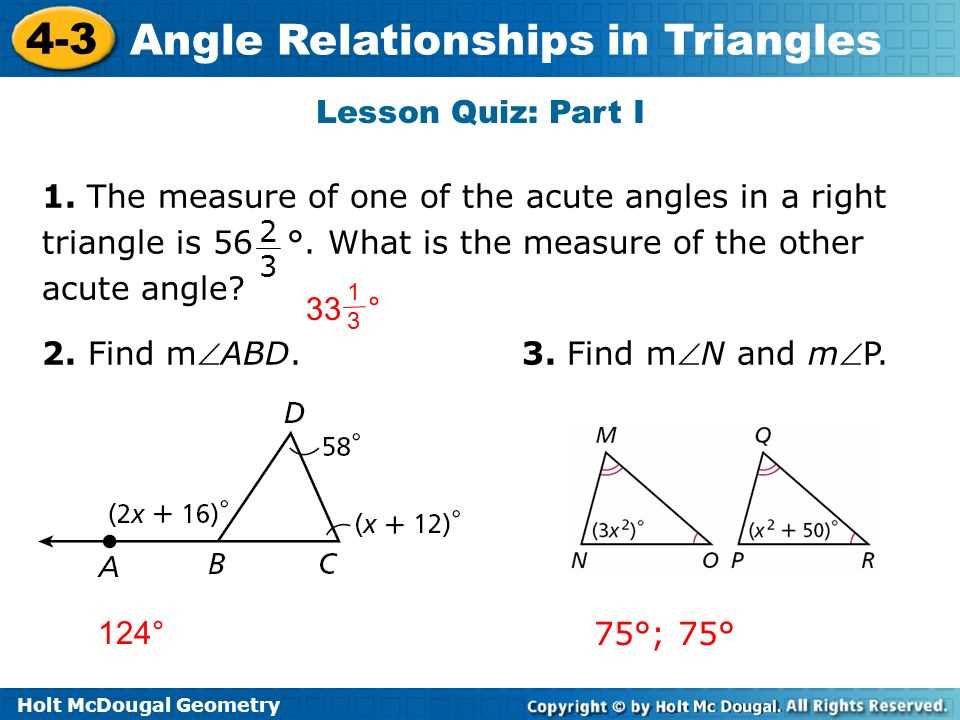
To truly master mathematical concepts, consistent practice is essential. Working through various problems, applying different methods, and reviewing solutions are key components of mastering any topic. In this section, we will explore effective techniques that can help strengthen your skills and build a deeper understanding of complex problems.
Key Techniques for Effective Practice
Use these strategies to enhance your learning experience and improve your problem-solving skills:
- Start with the Basics: Before tackling complex problems, ensure that you have a strong grasp of fundamental concepts. This includes understanding key definitions, theorems, and formulas.
- Work on Varied Problems: Practice with a variety of problems to improve your adaptability. This can include exercises that test different skills, such as calculations, logical reasoning, and visual analysis.
- Time Yourself: Practicing under timed conditions helps improve your speed and accuracy. Set a timer and try to complete each problem within a set period to simulate exam conditions.
- Break Problems into Steps: Break complex problems into smaller, more manageable steps. This can prevent feeling overwhelmed and help ensure that you follow a logical progression in solving the problem.
- Review and Correct Mistakes: After completing each problem, carefully review your solutions. If you made a mistake, figure out why it happened and how to correct it for next time.
- Use Online Tools and Resources: Take advantage of online resources, such as interactive exercises, tutorials, and instructional videos. These tools can provide additional perspectives and help reinforce what you’ve learned.
Tips for Efficient Study Sessions
Maximize the effectiveness of your practice sessions with the following tips:
- Set Clear Goals: Define specific goals for each session, such as mastering a particular concept or solving a set number of problems.
- Practice Regularly: Consistency is key. Set aside time each day or week to practice regularly, ensuring steady improvement over time.
- Take Breaks: Avoid burnout by taking short breaks during study sessions. This helps maintain focus and prevents fatigue.
- Ask for Help When Needed: If you’re stuck on a problem or concept, don’t hesitate to ask for help. This can be from a tutor, peer, or online forum.
By incorporating these practice techniques into your routine, you will steadily improve your skills and confidence, eventually mastering even the most challenging problems.
Geometry Theorems and Their Applications
Mathematical principles, especially theorems, play a vital role in understanding and solving various problems. These fundamental truths are based on proven concepts and form the foundation of logical reasoning in solving complex tasks. In this section, we will explore some of the most important theorems and their practical applications in real-world scenarios.
Key Theorems and Their Importance
Theorems serve as essential tools that allow us to approach problems with a solid foundation of knowledge. Here are a few fundamental theorems and how they are applied:
- Pythagorean Theorem: This theorem describes the relationship between the sides of a right triangle. It is widely used in construction, navigation, and physics to determine distances and angles.
- Triangle Sum Theorem: This states that the sum of the angles in any triangle is always 180 degrees. It is frequently used in both basic and advanced problem-solving, especially in architectural design and surveying.
- Parallel Postulate: Essential in understanding parallel lines and their properties, this theorem is used in everything from map-making to computer graphics, where accurate representations of space are needed.
- Angle Bisector Theorem: This theorem helps in understanding how angle bisectors divide angles into equal parts. It has applications in construction, design, and even fields like engineering where precision is key.
Practical Uses of Theorems
Theorems are not just abstract ideas; they have real-world applications that help us solve practical problems effectively. Here are a few examples of how these principles are put into action:
- Building Design and Architecture: Architects use geometric theorems to design safe and efficient structures. For example, the Pythagorean theorem helps in calculating accurate measurements when constructing buildings and bridges.
- Navigation and Mapping: Theorems are used in geodesy to calculate distances between points on Earth’s surface, helping mapmakers and navigators plot accurate courses.
- Engineering and Manufacturing: In engineering, understanding the properties of shapes and angles helps in creating more precise machines and structures. Theorems like the Triangle Sum Theorem guide engineers in creating safe, stable designs.
By studying and applying these theorems, students and professionals alike can gain a deeper understanding of both abstract mathematical concepts and their practical use in everyday problem-solving.
Time Management in Geometry Assignments
Effective time management is crucial when tackling mathematical tasks that require both logical reasoning and precision. Managing your time well ensures that you can allocate enough attention to each part of the task while avoiding unnecessary stress or errors. In this section, we’ll explore strategies to manage time effectively when working through assignments that require mathematical calculations, proofs, and problem-solving.
Key Strategies for Efficient Time Allocation

To excel in assignments, it’s essential to prioritize tasks and approach them in a structured manner. Below are a few strategies to help manage time while ensuring quality results:
- Prioritize Difficult Problems: Start with the most challenging problems, as these typically take more time. Once they are completed, the rest of the assignment will feel less daunting.
- Set Time Limits for Each Section: Break the task into smaller sections and set specific time limits for each. This will prevent spending too much time on any single problem and ensure consistent progress.
- Review Key Concepts First: Before diving into the problems, review relevant formulas, theorems, and concepts. A quick revision will help improve efficiency and reduce the time spent trying to recall information mid-task.
- Use a Timer: Set a timer to track the time spent on each problem or section. This helps keep you on track and prevents getting lost in a single task for too long.
How to Track Time Effectively
To manage your time successfully, it’s important to track how long you spend on different sections of an assignment. Use the following table to map out your time allocation:
| Task | Time Allotted | Time Spent | Notes |
|---|---|---|---|
| Problem 1 (Challenging) | 15 minutes | 16 minutes | Review formula for accuracy |
| Problem 2 (Moderate) | 10 minutes | 9 minutes | Completed quickly |
| Problem 3 (Easy) | 5 minutes | 6 minutes | Ensure answer is rounded correctly |
| Review and Proofread | 10 minutes | 10 minutes | Check for errors |
By following these time management techniques, students can ensure they stay on track while tackling complex problems and complete their assignments with ease. Time management not only improves efficiency but also reduces anxiety during assignments.
Understanding Geometric Shapes and Properties
Understanding the characteristics and features of various shapes is fundamental to solving many types of problems. Different forms in mathematics have distinct properties that dictate how they interact with other figures and how calculations are performed. This section will explore the essential properties of common shapes and the relationships between their elements.
Each shape has its own unique properties, such as symmetry, angles, and side lengths, which are crucial for identifying and solving related problems. By recognizing and applying these properties, one can approach problems with greater clarity and confidence. Below are some key types of shapes and their defining features:
- Triangles: A three-sided figure characterized by three angles. The sum of the angles always equals 180 degrees. Triangles can be classified into various types such as equilateral, isosceles, and scalene, based on side lengths and angles.
- Quadrilaterals: Four-sided polygons that include squares, rectangles, parallelograms, trapezoids, and rhombuses. Each type has specific properties such as parallel sides, equal angles, and congruent sides.
- Circles: Defined by a set of points equidistant from a central point. Key properties include radius, diameter, and circumference, all of which are used to solve related problems involving distance and area.
- Polygons: Multi-sided figures where the number of sides can vary. Regular polygons have equal-length sides and equal angles, while irregular polygons may not share these properties.
Mastering the properties of these shapes enables a deeper understanding of their applications in problem-solving. By combining knowledge of different shapes, you can tackle more complex problems and find solutions with greater ease.
Common Mistakes to Avoid in Mathematical Problems
In solving mathematical problems, especially those involving shapes and their properties, it’s easy to make mistakes that can lead to incorrect solutions. Many of these errors are common and can be avoided with careful attention and practice. Understanding where mistakes typically occur can help you become more efficient and accurate in your work.
Here are some common errors that often arise when solving mathematical problems and tips on how to avoid them:
Misunderstanding the Problem’s Requirements
One of the most frequent mistakes is misinterpreting the problem. It’s crucial to read the problem carefully and identify exactly what is being asked before starting the solution process. Missing key details such as required measurements or conditions can lead to incorrect results.
Incorrect Application of Formulas
Another common issue is applying the wrong formula or using a formula incorrectly. Many problems require specific formulas for calculating areas, volumes, or angles, and using the wrong one can easily lead to errors. Double-checking the formula and ensuring all terms are correct is vital.
- Confusing Perimeter and Area: Sometimes, the perimeter (the total length around a shape) is mistakenly calculated instead of the area (the space inside a shape), or vice versa. Make sure to distinguish between these two calculations.
- Forgetting Units: Units are often overlooked, especially when calculating areas or volumes. Always include appropriate units like square meters for area or cubic meters for volume to avoid confusion.
Relying Too Much on Visual Estimates
While visualizing a problem can be helpful, relying too heavily on estimates can lead to imprecise answers. It’s important to back up visual assessments with proper calculations and measurements to ensure accuracy.
By staying mindful of these common errors, you can improve both your problem-solving process and the accuracy of your solutions.