In this section, we explore essential principles and problem-solving techniques that will help you succeed in your upcoming assessment. By understanding the core elements and mastering the skills necessary for the tasks at hand, you’ll be able to approach each question with confidence.
Focusing on critical reasoning and practical approaches, we guide you through the most important methods for tackling challenging exercises. Whether you’re revisiting certain areas or enhancing your understanding of complex topics, these insights will give you the tools needed to excel.
Each solution provided is designed to help you not only comprehend the material more thoroughly but also to boost your problem-solving abilities. By reviewing these key points, you’ll strengthen your readiness and improve your overall performance on the exam.
Big Ideas Math Chapter 4 Test Answers

This section offers a detailed breakdown of key questions from the assessment, providing step-by-step solutions for each problem. By working through these examples, you’ll gain a clearer understanding of the concepts and improve your ability to tackle similar challenges in the future.
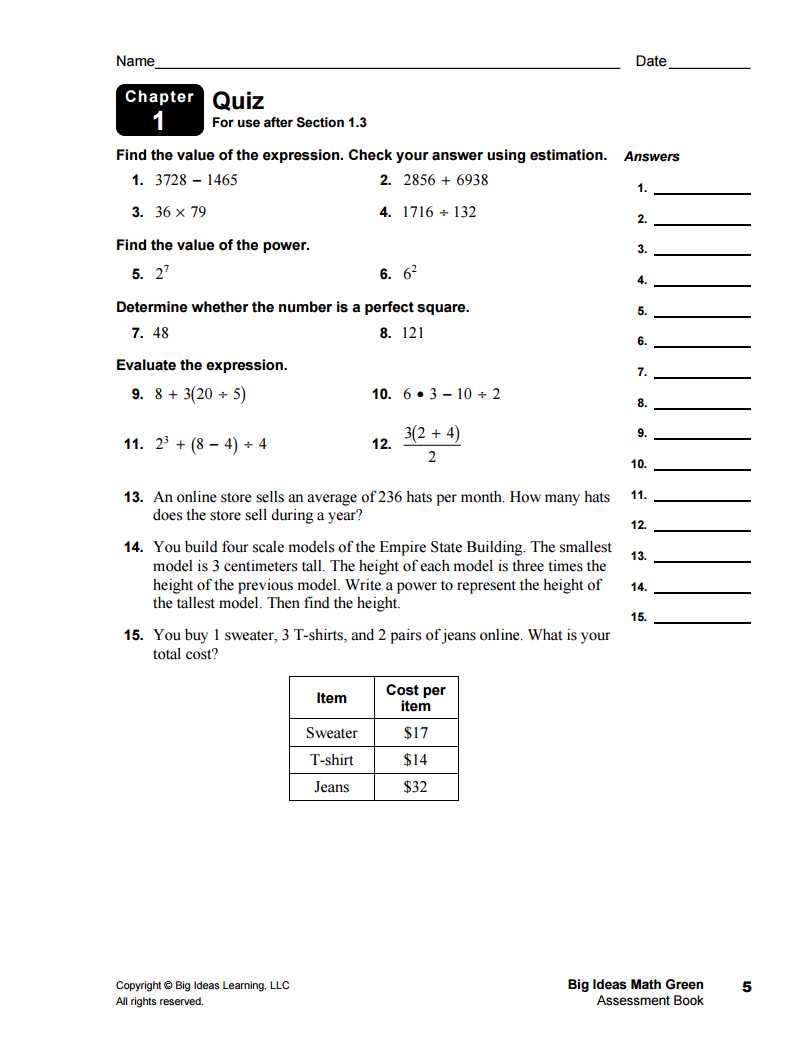
The following table outlines the problems from the section and their respective solutions. These solutions are designed to help you verify your approach and ensure you are on the right track when solving similar exercises.
| Problem | Solution |
|---|---|
| Problem 1: Solving Linear Equations | x = 4 |
| Problem 2: Factoring Quadratic Expressions | (x – 2)(x + 3) |
| Problem 3: Simplifying Rational Expressions | 3/x + 2/x = 5/x |
| Problem 4: Solving Word Problems | Solution: 15 hours |
| Problem 5: Graphing Linear Functions | Slope: 2, y-intercept: -3 |
By reviewing these solutions, you can deepen your comprehension and prepare more effectively for any similar problems on your assessment. Make sure to practice each type of question to build confidence and accuracy in your calculations.
Overview of Chapter 4 Concepts
This section covers essential principles that form the foundation of key topics, focusing on problem-solving strategies and core mathematical skills. It emphasizes understanding relationships between variables, manipulating expressions, and applying logical reasoning to solve various types of exercises effectively.
You’ll encounter concepts related to equations, functions, and algebraic operations, with a focus on simplifying complex problems and enhancing computational accuracy. Mastery of these principles is crucial for approaching more advanced topics in future lessons.
By gaining a thorough grasp of the material, you’ll be better equipped to handle a variety of scenarios and challenges, building a solid foundation for continued success in future assessments.
Key Topics Covered in Chapter 4
This section delves into the essential concepts and methods that serve as the core of the current unit. The focus is on developing skills necessary to solve a range of problems efficiently, from basic operations to more complex equations.
The following key areas are explored:
- Linear Equations: Understanding and solving equations involving variables.
- Graphing Functions: Plotting and interpreting graphs to analyze relationships between variables.
- Factoring: Techniques for simplifying expressions and solving polynomial equations.
- Rational Expressions: Working with fractions involving polynomials and simplifying them.
- Systems of Equations: Methods for solving sets of equations with multiple variables.
Each of these topics builds on foundational skills that are essential for progressing in more advanced mathematical areas. Mastering them will ensure a deeper understanding of key problem-solving techniques used throughout the subject.
How to Approach Test Questions
When facing an assessment, it’s crucial to develop a systematic strategy for tackling each problem. This ensures not only efficiency but also accuracy when solving different types of exercises. A well-planned approach can help you navigate through difficult questions with confidence and minimize errors.
1. Read and Understand the Problem
Start by carefully reading the question and identifying key information. Pay attention to any details that define the problem, such as given values, required operations, and the expected form of the answer. Breaking the problem into smaller, manageable parts often makes it easier to understand and solve.
2. Plan Your Solution Strategy
Once you understand the problem, determine the best approach to solve it. This might involve recalling specific formulas, performing algebraic manipulations, or choosing the right method for simplifying expressions. Planning your steps ahead of time can prevent mistakes and save time during the assessment.
By following this approach, you can improve both the accuracy and efficiency of your problem-solving process. Preparing ahead of time and practicing different techniques will help you tackle any challenge with greater ease.
Step-by-Step Solutions for Practice Problems
In this section, we will break down common problems into clear, understandable steps. Following each solution carefully will help you gain a deeper understanding of the techniques used and how to apply them to similar challenges. Each problem is accompanied by a detailed explanation of the approach, ensuring that you can tackle future exercises with confidence.
Problem 1: Solving Linear Equations
To solve a simple linear equation, follow these steps:
- Identify the equation: Look for the variable and constants.
- Isolate the variable: Use inverse operations to move terms to one side of the equation.
- Simplify: Perform the necessary calculations to solve for the variable.
- Check the solution: Substitute the solution back into the original equation to verify the result.
Problem 2: Factoring Quadratic Expressions
To factor a quadratic expression, follow these steps:
- Identify the quadratic expression: Recognize the standard form ax² + bx + c.
- Find two numbers: Look for two numbers that multiply to give ac and add up to b.
- Rewrite the middle term: Break up the middle term using the two numbers you found.
- Factor by grouping: Group the terms and factor each group separately.
- Factor completely: Factor out the common terms from both groups to get the final factorization.
By practicing these solutions, you will improve your skills and become more efficient at solving similar problems. Following each step methodically ensures that no steps are missed, leading to accurate and reliable results every time.
Common Mistakes in Chapter 4
As you work through problems in this section, it’s important to be aware of common errors that can occur. These mistakes often stem from misunderstanding key concepts or skipping critical steps in the problem-solving process. Recognizing and correcting these errors will help you avoid pitfalls and improve your overall performance.
1. Misinterpreting the Question
One of the most frequent mistakes is failing to fully comprehend the question. Whether it’s missing a key detail or not recognizing the required operations, misinterpreting the problem can lead to incorrect solutions. Always take the time to read the problem carefully before proceeding.
2. Incorrect Application of Formulas
Another common error is applying the wrong formula or using it incorrectly. Be sure to double-check that you’re using the right formula for the specific type of problem you’re solving. Additionally, make sure you’re correctly applying each part of the formula to avoid calculation mistakes.
| Mistake | Example | Correction |
|---|---|---|
| Misinterpreting the problem | Solving for x when the problem asks for y | Read the question thoroughly to ensure you’re solving for the correct variable |
| Forgetting to simplify | Leaving a fraction in unsimplified form | Always simplify your answer, if applicable, to its simplest form |
| Incorrect formula application | Using the wrong equation to solve for area | Review the formula and verify that it matches the given problem |
By understanding these common mistakes and practicing correct techniques, you’ll be better prepared to avoid them in the future and improve your overall problem-solving ability.
Review of Critical Math Skills

This section revisits fundamental skills that are essential for tackling the problems presented in this unit. Mastering these abilities is key to solving more complex exercises with confidence and accuracy. Whether you’re simplifying expressions or solving equations, these skills form the foundation for success.
Key skills include:
- Algebraic Manipulation: Simplifying and solving equations by applying basic algebraic rules.
- Factoring: Breaking down complex expressions into simpler components for easier solving.
- Graphing: Plotting functions on a coordinate plane to visualize relationships between variables.
- Solving Systems of Equations: Using substitution or elimination methods to find solutions to multiple equations with more than one variable.
- Operations with Polynomials: Adding, subtracting, multiplying, and dividing polynomials correctly.
By revisiting and practicing these critical skills, you’ll reinforce your understanding and ensure you’re well-prepared to handle more advanced topics in future lessons.
How to Prepare for the Chapter 4 Test
Preparation for an assessment is crucial for success. To perform well, it’s important to focus on the key concepts and techniques that will be evaluated. A well-structured study plan that includes reviewing core principles, practicing problem-solving, and identifying areas that need improvement can significantly boost your confidence and performance.
Follow these steps to ensure you’re ready:
- Review Key Concepts: Go over the main ideas and formulas covered in the unit. Understanding these core principles will help you approach any question with ease.
- Practice Regularly: Solve practice problems to reinforce your skills. Focus on problems you found difficult in previous exercises and work through them again.
- Identify Weak Areas: Take note of topics that require more attention and dedicate extra time to mastering them. This will ensure you’re prepared for any challenging questions.
- Seek Clarification: If you’re unsure about any concepts, ask questions or consult additional resources to gain a clearer understanding.
- Time Yourself: When practicing, simulate test conditions by timing yourself. This will help you manage your time more effectively during the actual assessment.
By focusing on these strategies, you’ll improve your readiness and approach the assessment with greater confidence and a deeper understanding of the material.
Understanding Key Formulas in Chapter 4
Grasping the essential formulas is vital for solving problems effectively and efficiently. These formulas serve as tools that allow you to break down complex problems into simpler, solvable parts. Understanding when and how to use them is key to achieving accurate results. In this section, we will explore the critical formulas that are central to the unit and show you how to apply them correctly.
Essential Formulas to Remember
Here are some of the key formulas you’ll need to master:
- Quadratic Formula: Used to solve equations of the form ax² + bx + c = 0. The formula is:
x = (-b ± √(b² - 4ac)) / 2a
m = (y₂ - y₁) / (x₂ - x₁)
d = √((x₂ - x₁)² + (y₂ - y₁)²)
A = 1/2 * base * height
How to Use the Formulas Effectively
To ensure success in solving problems, follow these steps:
- Identify the problem type: Determine which formula is appropriate for the given problem.
- Substitute values: Carefully substitute known values into the formula, ensuring accuracy.
- Simplify the expression: Follow the correct order of operations to simplify the expression and find the solution.
- Check your work: Always verify your answer by plugging it back into the original problem to ensure correctness.
By familiarizing yourself with these formulas and practicing their application, you’ll be better equipped to solve problems quickly and accurately during assessments.
Strategies for Solving Word Problems
Word problems can often feel overwhelming, but with the right approach, they become much more manageable. The key to solving these problems lies in breaking them down into simpler steps and understanding what the question is really asking. By using a clear strategy, you can transform a complex problem into something more straightforward.
Here are some strategies to help you solve word problems effectively:
- Read Carefully: Take your time to read the problem thoroughly. Identify the important information and figure out what is being asked. Pay attention to units and quantities that need to be calculated.
- Identify Known and Unknown Values: Highlight or underline the information provided in the problem and separate it from what needs to be determined. This will help you focus on the relevant details.
- Translate the Words into Mathematical Expressions: Convert the words into mathematical symbols or equations. This step often involves recognizing keywords that indicate specific operations, like “sum” for addition or “difference” for subtraction.
- Choose the Right Strategy: Decide which method or formula to apply. For example, if the problem involves finding distance, you might use the distance formula. If it’s about finding an unknown value in a proportional relationship, set up a proportion.
- Perform the Calculations: After setting up the appropriate equation or relationship, solve it step by step. Be careful with your calculations and check them as you go.
- Review Your Answer: Once you’ve arrived at a solution, revisit the problem to ensure it makes sense in the context. Double-check your math and verify that the answer addresses the question asked.
By applying these strategies, you’ll be able to approach word problems with greater confidence and accuracy, turning complex questions into simple solutions.
Tips for Managing Test Time
Effectively managing time during an assessment is essential for maximizing performance. A well-planned approach can help you allocate enough time to each question, ensuring you don’t feel rushed and can complete all sections. With a few strategic techniques, you can handle the pressure and make the most of your time.
Prioritize Questions

Start by scanning through the entire assessment to get an overview of the questions. Identify the ones that you can answer quickly and easily, then tackle them first. This allows you to build momentum and ensures that you don’t miss out on easy points. Save the more complex problems for later when you’ve had time to focus and think clearly.
Set Time Limits for Each Section
Before starting, divide your available time by the number of sections or questions in the assessment. Set a mental or written time limit for each part. Stick to these limits as closely as possible, so you don’t spend too much time on any one section. If you find yourself stuck on a difficult question, move on and come back to it later if needed.
By following these strategies, you’ll be able to manage your time more effectively, reduce stress, and improve your overall performance during an assessment.
Real-Life Applications of Chapter 4
The concepts learned in this section have far-reaching uses beyond the classroom. Understanding and applying these ideas can help solve real-world problems in a variety of fields. Whether it’s for personal finance, engineering, or even daily decision-making, the skills developed here are valuable in practical situations.
Applications in Everyday Life
One of the key ways to apply these concepts is in personal budgeting and managing finances. By understanding proportions, ratios, and calculations, you can make informed decisions about spending, saving, and investing. Here are a few ways these skills come into play:
- Budgeting: Track expenses and allocate funds appropriately by using ratios to compare different categories of spending.
- Shopping: Use percentages to calculate discounts or determine the best deal when comparing prices.
- Investing: Understand compound interest rates and how money grows over time, allowing for smarter investment decisions.
Professional Uses
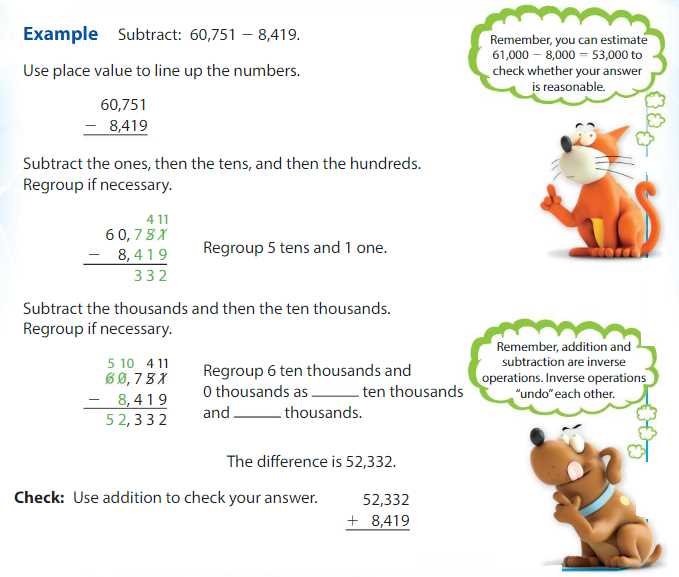
In the professional world, these concepts are widely used in various industries such as construction, technology, and business. Here’s how:
- Construction: Engineers and architects use ratios and proportions to ensure the accuracy of measurements and the stability of structures.
- Technology: Programmers rely on mathematical logic and problem-solving skills to write algorithms and optimize software applications.
- Business: Companies apply mathematical models to predict market trends, optimize production processes, and make data-driven decisions.
By mastering these principles, you can apply them in ways that enhance your personal and professional life, leading to smarter decisions and better outcomes.
How to Check Your Answers
Reviewing your work is a critical step in ensuring accuracy and understanding. By carefully examining your solutions, you can identify any mistakes or overlooked details. This process helps you gain confidence in your responses and improves the quality of your results.
Steps for Effective Review

To check your solutions effectively, follow these key steps:
- Double-check calculations: Revisit each computation, particularly for operations like addition, subtraction, multiplication, and division. Verify each step carefully.
- Review problem understanding: Ensure you have interpreted the problem correctly. Sometimes a misreading can lead to an incorrect solution.
- Check for consistency: Compare your answers with the context of the problem. Do they make sense? Are they realistic given the situation described?
- Verify units and conversions: If the problem involves units, ensure that conversions were done properly and consistently throughout the solution.
Using a Table for Verification
One effective method to verify your work is by using a table to organize your calculations and compare results.
| Step | Original Answer | Verification Check |
|---|---|---|
| Step 1: Initial Calculation | 15 | Recalculate the steps involved |
| Step 2: Final Answer | 30 | Re-check the logic and numbers |
By breaking down your process in this way, you can more easily spot errors and correct them, leading to greater accuracy in your work.
Importance of Accuracy in Math Tests
Precision plays a vital role when solving problems in any subject, but it is especially critical in calculations and logical reasoning. Small errors can lead to incorrect outcomes, even if the overall approach is sound. In academic assessments, ensuring that each step is carried out carefully contributes significantly to achieving the correct results and avoiding penalties for miscalculations.
Why Accuracy Matters
Achieving accuracy in solving problems involves more than just performing operations correctly. It encompasses understanding the problem, selecting the right method, and executing each step with care. Here’s why it is important:
- Confidence: When your solutions are accurate, you can trust in the results and feel more confident about your abilities.
- Minimized mistakes: Fewer errors mean less time spent revising and more time available for completing other tasks.
- Improved efficiency: A focus on accuracy can speed up your work in the long run, as fewer corrections are needed.
How to Maintain Accuracy
To maintain high levels of precision, consider these tips:
- Break down complex problems: Tackle each part of a problem systematically to reduce the chances of overlooking important details.
- Work methodically: Follow a consistent approach when solving problems. A structured process can help prevent careless mistakes.
- Review your work: Always check your solutions to ensure that your calculations and logic align with the problem requirements.
Focusing on precision not only helps in academic tests but also in real-world scenarios, where the correct application of knowledge is essential.
Resources for Additional Practice
To strengthen your understanding and improve your skills, it’s important to practice regularly. Many resources are available that provide a variety of exercises, explanations, and challenges to help reinforce the concepts you are learning. These materials can be accessed online or in print, and they offer the chance to apply theoretical knowledge in practical scenarios.
Online Platforms
Numerous websites and digital platforms offer interactive exercises, quizzes, and practice tests to aid your learning. Here are some valuable online resources:
- Khan Academy: Offers instructional videos, practice exercises, and quizzes for a wide range of topics.
- IXL: Provides personalized practice problems with instant feedback to help solidify understanding.
- Wolfram Alpha: Useful for checking answers and learning step-by-step solutions to complex problems.
- Coursera: Offers courses with detailed explanations and practice exercises in various subjects.
Printable Worksheets and Books
For those who prefer working offline or need additional structured exercises, printed resources can be highly beneficial. Consider the following:
- Workbooks: Many educational publishers provide workbooks filled with exercises that align with key concepts.
- Practice Problem Sets: You can find sets of practice problems in textbooks and supplemental guides that give further opportunities to apply your knowledge.
- Flashcards: Using flashcards can be an effective way to test and reinforce important facts and formulas.
Combining different resources, from online platforms to physical books, can offer a comprehensive approach to mastering the material and improving problem-solving techniques.
What to Do After the Test

Once you’ve completed the assessment, it’s important to reflect on your performance and identify areas for improvement. The time immediately following the completion of the exam is a valuable opportunity to evaluate how well you understood the material and pinpoint any challenges you may have faced. Instead of simply waiting for the results, take proactive steps to ensure continuous progress.
One of the first actions to take is to review your responses. Going over the questions again, even after submission, can help you realize any mistakes you may have made and reinforce your learning. It’s also an opportunity to identify which concepts you found difficult and need further practice.
Additionally, discussing the assessment with classmates or instructors can offer further insight. This interaction often reveals common mistakes and shared misunderstandings, allowing you to approach the material from different perspectives. Engaging in these discussions will not only help clarify any confusion but also deepen your understanding of the subject matter.
Finally, don’t forget to take note of any feedback you receive. If the instructor provides comments or corrections, use them to improve your skills for future evaluations. Developing a growth mindset, where every assessment becomes a learning experience, is essential for long-term success.
Final Review for Chapter 4 Exam
As you approach the final evaluation, it’s crucial to consolidate everything you’ve learned. A comprehensive review of the key concepts will help reinforce your understanding and prepare you for the assessment ahead. Rather than cramming at the last minute, focus on revisiting areas that you found challenging and reviewing your notes and practice exercises.
Start by reviewing the most important formulas, theorems, and problem-solving strategies that were covered. Make sure you understand not only how to apply them but also when and why they are used. This deeper understanding will help you solve complex problems more efficiently and accurately during the evaluation.
Next, take time to solve sample problems similar to those you expect to encounter. This will help you familiarize yourself with the question formats and improve your speed and accuracy. While practicing, focus on the areas where you’ve struggled in the past and make sure to practice those specific types of questions multiple times.
Additionally, it’s helpful to identify any recurring patterns or common mistakes you may have made in previous exercises. Reflect on these errors and create strategies to avoid them in the future. Consider discussing any remaining uncertainties with your instructor or classmates to clarify any doubts before the exam.
Finally, ensure you get plenty of rest the night before and approach the evaluation with confidence. With proper preparation and a focused mindset, you’ll be ready to tackle the challenges that lie ahead.