For students navigating through complex mathematical concepts, having structured and detailed assistance is crucial for mastering the material. In this section, we explore various learning aids designed to enhance understanding and solve problems effectively. These tools help break down challenging content into more manageable parts, providing clear explanations and practical examples.
The approach involves carefully selected exercises and solutions that guide learners through each concept step by step. With a focus on reinforcing fundamental skills and offering additional practice, students are better equipped to tackle progressively difficult problems with confidence. Whether you’re reviewing for exams or seeking to deepen your comprehension, these materials offer invaluable support throughout the learning process.
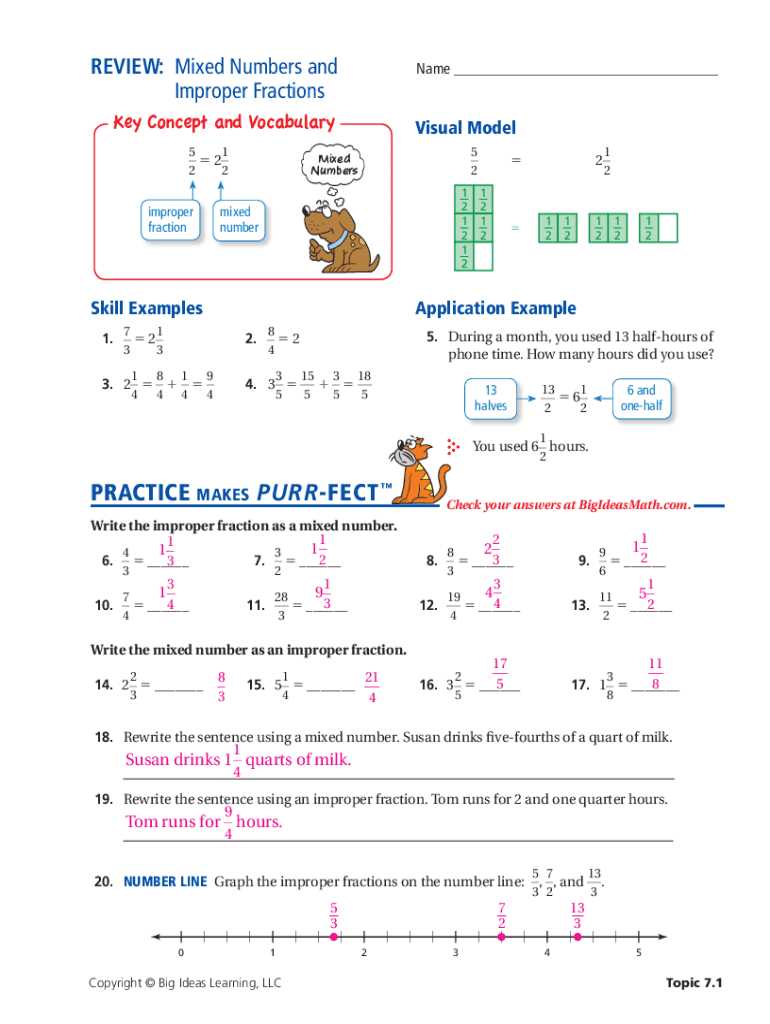
Big Ideas Math Chapter 10 Answer Key
In this section, we provide essential solutions and explanations for the problems covered in the tenth section of the curriculum. These solutions serve as a valuable reference for students looking to verify their understanding and improve their problem-solving techniques. The guide breaks down complex exercises into simpler steps, making it easier to follow and comprehend each solution.
With clear and detailed explanations, learners can use these solutions to identify where they might have made errors and how to correct them. The goal is to foster a deeper understanding of the concepts through practical examples that highlight key problem-solving strategies. Whether you’re working through homework or reviewing for exams, these step-by-step solutions are designed to support your learning progress and enhance retention.
Overview of Big Ideas Math Resources
This section provides a comprehensive look at the various tools and materials designed to support learning throughout the curriculum. These aids are structured to assist students in mastering core concepts and solving problems with greater confidence. They offer step-by-step guidance, ensuring learners can follow the logical progression of each topic and refine their understanding.
Types of Learning Materials Available
The learning materials are divided into different categories, each focused on specific aspects of the subject. These include exercises, practice tests, and visual aids to enhance comprehension. Below is an overview of the most commonly used resources:
| Material Type | Description |
|---|---|
| Practice Problems | Helps reinforce the core concepts with targeted exercises. |
| Step-by-Step Solutions | Guides students through complex problems, explaining each step. |
| Visual Aids | Offers diagrams and illustrations to simplify abstract ideas. |
| Review Quizzes | Provides practice tests to assess understanding and retention. |
Benefits of Using These Learning Aids
These materials provide students with the necessary support to excel, whether they’re tackling difficult problems, reviewing previous topics, or preparing for assessments. With well-structured explanations and ample practice, learners can develop a strong foundation and tackle challenges with greater ease.
How to Use Chapter 10 Resources Effectively

To make the most of the available study aids, it’s essential to approach them with a clear strategy. Properly utilizing these materials ensures that you not only understand the content but also apply it confidently to solve problems. The key is to engage with each resource actively and to focus on reinforcing your weak areas.
Start by reviewing the topics you find most challenging. Use exercises to test your understanding and identify areas where further clarification is needed. When working through examples, take your time to follow the step-by-step solutions carefully. This will help you grasp the methodology behind each problem-solving process.
Prioritize Practice: Consistent practice is crucial. Regularly solving problems helps solidify the concepts you’ve learned and builds confidence. Don’t skip exercises, even if they seem repetitive–repetition fosters mastery.
Track Progress: Keep track of your performance and focus on areas that need improvement. If you encounter persistent challenges, revisit the relevant sections and review the related materials until you feel comfortable.
Stay Organized: Use the resources in an organized manner. Break your study sessions into manageable blocks and use a variety of tools to reinforce learning. This structured approach will ensure you absorb the material effectively.
Step-by-Step Guide for Solving Chapter 10

Mastering complex problems requires a systematic approach, and a step-by-step guide can make all the difference in breaking down difficult tasks into manageable sections. This method not only helps you understand the material better but also builds the confidence needed to tackle more advanced exercises. By following each stage carefully, you will enhance your problem-solving skills and ensure that you grasp all key concepts.
Understanding the Problem
The first step is to fully understand what the problem is asking. Carefully read through each question, identifying important information and key requirements. Look for keywords that indicate the method or formula you might need to use, and make sure you know what the final answer should look like.
Breaking Down the Steps
Organize Your Approach: Once you have a clear understanding of the problem, break it down into smaller, more manageable steps. Start by identifying what you know and what you need to find out. Use any relevant formulas or techniques you’ve learned in previous sections to guide your next steps.
Apply the Method: After identifying the steps, apply the appropriate methods or calculations to solve the problem. Take your time with each stage and double-check your work as you proceed. If needed, refer to previous examples for clarification and to reinforce your understanding.
Review Your Solution: After solving the problem, take a moment to review your solution. Ensure that it makes sense and that you have answered all parts of the question. Double-check your work for any calculation errors, and make sure that your final result matches the expected outcome.
Key Concepts in Chapter 10 Explained
In this section, we dive into the essential ideas and principles that form the foundation of the material covered in the tenth part of the curriculum. Understanding these core concepts is crucial for solving the exercises and applying them to more advanced topics. Below, we explain the key elements and provide examples that clarify their application in problem-solving.
| Concept | Description |
|---|---|
| Linear Equations | Equations that represent straight lines on a graph, involving variables raised to the first power. |
| Systems of Equations | Two or more equations that share common variables, often solved simultaneously to find the variable values. |
| Proportional Relationships | Situations where two quantities increase or decrease at the same rate, maintaining a constant ratio. |
| Solving Inequalities | Finding the range of values that satisfy an inequality, often involving greater than, less than, or equal to symbols. |
Each concept is linked by a set of methods and techniques, which allows for a deeper understanding of their applications in real-world scenarios. By mastering these ideas, students are better prepared to approach more complex topics with confidence and precision.
Helpful Tips for Understanding Chapter 10
Grasping complex concepts requires a combination of strategies and consistent practice. To excel in the material covered, it’s essential to break down difficult topics into smaller, more manageable pieces. Below are some practical tips that can help you better understand the key ideas and improve your problem-solving skills.
- Focus on Understanding, Not Memorization: Aim to fully understand the reasoning behind each solution rather than simply memorizing formulas. This will allow you to apply concepts in a variety of situations.
- Use Visual Aids: Diagrams and graphs can help illustrate abstract concepts. Visualizing problems can make them easier to understand and solve.
- Practice Regularly: Solving a wide range of problems helps reinforce the material. Even if a topic seems challenging at first, regular practice will build confidence and improve your skills.
- Work with Examples: Start by reviewing solved examples. These provide a step-by-step guide that can make the process clearer and easier to follow.
- Don’t Skip the Difficult Parts: If you struggle with a particular section, don’t move on until you’ve grasped the concept. Revisiting the material from different angles can help clarify misunderstandings.
- Ask for Help When Needed: If you find certain problems confusing, reach out for help. Whether from a teacher, tutor, or peer, additional explanations can often provide valuable insights.
By following these tips, you’ll be able to improve your understanding and approach problems more confidently. Remember, consistent practice and a clear understanding of each concept are the keys to success in mastering the material.
Accessing the Big Ideas Math Answer Key
To effectively use the solutions for the exercises, it’s important to know how to access the relevant materials. Having the correct tools at your disposal ensures that you can verify your results and gain a deeper understanding of the methods used to solve problems. This section provides information on how to obtain and use these materials to support your learning journey.
Where to Find the Solutions
Solutions can typically be accessed through online platforms or provided physical copies, depending on your curriculum’s format. Many educational websites offer access to these materials for students enrolled in specific programs. In some cases, teachers may also distribute the solutions directly to students.
Using the Solutions Effectively
While it’s useful to refer to the solutions when you’re unsure about your progress, it’s important to approach them with care. Instead of simply looking up answers, use the provided explanations to understand the reasoning behind each step. This will help you learn the problem-solving techniques, making it easier to apply them to new problems in the future.
Common Mistakes in Chapter 10 and Solutions
As students progress through complex problems, certain errors tend to arise frequently. Recognizing these mistakes early on allows for quick corrections and ensures a deeper understanding of the concepts. Below, we highlight some of the most common issues encountered in this section, along with practical solutions to help avoid them.
Misinterpreting the Problem: One of the most common mistakes is misreading the problem or missing key information. This often leads to applying the wrong methods or formulas. To avoid this, always take time to carefully read each problem and underline the important details. Rephrase the question in your own words to ensure you fully understand what is being asked.
Skipping Steps: Many students try to jump straight to the solution without working through all the necessary steps. This can lead to errors in calculations or missed concepts. Always follow a structured approach, breaking the problem down into smaller parts. Even if you’re confident, double-check your work to ensure each step is covered.
Incorrectly Applying Formulas: Using formulas without fully understanding when and how to apply them is a common issue. Take time to review each formula’s derivation and the conditions under which it should be used. Practice with different examples to reinforce your understanding.
Calculation Errors: Simple arithmetic mistakes can derail an entire solution. Always double-check your calculations, especially when dealing with fractions, decimals, or large numbers. Use a calculator when necessary, but be mindful of the steps you take to avoid simple errors.
Overlooking Units: Forgetting to include or properly convert units can lead to incorrect results. Be mindful of units throughout the problem and ensure consistency in your answers. If applicable, convert all units to a standard before proceeding with calculations.
Understanding Chapter 10 Problem Types
In this section, we will explore the various types of problems typically encountered in the tenth part of the curriculum. By familiarizing yourself with the different problem types, you can approach each one with a tailored strategy, improving both your efficiency and accuracy. Recognizing the structure of the problems will allow you to identify patterns and apply the most effective methods to solve them.
Word Problems: These problems present real-world scenarios that require you to translate the given information into mathematical expressions. The challenge lies in understanding the context and determining the appropriate operations needed to reach the solution. Break the problem into smaller parts, identify known and unknown variables, and carefully construct equations based on the information provided.
Equations and Inequalities: These problems focus on solving for unknown values. They may involve linear equations or more complex systems, requiring you to isolate variables or use substitution and elimination methods. Pay close attention to the signs and operations involved, as even small mistakes in manipulation can lead to incorrect answers.
Graphing Problems: Some problems may ask you to plot data or functions on a graph. Understanding the relationship between variables and how to represent them visually is key. Review the principles of plotting points, drawing lines, and interpreting graphs to ensure accurate results.
Measurement and Proportions: These problems often deal with real-world quantities such as distance, volume, or rate, and require the use of proportional relationships or formulas. Be sure to convert units correctly and apply the right proportional methods to solve for the unknowns.
Data Interpretation: Problems may present data sets in the form of tables or charts, and you may be required to analyze the data to identify trends or solve for specific values. Develop a systematic approach to reading and interpreting data, paying close attention to key details such as averages, percentages, and relationships between variables.
What You Need for Chapter 10 Success
Achieving success in this section requires more than just hard work. To truly master the material and perform well, you’ll need a combination of essential tools, strategies, and a positive mindset. By preparing adequately and approaching each problem with a clear plan, you’ll set yourself up for success.
Essential Tools for Success
Having the right tools can make a significant difference in your learning experience. Here are the key resources you’ll need:
- Notebook or Practice Sheet: Keeping detailed notes and practicing problems regularly is essential for reinforcing concepts.
- Calculator: While it’s important to understand the math behind the problems, a calculator can help speed up calculations and prevent simple errors in arithmetic.
- Reference Materials: Having access to study guides, textbooks, or online tutorials can provide additional explanations and examples for better understanding.
- Graph Paper: For problems that involve plotting data or graphs, graph paper can help maintain accuracy and clarity in visual representation.
Effective Strategies for Mastery
In addition to the right tools, applying the right approach is crucial. Here are some strategies to help you succeed:
- Practice Regularly: Consistent practice helps solidify your understanding and builds confidence in solving different types of problems.
- Break Down Complex Problems: When faced with a challenging problem, break it into smaller, manageable steps to avoid feeling overwhelmed.
- Review Mistakes: Learning from errors is just as important as mastering new concepts. Take time to understand where you went wrong and adjust your approach accordingly.
- Stay Organized: Keeping your work and notes organized will help you quickly locate solutions and stay focused on the task at hand.
By combining the right tools with effective strategies, you will be better equipped to tackle the material and excel in this section. Stay committed, stay organized, and keep practicing for the best results.
Mastering Algebra in Chapter 10
Algebra can seem challenging at first, but with the right techniques and a bit of practice, you can easily master the concepts covered in this section. The key to success lies in breaking down complex expressions, understanding the relationships between variables, and applying systematic methods to solve equations and inequalities. In this section, we will focus on strategies and tips that will help you gain confidence and improve your skills in algebra.
Key Concepts in Algebra
Before diving into the problems, it’s important to review the fundamental principles that form the basis of algebra:
- Solving Linear Equations: The core of algebra involves finding the value of unknowns in equations. Understanding how to isolate variables and simplify equations is essential.
- Working with Inequalities: Inequalities often appear alongside equations. Learning to solve them requires understanding the direction of the inequality sign and how to apply it during operations.
- Factoring Expressions: Factoring is an important skill in simplifying algebraic expressions and solving quadratic equations. By factoring, you break down complex expressions into simpler components.
- Exponents and Polynomials: Knowing how to work with powers and expand polynomials is a crucial algebraic skill that comes up frequently in higher-level problems.
Tips for Success in Algebra
To become proficient in algebra, it’s important to develop a strategy for tackling problems. Here are some effective tips for mastering the material:
- Start with the Basics: Don’t rush through the fundamentals. Take time to review basic algebraic rules and operations, such as addition, subtraction, multiplication, and division with variables.
- Practice Different Problem Types: Algebra comes in many forms, from simple linear equations to more complex expressions. Try solving a variety of problems to build a well-rounded skill set.
- Work Step-by-Step: Always solve algebraic problems in a methodical, step-by-step manner. Don’t skip steps, as each one builds on the next and ensures accuracy.
- Check Your Work: After solving a problem, go back and check your steps. Look for errors in calculations or logic, especially when dealing with signs and variables.
- Seek Help When Needed: If you’re struggling with a specific concept, don’t hesitate to ask for help. Whether from a teacher, tutor, or online resources, extra guidance can make a big difference in your understanding.
By practicing these strategies and applying them to various algebraic problems, you’ll soon gain mastery over the concepts in this section and build a strong foundation for future mathematical challenges.
Geometry Concepts in Chapter 10
In this section, we explore essential geometry principles that are foundational for understanding spatial relationships and solving geometric problems. Geometry is not just about shapes; it’s about recognizing patterns, measuring dimensions, and applying logical reasoning to figure out how various elements interact. Understanding the key concepts covered will help you build a strong base for tackling more complex geometric problems in future lessons.
Core Geometry Principles
There are several fundamental concepts in geometry that students need to master in order to solve problems effectively. These include:
- Angles and Their Properties: Angles form the basis of geometric reasoning. Whether acute, obtuse, or right, understanding how angles relate to each other is essential for solving many types of problems.
- Triangles and Their Theorems: Triangles are among the simplest and most important shapes in geometry. Mastering concepts like the Pythagorean theorem, triangle inequalities, and congruence is crucial for solving geometric problems.
- Perimeter and Area Calculations: Understanding how to calculate the perimeter and area of different shapes is essential for solving real-world problems, from construction to land measurement.
- Circles and Circumference: The study of circles involves understanding the relationship between radius, diameter, circumference, and area. These concepts are vital in both theoretical and applied geometry.
- Volume and Surface Area: Understanding how to calculate the volume and surface area of three-dimensional shapes like cubes, spheres, and pyramids is key for solving spatial problems.
Tips for Success in Geometry
Geometry can seem daunting at first, but with a solid strategy, it becomes much more manageable. Below are some tips to help you navigate geometric concepts successfully:
- Visualize the Problem: Many geometry problems require you to visualize shapes and their relationships. Drawing diagrams can help make abstract concepts more tangible and easier to work with.
- Familiarize Yourself with Theorems: Geometry relies heavily on theorems and postulates. Make sure to understand key theorems like the Pythagorean theorem, properties of similar triangles, and angle relationships.
- Practice with Real-Life Examples: Geometry is all around us, from architecture to nature. Try applying geometric concepts to real-life scenarios to make the material more relatable and interesting.
- Work Through Multiple Problems: The more problems you solve, the more confident you will become in applying geometric concepts. Practice a variety of problems to strengthen your skills.
- Ask for Clarification: If you’re struggling with a specific concept or problem, don’t hesitate to ask for clarification. Geometry can be challenging, but seeking help can guide you through difficult topics.
By understanding these fundamental geometric concepts and following these tips, you will be well on your way to mastering the material in this section and preparing for more advanced geometric challenges in the future.
Exploring Word Problems in Chapter 10
Word problems are an essential part of understanding mathematical concepts in real-world contexts. They often require translating a written description into mathematical expressions or equations. In this section, we will explore different strategies for approaching word problems, including how to break down the information and identify the key elements needed to solve them. By practicing these strategies, you can improve your problem-solving skills and gain a deeper understanding of how to apply mathematical concepts in everyday situations.
Key Strategies for Solving Word Problems
To tackle word problems effectively, it’s important to follow a structured approach. The following steps can guide you in solving various types of problems:
- Read the Problem Carefully: Begin by reading the problem multiple times to ensure that you fully understand the context and what is being asked. Pay attention to key details such as numbers, units, and relationships between different elements.
- Identify the Question: Determine exactly what the problem is asking you to find or solve. This will help you focus on the relevant information and avoid unnecessary steps.
- Translate Words into Mathematical Expressions: Convert the information given in the problem into a mathematical form. This could involve setting up equations, identifying variables, or choosing appropriate operations.
- Organize Your Work: Show your work step-by-step, making sure to clearly label any variables or calculations. This will help you stay organized and make it easier to identify errors if they arise.
- Check Your Answer: Once you’ve arrived at a solution, review the problem and your work to ensure that the answer makes sense in the context of the question.
Examples of Word Problems and Solutions
Here are a few examples of common word problems and how to approach solving them:
| Problem | Solution Approach |
|---|---|
| A car travels 60 miles per hour. How long will it take to travel 180 miles? | Divide the total distance by the speed to find the time: 180 ÷ 60 = 3 hours. |
| A store sells pens for $2 each. How much would 15 pens cost? | Multiply the cost per pen by the number of pens: 2 × 15 = $30. |
| If a rectangle has a length of 8 meters and a width of 5 meters, what is its area? | Use the area formula for a rectangle (length × width): 8 × 5 = 40 square meters. |
By following these strategies and practicing with various examples, you will become more confident in solving word problems and applying your mathematical knowledge in real-world scenarios.
Practicing with Sample Problems and Answers
Practicing with sample problems is one of the most effective ways to build confidence and improve your problem-solving skills. By working through examples, you can better understand how to apply the concepts learned in your studies. In this section, we’ll explore how to tackle a variety of problems and the importance of reviewing both the solutions and the steps taken to solve them.
Sample problems offer a valuable opportunity to reinforce your understanding of mathematical principles. Whether you are revisiting basic concepts or delving into more complex material, consistent practice with problems will allow you to become more proficient and comfortable with the material.
Key Steps for Solving Sample Problems
To make the most of practicing with sample problems, follow these important steps:
- Understand the Problem: Begin by reading the problem carefully and identifying what is being asked. Pay attention to any details or constraints provided within the problem.
- Identify Relevant Concepts: Determine which concepts or formulas are necessary to solve the problem. This will help you focus your approach and select the correct method.
- Work Through the Problem Step-by-Step: Break the problem down into smaller, manageable steps. Write down each step clearly and perform calculations methodically.
- Check Your Work: After completing the problem, review your solution to ensure that it makes sense and matches the conditions set in the problem. Double-check any calculations for accuracy.
- Analyze the Solution: Reflect on the approach used to solve the problem and see if there are any other methods that could have been applied. This will help you deepen your understanding.
Sample Problem and Solution
Let’s take a look at an example problem to illustrate the process:
- Problem: A bag contains 3 red marbles, 5 blue marbles, and 2 green marbles. What is the probability of drawing a blue marble?
- Solution: To solve this problem, we first calculate the total number of marbles in the bag: 3 + 5 + 2 = 10. The probability of drawing a blue marble is the ratio of blue marbles to the total number of marbles: 5/10 = 1/2.
By working through a variety of sample problems like this one, you will gain the experience necessary to solve similar questions with confidence and ease.
Strategies for Completing Chapter 10 Exercises
Successfully completing exercises requires not only understanding the concepts but also adopting effective strategies to approach problems efficiently. By using the right techniques, you can break down complex questions into manageable steps and ensure accuracy in your solutions. In this section, we’ll discuss various strategies that will help you approach the exercises with confidence and improve your problem-solving abilities.
Mastering the techniques for working through exercises can greatly enhance your learning experience. These strategies are designed to help you stay organized, focus on essential steps, and minimize errors as you work through the material. By practicing these strategies, you will increase both your efficiency and your comprehension of the subject.
Effective Strategies for Success
Here are several strategies that will help you complete exercises more effectively:
- Preview the Problems: Before diving into the exercises, quickly scan through the questions. This will help you understand the general structure and identify any challenging problems that may require more time or focus.
- Break Problems Into Smaller Parts: If a problem seems overwhelming, break it down into smaller, more manageable parts. Solve each section individually before putting everything together for the final solution.
- Use Process of Elimination: For multiple-choice questions, eliminate obviously incorrect answers first. This will increase your chances of selecting the correct option by narrowing down your choices.
- Stay Organized: Write out each step clearly as you solve a problem. Keeping your work neat and organized will reduce mistakes and make it easier to spot errors later on.
- Use Practice Problems: Before attempting the exercises in the book, practice similar problems to familiarize yourself with the techniques. This can help you build confidence and make you more comfortable when tackling new problems.
- Review the Instructions Carefully: Always read the problem and instructions thoroughly before starting. Missing important details or misinterpreting the problem can lead to unnecessary errors.
Time Management Tips
Managing your time effectively is essential for completing exercises in a timely manner:
- Set Time Limits: Allocate a specific amount of time for each problem or section of the exercise. This will help you stay focused and avoid spending too much time on any single question.
- Prioritize Easier Problems: Start with the problems that you find easiest. This will build momentum and give you more time for more difficult problems.
- Take Breaks When Needed: If you’re feeling stuck or overwhelmed, take a short break. Sometimes a few minutes of rest can help you come back with a fresh perspective.
By implementing these strategies, you will improve your approach to completing exercises, ultimately leading to better results and a deeper understanding of the material.
Using Online Resources for Chapter 10 Help
In today’s digital age, online platforms provide an abundance of tools and materials that can enhance your learning experience. These resources offer valuable support for tackling complex problems, reviewing concepts, and getting step-by-step guidance when you need it most. By utilizing the right online tools, you can strengthen your understanding of the material and gain a clearer perspective on the topics you are working through.
Whether you need instructional videos, practice exercises, or interactive solutions, online platforms are designed to help you reinforce your learning and address areas where you might need extra support. In this section, we will explore various online resources that can assist you in mastering the content and improving your problem-solving skills.
Types of Online Help
Here are some valuable types of online help you can use to enhance your learning:
- Instructional Videos: Websites like YouTube and educational platforms offer a wide range of tutorial videos. These videos break down difficult concepts into manageable steps, providing visual explanations that can make abstract ideas more accessible.
- Interactive Problem Solvers: Websites with interactive problem solvers let you input your own data and step through solutions. This can help you visualize the process and understand how to approach similar problems in the future.
- Online Practice Exercises: Platforms with practice problems offer instant feedback and explanations for each solution. They allow you to test your understanding and build confidence by solving problems in a structured format.
- Online Tutoring Services: Many websites provide live tutoring sessions with experienced instructors. These services allow you to ask specific questions and receive personalized guidance tailored to your needs.
How to Make the Most of Online Resources
To maximize the benefits of online resources, it’s important to follow a few strategies:
- Stay Organized: Keep track of the websites and platforms you use. Make sure to organize the resources in a way that allows you to quickly access relevant information when needed.
- Practice Regularly: Consistent practice is essential for improvement. Regularly use online exercises to reinforce what you’ve learned and identify areas for further study.
- Use Multiple Sources: Don’t rely on just one resource. By exploring a variety of websites and platforms, you can gain different perspectives and find the methods that work best for you.
- Ask for Help When Needed: If a resource doesn’t provide clear answers or explanations, seek out additional help. Many online platforms also offer forums or communities where you can ask questions and get advice from peers or instructors.
By using these online resources strategically, you can supplement your learning and strengthen your ability to solve problems effectively.
Why Chapter 10 is Important in Math
Understanding the concepts introduced in this particular section of your course is crucial for developing a strong foundation in mathematics. The material covered is not only vital for mastering current topics but also serves as the basis for future studies in more advanced areas. Grasping these concepts helps build critical thinking skills that are essential for problem-solving and applying mathematical methods to real-world situations.
Whether you’re solving equations, working with geometric shapes, or tackling abstract concepts, the knowledge gained here will empower you to approach complex problems with confidence and clarity. As you move forward, the lessons from this section will provide essential tools that are needed in other areas of study, making this part of the curriculum particularly important.
Real-World Applications
Mathematical principles from this section are widely applicable beyond the classroom. They are used in fields such as engineering, economics, architecture, and computer science. By mastering these concepts, you’ll be able to tackle practical challenges in various industries, demonstrating how math can be a powerful tool in real-life problem-solving.
Foundation for Future Topics
The ideas explored here are foundational for more advanced mathematical studies. Without a solid understanding of the key topics in this section, it would be difficult to progress to higher-level concepts. Each new mathematical principle often builds on those learned previously, making it essential to understand this material thoroughly before moving forward.
How to Prepare for Chapter 10 Assessments
Preparing for assessments is essential to achieving success in this section. By focusing on understanding the core concepts and practicing the necessary skills, you’ll be equipped to perform at your best. Effective preparation involves reviewing key material, practicing problem-solving techniques, and identifying any areas of weakness that need additional attention. The more thoroughly you prepare, the more confident you’ll feel when it comes time for the assessment.
Review Key Concepts and Methods
Start by reviewing the essential concepts and methods covered in this section. Pay attention to the types of problems you’ve worked on in exercises and practice questions. Ensure that you fully understand how to approach each problem type and the steps required to solve them. Revising the explanations and examples will solidify your understanding.
Practice with Sample Problems
One of the most effective ways to prepare is by practicing sample problems. Work through a variety of problems to ensure that you’re comfortable with the material. Focus on problems that challenge you, as these will help identify areas where you need to improve. By solving different types of questions, you’ll build the confidence needed to tackle the actual assessment.
Tip: Time yourself while working through sample problems to simulate the actual test environment. This will help you manage your time effectively during the assessment.
Final Step: Review mistakes from practice problems and understand why you made them. This will prevent similar errors during the assessment and improve your problem-solving skills.