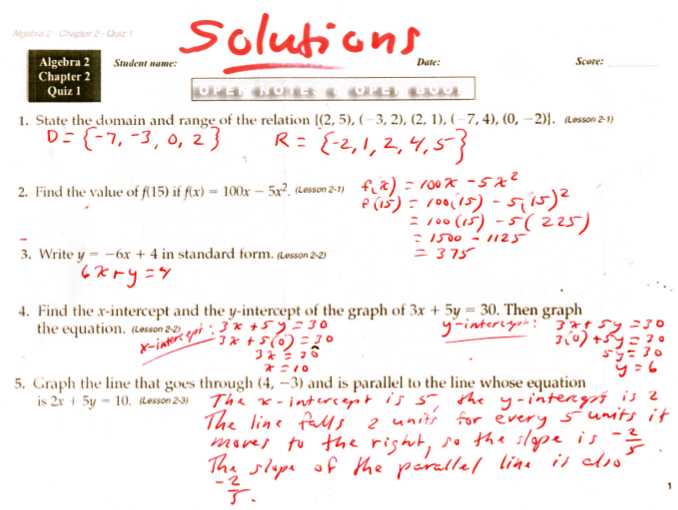
In this section, we explore essential techniques and methods that are fundamental to solving mathematical problems involving variables, equations, and functions. Understanding these concepts is crucial for building a strong foundation in mathematics and achieving success in exams.
Efficient problem-solving involves recognizing patterns, applying logical steps, and practicing various techniques. By mastering these skills, you can tackle a wide range of exercises with confidence and precision.
Preparation is key to excelling in mathematical assessments. Through focused practice and thorough understanding of core principles, you’ll improve your ability to solve problems accurately and quickly. This section offers helpful resources and guidance for sharpening your skills.
Algebra 1 Chapter 3 Test Answers
In this section, we will focus on key solutions and techniques needed to solve a variety of problems related to equations, variables, and functions. A solid understanding of these core principles is essential for progressing in mathematical studies and improving your problem-solving skills.
Mastering each method is crucial to navigating through the various exercises with confidence. By practicing different types of problems, you can develop a systematic approach to tackling each one effectively. Accuracy and speed are the result of consistent effort and familiarity with the material.
To further enhance your understanding, it’s important to go beyond memorization and focus on applying strategies in real-world scenarios. This section provides valuable insights into simplifying complex expressions, graphing lines, and solving systems of equations, all of which are necessary for achieving mastery.
Understanding Linear Equations and Graphing
Grasping the concept of straight-line equations and their graphical representation is essential for solving various types of problems. These relationships describe how variables interact, and visualizing them on a graph offers insights into their behavior. A strong understanding of this topic provides the foundation for solving more complex problems later on.
Linear equations are expressions where the highest exponent of the variable is one, creating a straight line when plotted on a coordinate plane. The ability to understand and manipulate these equations is a key skill in mathematics.
When working with these equations, it’s important to consider the following points:
- The general form of a linear equation is y = mx + b, where m represents the slope and b the y-intercept.
- The slope (m) indicates the steepness of the line, showing how much y changes for a given change in x.
- The y-intercept (b) represents the point where the line crosses the y-axis, indicating the value of y when x is zero.
To graph these equations, start by plotting the y-intercept on the graph, then use the slope to find other points. Connect the points to form the line. This visual approach makes it easier to understand the relationship between the variables and how they change in relation to each other.
Solving Systems of Linear Equations
When working with multiple equations involving the same set of variables, the goal is to find values that satisfy all equations simultaneously. This process is crucial in a variety of mathematical contexts, from real-world applications to abstract problem-solving scenarios. The solution to such a system is where the equations intersect, either as a point on a graph or as a set of values that solve all equations at once.
There are several methods for solving these types of problems, each of which provides a systematic way to find the solution:
- Graphing Method: Plot each equation on the same coordinate plane and identify the point where the lines intersect. The coordinates of the intersection point represent the solution.
- Substitution Method: Solve one equation for one variable and substitute that expression into the other equation to find the values of both variables.
- Elimination Method: Add or subtract the equations to eliminate one variable, making it easier to solve for the remaining variable.
Mastering these methods allows for flexibility when dealing with systems of equations, enabling you to choose the most efficient approach depending on the situation. Understanding the underlying principles makes it easier to interpret and solve problems with multiple variables, ultimately enhancing your mathematical skills.
How to Simplify Algebraic Expressions
Simplifying expressions is a crucial skill that helps in solving more complex problems. By reducing expressions to their simplest form, you can make calculations more straightforward and easier to manage. This process often involves combining like terms, eliminating unnecessary parentheses, and applying fundamental rules of arithmetic and operations.
Combining Like Terms
One of the first steps in simplifying is identifying and combining like terms. These are terms that have the same variable and exponent. For example, in the expression 3x + 5x, both terms contain the variable x, so they can be combined into 8x.
Applying Distribution and Simplifying
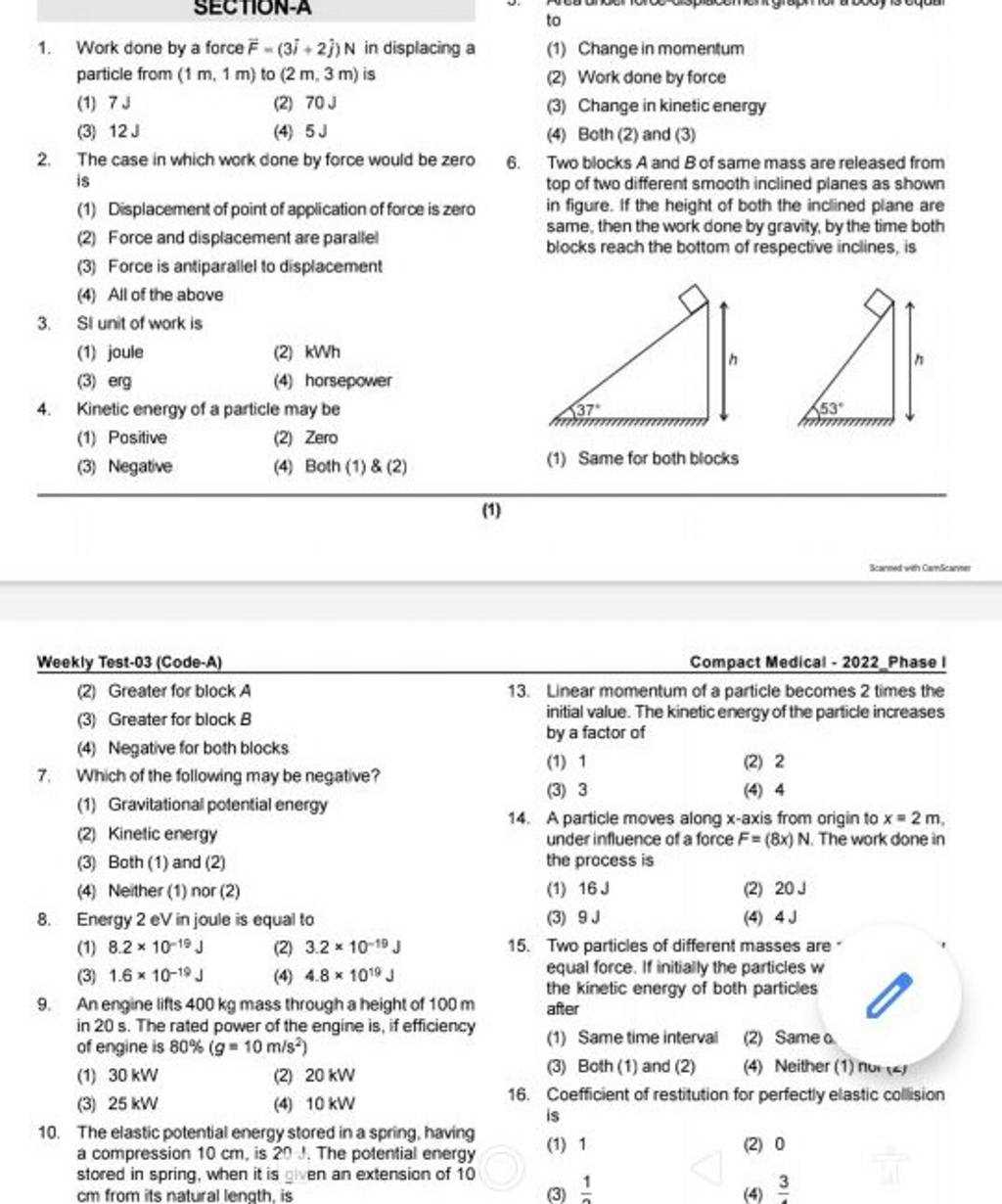
The distributive property allows you to simplify expressions where a term outside parentheses multiplies every term inside. For instance, in 3(2x + 4), you would distribute the 3 to both terms inside the parentheses, resulting in 6x + 12. This approach reduces the expression to a simpler form.
By following these steps and consistently practicing, you can efficiently simplify expressions, making it easier to solve equations and tackle more advanced mathematical tasks.
Techniques for Solving Word Problems
Word problems often require translating a real-world situation into a mathematical equation. This process can seem daunting at first, but with the right approach, solving these problems becomes much more manageable. The key is to break down the problem, identify important information, and systematically apply appropriate methods to find the solution.
One effective strategy is to follow a step-by-step approach. The steps include reading the problem carefully, defining variables, setting up equations, solving for the unknown, and finally, interpreting the solution in the context of the original problem. Here is a summary of these steps:
| Step | Description |
|---|---|
| 1. Read and Understand | Read the problem carefully, highlighting key information and identifying what is being asked. |
| 2. Define Variables | Assign variables to represent unknown quantities. This helps in translating the problem into an equation. |
| 3. Set Up an Equation | Write a mathematical equation based on the relationships described in the problem. |
| 4. Solve the Equation | Use appropriate methods (e.g., solving for x, simplifying expressions) to find the value of the unknown. |
| 5. Interpret the Solution | Review the answer in the context of the problem to ensure it makes sense. |
By following these steps, you’ll improve your ability to tackle word problems efficiently and accurately, turning complex situations into solvable mathematical tasks.
Identifying Slope and Y-Intercept
Understanding the relationship between variables in a linear equation is crucial for solving various problems. The slope and y-intercept are two key components that describe how the values of the variables change in relation to one another. By recognizing these elements, you can graph lines and interpret their behavior accurately.
Slope represents the rate of change between the two variables. It indicates how much one variable increases or decreases in response to a change in the other. In mathematical terms, the slope is often expressed as the ratio of the vertical change to the horizontal change between two points on the graph.
Y-intercept refers to the point where the line crosses the vertical axis. This value represents the value of the dependent variable when the independent variable is zero. Identifying the y-intercept helps in graphing and understanding the line’s position on the coordinate plane.
By carefully analyzing an equation in slope-intercept form, such as y = mx + b, you can quickly identify the slope m and the y-intercept b, making it easier to graph and interpret the line’s behavior.
Understanding Function Notation in Algebra
Function notation is a way of representing mathematical relationships between variables, making it easier to describe and work with functions. It is a shorthand method that helps identify how one quantity depends on another. Understanding this notation is essential for interpreting and solving equations involving functions.
What is Function Notation?
In function notation, a function is typically represented as f(x), where f represents the function name, and x is the input variable. The value of the function is determined by substituting the value of x into the function expression.
How to Interpret Function Notation
To fully understand function notation, it’s important to recognize its components:
- f(x): Represents the output of the function when the input is x.
- x: The independent variable, or the input value for which you want to calculate the output.
- f(3): This would be the value of the function when x = 3. You substitute 3 into the function to find the corresponding output.
For example, if the function is f(x) = 2x + 1, and you are asked to find f(4), you would substitute 4 for x and calculate the result: f(4) = 2(4) + 1 = 9.
Understanding this notation is crucial for solving problems that involve functions, whether in graphing, evaluating expressions, or analyzing relationships between variables.
Practice Problems for Chapter 3 Test
To improve your skills and prepare for upcoming assessments, practicing with sample problems is essential. These exercises will help reinforce the concepts you’ve learned and ensure you’re confident in applying them to solve real-world and mathematical situations. Here are a variety of practice problems to test your understanding of the material.
Below are some problems that will help you practice the key techniques:
- Problem 1: Solve for x: 3x + 5 = 20
- Problem 2: Find the slope and y-intercept of the line represented by the equation y = 2x – 4
- Problem 3: Simplify the expression: 5x + 3x – 2
- Problem 4: Solve the system of equations:
- 2x + y = 8
- x – y = 2
- Problem 5: Determine the value of f(5) for the function f(x) = 3x – 7
As you work through these problems, make sure to check your solutions carefully. Practice is the key to mastering these concepts, and with consistent effort, you’ll build your confidence in handling similar questions when it counts.
Common Mistakes to Avoid in Algebra 1
When solving mathematical problems, it’s easy to make small mistakes that can lead to incorrect answers. Understanding the most common errors can help you avoid them and improve your problem-solving skills. By being aware of these pitfalls, you can approach each problem more confidently and accurately.
Here are some of the frequent mistakes that students encounter and tips on how to avoid them:
| Mistake | How to Avoid It |
|---|---|
| Incorrectly distributing terms | Make sure to apply distribution rules carefully. For example, when multiplying a term by a binomial, remember to multiply each term separately. |
| Forgetting to apply the order of operations | Always follow PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) to ensure the correct order in your calculations. |
| Misinterpreting negative signs | Pay close attention to negative signs when adding or subtracting terms. A common mistake is confusing -(-x) with x. |
| Skipping steps when solving equations | It’s easy to rush and skip intermediate steps. Always show all the work so you can spot any errors along the way. |
| Improperly simplifying fractions | Make sure to simplify fractions fully by dividing both the numerator and denominator by their greatest common factor. |
By being mindful of these common errors and practicing careful problem-solving techniques, you’ll be better equipped to handle more complex problems with accuracy and confidence.
Step-by-Step Guide to Solving Equations
Solving equations is a fundamental skill in mathematics that requires following a systematic approach to isolate the unknown variable. Whether you’re working with simple linear equations or more complex expressions, the steps remain largely the same. Mastering these steps ensures that you can confidently solve any equation you encounter.
Step 1: Simplify Both Sides
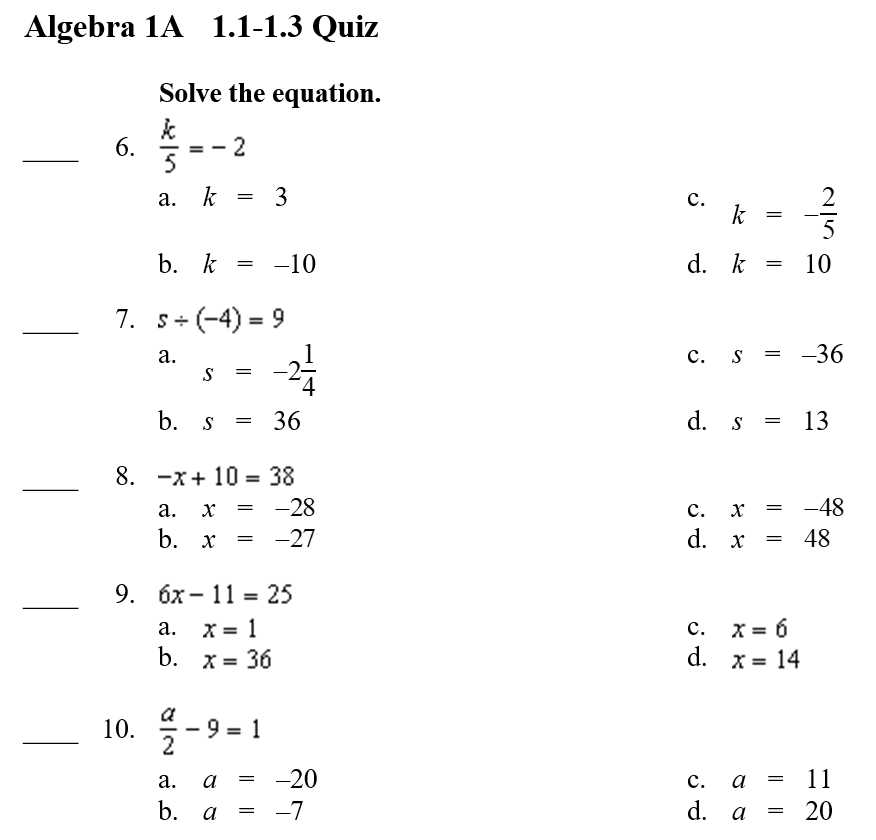
Start by simplifying both sides of the equation. This might involve combining like terms or distributing any coefficients. Simplifying makes the equation easier to solve and reduces the chance of errors later on.
Step 2: Isolate the Variable
The next step is to isolate the unknown variable on one side of the equation. Use inverse operations to move terms from one side to the other. For example, if the variable is multiplied by a number, divide both sides by that number. If it’s added to a value, subtract that value from both sides.
Repeat this process as necessary until the variable is by itself on one side of the equation. The result will give you the value of the variable.
For example, consider the equation: 3x + 4 = 16. First, subtract 4 from both sides: 3x = 12. Then, divide both sides by 3: x = 4.
By following these steps, you can efficiently solve equations and reach the correct solution with confidence.
How to Check Your Algebra Answers

After solving a mathematical problem, it’s important to verify your solution to ensure accuracy. Double-checking helps identify any mistakes and reinforces your understanding of the problem-solving process. Here are several methods to confirm your results:
- Substitute the solution back into the original equation: Once you have found a value for the variable, plug it back into the equation to see if both sides are equal. If the equation holds true, your solution is correct.
- Use inverse operations: After isolating the variable, you can reverse the operations step by step to check if you get back to the original equation.
- Estimate the answer: Before solving, estimate a reasonable range for the solution. After calculating, compare your result with the estimate to see if it makes sense.
- Check for common errors: Review the steps you took to solve the problem. Look for mistakes such as incorrect distribution, adding instead of subtracting, or misapplying the order of operations.
By following these strategies, you can ensure your solutions are correct and identify any potential errors quickly. Regularly checking your work will help you become more confident in solving problems accurately.
Key Formulas for Chapter 3 Success
In this section, we will explore the essential formulas that form the foundation for solving a variety of problems. These formulas serve as tools for simplifying expressions, solving equations, and understanding relationships between different quantities. Mastering these formulas is crucial for achieving success in the given material.
Linear Equation Formula
One of the most important formulas to know is the linear equation formula, which is often written as:
y = mx + b
Here, m represents the slope of the line, and b is the y-intercept. This formula is key to understanding the relationship between variables and graphing linear equations effectively.
Point-Slope Formula
The point-slope formula is another helpful tool when working with linear equations. It allows you to find the equation of a line when you know a specific point on the line and the slope:
y – y₁ = m(x – x₁)
In this formula, (x₁, y₁) represents a point on the line, and m is the slope. This formula is particularly useful when you need to quickly determine an equation based on known data.
By memorizing and applying these formulas, you will be better equipped to handle a variety of problems and strengthen your problem-solving abilities. Practice using these formulas regularly to build confidence and improve your skills.
Tips for Improving Test Scores

Achieving higher scores in any math-related assessment requires both strategic preparation and consistent practice. By focusing on key techniques and improving your approach to problem-solving, you can enhance your understanding and performance. Here are some effective tips to boost your results:
- Practice Regularly: Consistent practice helps reinforce concepts and improve your problem-solving speed. Try solving a variety of problems daily to build familiarity and confidence.
- Understand the Concepts: Rather than memorizing formulas and steps, focus on understanding the underlying concepts. This will allow you to adapt to different problem types and avoid common mistakes.
- Review Mistakes: After completing practice problems or previous assessments, review any mistakes carefully. Identify where you went wrong and learn from those errors to avoid repeating them.
- Use Study Aids: Utilize additional resources such as textbooks, online tutorials, and practice worksheets to deepen your knowledge and gain more practice with challenging topics.
- Manage Your Time: During your study sessions, create a structured schedule that allows you to focus on each topic without feeling overwhelmed. Time management during the exam is also crucial for ensuring you complete all questions.
By applying these strategies, you can gradually improve your performance, feel more confident, and achieve better results in your assessments.
Reviewing Key Concepts Efficiently
Efficiently reviewing important mathematical concepts is crucial for solidifying your understanding and improving problem-solving skills. Instead of passively reading through notes, focus on active strategies that reinforce your knowledge and help identify areas needing further attention. Here are some tips to maximize your review sessions:
- Break It Down: Divide the material into smaller, manageable sections. Tackling one topic at a time helps prevent feeling overwhelmed and ensures deeper understanding of each concept.
- Practice with Purpose: Instead of solving random problems, focus on areas that you find most challenging. This targeted practice strengthens your weak spots and ensures you’re prepared for various question types.
- Summarize Key Ideas: After reviewing each topic, write a brief summary of the main ideas and methods involved. This reinforces your understanding and serves as a quick reference when needed.
- Use Flashcards: Create flashcards with important formulas, theorems, or steps to solve common problems. Review them regularly to keep key concepts fresh in your mind.
- Group Study Sessions: Join study groups to discuss complex topics. Explaining concepts to others can enhance your own understanding and reveal any gaps in knowledge.
By applying these strategies during your review, you can increase retention, reduce stress, and gain the confidence needed to tackle assessments with ease.
Using Online Resources for Practice
Online platforms offer a wealth of interactive tools and materials that can significantly enhance your understanding of mathematical principles. These resources provide immediate feedback, step-by-step guidance, and a variety of practice problems that cater to different learning styles. By incorporating online tools into your study routine, you can engage in active learning and deepen your mastery of challenging topics.
- Interactive Problem Solvers: Websites and apps that allow you to input problems and receive instant solutions with explanations help you understand the reasoning behind each step. This is especially helpful for complex equations or unfamiliar concepts.
- Video Tutorials: Many online platforms offer detailed video lessons that break down difficult topics into digestible parts. Visual learners can benefit greatly from these tutorials, as they often include demonstrations and real-world examples.
- Practice Quizzes: Take advantage of quizzes that test your skills on various topics. Regularly practicing under timed conditions helps simulate test environments and improve problem-solving speed.
- Discussion Forums: Join online study groups or forums where you can ask questions and collaborate with others. Engaging in discussions with peers or experts can clarify doubts and offer new perspectives on solving problems.
- Progress Tracking: Many online resources track your performance, allowing you to identify areas where you need improvement. This helps you focus your efforts more effectively and monitor your growth over time.
By integrating these online tools into your study routine, you can supplement traditional learning methods and gain a more comprehensive understanding of mathematical concepts. Regular practice using these resources will strengthen your skills and build confidence.