
Mastering complex mathematical problems requires both a clear understanding of the concepts and the ability to apply them effectively in various situations. Whether preparing for a standardized test or reviewing key ideas, focusing on problem-solving strategies can significantly enhance your performance. This guide provides insights into tackling challenging exercises, offering step-by-step solutions and explanations to ensure a deeper grasp of the material.
By breaking down each question and explaining the methods used to arrive at the correct solution, learners can develop a systematic approach to mathematics. Practicing regularly and analyzing different types of questions will not only improve accuracy but also increase confidence. A well-structured study plan focused on understanding rather than memorization is essential for success in any evaluation.
Achieving proficiency in these advanced topics involves recognizing patterns, selecting the right techniques, and refining problem-solving skills through consistent practice. Whether you are revisiting concepts or facing an exam, this resource will guide you through each stage, helping to unlock your potential and achieve your best results.
Understanding Algebra 2 Regents Format
Familiarizing yourself with the format of standardized math assessments is crucial for successful preparation. Knowing the structure of the test allows you to approach each section confidently and effectively. These exams are designed to evaluate your ability to solve a variety of mathematical problems, each requiring different techniques and methods.
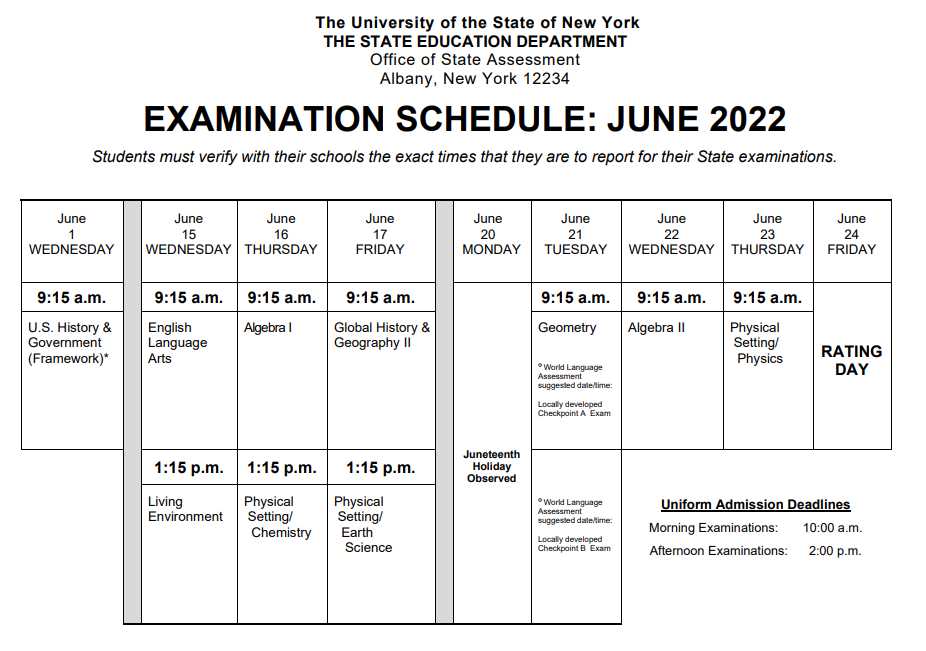
The test typically consists of multiple sections, each focusing on different aspects of mathematics. Understanding the distribution of questions, as well as the types of problems included, can help in managing time and prioritizing areas of study. Below is a breakdown of the common sections you will encounter:
| Section | Type of Problems | Skills Tested |
|---|---|---|
| Multiple Choice | Various question formats with one correct answer | Basic concept understanding, quick calculation |
| Short Response | Problems requiring written explanations or calculations | Problem-solving steps, clarity of explanation |
| Extended Response | Complex problems that require detailed solutions | Critical thinking, thorough solution presentation |
Each section is designed to assess your ability to apply mathematical principles in various contexts. Preparation for such exams should focus on developing both speed and accuracy, as well as the ability to explain and justify your reasoning. By practicing different types of questions, you can gain the confidence needed to tackle any problem that appears on the exam.
Key Topics Covered in Algebra 2 Exam

The exam assesses a range of mathematical concepts that test your ability to apply logical thinking, problem-solving techniques, and various mathematical strategies. A strong understanding of key topics is essential for performing well in these evaluations. The exam usually covers topics that involve both basic operations and more complex problem-solving methods.
Students are expected to demonstrate their knowledge in areas such as polynomials, functions, and advanced graphing techniques. Topics related to equations, inequalities, and systems of equations are also heavily emphasized. In addition, the test may include questions on quadratic functions, exponential growth, and statistical analysis, requiring both theoretical and practical application.
Preparation should include mastering these core areas, as each plays a crucial role in answering a variety of problem types. Focusing on the specific skills required for each topic can significantly improve performance and help you approach the exam confidently.
Step-by-Step Solutions for Algebra 2
Breaking down complex problems into manageable steps is a proven method for mastering mathematical challenges. By following a structured approach, you can ensure that each solution is both accurate and clear. This section will guide you through various problem-solving techniques using detailed steps for each solution.
The key to success lies in understanding the problem fully before jumping into calculations. Here’s a general approach to solving these problems:
- Read the problem carefully: Ensure you understand what is being asked. Identify key information and what needs to be solved.
- Choose an appropriate method: Depending on the problem type, decide whether to use factoring, substitution, graphing, or another strategy.
- Perform the necessary operations: Apply the chosen method, ensuring that every step is done correctly.
- Check your work: Once a solution is found, review the process to verify accuracy.
Here are a few examples of common steps involved in solving typical problems:
- Factoring equations: Begin by factoring out the greatest common factor, then set each factor equal to zero to solve.
- Graphing functions: Identify key points, plot them on a graph, and analyze the shape of the curve or line to find solutions.
- Solving systems of equations: Use substitution or elimination to simplify the system and find the variables.
Each problem requires specific attention to detail, and practicing these steps regularly will improve your problem-solving skills. By using a step-by-step approach, you can work through even the most challenging questions with confidence and precision.
How to Approach Problem Solving
Effectively tackling mathematical challenges requires a structured approach. It’s important to remain focused and methodical, breaking down each problem into manageable parts. By following a clear set of steps, you can better organize your thoughts and reach a solution more efficiently. Understanding the underlying principles and applying them to different scenarios is key to success.
Identify the Problem
The first step in any problem-solving process is to fully understand what is being asked. Carefully read the question and highlight important details such as numbers, operations, and any conditions provided. Make sure to clarify what the solution should represent, whether it’s a specific value, a range of values, or a general expression.
Choose the Right Strategy
Once you understand the problem, decide on the most suitable method for solving it. Depending on the type of question, you might need to apply different techniques such as simplifying equations, factoring, or using graphing methods. Selecting the correct strategy from the outset can save valuable time and increase accuracy in your solution.
By following these basic steps–analyzing the problem and choosing the right approach–you’ll be able to handle even the most challenging questions with confidence and precision.
Reviewing Algebra 2 Regents Question Types
Understanding the different types of questions commonly found in math assessments is essential for efficient preparation. Each problem type is designed to test specific skills, from basic calculations to more complex problem-solving methods. Familiarity with these question types will help you approach each task confidently and methodically.
Multiple-Choice Questions
These questions offer a set of possible answers, from which you must select the correct one. Multiple-choice questions are often designed to test fundamental concepts and quick thinking. While the answer may seem straightforward, it’s important to carefully consider each option and ensure that it aligns with the principles being tested. Elimination techniques can be useful when narrowing down your choices.
Open-Ended and Short Response Questions

Unlike multiple-choice questions, these require you to show your full solution process. These problems test your ability to articulate your reasoning and demonstrate your approach step by step. It’s important to include all necessary calculations and clearly explain the logic behind your solution. Thoroughness and clarity are key to scoring well on these types of questions.
By practicing a variety of question types, you will develop a flexible problem-solving mindset, capable of tackling any challenge presented in the assessment.
Important Formulas for Exam Success
Mastering key mathematical formulas is essential for performing well in assessments. These formulas serve as the foundation for solving a wide range of problems, from basic calculations to more complex equations. Knowing when and how to apply them can save valuable time and increase accuracy during the exam.
Core Formulas to Remember
There are several formulas that are frequently used in various problem types. Here are some of the most important:
- Quadratic Formula: Used to solve quadratic equations:
x = (-b ± √(b² – 4ac)) / 2a - Slope Formula: Used to find the slope between two points:
m = (y₂ – y₁) / (x₂ – x₁) - Pythagorean Theorem: Used to find the sides of a right triangle:
a² + b² = c² - Exponential Growth Formula: Used to calculate exponential change:
y = a(1 + r)^t
Advanced Formulas for Complex Problems
For more complex challenges, advanced formulas are often required. Here are a few more formulas to familiarize yourself with:
- Distance Formula:
d = √((x₂ – x₁)² + (y₂ – y₁)²) - Logarithmic Properties:
log_b(xy) = log_b(x) + log_b(y) - Area of a Circle:
A = πr²
By memorizing these formulas and understanding when to apply them, you will be better prepared to tackle a variety of problems during the exam.
Common Mistakes to Avoid in Algebra 2
Even the most well-prepared students can make errors during problem-solving, especially when working under time pressure. Recognizing and avoiding common pitfalls can help improve both accuracy and efficiency. Being aware of frequent mistakes allows you to approach problems with greater confidence and avoid simple missteps that could cost valuable points.
Here are some of the most common mistakes students make, and tips on how to avoid them:
- Misinterpreting the Problem: Sometimes students rush through reading a problem and miss key details. Always take time to understand what is being asked before proceeding.
- Incorrectly Applying Formulas: Using the wrong formula or misapplying it can lead to incorrect results. Double-check that you are using the appropriate formula for the problem at hand.
- Neglecting to Simplify Expressions: Leaving answers in complex forms or failing to simplify can lead to unnecessary confusion. Make sure to reduce equations or expressions to their simplest forms.
- Forgetting to Check Work: Rushing to complete the test can lead to overlooking simple mistakes. Always leave time to review your work and verify calculations.
- Sign Errors: Negative signs are a common source of mistakes, especially when working with polynomials or solving equations. Carefully track signs throughout the process.
Avoiding these common mistakes will not only improve your accuracy but also build your problem-solving skills, helping you tackle complex challenges more effectively.
Using Graphing Techniques Effectively
Graphing is a powerful tool for visualizing mathematical relationships and understanding the behavior of functions. When used effectively, it can simplify complex problems, making them easier to solve and interpret. Mastering graphing techniques allows you to find solutions quickly and accurately, especially when dealing with equations or inequalities.
Plotting Key Points

One of the most essential graphing techniques is identifying key points on a graph. Start by finding the x- and y-intercepts, as these often provide valuable information about the function’s behavior. Plotting these points helps establish a basic framework for the graph and makes it easier to sketch the overall shape of the function.
Analyzing the Graph’s Shape
Once key points are plotted, the next step is to analyze the graph’s overall shape. Look for patterns such as symmetry, slopes, or curves that can help you determine the nature of the function. Understanding how the graph behaves will assist in predicting its behavior for other values and provide insights into the solution to the problem.
By using these graphing techniques effectively, you can approach problems with greater confidence and clarity, allowing you to solve them more efficiently and accurately.
Time Management Tips for the Exam
Effective time management is essential for success in any exam. Properly allocating your time during the test ensures that you can complete all sections and maximize your performance. By following strategic approaches to time management, you can reduce stress and stay focused throughout the entire assessment.
Here are some key tips to help manage your time during the exam:
- Prioritize Easy Questions: Begin with questions that you find easiest or most familiar. This builds confidence and ensures you secure those points quickly.
- Set Time Limits: Allocate a specific amount of time for each section or question. Stick to these limits to avoid spending too much time on any one task.
- Leave Time for Review: Always reserve a few minutes at the end of the exam to review your answers. This will help catch any mistakes you may have overlooked.
- Don’t Get Stuck: If you encounter a difficult question, move on and come back to it later. Spending too much time on one problem can cost you more points in the long run.
- Stay Calm and Focused: Keep track of the clock but don’t let it create unnecessary pressure. Stay calm, and pace yourself throughout the exam.
By following these time management tips, you’ll be able to tackle the exam with confidence and efficiency, giving yourself the best chance for success.
Breaking Down Complex Problems
When faced with a challenging question, it’s easy to feel overwhelmed. However, the key to solving difficult problems lies in breaking them down into smaller, more manageable parts. By approaching each component step by step, you can simplify the problem and avoid feeling stuck. This method allows for a clearer understanding of the overall process and improves accuracy in reaching the solution.
Here are some effective strategies for breaking down complex problems:
- Identify the Given Information: Begin by reading the problem carefully and highlighting the key facts. Understand what is given and what needs to be determined.
- Divide the Problem into Steps: Break the problem into smaller steps or stages. Focus on solving each part individually before combining the results.
- Look for Patterns: Often, recognizing patterns can help simplify the problem. Look for relationships between the variables or repeated elements that may provide insight into the solution.
- Use Substitutions or Approximations: When appropriate, substitute simpler values or make approximations to ease the calculations and test your understanding of the problem’s structure.
- Review the Solution: After completing the steps, review your solution. Ensure that all parts are addressed and check for any errors that might have been made along the way.
By following these strategies, you can break down even the most complex problems into more approachable tasks, ultimately making them easier to solve and ensuring a higher degree of accuracy.
Tips for Memorizing Algebra 2 Concepts

Mastering mathematical concepts requires not only practice but also effective memorization techniques. By utilizing various methods to retain key principles and formulas, you can enhance your recall and improve your problem-solving abilities. This section focuses on strategies to help you memorize important concepts more efficiently, ensuring that you can apply them confidently during assessments.
Here are some useful tips to aid in memorizing complex concepts:
- Practice Regularly: Repetition is one of the most effective ways to commit concepts to memory. Consistently practicing problems will reinforce your understanding and help you remember key ideas.
- Create Mnemonics: Mnemonics are memory aids that simplify the process of remembering complex information. Creating acronyms or rhymes can help you recall formulas or procedures quickly.
- Visualize the Concepts: Drawing diagrams or visualizing the relationships between variables can help reinforce abstract ideas. This is especially useful for functions, equations, and graphing techniques.
- Teach What You Learn: Teaching a concept to someone else forces you to articulate your understanding clearly. This method strengthens your grasp of the material and helps highlight areas that need further clarification.
- Group Similar Concepts: Organizing related ideas together can make them easier to memorize. Categorizing formulas or methods into logical groups allows for better recall when solving problems.
By incorporating these techniques into your study routine, you’ll improve both your retention and application of mathematical concepts, ensuring success on tests and assignments.
How to Check Your Work Quickly
Ensuring the accuracy of your solutions is an essential part of problem-solving. By efficiently reviewing your answers, you can identify any mistakes before submitting your work. This section outlines strategies for quickly verifying the correctness of your results, allowing you to catch errors without spending too much time on the review process.
Here are some effective methods to check your work:
- Double-Check Key Steps: Go over the most critical parts of your solution, such as calculations or important formulas. Confirm that each step follows logically from the previous one.
- Use Estimation: For numerical problems, estimate the expected range of the answer before you begin. After solving, check if your result falls within that range to identify any obvious errors.
- Reverse Your Process: If possible, work backward from your final answer to the original problem. This can help identify mistakes that may have been made during the solution process.
- Check Units and Variables: Verify that all units are consistent, and that variables are used correctly throughout your solution. Mistakes in unit conversion or variable substitution are common.
- Review Your Work in Chunks: Rather than checking everything at once, review your solution in manageable sections. This makes it easier to spot errors that you might have missed if reviewing all at once.
By implementing these techniques, you can improve the accuracy of your solutions and save time during the verification process, ensuring that you complete each problem correctly and efficiently.
How to Interpret Word Problems
Word problems can seem daunting at first, but they are simply a way to apply mathematical concepts to real-life situations. Understanding how to extract the relevant information from a word problem and translate it into a solvable equation or process is key to solving them efficiently. This section covers strategies to help you approach and interpret word problems with confidence and clarity.
Here are some steps to effectively interpret word problems:
- Read the Problem Carefully: Begin by reading the entire problem carefully to get an understanding of what is being asked. Pay attention to any specific instructions or conditions given in the problem.
- Identify Key Information: Highlight or underline the important details such as numbers, units, and relationships. These pieces of information will form the foundation of your solution.
- Define Variables: If the problem involves unknowns, assign variables to represent them. This makes it easier to set up an equation later on.
- Understand the Question: Make sure you know exactly what the problem is asking. Are you solving for a specific variable, calculating a total, or finding a rate? Clarifying this will guide your approach.
- Translate into Mathematical Terms: Convert the words into mathematical operations. For example, phrases like “total cost” may indicate addition, while “difference” could suggest subtraction. Identify the relationships between the given values.
- Set Up the Equation: Use the information and relationships you’ve identified to create an equation or formula. Ensure that all variables and constants are correctly placed in the equation.
- Check for Consistency: Before solving, ensure that all units and variables are consistent. This helps avoid errors during calculations.
By following these steps, you’ll be able to break down word problems into manageable parts, allowing you to focus on the mathematics involved and find the solution more easily.
Exploring Practice Questions for Preparation
Practicing problems is an essential part of preparing for any exam. By regularly solving practice questions, you can familiarize yourself with the format, test your knowledge, and improve your problem-solving skills. This section provides insight into how to use practice questions effectively for exam preparation.
Here are some tips for making the most of practice questions:
- Start with Basic Problems: Begin by tackling simpler questions to build your confidence. These problems help reinforce foundational concepts and ensure you’re comfortable with basic techniques.
- Gradually Increase Difficulty: As you become more confident, move on to more challenging problems. These questions test your ability to apply concepts in more complex scenarios and prepare you for the real exam.
- Review Incorrect Answers: After completing a set of questions, focus on any problems you got wrong. Analyze where you made mistakes and understand why the correct solution works. This helps you avoid similar errors in the future.
- Simulate Exam Conditions: Practice solving questions under timed conditions. This helps you manage time effectively during the actual exam and allows you to gauge your pacing.
- Mix Topics: Don’t just practice one type of problem. Mix different topics and question types. This helps you become versatile and ensures you’re prepared for any question that may come up.
Incorporating these practices into your study routine will enhance your understanding of key concepts and make you more adept at tackling questions under exam pressure.
Strategies for Tackling Multiple-Choice Questions
Multiple-choice questions can seem daunting, but with the right approach, they become much easier to navigate. In this section, we will explore some strategies that can help you approach these types of questions efficiently and effectively. By using these techniques, you can increase your accuracy and speed while answering.
Key Strategies for Multiple-Choice Success
To tackle multiple-choice questions effectively, it’s important to have a structured approach:
- Read the Question Carefully: Before jumping to the answer choices, ensure that you fully understand the question. Look for keywords or phrases that tell you what is being asked.
- Eliminate Clearly Wrong Answers: Often, there will be one or more choices that are obviously incorrect. Crossing out these options narrows down your choices, improving the chances of selecting the correct answer.
- Use Process of Elimination: If you’re unsure of the correct answer, eliminate the answers you know are incorrect. This will leave you with fewer options and a higher probability of selecting the right one.
- Look for Patterns: Some tests may have answer choices that follow a pattern (e.g., all numbers are increasing or decreasing). Recognizing these patterns can help you eliminate certain answers.
- Guess Strategically: If you must guess, make an educated guess. Avoid randomly selecting an option, and instead use clues from the question or other questions to guide your decision.
Example of a Multiple-Choice Question
Let’s take a look at an example of how to apply these strategies:
| Question | Answer Choices |
|---|---|
| What is the value of x in the equation 3x + 5 = 20? |
A) 5 B) 7 C) 15 D) 10 |
Step 1: Read the question carefully: “What is the value of x in the equation 3x + 5 = 20?”
Step 2: Eliminate obviously incorrect answers: C and D don’t make sense when substituted into the equation.
Step 3: Use the remaining options (A and B) to solve the equation. You’ll find that the correct answer is B, 7.
By practicing these strategies, you can approach multiple-choice questions with confidence and increase your chances of selecting the correct answer. Keep honing these skills, and soon, answering these questions will become second nature.
Focus Areas for Maximum Scoring
Achieving a high score in any assessment requires a strategic focus on key areas. Certain topics tend to carry more weight and often appear more frequently in evaluations. By prioritizing these areas and mastering the core concepts, you can significantly improve your performance. This section highlights essential topics to focus on for maximum scoring potential.
1. Problem-Solving Skills: Being able to approach and solve problems efficiently is vital. Focus on developing strong critical thinking abilities, so you can quickly identify the most effective strategies for solving different types of questions.
2. Key Concepts and Formulas: Understanding fundamental principles and knowing when to apply specific formulas is crucial. Review and memorize the most commonly used equations, as well as the logical steps to implement them.
3. Graphing and Visual Representation: Many questions require the ability to interpret and create graphs. Practice plotting functions, identifying key features such as intercepts and slopes, and analyzing graphical data to draw conclusions.
4. Word Problems and Interpretation: Word problems can often be the most challenging. It’s essential to practice translating real-world scenarios into mathematical expressions. Focus on breaking down these problems into manageable parts and identifying the key information needed to solve them.
5. Time Management: Efficient use of time is vital during any timed assessment. Practice solving problems under timed conditions, and learn to gauge how long to spend on each question. Prioritize simpler questions to ensure you secure as many points as possible early on.
By focusing on these key areas, you’ll be well-prepared to tackle any challenge and achieve your desired score. Mastery of these topics will not only help you understand the material but will also give you the confidence to perform at your best.