In this guide, we will explore a resource designed to help students strengthen their mathematical abilities. This platform offers interactive challenges that engage learners in solving a variety of problems, allowing them to develop a deeper understanding of mathematical principles.
With each task, you’ll have the opportunity to practice and refine your problem-solving techniques, ensuring you grasp important concepts and improve your overall performance. By approaching each question step by step, you can build confidence in tackling more complex problems in the future.
Our goal is to provide effective solutions and strategies for overcoming common obstacles, enabling you to progress at your own pace. Whether you’re preparing for an exam or simply looking to enhance your skills, this resource serves as an essential tool for mastering mathematical techniques.
Mastering Algebra Nation Section 6

This part of the learning platform offers a set of exercises that are designed to strengthen your mathematical skills. By engaging with a variety of problems, you can deepen your understanding and improve your approach to solving different types of equations. The focus is on practical problem-solving techniques that help you apply theoretical knowledge in real-world scenarios.
Building a Strong Foundation
To succeed in this section, it’s important to first grasp the foundational concepts that are repeatedly tested. Strong comprehension of these basics will make tackling more complex challenges much easier. Start by reviewing key principles and ensure you’re confident in applying them before moving on to more advanced topics.
Approaching Each Problem with Confidence
When you face a problem, break it down into manageable steps. Focus on identifying what the question is asking and how to approach it systematically. Avoid rushing through the process, as careful attention to each detail can prevent mistakes and lead to more accurate results. As you practice, you’ll develop a rhythm that allows you to solve problems more efficiently.
How to Approach Section 6 Questions
When facing the exercises in this part of the platform, it’s crucial to approach each problem with a clear strategy. Instead of rushing through the questions, take your time to carefully analyze the problem, identify the variables involved, and choose the most efficient method to find a solution. By breaking down each problem step by step, you ensure accuracy and build confidence in your abilities.
Step-by-Step Problem Breakdown

The first step in tackling each challenge is to carefully read the problem and underline the key information. Look for numbers, operations, and any conditions or restrictions mentioned. Once you’ve identified the essential elements, plan your approach based on the tools you have learned. Whether it’s solving for an unknown variable or simplifying an expression, always follow a logical order.
Reviewing and Refining Your Solutions
After you’ve arrived at a solution, take the time to review your work. Check each step for accuracy and ensure that you didn’t overlook any details. It’s also helpful to recheck the problem’s requirements and make sure that your solution answers the original question completely. This final step will help you avoid careless mistakes and confirm that you understand the material.
Understanding Key Algebraic Concepts
To succeed in mathematical problem-solving, it’s essential to have a strong grasp of the fundamental concepts that underlie many of the problems you’ll encounter. These core principles provide the foundation for more complex tasks and are crucial for approaching challenges with confidence. Mastering these ideas ensures you’re well-prepared to tackle a wide range of problems and apply strategies effectively.
Key Concepts to Focus On
Here are some of the core ideas that play a critical role in solving problems:
- Equations: Understanding how to form, solve, and manipulate equations is essential in nearly every problem.
- Variables: Recognizing the role of variables and knowing how to work with them allows you to find solutions and express relationships between different elements.
- Operations: Mastering basic mathematical operations such as addition, subtraction, multiplication, and division is crucial for simplifying and solving problems.
- Factoring: Factoring expressions is a key skill that helps simplify complex problems and find solutions more easily.
- Functions: Understanding how functions work, including their behavior and graphing, is important for solving real-world scenarios.
Building Your Understanding
Once you’ve identified the key concepts, it’s important to practice applying them. Try solving various problems that require these skills, gradually increasing the complexity as you gain confidence. The more you practice, the more intuitive these concepts will become, helping you to recognize patterns and solve problems efficiently.
Breaking Down Section 6 Problems
When tackling a set of problems, it’s essential to approach each one methodically. Rather than diving straight into calculations, start by carefully analyzing the structure of the problem. This will allow you to identify the key elements and choose the most appropriate method for solving it. Breaking down each challenge step by step is the most effective way to avoid errors and ensure clarity in your solutions.
Steps for Efficient Problem Solving

Follow these steps to break down and solve each problem with precision:
- Read the problem carefully: Identify all relevant information and ensure you understand the question being asked.
- Highlight important data: Underline or circle numbers, operations, and any conditions given in the problem.
- Decide on the approach: Choose the most appropriate method based on the problem type (e.g., simplification, solving for an unknown, or graphing).
- Apply the correct operations: Follow through with calculations or steps needed to solve the problem, being careful with each step.
- Check your work: After reaching a solution, review each step to ensure accuracy and consistency with the problem’s requirements.
Common Challenges and Solutions
Some problems may present specific difficulties, but breaking them down into smaller parts can help. Here are a few common issues and how to overcome them:
- Complex expressions: Simplify the equation step by step before solving to avoid confusion.
- Multiple variables: If multiple variables are involved, solve for one variable at a time or use substitution to simplify.
- Confusing wording: If the problem seems unclear, rewrite it in your own words to clarify the meaning.
Step-by-Step Guide to Solutions
Solving problems effectively requires a clear, structured approach. Instead of rushing to a solution, breaking down each task into manageable steps allows you to stay organized and reduce the chances of mistakes. By following a systematic method, you can ensure that each part of the problem is addressed thoroughly and accurately.
Start by carefully reading the problem to understand what is being asked. Identify the key elements and decide on the best strategy to approach the solution. Then, proceed with the calculations or operations one step at a time, ensuring each step is logical and based on the previous one. Once you’ve reached a solution, take the time to verify your work, checking for any errors or overlooked details.
This method not only helps you arrive at the correct solution but also builds confidence in your problem-solving abilities, making it easier to tackle even more complex challenges in the future.
Common Mistakes to Avoid
While working through mathematical problems, it’s easy to make simple errors that can affect the accuracy of your solutions. Recognizing and avoiding these common mistakes is key to improving your overall performance. By paying attention to the details and following a structured approach, you can significantly reduce the likelihood of making these errors.
Overlooking Important Details
One of the most common mistakes is skipping over essential information within the problem. Make sure to read the question carefully and highlight important data such as numbers, variables, and any specific instructions. Missing even a small detail can lead to incorrect answers, so taking the time to fully understand the problem is crucial.
Rushing Through Calculations
Another frequent mistake is rushing through the steps without double-checking your work. It’s tempting to move quickly through calculations, but this often leads to careless mistakes. Always take a moment to review each step before moving on to ensure that you haven’t missed any important details. Accuracy is more important than speed when solving problems, so focus on taking your time and following the correct process.
By avoiding these common mistakes, you’ll improve your ability to solve problems effectively and increase your confidence in tackling even the most complex challenges.
Tips for Efficient Problem Solving
Solving problems efficiently requires more than just knowledge; it involves adopting strategies that allow you to work quickly without sacrificing accuracy. By focusing on key techniques and organizing your approach, you can save time and reduce the chances of making mistakes. These strategies will help you approach each challenge with clarity and precision, making the process smoother and more manageable.
One important technique is to break down each problem into smaller, more manageable parts. This allows you to focus on one step at a time without feeling overwhelmed. Additionally, familiarize yourself with common methods and formulas that can speed up your process, such as factoring or simplifying expressions. The more you practice, the more intuitive these methods will become.
Another helpful tip is to always double-check your work at various stages. Reviewing your calculations early can prevent small errors from snowballing into larger mistakes. Don’t rush through the problem-solving process. Take your time to understand the question and apply the best possible method for a precise solution.
Practicing with Sample Questions
Consistent practice is one of the most effective ways to improve problem-solving skills. Working through sample questions helps solidify understanding and reinforces the strategies needed to solve similar problems. By exposing yourself to various types of challenges, you become more adaptable and confident in applying different methods to find solutions.
Using practice questions allows you to identify patterns and familiar techniques that can be applied across multiple problems. It also gives you the opportunity to test your knowledge in real-world scenarios, helping you to recognize areas that might need more attention. The more you practice, the quicker you’ll be able to recognize the best approach for each unique situation.
Incorporating regular practice into your study routine will enhance both your speed and accuracy, ultimately leading to better results when faced with new and complex problems.
Using Algebra Nation for Better Results
Leveraging online platforms designed for learning and practice can significantly enhance your ability to grasp key concepts and improve your problem-solving skills. These platforms often offer a range of tools, including interactive lessons, practice exercises, and step-by-step guides, all aimed at reinforcing your understanding and helping you master challenging topics.
Key Features for Effective Learning
Here are some of the key benefits of using such a platform:
- Interactive Lessons: Engaging, user-friendly tutorials break down complex concepts into simpler, more digestible parts.
- Instant Feedback: Immediate results from practice problems allow you to identify mistakes and adjust your approach accordingly.
- Practice Exercises: Regular problem sets help reinforce what you’ve learned and boost retention.
- Progress Tracking: Keeping track of your improvement helps maintain motivation and ensures you’re progressing in the right direction.
Maximizing Your Success
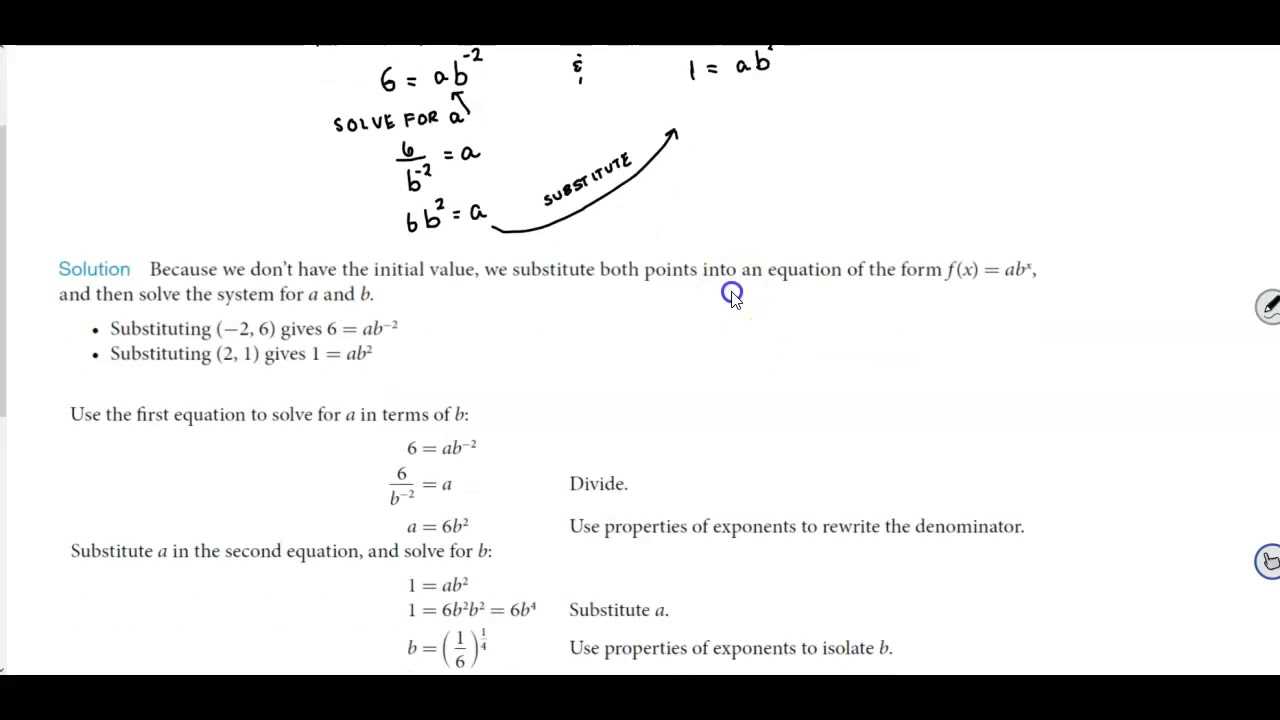
To get the most out of your study sessions, make use of all available features. Start with the interactive lessons to build a strong foundation, then move on to practice exercises to reinforce your learning. Don’t hesitate to revisit difficult topics and keep track of your progress to identify areas that need more attention.
Interactive Features in Section 6
Interactive tools provide an engaging way to strengthen your skills and deepen your understanding of key concepts. These features are designed to make learning more dynamic, allowing you to actively participate in the problem-solving process. By using such tools, you can work through problems at your own pace, receiving instant feedback and adjusting your approach as needed.
Interactive elements often include simulations, guided exercises, and visual aids that make complex topics easier to grasp. These tools allow you to experiment with different strategies, see the results of your work in real-time, and track your progress over time. The more you engage with these features, the better you become at recognizing patterns and solving problems efficiently.
By incorporating interactive features into your study routine, you can make learning more enjoyable and enhance your ability to solve problems with confidence.
Strategies for Faster Calculations
Improving the speed of your calculations is essential for efficiency, especially when solving multiple problems within a limited time frame. With the right strategies and techniques, you can reduce the time spent on each problem while maintaining accuracy. Mastering these methods requires practice and understanding of various shortcuts, which can be applied across different problem types.
One effective strategy is to simplify expressions before performing calculations. This allows you to work with smaller, more manageable numbers. Additionally, recognizing patterns or applying known formulas can save significant time in more complex problems.
| Strategy | Benefit |
|---|---|
| Breaking down complex problems | Helps focus on one step at a time, reducing confusion and errors. |
| Using mental math | Allows for faster calculations without the need for writing everything down. |
| Recognizing common patterns | Quickly identifies the most efficient solution method for a problem. |
| Applying known shortcuts | Speeds up calculations for multiplication, division, and other operations. |
By practicing these strategies, you’ll be able to solve problems more quickly and confidently, ultimately improving your overall performance.
Understanding Algebraic Equations in Detail
Grasping the underlying structure of mathematical equations is essential for solving them effectively. Each equation represents a relationship between different variables, and understanding how these components interact allows you to find solutions more efficiently. The process involves recognizing patterns, simplifying expressions, and applying appropriate strategies to isolate the unknowns.
At the core of equation solving is the concept of balancing both sides. This means that whatever operation you perform on one side must also be performed on the other to maintain equality. By following this principle, you’ll be able to work through increasingly complex problems with confidence.
Key Elements of an Equation
Before diving into solutions, it’s important to identify the key parts of any equation:
| Element | Description |
|---|---|
| Variables | Symbols that represent unknown values (often denoted by x, y, z, etc.). |
| Constants | Fixed numbers that do not change in the equation. |
| Operators | Symbols that indicate mathematical operations, such as +, -, *, or /. |
| Equality Sign | Indicates that both sides of the equation are equal in value. |
Steps for Solving Equations
Solving equations involves a series of systematic steps to isolate the variable and find its value. Here is a general approach:
- Step 1: Simplify both sides of the equation, if possible.
- Step 2: Apply inverse operations to move constants and coefficients to the appropriate sides.
- Step 3: Solve for the variable by isolating it on one side.
- Step 4: Check your solution by substituting it back into the original equation.
By fully understanding the components and structure of equations, you’ll be equipped to tackle even the most challenging problems.
Boosting Your Test Performance
Achieving a high score on any assessment requires more than just knowing the material; it involves strategic preparation and effective time management. Whether you are working through practice exercises or facing an actual exam, optimizing your approach can make a significant difference. The key to success lies in adopting the right techniques to maximize your performance and minimize stress during the test.
One important factor in enhancing test results is building familiarity with the types of problems you’re likely to encounter. This allows you to approach each question confidently and efficiently. Additionally, developing strong problem-solving habits and staying organized during the exam can help prevent costly mistakes and save valuable time.
Key Strategies for Improving Test Results
Here are some effective methods to help you perform at your best:
- Practice Regularly: Consistent practice with different problem types ensures you’re well-prepared for any challenges that arise.
- Manage Your Time: Allocate time for each section and avoid spending too long on a single question.
- Stay Calm and Focused: Reducing anxiety can help you think clearly and make better decisions.
- Review Mistakes: After completing practice tests, review your errors and learn from them to avoid repeating them.
Effective Test-Day Habits
On the day of the assessment, having a clear and calm mindset is just as important as knowing the material. Here are a few habits that can boost your performance:
- Get Enough Rest: A good night’s sleep before the exam helps improve concentration and memory.
- Eat a Balanced Meal: Fuel your body with the right nutrients to keep your energy levels stable throughout the test.
- Read Instructions Carefully: Make sure you understand each question fully before beginning to solve it.
- Double-Check Your Work: If time allows, go over your answers to ensure accuracy and catch any mistakes.
By applying these techniques and maintaining a calm and focused mindset, you’ll significantly improve your performance and achieve the results you’re aiming for.
How to Check Your Work Effectively
Reviewing your work is a crucial step in ensuring accuracy and identifying any mistakes you might have overlooked. Checking your solutions carefully can help you catch errors before they affect your final result. The process of verification is not only about finding mistakes but also about solidifying your understanding of the material and improving your problem-solving skills.
When reviewing your calculations or answers, it’s important to approach the task with a clear strategy. Focus on both the details and the overall structure of your work to ensure consistency and precision. By following a systematic approach to checking, you can identify errors more easily and make the necessary corrections.
Effective Strategies for Reviewing Your Work
Here are some strategies that can help you verify your solutions efficiently:
- Retrace Your Steps: Go through each step of your process again, checking for any logical or arithmetic errors. This helps you identify where you may have gone off track.
- Use a Different Method: If possible, try solving the problem using an alternative method or approach. This can provide additional confirmation of your result.
- Check for Consistency: Ensure that your final answer is consistent with any given information or conditions in the problem statement.
- Work Backwards: For certain types of problems, retracing your solution from the final answer back to the beginning can help reveal errors.
Organizing Your Review Process
To make your review process more efficient, it’s helpful to establish a clear order for checking your work. Below is an example of an organized approach:
| Step | Action |
|---|---|
| Step 1 | Recheck all calculations and basic operations (addition, subtraction, multiplication, etc.) for accuracy. |
| Step 2 | Ensure all variables and constants are correctly substituted into the equation. |
| Step 3 | Verify that your solution makes sense in the context of the problem. |
| Step 4 | Cross-check the solution against the initial problem to confirm completeness. |
By following a structured approach to reviewing your work, you can improve your accuracy and reduce the likelihood of making avoidable mistakes. This practice not only helps you identify errors but also boosts your confidence in the correctness of your solutions.
Reviewing the Most Challenging Questions

Some problems can be particularly difficult, often requiring extra time and effort to solve. These questions may involve multiple steps, complex reasoning, or unfamiliar concepts. Reviewing these difficult problems allows you to better understand the underlying principles and identify where challenges arise. By breaking down tough questions and examining your approach, you can strengthen your skills and improve your performance on similar tasks in the future.
When faced with the most challenging problems, it’s essential to take a step-by-step approach. Instead of getting overwhelmed, focus on understanding each part of the problem individually. Revisit the problem after a short break if necessary, and don’t hesitate to seek alternative methods of solving. This process helps not only to identify errors but also to refine your problem-solving strategies for future success.
Key Strategies for Tackling Difficult Problems
- Break it Down: Break complex questions into smaller, more manageable parts. Focus on solving one piece at a time rather than trying to tackle the entire problem at once.
- Look for Patterns: Often, tough problems contain patterns or repetitions that can help you simplify the solution. Identify these patterns to make the process smoother.
- Consider Alternative Methods: If one approach isn’t working, try a different method. For example, switching from solving algebraically to graphically can provide fresh insights into the problem.
- Check Your Work: After solving a challenging problem, always go back and check your work. This ensures that you didn’t make any simple arithmetic or logical errors along the way.
How to Build Confidence in Challenging Questions

Building confidence in tackling difficult questions requires practice and patience. Here are some tips to help you become more comfortable with tough problems:
- Practice Regularly: The more often you engage with complex problems, the more confident you will become in solving them. Regular practice builds familiarity and comfort with the process.
- Review Mistakes: Don’t shy away from mistakes. Instead, review them thoroughly to understand where you went wrong and how to correct it in the future.
- Ask for Help: If you’re stuck on a problem, ask for guidance. A fresh perspective can often illuminate a solution you may have missed.
By reviewing and tackling the most challenging problems methodically, you can significantly improve your understanding and ability to solve difficult questions in the future. Remember, persistence and patience are key to overcoming challenges and mastering even the toughest tasks.
Tracking Your Progress in Mathematics
To truly master mathematical concepts, it’s crucial to monitor your improvement over time. Tracking your progress helps you recognize patterns, identify areas where you’re excelling, and pinpoint concepts that need more attention. By actively reflecting on your understanding and applying strategies to track growth, you gain a clearer sense of where to focus your efforts for continuous development.
Keeping track of your progress is an ongoing process. It can involve various methods such as reviewing past exercises, taking practice assessments, or noting your success in specific types of problems. Regular self-reflection and comparison of your current skills with earlier benchmarks provide valuable insights into your strengths and areas for improvement.
Methods for Tracking Your Growth
- Record Your Results: Keep a log of your performance on various exercises and activities. By reviewing this log periodically, you can measure your progress and spot patterns in your problem-solving approaches.
- Set Specific Goals: Create clear, achievable goals for improvement, such as mastering a particular type of problem or improving your speed. Breaking down larger goals into smaller milestones will help you track progress more effectively.
- Review Mistakes: When errors occur, take time to understand them rather than merely moving on. Mistakes often reveal areas of weakness that can be addressed to prevent future errors.
- Use Practice Exercises: Regular practice is key to mastering any skill. Use exercises to reinforce your knowledge and challenge yourself with increasingly complex problems to gauge your improvement.
How to Stay Motivated While Tracking Your Progress
Tracking progress isn’t only about identifying weaknesses–it’s also a powerful way to stay motivated. Seeing tangible growth, even if small, can encourage continued effort and increase confidence. Here are a few tips for maintaining motivation while monitoring your journey:
- Celebrate Small Wins: Acknowledge every milestone, whether it’s solving a challenging problem or improving your score on a practice assessment. Recognizing these victories keeps you motivated.
- Stay Consistent: Make tracking a routine part of your study process. The more consistently you monitor your progress, the more you’ll see the results of your effort over time.
- Be Patient: Improvement in any field takes time. Understand that mastering complex concepts may take several attempts, and perseverance is key.
By actively tracking your progress and adjusting your focus as needed, you’ll build a more solid foundation in mathematics. The process will guide you towards consistent improvement, helping you tackle increasingly challenging problems with confidence and skill.