Algebra with Pizzazz Worksheet Answers for Creative Learning

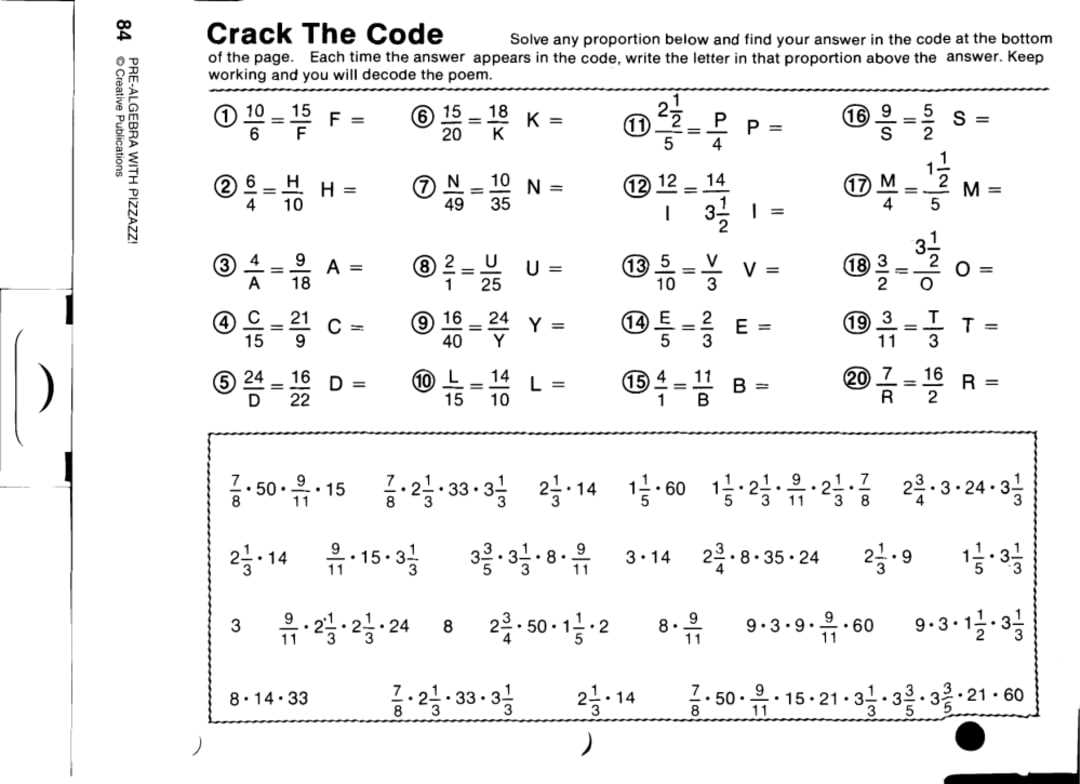
Mathematics can be both exciting and rewarding when approached through engaging and dynamic exercises. These activities are designed to help learners grasp fundamental concepts and develop critical problem-solving skills. They offer a hands-on approach to tackling complex topics, transforming abstract ideas into tangible learning experiences.

By working through carefully structured tasks, students can reinforce their understanding of essential mathematical principles. These exercises not only enhance comprehension but also foster creativity and independent thinking, allowing learners to connect theoretical knowledge to real-life situations.

Incorporating these activities into daily study routines provides a unique opportunity to deepen one’s mathematical proficiency while making the learning process more enjoyable. Whether practicing equations or exploring new concepts, these exercises empower students to confidently overcome challenges and build a strong foundation for future success.

Understanding Mathematical Exercises for Enhanced Learning

Engaging exercises are essential tools for grasping mathematical principles in a hands-on, interactive manner. These tasks are designed to present challenges that require critical thinking, helping learners develop the necessary skills to solve problems efficiently. By incorporating a variety of methods, these exercises allow students to approach topics from different angles, building a solid foundation for future learning.

Each exercise focuses on encouraging active participation, making abstract concepts more accessible. The structure of these activities promotes a deeper understanding, enabling students to connect theoretical knowledge with practical applications. As learners work through each step, they gain confidence and improve their ability to think analytically and solve complex problems.

Below is an example of how different types of tasks are organized, highlighting their role in reinforcing key concepts and promoting comprehension:

Type of Task	Description	Learning Outcome
Equation Solving	Focuses on finding the value of unknowns through step-by-step methods.	Enhances problem-solving abilities and understanding of operations.
Word Problems	Involves applying learned techniques to real-world scenarios.	Improves logical thinking and application of concepts in practical situations.
Graphing Exercises	Teaches the representation of equations visually to interpret relationships.	Strengthens spatial reasoning and understanding of data visualization.

These tasks not only promote learning but also encourage creativity in solving problems, making the educational process more enjoyable and effective. By actively participating in each activity, students develop a comprehensive understanding of key concepts while reinforcing their skills through practical experience.

How Creative Materials Enhance Learning

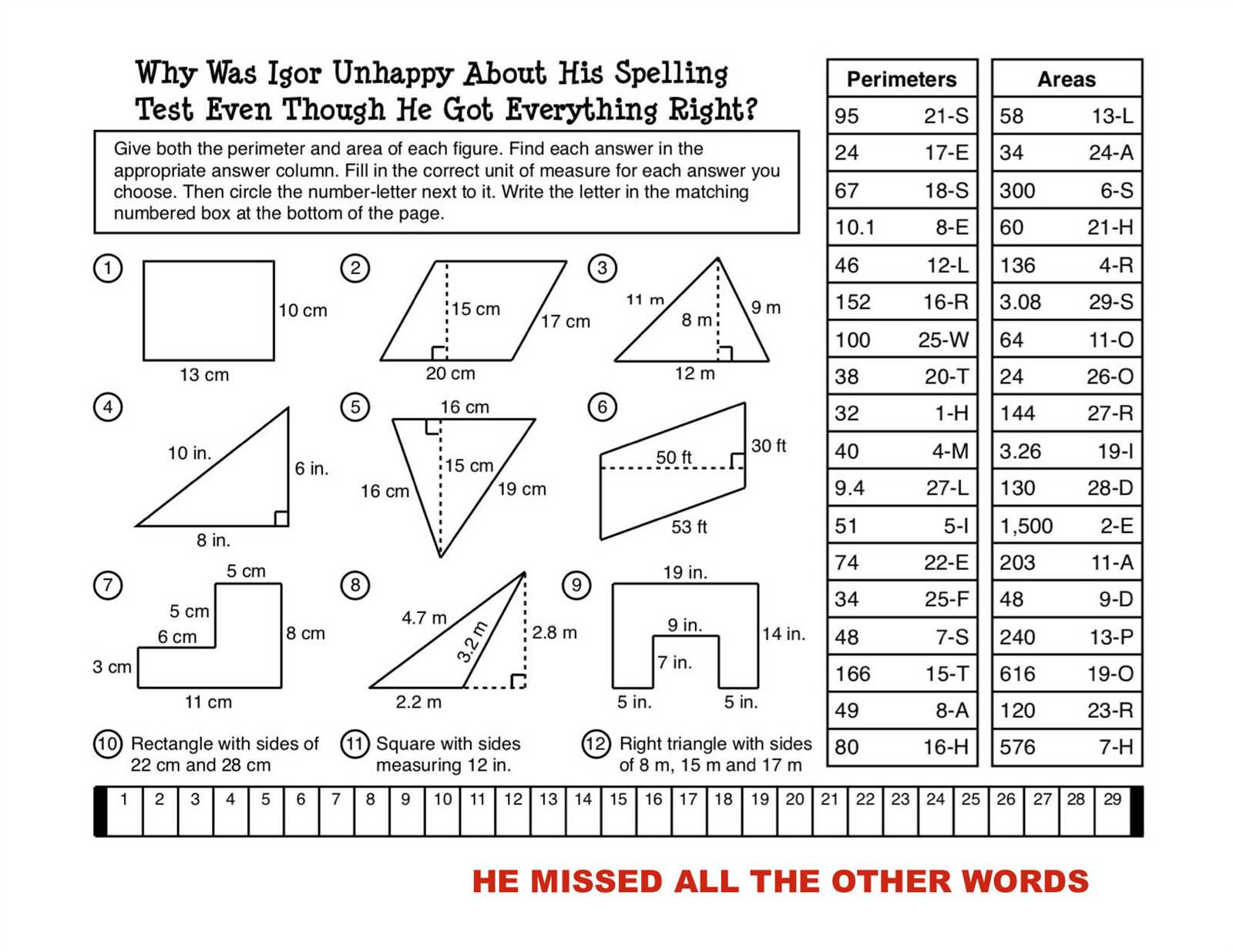
Engaging learning resources play a crucial role in making education more dynamic and accessible. These materials are designed to encourage active participation, turning traditional study methods into interactive and enjoyable experiences. By presenting information in novel and interesting formats, students are more likely to connect with the content and retain key concepts.

Fostering Engagement Through Visuals and Interactive Content

Incorporating visually appealing elements and interactive exercises into study resources helps learners stay engaged. These elements stimulate interest and provide students with multiple ways to interact with the material. Instead of just reading, they are encouraged to think critically, solve problems, and apply concepts in various contexts.

Visual aids support retention by presenting information in an easily digestible format.
Interactive challenges promote active thinking and problem-solving.
Colorful designs and fun themes make learning feel less like a task and more like a game.

Building Confidence and Critical Thinking Skills

Incorporating diverse approaches helps students build a deeper understanding of the subject matter. By approaching problems from different angles, learners are encouraged to think critically and develop confidence in their ability to solve complex issues independently.

Students gain confidence as they practice and improve their skills.
Different problem-solving strategies help develop analytical thinking.
Creative exercises challenge students to explore new ideas and test their abilities.

Ultimately, these materials create an environment where learning becomes more engaging, motivating students to keep exploring and mastering new concepts. With the right tools, students are empowered to take ownership of their education, leading to more effective learning outcomes and a greater sense of accomplishment.

Step-by-Step Solutions for Math Problems

Breaking down complex problems into manageable steps is essential for developing a strong understanding of mathematical concepts. This method not only clarifies the process but also allows learners to follow along and see the logical progression of each solution. Step-by-step guidance helps students identify key elements and apply the right techniques with confidence.

Understanding the Process from Start to Finish

By addressing each part of a problem individually, students can easily identify where mistakes may have occurred and correct them. This approach fosters a deeper understanding of how different mathematical principles interconnect and reinforces critical thinking skills.

Starting with a clear understanding of the problem helps in formulating an effective approach.
Solving one step at a time ensures no details are overlooked.
Reviewing the steps after completion enhances comprehension and retention.

Building Confidence with Practice and Reflection

As learners become more familiar with the problem-solving process, they develop a greater sense of confidence. Consistently practicing step-by-step methods allows students to internalize strategies, making it easier to tackle new challenges independently.

Repetition reinforces techniques and strengthens memory.
Reflecting on completed steps helps solidify knowledge.
Learning from mistakes encourages growth and resilience in problem-solving.

This structured approach ensures that learners not only complete problems successfully but also gain a deeper understanding of the concepts that drive them. By mastering each step, students are better prepared to tackle increasingly difficult tasks with confidence and ease.

Key Benefits of Using Exercises in Education

Incorporating structured exercises into learning offers a range of benefits that enhance the overall educational experience. These activities provide a focused way for students to practice skills, reinforce concepts, and assess their progress. By engaging with these materials, learners can develop both their knowledge and their ability to think critically.

Enhancing Understanding and Retention

One of the primary advantages of exercises is their ability to solidify understanding. By applying learned concepts to different problems, students strengthen their grasp of the material. This repeated exposure allows for better retention, making it easier to recall information when needed.

Repetition reinforces key ideas and techniques.
Active involvement helps deepen comprehension of complex topics.
Gradually increasing difficulty encourages mastery of concepts.

Promoting Independent Thinking and Problem-Solving

These activities not only support memorization but also promote independent thinking. Learners are encouraged to approach challenges on their own, fostering problem-solving skills and confidence. As they work through exercises, they become more capable of tackling unfamiliar questions and applying strategies to new situations.

Exercises develop critical thinking by requiring students to analyze and synthesize information.
Through practice, students learn to find solutions independently.
Engagement in diverse problem-solving tasks builds a strong foundation for future challenges.

In addition to improving academic performance, exercises contribute to a more active and personalized learning experience. Students are empowered to take control of their education, setting the stage for long-term success and a deeper connection to the material.

Boosting Student Engagement with Mathematics

Increasing student involvement in mathematics requires creating an environment where learning is both interactive and stimulating. By incorporating engaging activities and dynamic problem-solving opportunities, students are encouraged to actively participate and take ownership of their learning. This approach helps foster a deeper connection to the subject and motivates learners to continue exploring challenging topics.

Engagement thrives when learners can see the relevance of the material and feel empowered to tackle problems on their own. The use of varied strategies, such as hands-on tasks, collaborative projects, and real-world applications, enhances their interest and participation. As students discover new ways to approach and solve problems, they become more confident and excited about their progress.

Engagement Strategy	Impact on Learning	Example
Interactive Problem-Solving	Encourages active participation and collaboration.	Group discussions to solve complex challenges.
Real-Life Applications	Helps students see the relevance of mathematical concepts.	Using budgeting or shopping scenarios to apply calculations.
Game-Based Learning	Promotes friendly competition and boosts motivation.	Math-related games or quizzes to reinforce skills.

By incorporating these strategies, educators can create an environment where students are motivated to engage actively, think critically, and persist through challenges. This approach not only improves understanding but also makes learning an enjoyable and rewarding experience.

Approaching Complex Math Concepts Effectively

Mastering advanced mathematical topics often requires a strategic approach to ensure deep understanding and successful application. Breaking down intricate concepts into manageable steps is key to making them more accessible. By focusing on one element at a time, learners can build a solid foundation before moving on to more complex ideas. This gradual progression fosters confidence and allows students to grasp even the most challenging material.

Breaking Down Concepts Into Manageable Steps

One effective method for understanding complex problems is to dissect them into smaller, simpler parts. This allows learners to focus on each component individually, making it easier to understand how they fit together to form the whole. Starting with the basics and gradually building complexity is essential for long-term retention.

Start with the fundamentals: Ensure a solid grasp of basic principles before diving into more advanced material.
Use examples: Work through sample problems to reinforce learning and test understanding.
Take your time: Avoid rushing through complex topics; allow sufficient time for practice and reflection.

Fostering Confidence Through Practice and Application

Confidence grows as students practice and apply what they’ve learned. Regularly solving problems reinforces the concepts and shows students how their knowledge can be used to find solutions. The more learners engage with the material, the more comfortable they become with handling challenges and abstract concepts.

Practice regularly: Consistent problem-solving helps cement understanding.
Seek out real-world applications: Connect theoretical concepts to everyday scenarios to highlight their usefulness.
Reflect on progress: Review previous problems to see how much has been learned and identify areas for improvement.

By taking a structured and patient approach, students can navigate even the most difficult topics with greater ease and develop a lasting understanding that supports further learning.

Strategies for Mastering Math Skills

Mastering mathematical skills requires a combination of consistent practice, strategic thinking, and a clear understanding of core principles. By applying focused techniques, learners can break down complex topics into simpler concepts and gradually build their expertise. These strategies not only help students understand the material but also enhance their problem-solving abilities, paving the way for future success in mathematics.

Building a Strong Foundation

Before tackling advanced topics, it’s important to ensure a solid understanding of the basics. Strengthening the foundational knowledge allows students to tackle more complex problems with greater confidence and accuracy. Regular review and practice of core concepts are essential for long-term success.

Review key concepts: Revisit fundamental ideas regularly to reinforce understanding.
Understand the theory: Focus on the reasoning behind each method, not just the steps.
Identify patterns: Recognize recurring structures in problems to simplify the solving process.

Developing Problem-Solving Skills

Problem-solving is a crucial aspect of mastering any mathematical discipline. Developing an organized approach to solving problems helps students break down challenges into manageable parts, making it easier to find solutions. Practice is key, and students should aim to tackle a variety of problems to strengthen their skills.

Work through problems step by step: Break problems into smaller, easier-to-handle pieces.
Apply different strategies: Experiment with different methods of solving problems to find the most effective approach.
Analyze mistakes: Reflect on errors to understand where improvements can be made and learn from them.

Staying Consistent and Motivated

Consistency is essential for mastering any skill. Set aside regular time for practice and ensure that progress is tracked. Staying motivated through small successes and challenges will help keep momentum high and foster continued learning.

Set realistic goals: Break larger tasks into smaller, manageable objectives.
Celebrate progress: Acknowledge improvements to stay motivated.
Seek help when needed: Don’t hesitate to ask for assistance or consult resources when stuck.

By consistently applying these strategies, students can strengthen their understanding of mathematical concepts and improve their ability to tackle any challenge with confidence and skill.

Creative Approaches to Solving Mathematical Equations

Approaching mathematical equations with innovative techniques can make solving them more engaging and efficient. By using unconventional methods, students can gain a deeper understanding of the underlying concepts while improving their problem-solving skills. These approaches encourage a flexible mindset, allowing learners to explore various strategies to find the most effective solution for any given equation.

Visualizing Solutions with Diagrams

One creative approach to solving equations is visualizing them through diagrams or graphs. By plotting the equation on a coordinate plane, students can see the relationship between variables and gain insight into potential solutions. This method helps make abstract concepts more tangible and easier to comprehend.

Approach	Benefit	Example
Graphical Representation	Visualizes the equation, making patterns and solutions easier to identify.	Plotting a linear equation to find the point where it intersects the x-axis.
Flowcharts	Provides a structured approach to solving equations step by step.	Using a flowchart to break down the steps of solving a quadratic equation.
Number Lines	Helps with understanding solutions in terms of intervals or regions.	Using a number line to visualize the solution set of an inequality.

Using Real-Life Analogies

Another effective strategy is connecting mathematical problems to real-life situations. By using analogies, students can make sense of abstract equations in a more relatable way. For example, thinking of variables as quantities that need to be balanced can help simplify the process of solving for unknowns. These analogies turn equations into practical, everyday problems that students can easily grasp.

Balance approach: Imagine the equation as a scale where both sides must be equal.
Journey analogy: Consider each step of solving as progressing along a path or journey.
Container analogy: Treat variables like containers that need to be filled to a certain level.

These creative approaches not only enhance understanding but also foster a more enjoyable and interactive learning experience. By embracing diverse strategies, students can approach mathematical equations with confidence and creativity, ultimately strengthening their problem-solving abilities.

Encouraging Problem-Solving Through Practice Exercises

Providing students with structured practice opportunities is an effective way to develop their problem-solving skills. By engaging in carefully designed activities, learners can refine their techniques and build a deeper understanding of mathematical concepts. These exercises not only reinforce knowledge but also promote critical thinking and the ability to apply learned strategies in various scenarios.

Building Confidence Through Step-by-Step Practice

When students encounter a challenging problem, breaking it down into smaller, manageable steps can help them stay focused and confident. Structured exercises guide students through the process, encouraging them to think critically about each phase of the solution. This step-by-step approach builds confidence and allows learners to tackle increasingly difficult problems with greater ease.

Break down complex problems: Divide problems into smaller sections to make them less overwhelming.
Highlight key steps: Emphasize important stages in the problem-solving process for clarity.
Provide hints when necessary: Offer subtle prompts to encourage independent thought while still guiding students.

Fostering Critical Thinking and Creativity

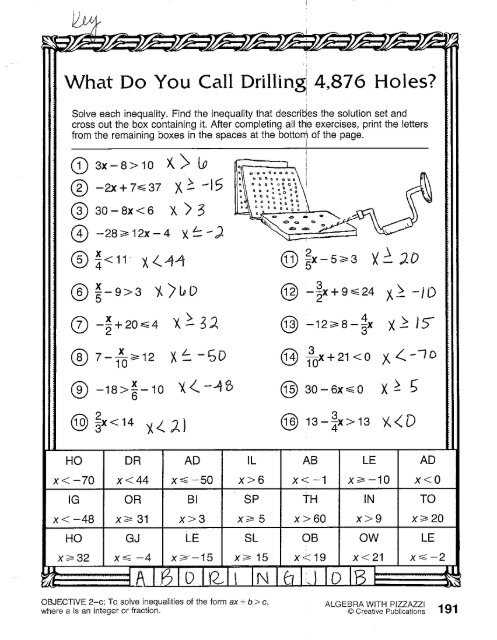
Beyond simple practice, exercises also challenge students to think creatively and develop new strategies for solving problems. By encouraging learners to explore multiple solutions or approaches, educators can help students expand their problem-solving toolbox. This creative thinking fosters resilience, as students learn to approach unfamiliar problems from different angles.

Encourage alternative methods: Prompt students to consider different ways to solve a problem.
Allow room for exploration: Provide open-ended exercises that require students to think critically and test their ideas.
Promote collaborative problem-solving: Allow students to work together, sharing ideas and learning from each other’s approaches.

By incorporating problem-solving exercises into the learning process, educators help students develop not only their technical abilities but also their confidence, creativity, and resilience in the face of challenges.

Common Challenges in Mathematical Education

In the process of teaching mathematics, several obstacles often arise that hinder student progress. Many learners struggle with abstract concepts, while others may find it difficult to connect new material with prior knowledge. Identifying and addressing these challenges is essential to ensuring students develop a solid understanding of fundamental mathematical principles. By recognizing common difficulties early, educators can tailor their teaching strategies to better meet the needs of their students.

Difficulty Grasping Abstract Concepts

One of the primary challenges students face is understanding abstract concepts that do not have clear visual representations. These topics can be intimidating, as students are often required to work with symbols and operations that seem disconnected from the real world. As a result, students may feel overwhelmed or frustrated, making it difficult for them to see the relevance of the material.

Make connections to real-life scenarios: Relating abstract ideas to familiar situations helps students understand their practical applications.
Use visual aids: Diagrams, graphs, and models can make abstract concepts more concrete and accessible.
Introduce concepts gradually: Break down complex ideas into simpler, more digestible pieces to reduce cognitive overload.

Difficulty Applying Knowledge to New Problems

Another challenge students often face is applying previously learned concepts to solve unfamiliar problems. While they may perform well on routine exercises, they may struggle when faced with more complex or novel situations. This difficulty stems from the need to adapt existing knowledge to new contexts, requiring flexibility and problem-solving skills that take time to develop.

Practice with varied examples: Provide students with a range of problem types to encourage flexible thinking.
Focus on critical thinking: Encourage students to approach problems methodically and reflect on their strategies.
Provide opportunities for exploration: Allow students to experiment with different approaches to build their confidence in solving diverse problems.

By acknowledging and addressing these common challenges, educators can help students build a more resilient and adaptable mindset, making them better equipped to tackle increasingly difficult mathematical problems.

How to Use Solutions for Practice

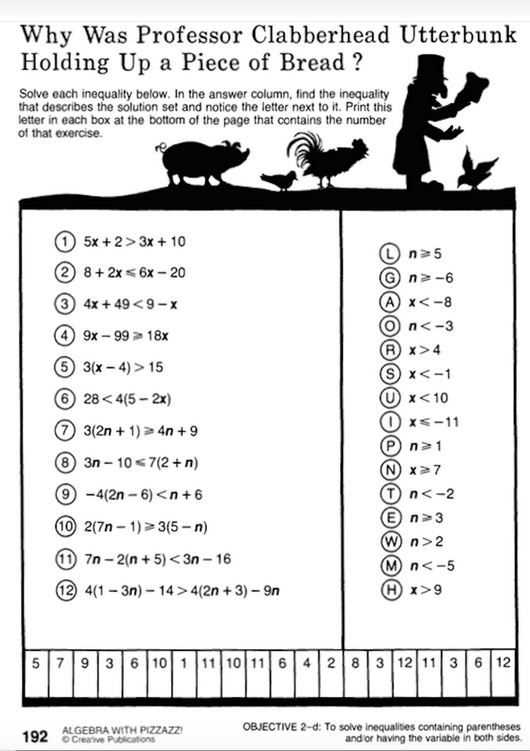
Using solutions as a tool for practice is an effective way to reinforce learning and build problem-solving skills. When students work through problems and later check their results, they gain valuable insights into the methods and strategies that lead to correct answers. By analyzing each step of the solution process, learners can identify areas of strength and areas that require improvement, allowing them to focus on specific aspects of their learning.

Understanding the Process Behind the Solution

One of the most effective ways to use solutions for practice is by breaking down each step of the process. Rather than simply memorizing the final result, students should focus on understanding how the solution is reached. This approach not only solidifies their understanding of the material but also helps them develop the critical thinking skills necessary to solve similar problems independently.

Review each step: Pay close attention to how the problem is approached and solved at each stage.
Understand the logic: Focus on the reasoning behind each step, not just the operations performed.
Identify patterns: Recognize recurring methods or strategies that can be applied to other problems.

Using Solutions for Self-Assessment

Another valuable way to use solutions is for self-assessment. After attempting a problem on your own, compare your approach with the provided solution. If there are discrepancies, it’s important to analyze where things went wrong and adjust your approach accordingly. This process encourages self-reflection and helps identify areas where additional practice is needed.

Compare your solution: Check your method against the provided steps to identify any mistakes.
Ask questions: If a solution seems unclear, try to rework the problem to better understand each part.
Track progress: Keep a record of areas that you find challenging and review them regularly to improve.

By using solutions to guide your practice, you can not only improve your understanding of key concepts but also develop greater confidence in tackling complex problems.

Improving Understanding of Mathematical Operations

To develop a deeper comprehension of mathematical operations, it is essential to focus on building a strong foundation in the core principles. Mastering these fundamental skills allows students to handle more complex problems with confidence. By practicing a variety of methods and approaches, learners can solidify their understanding and improve their ability to apply these operations in different contexts.

One effective approach is to break down operations into smaller, more manageable steps. This allows students to focus on the individual components of each process before putting them together into a larger solution. In addition, consistently practicing these operations helps students build muscle memory, making it easier to recall the necessary steps when tackling problems in the future.

Another valuable strategy is to relate operations to real-world scenarios. By applying mathematical concepts to everyday situations, students can better understand their practical applications. This connection helps to reinforce the importance of mastering these skills and provides motivation for continued practice and improvement.

In summary, improving one’s understanding of mathematical operations involves a combination of clear explanations, consistent practice, and making real-world connections. By focusing on these areas, students can enhance their skills and build the confidence they need to succeed in more advanced topics.

How to Stay Motivated While Studying Mathematics

Maintaining motivation while studying can be challenging, especially when the material feels difficult or overwhelming. However, developing strategies to stay focused and engaged can make the learning process more enjoyable and productive. A positive mindset and consistent effort can help overcome obstacles and foster long-term success in mastering new concepts.

One way to stay motivated is by setting clear and achievable goals. Break down larger tasks into smaller, more manageable steps, and celebrate each milestone along the way. This not only provides a sense of accomplishment but also helps to keep progress visible, which can be highly motivating.

Another effective method is to vary your study techniques. Try mixing different activities such as solving problems, watching educational videos, or discussing concepts with peers. By changing the way you engage with the material, it prevents monotony and keeps things interesting. You can also apply concepts to real-life scenarios, making the learning experience more relevant and rewarding.

Lastly, it’s important to maintain a balance between work and rest. Taking regular breaks, practicing mindfulness, or engaging in physical activity can help refresh your mind, improve focus, and reduce stress. Consistent breaks and a healthy study routine contribute to a more productive and enjoyable learning experience.

Creating a Positive Learning Experience

A positive learning environment is essential for fostering both engagement and understanding. When students feel comfortable and motivated, they are more likely to approach new challenges with confidence and enthusiasm. Creating such an environment requires a combination of effective teaching strategies, encouragement, and a supportive atmosphere that caters to diverse learning needs.

Key Factors for Success

Active Engagement: Encouraging active participation through interactive activities, discussions, and problem-solving exercises helps keep students focused and involved.
Clear and Positive Communication: Providing clear instructions and feedback in a constructive and encouraging manner builds trust and keeps students motivated.
Personalized Learning: Recognizing the unique learning styles and abilities of each student allows for tailored approaches, which enhances overall comprehension and retention.

Building Confidence Through Support

Frequent Encouragement: Acknowledge progress and provide positive reinforcement to help build students’ self-confidence and reduce fear of mistakes.
Safe Learning Space: Creating a non-judgmental environment where students feel free to ask questions and make mistakes promotes a growth mindset.
Constructive Feedback: Offering feedback that focuses on improvement rather than criticism helps students learn from their mistakes and strive for continuous progress.

In summary, a positive learning experience is achieved through a mix of interactive teaching methods, clear communication, personalized support, and a nurturing environment. These elements work together to create an atmosphere that motivates students to reach their full potential and embrace challenges with confidence.

Connecting Algebra to Real-World Applications

Bringing mathematical concepts into practical use is essential for helping students understand the value of their studies. By connecting abstract ideas to real-life scenarios, learners can better grasp how these principles are used in everyday situations. Whether in business, technology, or personal finances, the skills acquired through solving equations can play a significant role in solving actual problems.

For example, consider the world of finance, where mathematical concepts are crucial for budgeting, calculating interest, and predicting future expenses. By modeling financial situations, students can visualize how math plays a role in making informed decisions. Similarly, industries such as construction and engineering rely on these skills to ensure accurate measurements, design structures, and optimize resources efficiently.

Incorporating hands-on projects and real-world case studies into the curriculum also fosters critical thinking and problem-solving abilities. Students can apply what they’ve learned to tackle practical challenges, which not only reinforces the material but also builds confidence in their ability to use math beyond the classroom. This connection to the real world shows how mathematical thinking extends far beyond academic exercises and is a powerful tool in navigating modern life.

Maximizing the Impact of Algebra Worksheets

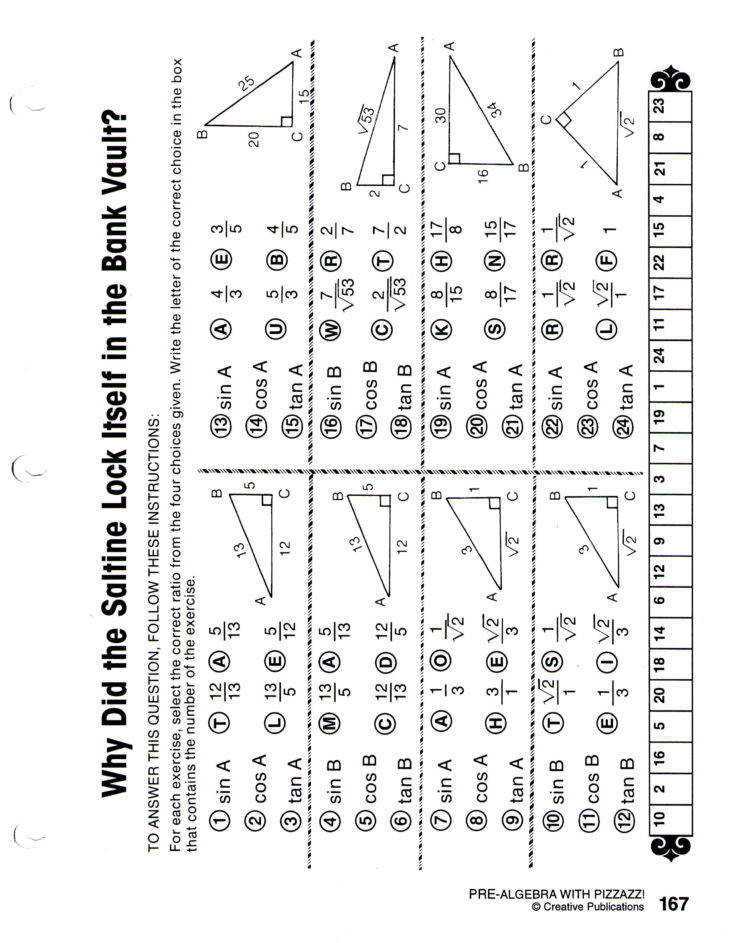
To fully harness the potential of practice sheets in mathematics education, it is essential to employ strategies that engage students and help them understand the underlying principles. Effective use of these tools goes beyond simple completion; it involves integrating them into a comprehensive learning plan that encourages mastery and promotes retention.

One of the most effective ways to maximize the impact of these resources is through a structured approach. Here are several key strategies:

Start with the Basics: Begin by reinforcing foundational concepts before introducing more complex problems. This ensures that students are well-prepared for more challenging material.
Use a Variety of Problems: Incorporate a mix of question types, ranging from basic to advanced, to cater to different skill levels and encourage critical thinking.
Encourage Self-Reflection: After completing each set of exercises, encourage students to review their answers and identify any mistakes. This fosters a deeper understanding of the material.
Integrate Real-Life Applications: Relating problems to real-world scenarios makes the material more relevant and shows students the practical value of what they are learning.

By consistently using these techniques, students can improve their problem-solving skills, develop confidence in their abilities, and gain a deeper understanding of the concepts being taught. This approach helps to maximize the effectiveness of practice materials and ensures that students get the most out of their learning experience.