Mathematical challenges often require a deep understanding of core principles and the ability to apply them in various scenarios. Whether dealing with simple equations or more complex expressions, developing problem-solving skills is key to achieving success. In this section, we will explore effective strategies to approach and solve a wide range of mathematical tasks.
Problem-solving in mathematics involves more than just memorizing formulas; it requires critical thinking and the ability to break down complex problems into manageable steps. By focusing on logical reasoning and step-by-step procedures, students can build confidence in tackling difficult questions.
For many, these problems seem daunting at first, but with the right methods and plenty of practice, anyone can improve their understanding. This guide will provide insights into how to effectively approach and solve math-related tasks, enhancing both comprehension and performance in this subject area.
Understanding Mathematical Problem Solving
Mastering mathematical tasks requires more than just knowing formulas; it involves understanding the core principles that underpin each problem. The approach to solving these tasks often revolves around breaking down complex concepts into simpler steps. Developing a strong foundation in this area is essential for success, as it helps students to tackle progressively more difficult problems with confidence.
Building Strong Foundations
When faced with challenging mathematical tasks, it’s crucial to first grasp the fundamental concepts. These concepts act as building blocks for solving more advanced problems. By practicing basic operations and learning how to recognize patterns, students can strengthen their problem-solving abilities and enhance their analytical thinking.
Approaching Complex Problems
As the complexity of the tasks increases, so does the need for strategic thinking. Tackling these problems involves a step-by-step method that ensures accuracy and reduces the risk of errors. Understanding the different types of equations, their structure, and the relationships between variables makes it easier to identify the most efficient path to a solution.
Key Concepts in Mathematical Problem Solving

To successfully navigate mathematical tasks, it’s essential to understand the foundational concepts that form the basis for most problems. These concepts provide the framework needed to approach, solve, and check for accuracy in more complex challenges. A solid grasp of these principles is critical for building problem-solving skills that can be applied in various scenarios.
Essential Mathematical Principles

There are several key ideas that help guide learners through various mathematical challenges. Understanding these basic principles is the first step in mastering more advanced topics. Some of the core concepts include:
- Equations and Expressions – Learning how to manipulate and solve different types of mathematical statements.
- Variables and Constants – Recognizing the role of unknowns and fixed values in mathematical models.
- Operations and Properties – Mastering the fundamental operations (addition, subtraction, multiplication, division) and understanding their properties.
Advanced Problem-Solving Techniques
Once the foundational concepts are clear, it’s important to explore more advanced techniques to solve increasingly complex tasks. These techniques may include:
- Factoring and Expanding – Breaking down expressions into simpler forms to make them easier to solve.
- Graphing – Visualizing relationships between variables to better understand solutions.
- Substitution and Elimination – Using these strategies to solve systems of equations efficiently.
Step-by-Step Approach to Problem Solving
Breaking down complex tasks into smaller, manageable steps is key to finding solutions with precision and confidence. This approach ensures that each aspect of the problem is understood and addressed, minimizing errors and improving accuracy. A structured method not only helps in solving a single problem but also strengthens overall problem-solving abilities.
One effective way to approach a problem is by following a systematic process. This process can be applied to various types of mathematical challenges and involves evaluating the problem, identifying relevant strategies, and applying the correct operations. The table below outlines a typical step-by-step strategy:
| Step | Action | Description |
|---|---|---|
| 1 | Understand the Problem | Read through the task carefully and determine what is being asked. |
| 2 | Identify Key Information | Highlight important numbers, variables, and relationships within the problem. |
| 3 | Choose a Strategy | Select an appropriate method or formula to apply to the problem. |
| 4 | Execute the Plan | Carry out the necessary calculations or operations step-by-step. |
| 5 | Review and Check | Double-check the solution to ensure it makes sense and is accurate. |
Common Mistakes in Mathematical Problem Solving
Even the most skilled individuals can fall into the trap of making simple errors while solving mathematical tasks. These mistakes can often lead to incorrect conclusions, despite understanding the underlying concepts. Recognizing these common pitfalls is an important step in improving accuracy and developing better problem-solving strategies.
One frequent mistake is rushing through calculations without paying attention to detail. This can lead to misplacing decimal points, applying the wrong operations, or neglecting signs in expressions. Another common error is misunderstanding the problem’s requirements, which can result in using an inappropriate method to find the solution. Additionally, overlooking intermediate steps and skipping checks for accuracy can cause small errors to go unnoticed, ultimately affecting the final result.
Effective Time Management for Math Tasks
Managing time effectively is crucial when tackling complex mathematical problems. Without a clear plan, it’s easy to feel overwhelmed, leading to rushed solutions or incomplete tasks. A structured approach to time management ensures that each problem is given the attention it needs, while also allowing for breaks to maintain focus and productivity.
Setting Realistic Goals
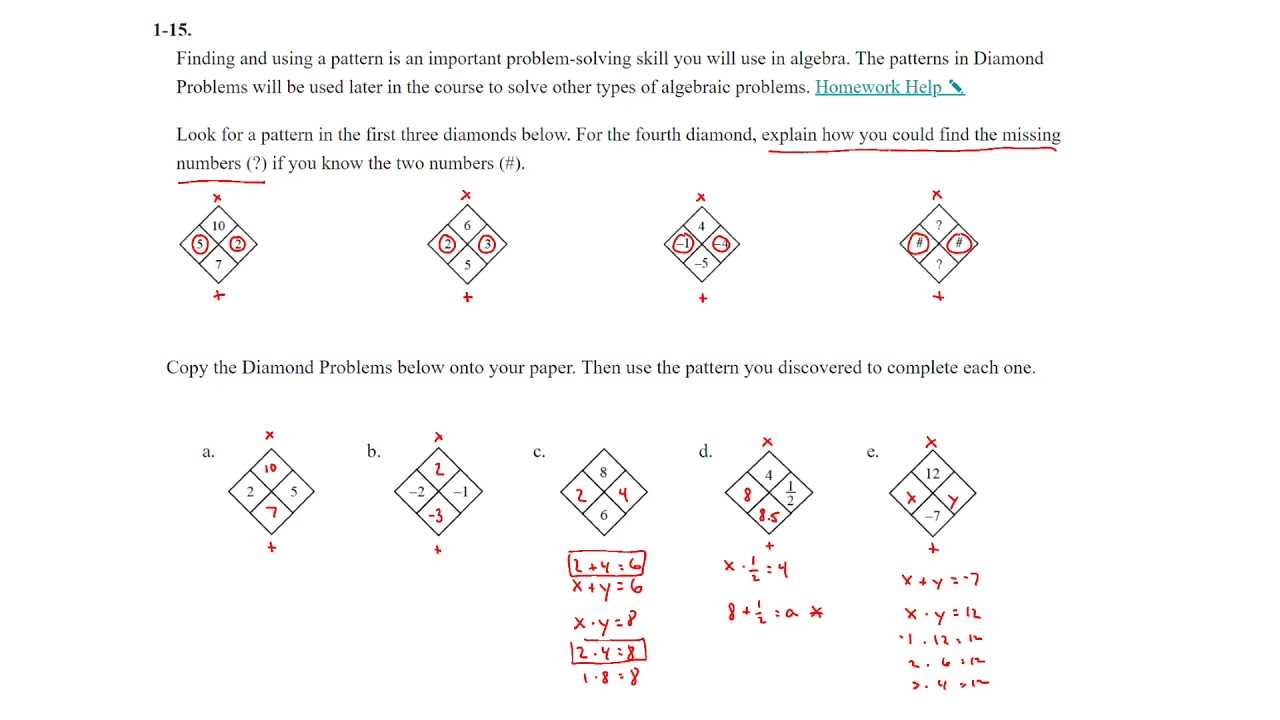
Before starting any set of problems, it’s important to set clear, achievable goals. This can include determining how much time to spend on each task or identifying specific objectives for each session. By breaking down the overall workload into smaller, manageable parts, you can track progress more effectively and avoid feeling overwhelmed.
Prioritizing Tasks

Some tasks may be more challenging than others, requiring extra time and effort. Prioritize the more difficult problems first, allowing more time to work through complex steps. Once the harder tasks are completed, the easier ones will feel less time-consuming and can be finished more quickly.
How to Use Mathematical Formulas
Formulas are essential tools that simplify complex problems by providing a structured way to relate different quantities. Knowing how and when to apply these formulas can greatly enhance problem-solving efficiency. Understanding the underlying concepts behind each formula ensures that it is used correctly and in the right context.
To effectively use mathematical formulas, follow these key steps:
- Identify the Formula – Recognize which formula is needed based on the given problem. Familiarize yourself with various formulas, such as those for area, volume, or rate.
- Understand the Variables – Carefully identify each variable within the formula and understand what they represent in the context of the problem.
- Substitute Values – Replace the variables with their respective values from the problem. Ensure the units match, and check that the values are correct before proceeding.
- Perform the Calculation – Carefully carry out the operations required by the formula. Be sure to follow the order of operations to avoid errors.
- Check the Result – After obtaining a result, verify that it makes sense in the context of the problem and that the units are consistent.
By following these steps, you can apply formulas effectively to a wide range of problems, ensuring accuracy and clarity in your solutions.
Tips for Tackling Word Problems
Word problems can often seem intimidating due to the combination of narrative text and mathematical operations. However, breaking them down into manageable steps can help clarify the situation and reveal the necessary steps to find a solution. By following a structured approach, you can efficiently translate the text into a solvable problem.
Here are some helpful strategies for approaching word-based mathematical challenges:
- Read the Problem Carefully – Take time to fully understand the scenario being described. Pay close attention to the details, as they often contain critical information.
- Identify Key Information – Highlight or underline important numbers, terms, and relationships that will help form the mathematical model of the problem.
- Translate Words into Numbers – Convert the descriptions into mathematical expressions or equations. Look for phrases that indicate operations like “sum,” “difference,” “product,” or “quotient.”
- Set Up an Equation – Based on the key information, write an equation or set of equations that represent the problem. This will serve as your roadmap to the solution.
- Solve Step-by-Step – Approach the equation methodically, solving one step at a time. This ensures you don’t overlook important details along the way.
- Double-Check Your Work – After reaching a solution, review the problem and your work to ensure the result makes sense in the context of the original scenario.
By following these tips, you can gain confidence in solving word problems, transforming them from challenging puzzles into manageable tasks.
Mastering Linear Equations with Ease
Linear equations form the foundation of many mathematical problems and are crucial for solving real-world scenarios. With the right approach, these equations can be tackled efficiently and accurately. By understanding the core principles behind linear equations, you can solve them quickly and with confidence, whether dealing with simple or more complex scenarios.
Steps to Solve Linear Equations
The process of solving linear equations can be broken down into clear, manageable steps. Following these steps will help simplify even the most challenging problems:
| Step | Action | Description |
|---|---|---|
| 1 | Identify the Equation | Recognize the form of the equation (e.g., ax + b = c) and determine the variables and constants involved. |
| 2 | Isolate the Variable | Move all terms with the variable to one side of the equation and constants to the other side. |
| 3 | Simplify the Expression | Combine like terms and simplify any fractions or decimals to make the equation easier to solve. |
| 4 | Solve for the Variable | Perform the necessary operations (addition, subtraction, multiplication, division) to isolate the variable completely. |
| 5 | Check the Solution | Substitute the value of the variable back into the original equation to verify the solution. |
Common Techniques for Solving Linear Equations
To master linear equations, it’s helpful to become familiar with a few key techniques:
- Elimination Method – Used when solving systems of equations, this technique involves adding or subtracting equations to eliminate one variable.
- Substitution Method – Involves solving one equation for a variable and substituting this value into the other equation.
- Graphing – Graphing both equations on a coordinate plane to find their point of intersection, which is the solution to the system.
By practicing these methods, you can quickly become proficient at solving linear equations and gain confidence in your ability to tackle mathematical challenges.
Understanding Polynomials in Mathematics
Polynomials are a fundamental concept in mathematics, representing expressions with multiple terms that involve variables raised to different powers. These expressions are commonly encountered in various problem-solving scenarios and are key to understanding more advanced mathematical topics. Recognizing the structure of polynomials and knowing how to work with them is crucial for building a strong mathematical foundation.
At their core, polynomials are made up of terms connected by addition or subtraction, where each term consists of a constant multiplied by a variable raised to a specific power. Understanding how to identify, classify, and manipulate these expressions is the first step toward mastering their use in solving problems.
Key Features of Polynomials
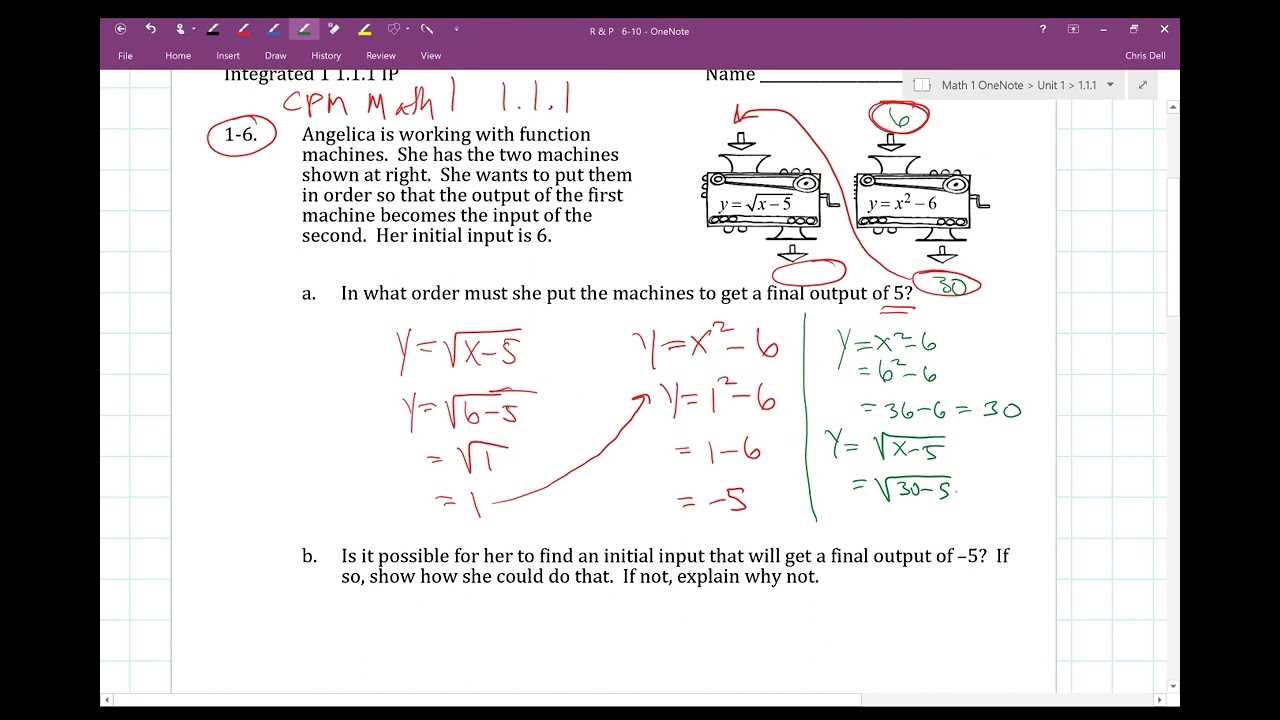
- Terms – A polynomial is made up of one or more terms. Each term includes a coefficient (a constant) and a variable raised to a power.
- Degree – The degree of a polynomial is determined by the highest power of the variable. For example, in the polynomial 3x² + 2x + 5, the degree is 2.
- Coefficients – These are the numerical factors in each term of the polynomial. They determine the weight of each term in the expression.
- Constant Term – This is the term that does not contain any variables, such as the 5 in the example above.
Common Operations with Polynomials
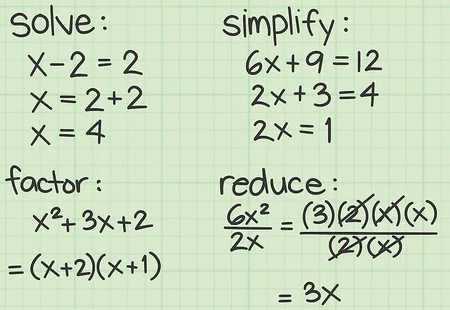
Once the basic components of polynomials are understood, there are several key operations that can be performed:
- Adding and Subtracting – Combine like terms to simplify the polynomial. Like terms have the same variable and exponent.
- Multiplying – Multiply each term in one polynomial by each term in another polynomial, applying the distributive property.
- Factoring – Factor a polynomial to break it down into simpler expressions, often to solve equations or simplify calculations.
- Evaluating – Substitute specific values for the variables in the polynomial and perform the arithmetic to find the result.
Mastering these operations will enable you to solve a wide range of mathematical problems and provide a solid foundation for exploring more advanced topics. Polynomials are a versatile tool, used in everything from basic algebraic manipulation to calculus and beyond.
Strategies for Solving Quadratic Equations
Quadratic equations are an essential part of mathematics, appearing in various real-world applications and higher-level problem-solving. These equations involve a variable raised to the second power and can be solved through a variety of methods, depending on the complexity of the equation. By understanding the different strategies available, you can confidently approach these types of problems and find the solutions more efficiently.
There are several tried-and-true techniques for solving quadratic equations, each suited to different scenarios. Whether you’re dealing with simple or more complex forms, choosing the right approach can simplify the process and lead to quicker solutions.
Methods for Solving Quadratic Equations
- Factoring – This method involves expressing the quadratic equation as the product of two binomials. It’s useful when the equation can be easily factored into integer values.
- Completing the Square – In this approach, you rewrite the equation to make one side a perfect square trinomial, allowing you to solve for the variable by taking the square root.
- Quadratic Formula – The quadratic formula is a universal method that can be applied to any quadratic equation. The formula, x = (-b ± √(b² – 4ac)) / 2a, provides the roots of the equation when it’s in standard form ax² + bx + c = 0.
- Graphing – By plotting the equation on a graph, you can find the points where the curve intersects the x-axis. These points are the solutions to the equation.
Choosing the Right Method
Each method has its advantages and is best suited to different types of equations. Factoring is often the quickest method when the equation can be factored easily. Completing the square is useful when the equation is not easily factorable but still manageable. The quadratic formula works in all cases, especially when other methods are more difficult or time-consuming. Graphing, while not always as precise, can provide a quick visual understanding of the solutions.
By practicing these strategies, you will become more adept at solving quadratic equations and build confidence in tackling increasingly complex mathematical challenges.
Common Mathematical Resources for Learning and Practice
When working through mathematical problems, it is essential to have access to reliable resources that can support understanding and offer guidance. There are a variety of tools and materials available, ranging from online platforms to textbooks, that help reinforce concepts, clarify doubts, and provide extra practice. By using these resources effectively, learners can improve their skills and tackle challenges more confidently.
These resources come in different formats, allowing students to choose what best suits their learning style. From interactive websites to step-by-step guides, there are plenty of ways to enhance your understanding and gain new insights into solving problems.
Types of Useful Resources
| Resource Type | Description | Examples |
|---|---|---|
| Online Tutorials | Interactive lessons and video tutorials provide visual explanations and step-by-step guides for solving problems. | Khan Academy, Coursera, YouTube channels |
| Practice Worksheets | Printable or online worksheets with practice problems allow for additional practice and skill reinforcement. | Mathway, Mathisfun, WorksheetWorks |
| Math Forums | Online communities where students can ask questions and get help from peers or experts in mathematics. | Stack Exchange, Reddit (r/learnmath), Math Help Forum |
| Textbooks | Traditional textbooks offer a structured approach to learning, with a comprehensive range of exercises and explanations. | Elementary and Intermediate Algebra by Lial, Sullivan, or open textbooks from OpenStax |
| Mobile Apps | Apps that provide instant solutions, practice, and tutorials for learning concepts on the go. | Photomath, Wolfram Alpha, Microsoft Math Solver |
Using these resources regularly not only helps in mastering difficult concepts but also builds a deeper understanding of mathematical theory and application. Whether you prefer learning visually, through reading, or by practicing problems, the right resources can significantly enhance your learning experience.
Using Online Tools for Mathematical Help
In the digital age, students have access to a wide variety of online tools that can assist with solving mathematical problems, providing explanations, and reinforcing learning. These platforms offer an interactive and convenient way to tackle complex problems outside of the classroom environment. By utilizing these online resources, learners can get personalized assistance and enhance their understanding of key concepts.
Online tools come in many forms, from step-by-step problem solvers to video tutorials, offering flexibility for different learning styles. Whether you’re working on simple calculations or advanced problems, these tools can guide you through the process and offer immediate feedback.
Types of Online Tools
- Interactive Problem Solvers: These tools allow you to enter a problem and get a detailed solution with explanations, making them ideal for understanding each step of the process.
- Math Learning Websites: Dedicated websites provide free lessons, practice exercises, and quizzes to help reinforce learning and provide additional practice.
- Video Tutorials: Platforms like YouTube and Khan Academy offer visual demonstrations of concepts and problem-solving techniques that can be paused and rewatched for clarity.
- Graphing Calculators: Online graphing tools help you visualize functions and equations, making it easier to understand their behavior and solutions.
- Math Forums: Online communities and forums are excellent places to ask questions and get help from both peers and experts in mathematics.
Benefits of Using Online Resources
- Instant Feedback: Online tools provide immediate feedback, helping you quickly identify mistakes and understand how to correct them.
- Personalized Learning: Many platforms offer tools to track your progress, suggest areas for improvement, and adapt to your learning pace.
- Access Anywhere: Online tools can be accessed from any device with an internet connection, making them available at all times, whether at home or on the go.
- Variety of Learning Styles: Whether you prefer written explanations, visual aids, or interactive quizzes, there is an online tool suited to your preferred way of learning.
By incorporating online tools into your study routine, you can gain a deeper understanding of mathematical principles and enhance your problem-solving abilities. With the vast array of resources available, mastering complex concepts has never been more accessible.
The Role of Practice in Mastery

Achieving mastery in any skill requires consistent effort and repetition. In mathematics, the more problems you solve, the better you become at understanding the underlying concepts. Practice helps to reinforce learning, identify areas of weakness, and build confidence. It transforms theoretical knowledge into practical ability, allowing you to apply what you’ve learned effectively.
Regular practice ensures that complex problems become more manageable over time, and the process of solving these problems becomes increasingly intuitive. It also helps in recognizing patterns, which is key to tackling more advanced topics. The more you engage with the material, the clearer and more automatic the problem-solving steps will become.
Why Practice is Essential
- Improves Retention: The act of solving problems regularly reinforces memory and helps retain information in the long term.
- Increases Speed: With consistent practice, you become faster at recognizing problem types and applying the appropriate methods.
- Builds Confidence: Successfully solving problems boosts your self-assurance and reduces anxiety when approaching more challenging exercises.
- Enhances Problem-Solving Skills: Repeated exposure to various types of problems enhances your ability to think critically and approach challenges from different angles.
Effective Practice Techniques
- Start with Basics: Begin with simpler problems to build a solid foundation before progressing to more complex ones.
- Mix Problem Types: Practice a variety of problem types to ensure a well-rounded understanding of concepts and techniques.
- Review Mistakes: Analyze any errors made during practice to understand where you went wrong and learn from them.
- Set Consistent Goals: Establish specific goals for each practice session to stay focused and track your progress.
By incorporating regular practice into your routine, you can effectively master complex topics and approach new challenges with ease. The key is consistency and a willingness to learn from each attempt, turning practice into a powerful tool for success.
How to Check Your Solutions
Verifying your solutions is a crucial part of problem-solving in mathematics. Once you have arrived at a potential answer, it’s important to confirm that it is correct. This process not only helps ensure accuracy but also reinforces your understanding of the concepts at hand. Checking your work allows you to catch any potential mistakes and provides a deeper insight into the solution process.
There are several methods to validate your solutions, ranging from simple substitution to more advanced techniques. Regardless of the complexity of the problem, always take the time to check and confirm your results. This practice not only helps in academic settings but also strengthens problem-solving skills for real-life applications.
Methods to Verify Your Results

- Substitute the Solution: For equations, substitute your solution back into the original problem. If both sides of the equation are equal, your answer is correct.
- Use a Different Approach: Solve the problem using an alternative method, if possible, and compare the results. This can help identify any errors in the first approach.
- Estimate the Answer: Before solving, estimate the expected range for the answer. After solving, compare the result with your estimation to see if it makes sense.
- Check for Logical Consistency: Ensure that each step of your solution follows logically from the previous one. If something doesn’t seem right, retrace your steps.
Common Mistakes to Watch For
- Sign Errors: Double-check your positive and negative signs throughout the problem, as sign errors are a common mistake.
- Misinterpreting the Problem: Ensure you fully understand the problem’s requirements before beginning. Sometimes errors arise from misreading or misunderstanding the question.
- Skipping Steps: Avoid rushing through the steps. Missing even a small step can lead to incorrect results.
- Wrong Units: When dealing with measurements or units, ensure you are using the correct ones and converting them properly when necessary.
By adopting these techniques, you can develop a habit of double-checking your work and ensuring accuracy. Verifying your solutions not only helps in avoiding errors but also builds confidence in your mathematical abilities.
Understanding Graphs and Functions
Graphs are visual representations of mathematical relationships, providing a clear and intuitive way to understand how one quantity changes in relation to another. By plotting data points on a coordinate plane, we can visually explore patterns, trends, and behavior. Functions, on the other hand, describe how one variable depends on another. They establish a clear rule or relationship between inputs and outputs, which can be represented graphically for a better understanding.
Learning to interpret and create graphs is essential for mastering mathematical concepts. A graph allows you to quickly see the nature of the relationship between variables–whether it is linear, exponential, or follows some other pattern. Functions give you the tools to model real-world situations, like growth rates, costs, and other dynamic processes. By understanding the connection between graphs and functions, you can gain deeper insights into both theoretical and practical problems.
Finding the Right Tutor
Choosing the right tutor can make a significant difference in your academic journey. A tutor can provide personalized guidance, help clarify complex concepts, and boost your confidence. However, with so many options available, it’s important to find someone who matches your learning style and can address your specific needs effectively. The right tutor not only explains the material but also motivates and encourages you to take an active role in your learning.
When looking for a tutor, consider their qualifications, teaching experience, and approach to instruction. A good tutor should have a deep understanding of the subject matter and be able to break down challenging topics in a way that makes them easier to grasp. Additionally, it’s helpful to choose someone who is patient and can adapt their methods based on your pace and progress. With the right support, mastering difficult concepts becomes much more achievable.
Improving Skills Over Time
Mastering a subject requires consistent practice, patience, and a gradual buildup of knowledge. Improving your skills in any area takes time, and the process often involves revisiting concepts, refining techniques, and tackling increasingly complex problems. Whether you’re working through basic concepts or advancing to higher-level material, a steady approach can help solidify your understanding and enhance your ability to solve problems effectively.
Over time, regular practice allows you to identify patterns, internalize methods, and improve accuracy. It’s essential to build a solid foundation and gradually expand your understanding by applying what you’ve learned in new contexts. Persistence, combined with a willingness to seek help when needed, can significantly accelerate progress and lead to mastery in the subject.