In this section, we will explore detailed solutions and strategies for a critical high school mathematics exam that covers a range of advanced topics. The exam in question challenges students to demonstrate their proficiency in various mathematical concepts, with a particular focus on problem-solving and analytical thinking.
By reviewing the key questions and approaches, students can gain a deeper understanding of how to tackle similar problems in future assessments. Through comprehensive breakdowns and step-by-step guidance, we aim to offer valuable insights for effective preparation and improved performance on such challenging tests.
Mastering core principles and applying learned techniques are essential for success in any examination. By analyzing past materials, students can refine their skills and boost their confidence in approaching complex questions.
January 2012 Algebra 2 Trig Regents Overview
This section provides an overview of a comprehensive mathematics assessment designed to test students’ understanding of advanced mathematical concepts. The exam evaluates a variety of skills, including problem-solving, equation manipulation, and the ability to apply learned principles to complex scenarios. The questions are carefully structured to assess both theoretical knowledge and practical application in multiple contexts.
Key Concepts and Topics Covered
The exam encompasses a wide range of mathematical areas, including advanced functions, graphs, and the application of various formulas. Students are tested on their ability to manipulate equations and interpret complex problems, focusing on both conceptual understanding and technical proficiency. The exam structure includes both multiple-choice questions and open-ended problems, offering students opportunities to demonstrate their depth of knowledge.
Skills Required for Success
Success on this test requires a strong foundation in key mathematical skills such as graphing functions, solving systems of equations, and working with geometric principles. Students must also be able to quickly identify the most efficient methods for solving problems and make connections between abstract concepts and real-world applications. Critical thinking and time management are essential for navigating the exam effectively.
Key Topics Covered in the Exam
This examination tests a wide range of mathematical concepts, focusing on both theoretical understanding and practical problem-solving. It emphasizes the application of various techniques and principles to tackle complex problems across different areas of mathematics. The following table outlines the main topics and their respective areas of focus.
| Topic | Focus Areas |
|---|---|
| Functions and Graphs | Understanding properties of functions, graph interpretation, transformations, and the relationship between variables. |
| Equations and Inequalities | Solving linear, quadratic, and higher-order equations, as well as solving inequalities and systems of equations. |
| Trigonometry | Working with angles, sine, cosine, and tangent functions, solving for unknowns, and applying trigonometric identities. |
| Advanced Topics | Exploring polynomial functions, rational expressions, and logarithmic and exponential equations. |
| Geometry and Measurement | Applying geometric principles to calculate areas, volumes, and understanding geometric proofs and theorems. |
These topics cover essential areas needed to solve a variety of complex problems and demonstrate proficiency in mathematics. Mastery of these subjects is crucial for performing well in this exam and similar future assessments.
Understanding the Algebra 2 Trig Format
The structure of this mathematics exam is carefully designed to test a range of skills and knowledge across various mathematical disciplines. It includes both multiple-choice questions and open-ended problems, offering students the chance to demonstrate their abilities in different formats. The exam layout is intended to evaluate how well students can apply learned techniques and theories to solve real-world problems.
Questions are divided into sections based on topic areas, with some sections focusing on theoretical concepts while others emphasize practical application. The format encourages students to not only recall information but also interpret data, analyze patterns, and use logical reasoning to arrive at solutions.
Successful completion of the exam requires a balance of quick recall for straightforward questions and deeper analytical thinking for more complex problems. Time management is essential as students must efficiently navigate between question types while ensuring accuracy in their answers.
How to Approach Regents Questions
Approaching exam questions requires a clear and structured strategy to maximize performance. Each problem is designed to test specific skills, and having a plan can make a significant difference in how efficiently you solve them. Below is a table outlining helpful strategies for tackling different types of questions effectively.
| Question Type | Strategy |
|---|---|
| Multiple-Choice | Eliminate clearly incorrect answers first, then focus on narrowing down your choices by carefully reviewing each option. Check for subtle differences between choices. |
| Open-Ended | Read the problem thoroughly and break it into smaller parts. Show all work, even for simple steps, to ensure clarity in your reasoning and calculations. |
| Graph Interpretation | Carefully analyze the axes and scale of the graph. Pay attention to key features like intercepts, slopes, and asymptotes, and refer back to the problem’s context when interpreting the graph. |
| Word Problems | Highlight key information in the problem, set up equations or expressions, and solve step by step. Make sure to double-check units and ensure the answer matches the problem’s request. |
By staying organized and applying these strategies, you can approach each question with confidence and efficiency. The key is to remain focused and take the time to think through each problem before jumping into a solution.
Tips for Tackling Trigonometry Problems
Trigonometric problems often require a combination of visual interpretation, mathematical techniques, and careful attention to detail. When approaching these types of questions, it’s important to stay organized and follow a systematic approach. Below are some effective tips to help you navigate through trigonometric challenges with confidence.
Key Strategies for Success
- Understand the fundamentals: Be sure you have a solid grasp of key trigonometric functions such as sine, cosine, and tangent. Familiarity with their graphs and relationships is essential for solving problems efficiently.
- Use reference angles: When working with angles greater than 90°, use reference angles to simplify your calculations. This helps break down more complex problems into simpler steps.
- Apply identities: Familiarize yourself with important trigonometric identities, such as Pythagorean identities, sum and difference formulas, and double-angle identities. These can help simplify expressions and solve equations.
Problem-Solving Tips
- Start by carefully reading the question and identifying known values, including angle measures and side lengths, if applicable.
- Sketch a diagram if necessary. Visualizing the problem can make it easier to understand relationships between the angles and sides of a triangle or circle.
- Choose the appropriate trigonometric function based on the information provided. Decide whether sine, cosine, or tangent will be most useful for solving the problem.
- Check your calculations. Make sure your results are consistent with the problem’s constraints and logical reasoning.
By following these tips and practicing regularly, you can improve your problem-solving skills and gain confidence in tackling trigonometric questions during the exam.
Exploring the Algebra Section Details
The algebra section of the exam focuses on testing students’ ability to manipulate expressions, solve equations, and apply various mathematical concepts to real-world scenarios. It challenges students to demonstrate their proficiency in handling both linear and non-linear problems, often requiring a deeper understanding of relationships between variables. Below are the key topics and strategies to keep in mind when tackling the algebra section.
Key Areas to Focus On
- Solving Equations: Be prepared to solve both simple and complex equations, including linear, quadratic, and rational expressions. Pay attention to factoring, completing the square, and using the quadratic formula when necessary.
- Working with Expressions: Simplifying and manipulating algebraic expressions is a key skill. Practice combining like terms, distributing, and factoring to streamline expressions.
- Systems of Equations: Expect questions involving systems of linear equations. You may need to use substitution, elimination, or graphing methods to find the solution.
Approach to Problem Solving
- Start by identifying the type of equation or problem. This will help determine which techniques or formulas are most appropriate.
- For factoring problems, look for common factors or use the grouping method to break down more complex expressions.
- For systems of equations, choose the most efficient method (substitution or elimination) based on the given problem.
- Always check your solutions by substituting values back into the original equation to ensure accuracy.
By mastering these topics and practicing problem-solving techniques, you will be better prepared to tackle the algebra section and perform at your best on the exam.
Common Mistakes to Avoid
When taking a math exam, it’s easy to make small mistakes that can lead to incorrect answers, even if you understand the concepts. Being aware of common pitfalls can help you avoid these errors and improve your performance. Below are some frequent mistakes students make and tips on how to prevent them.
Key Mistakes to Watch Out For
- Misinterpreting the Problem: Always read the problem carefully before jumping into the solution. Misunderstanding the question can lead you down the wrong path.
- Skipping Steps: Skipping intermediate steps in an equation or calculation might seem faster but can result in simple errors. Always show your work clearly to avoid mistakes.
- Forgetting to Check Your Work: Never assume your first answer is correct. After solving a problem, take a moment to review your solution and verify that it fits the given conditions.
- Incorrect Use of Formulas: Using the wrong formula or incorrectly applying it can lead to mistakes. Always ensure you’re using the appropriate equation for the problem.
- Rushing Through the Test: Trying to answer too quickly often results in careless errors. Manage your time, but make sure you’re fully focused on each question.
How to Prevent These Mistakes
- Carefully read each question multiple times to ensure you understand the requirements before solving.
- Write down every step when working through calculations. This not only helps prevent errors but also makes it easier to spot mistakes if they occur.
- Double-check your calculations and solutions before finalizing your answers.
- Familiarize yourself with all relevant formulas and practice applying them correctly in different scenarios.
- Take your time, but don’t rush. Pace yourself to ensure accuracy and precision in your work.
By avoiding these common errors, you can improve your accuracy and boost your confidence when taking the exam.
How to Prepare for Similar Exams
Preparing for standardized math assessments requires a combination of reviewing core concepts, practicing problem-solving techniques, and developing effective test-taking strategies. To succeed in similar exams, it’s crucial to focus on both the theoretical aspects and the practical application of mathematical knowledge. Below are some helpful steps to guide you through your preparation process.
Effective Study Strategies
- Review Key Topics: Focus on understanding the fundamental concepts that will be tested, such as equations, functions, and graphs. Ensure you have a strong grasp of both basic and advanced topics.
- Practice Regularly: Consistent practice is essential for reinforcing what you’ve learned. Work through past exam papers, sample problems, and online practice tests to get a feel for the format and difficulty of the questions.
- Identify Weak Areas: Pay attention to topics where you struggle. Spend extra time reviewing these areas, seeking help if necessary, and practicing additional problems to build confidence.
Test-Taking Tips
- Begin by reading each question carefully to understand what is being asked before attempting to solve it.
- Organize your work clearly, showing all steps when solving problems. This helps you track your reasoning and increases the likelihood of finding errors.
- Manage your time effectively, ensuring you have enough time to complete all sections of the exam. Don’t spend too long on one question; move on and come back if necessary.
- Practice test-taking under timed conditions. This helps you adjust to the pressure of the exam and improves your pacing.
By following these steps and committing to regular practice, you can build the skills and confidence necessary to perform well on any similar assessments.
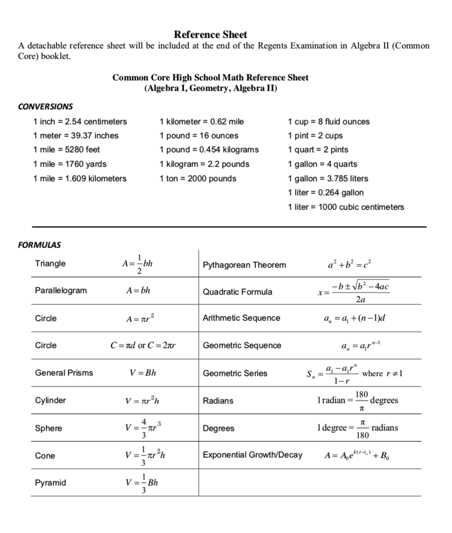
Reviewing Key Formulas for Success
Mastering essential formulas is crucial for tackling mathematical problems efficiently. Knowing when and how to apply these formulas can greatly improve your problem-solving ability and help you save valuable time during exams. Below is a review of some of the most important formulas you should be familiar with to achieve success in your assessment.
Essential Formulas to Remember
| Formula | Description | Application |
|---|---|---|
| Pythagorean Theorem: a² + b² = c² | Used to calculate the sides of a right triangle. | Apply when given two sides of a right triangle to find the third side. |
| Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a | Solves quadratic equations in the form ax² + bx + c = 0. | Use when factoring is difficult or impossible; solve for x directly. |
| Slope Formula: m = (y₂ – y₁) / (x₂ – x₁) | Determines the slope between two points on a coordinate plane. | Use to find the slope of a line when given two points. |
| Distance Formula: d = √((x₂ – x₁)² + (y₂ – y₁)²) | Finds the distance between two points in the plane. | Useful when calculating the distance between two given points. |
| Area of a Circle: A = πr² | Finds the area enclosed by a circle. | Use to calculate the area when the radius is known. |
Familiarity with these formulas will not only help you solve problems more efficiently but also enhance your understanding of key concepts in mathematics. Regular practice in applying these formulas will ensure you’re ready for any challenge that arises during the exam.
Strategies for Time Management
Effective time management is essential for performing well on any exam. By allocating your time wisely, you can ensure that you have enough moments to address each section without feeling rushed. The goal is to maintain a balance between speed and accuracy, which can be achieved through proper planning and focus.
Planning Your Approach
Before starting the test, take a moment to familiarize yourself with its structure. Understand how much time is allocated for each section and estimate how long to spend on each question. Here are a few strategies to help manage your time effectively:
- Allocate Time for Each Section: Divide the total exam time by the number of sections. This will help you determine how long to spend on each section without overcommitting to one.
- Set Time Limits for Questions: For multiple-choice questions, set a specific time limit, such as 1-2 minutes per question. For more complex problems, spend a little extra time, but be mindful not to get stuck.
- Start with Easy Questions: Begin with the questions you find easiest to gain confidence and build momentum. This will ensure you’re making the most of your time and not wasting it on harder problems initially.
Staying Focused During the Exam
During the exam, it’s essential to stay focused and avoid distractions. Here are some tips to maintain concentration:
- Avoid Spending Too Much Time on One Question: If you get stuck, move on to the next question and return to it later if time permits. Don’t let one question take up all your time.
- Keep an Eye on the Clock: Regularly check the time to stay on track. If you’re running behind, adjust your pace to ensure you can finish every section.
- Leave Time for Review: After answering all the questions, reserve the last 5-10 minutes for reviewing your answers. Double-check calculations and ensure you haven’t missed anything important.
By using these time management strategies, you’ll be able to approach the exam with confidence and efficiency, maximizing your chances for success.
Sample Questions from the January 2012 Exam
Familiarizing yourself with sample questions from past exams can be an excellent way to prepare for upcoming tests. By reviewing these examples, you can get a sense of the types of problems you’ll encounter and the level of difficulty you can expect. Here are a few sample questions based on the format and content typically found on this exam.
Sample Question 1
A function is defined by f(x) = 3x² – 5x + 2. Which of the following is the value of f(4)?
- A. 18
- B. 24
- C. 28
- D. 30
Sample Question 2
What is the solution to the equation 2x – 3 = 5x + 7?
- A. x = -4
- B. x = 4
- C. x = -5
- D. x = 5
Sample Question 3
In the right triangle below, the length of the hypotenuse is 10 units, and one of the legs is 6 units. What is the length of the other leg?
- A. 4
- B. 5
- C. 8
- D. 7
These sample questions highlight key areas typically tested, such as solving quadratic equations, working with linear expressions, and applying the Pythagorean theorem. Reviewing similar problems will help strengthen your skills and prepare you for a successful test experience.
Interpreting Graphs and Equations
Understanding how to read and analyze graphs and equations is a crucial skill for solving mathematical problems. Whether you’re working with linear, quadratic, or other types of functions, being able to interpret these visual and algebraic representations will allow you to draw accurate conclusions and solve problems more effectively.
Understanding Graphs

Graphs provide a visual representation of mathematical relationships, and interpreting them can give insight into key features such as slope, intercepts, and behavior at extreme values. To interpret graphs effectively:
- Identify Key Points: Look for the x-intercepts (where the graph crosses the x-axis) and y-intercepts (where the graph crosses the y-axis).
- Determine the Slope: The slope represents the rate of change of the function. For linear functions, the slope is constant and can be determined from the rise over run.
- Analyze the Shape: The shape of the graph can indicate the type of function, such as a straight line for linear functions or a parabola for quadratic functions.
Working with Equations
Equations describe relationships between variables, and interpreting them involves understanding how changes in one variable affect another. When working with equations:
- Solve for Unknowns: Equations often require you to solve for one variable in terms of others, such as finding x when given the equation of a line or a curve.
- Identify Function Types: The form of an equation can reveal the type of relationship it describes. For example, a linear equation will have a form like y = mx + b, while a quadratic equation will follow the form y = ax² + bx + c.
- Use Substitution: Substitute known values into equations to solve for unknown variables or confirm solutions that match with graph behavior.
By combining your understanding of both graphs and equations, you’ll be better equipped to solve problems and interpret the mathematical relationships they represent. Whether analyzing a graph or solving an equation, these skills are essential for tackling a variety of mathematical challenges.
Practice Problems and Solutions
Practicing with a variety of problems is essential for mastering key concepts and building confidence in solving mathematical challenges. By working through different types of exercises, you can strengthen your understanding of the material and prepare for similar assessments. Below, we present a few practice problems along with detailed solutions to guide your study process.
Problem 1: Solving Linear Equations
Solve the following equation for x:
2x + 5 = 19
Solution:
- Start by subtracting 5 from both sides: 2x = 14.
- Next, divide both sides by 2: x = 7.
- The solution is x = 7.
Problem 2: Working with Quadratic Equations
Solve the following quadratic equation:
x² – 5x + 6 = 0
Solution:
- Factor the equation: (x – 2)(x – 3) = 0.
- Set each factor equal to zero: x – 2 = 0 or x – 3 = 0.
- Solving these gives the solutions x = 2 or x = 3.
By practicing similar problems, you can become more efficient at solving equations and identifying patterns in the problems. These exercises provide a great foundation for tackling more complex questions and improving problem-solving skills.
Understanding Scoring Criteria
Grasping how assessments are evaluated is crucial for understanding the expectations and improving performance. The scoring process involves multiple components that assess both the accuracy of answers and the approach used to solve problems. Being aware of the criteria can help in optimizing your strategies to achieve better results.
Each exam typically consists of multiple sections, and each section has a designated number of points based on its complexity. The overall score is determined by the total points earned across all sections, which are then compared to established benchmarks. Understanding the scoring breakdown is vital for focusing on areas that carry more weight.
Additionally, partial credit is often awarded for correctly applying concepts, even if the final answer is incorrect. This encourages showing work clearly and demonstrating an understanding of the methodology used to arrive at a solution. It’s essential to follow proper steps and logic, as points may be deducted for missing or unclear explanations.
Knowing how the exam is scored allows for targeted preparation, helping to maximize performance by focusing efforts on higher-weighted areas and ensuring that every question is tackled strategically.
How to Analyze Your Performance
After completing any assessment, reviewing your performance is essential for understanding your strengths and areas for improvement. Analyzing your results allows you to identify patterns in the types of questions you struggled with and those you excelled at. This self-reflection is key to refining your skills for future exams or assignments.
Identifying Mistakes
Start by thoroughly reviewing each question, especially those you answered incorrectly. Understand why your initial approach didn’t work and whether it was due to a conceptual misunderstanding or a miscalculation. It’s also important to assess if time management played a role in the errors. By pinpointing the specific issues, you can focus your future studies on addressing them effectively.
Recognizing Strengths
In addition to identifying weaknesses, it’s equally important to recognize the areas where you performed well. Acknowledging your successes builds confidence and helps you understand which concepts or problem-solving strategies are already solid. This reflection can guide you to spend less time on topics you’ve mastered, allowing you to focus on more challenging material.
By breaking down your performance and focusing on both areas for growth and strengths, you can develop a more targeted study plan for improving your overall academic proficiency.
Useful Resources for Regents Preparation
To effectively prepare for exams, it’s crucial to use a variety of resources that reinforce key concepts and provide practice opportunities. These tools can help students strengthen their understanding, practice problem-solving skills, and familiarize themselves with the format of the questions. Whether you’re looking for study guides, practice tests, or interactive lessons, there are plenty of resources available to support your preparation.
One of the most effective ways to study is through practice problems. Many websites and textbooks provide sample questions that closely mirror the actual exam format. These can help you identify the areas where you need improvement while also giving you a feel for the timing and structure of the test.
In addition to practice questions, instructional videos and online tutorials offer in-depth explanations of challenging topics. These resources can break down complex ideas into simpler, more digestible steps. Interactive platforms often allow you to work through problems at your own pace, offering hints and solutions along the way.
Another useful tool is peer study groups. Collaborating with classmates provides an opportunity to discuss difficult concepts and learn from each other. Additionally, teachers and tutors can be invaluable resources, offering personalized guidance and clarifying any questions you may have.
Using a combination of these resources can ensure a well-rounded and thorough approach to exam preparation. By utilizing a variety of methods, you’ll be better equipped to tackle the challenges that arise during the test.