
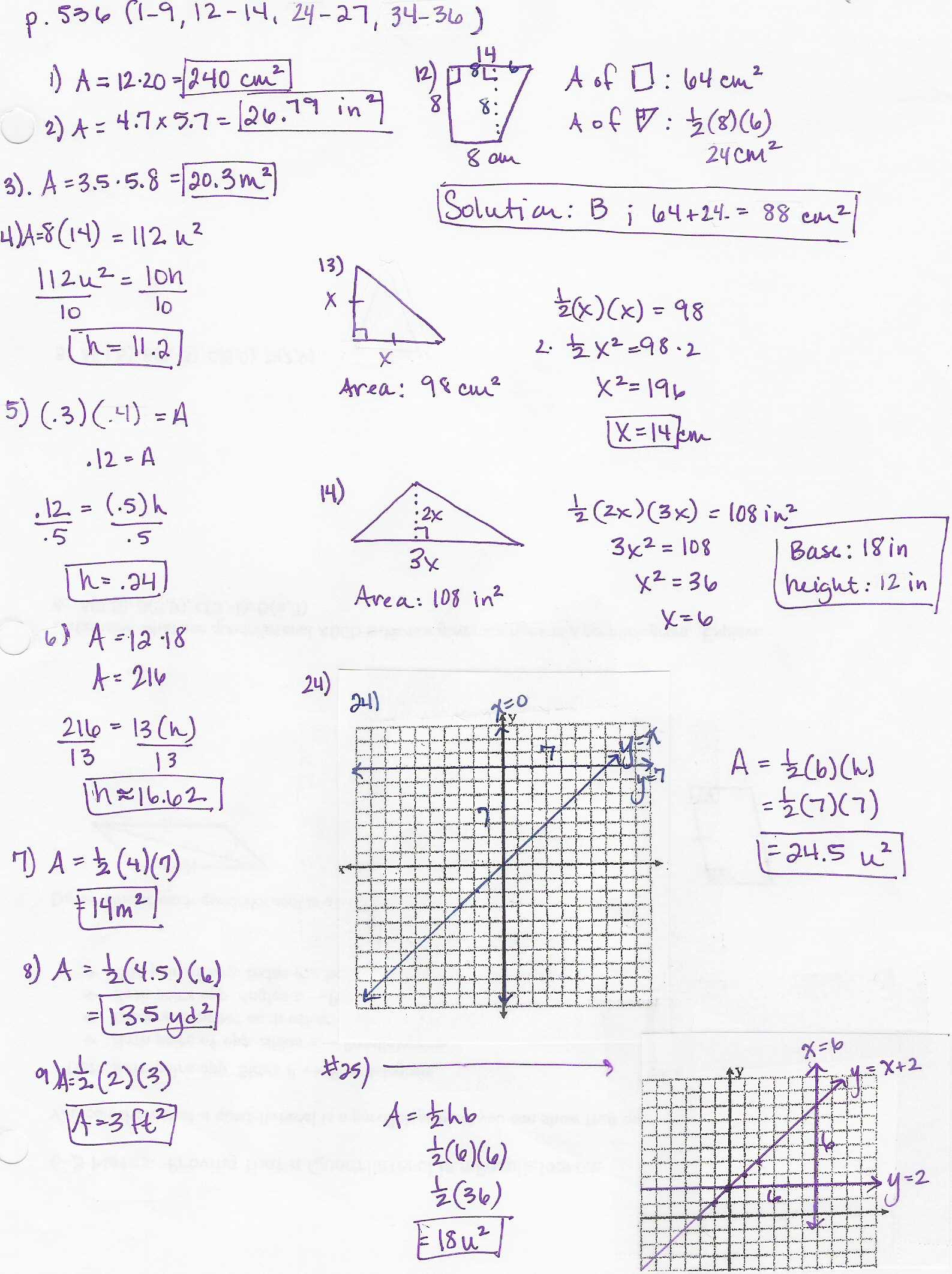
This section provides a comprehensive exploration of key mathematical ideas that are essential for building a strong foundation in problem-solving. By focusing on solving various equations and interpreting data, learners can strengthen their understanding of mathematical principles and improve their ability to tackle complex tasks. With step-by-step solutions and clear examples, the content offers a structured approach to mastering these concepts.
In this section, students are introduced to different strategies for addressing equations involving variables and inequalities. The emphasis is placed on clarity and precision, ensuring that even challenging problems become manageable through systematic methods. By mastering these techniques, learners can apply them confidently to a wide range of scenarios, enhancing both their skills and their confidence in mathematics.
Mathematical Solutions and Techniques
This section delves into essential mathematical techniques and strategies for solving equations and inequalities. The focus is on providing learners with a clear framework to approach various types of problems, enhancing both understanding and practical application of key concepts. By breaking down the steps involved, the process becomes more accessible, leading to improved problem-solving abilities.
Through detailed explanations and examples, students can gain confidence in tackling different scenarios. The key areas explored include:
- Solving linear equations with one variable
- Understanding and solving inequalities
- Graphing linear relationships
- Identifying key characteristics of equations
- Working through word problems effectively
Each of these topics is presented with practical examples to reinforce learning. Additionally, learners are encouraged to apply these methods to a variety of exercises to solidify their understanding and improve their overall mathematical skills.
By following a logical progression and addressing common challenges, this section aims to provide the tools necessary for students to successfully master these critical topics and move forward with confidence in their mathematical journey.
Overview of Section 4
This section focuses on developing a deeper understanding of key mathematical concepts that are crucial for solving different types of equations and analyzing mathematical relationships. It introduces essential tools and methods that allow learners to approach problems systematically, making the process of finding solutions more structured and manageable.
Throughout this section, students will explore a variety of techniques for working with linear equations, inequalities, and functions. Practical examples illustrate how these methods can be applied in different contexts, helping learners connect theoretical knowledge to real-world situations. With clear explanations and step-by-step guidance, learners are encouraged to develop both their problem-solving skills and their critical thinking abilities.
The main goal of this section is to equip students with the necessary skills to handle more complex mathematical problems with confidence and precision. By understanding the fundamental principles, students can build a solid foundation for further study and application of mathematics in more advanced topics.
Key Concepts in Section 4

This section introduces several fundamental mathematical principles that are essential for solving equations and interpreting relationships between variables. The focus is on providing a solid understanding of these core ideas, which serve as the foundation for more advanced topics. By mastering these key concepts, students can approach mathematical problems with greater confidence and clarity.
Among the important concepts covered are:
- Understanding the structure of linear equations
- Solving and graphing inequalities
- Identifying and working with variables in expressions
- Applying mathematical operations to simplify and solve problems
- Interpreting solutions within real-world contexts
Each concept is explained with clear examples and practical exercises, making it easier for learners to grasp the material and apply it in various scenarios. By reinforcing these core ideas, students are well-equipped to progress to more complex mathematical challenges with ease.
Solving Equations with Variables
In this section, the focus is on understanding how to solve mathematical problems that involve unknown values represented by variables. The process of solving these types of problems requires applying a series of logical steps to isolate the variable and determine its value. By mastering these techniques, students gain the ability to tackle a wide range of problems systematically and accurately.
To solve equations involving variables, it’s essential to:
- Identify the variable and its relationship to other terms in the equation
- Apply inverse operations to both sides of the equation
- Simplify the equation step by step until the variable is isolated
- Check the solution by substituting the value of the variable back into the original equation
By following these steps, learners can gain a deeper understanding of how equations work and how to manipulate them to find solutions. This approach also builds problem-solving skills that are applicable to more advanced mathematical tasks and real-life situations.
Understanding Inequalities in Mathematics
Inequalities are mathematical expressions that describe the relationship between two values, indicating whether one is greater than, less than, or not equal to the other. Unlike equations, inequalities provide a range of possible solutions rather than a single value. Understanding how to solve and interpret these expressions is essential for progressing in mathematical problem-solving and real-world applications.
Key Features of Inequalities
Inequalities involve various symbols to represent the relationship between terms:
- Greater than: >
- Less than: <
- Greater than or equal to: ≥
- Less than or equal to: ≤
- Not equal to: ≠
Each symbol has a specific meaning that determines how solutions are represented. Solving these inequalities often requires similar steps to solving equations, with the added complexity of considering a range of possible outcomes.
Solving Inequalities

To solve an inequality, follow these steps:
- Isolate the variable on one side of the inequality.
- Apply inverse operations to both sides, ensuring the inequality sign remains correctly oriented.
- If you multiply or divide by a negative number, flip the inequality symbol.
- Express the solution as a range of values that satisfy the inequality.
Understanding inequalities helps build critical thinking skills, as it involves analyzing ranges rather than fixed solutions. This knowledge is useful not only in mathematics but also in fields like economics, engineering, and social sciences, where variables often have multiple possible outcomes.
Step-by-Step Guide to Solutions

Solving mathematical problems becomes more manageable when broken down into clear, logical steps. This guide provides a structured approach to help you solve various types of problems with precision and confidence. By following a systematic method, each step leads to the next, ensuring that all necessary aspects of the problem are addressed.
General Approach to Solving Problems
When solving any problem, start by organizing the information and clearly identifying what is being asked. A consistent approach can simplify even the most complex tasks:
- Step 1: Read and understand the problem statement.
- Step 2: Identify the unknowns and variables.
- Step 3: Set up the equation or expression based on the problem.
- Step 4: Simplify the equation where possible.
- Step 5: Solve for the unknowns using appropriate methods.
- Step 6: Check the solution by substituting the values back into the original equation.
Applying the Solution Process
For example, when solving for a variable in an equation, follow these steps:
- Isolate the variable by performing inverse operations on both sides.
- Simplify each side as much as possible.
- For inequalities, remember to reverse the inequality sign when multiplying or dividing by a negative number.
- Verify the solution by substituting it into the original equation or inequality.
By using this step-by-step method, you ensure that all aspects of the problem are considered and the solution is found in a clear, logical manner. Whether you are solving simple equations or more complex problems, this approach helps to build confidence and consistency in mathematical problem-solving.
Common Mistakes to Avoid
When solving mathematical problems, even small errors can lead to incorrect solutions and confusion. Being aware of common mistakes helps ensure more accurate results and a deeper understanding of the concepts. This section highlights some of the most frequent errors students make and provides tips on how to avoid them.
One common mistake is misinterpreting the operations involved in an equation or inequality. For example, failing to properly apply the order of operations can lead to incorrect answers. Similarly, not recognizing when to reverse the inequality sign when multiplying or dividing by a negative number can result in the wrong solution. To avoid these pitfalls, always double-check each step and ensure that operations are performed in the correct sequence.
Another common mistake is neglecting to check the solution. After solving an equation, it is important to substitute the result back into the original equation to verify that it holds true. Skipping this verification step can result in missed errors that would otherwise be caught.
- Misinterpreting the problem: Always read the problem carefully and identify all given values before proceeding.
- Incorrectly simplifying equations: Take time to simplify expressions step by step, paying close attention to signs and terms.
- Overlooking variable signs: Be mindful of positive and negative signs when isolating variables.
- Forgetting to check the solution: Always substitute your solution back into the original equation to ensure its accuracy.
By being aware of these common mistakes and taking the time to avoid them, students can significantly improve their problem-solving accuracy and efficiency. With practice and attention to detail, these errors become less frequent, and mathematical understanding deepens.
Practical Examples of Mathematical Problems
Applying theoretical concepts to real-world situations is a crucial step in understanding how mathematical principles work. This section presents a variety of practical examples that demonstrate how algebraic techniques can be used to solve everyday problems. By working through these examples, learners can connect abstract concepts to tangible scenarios, enhancing their problem-solving skills.
Example 1: Solving for an Unknown Cost
Imagine you need to calculate the cost of an item after a discount. Suppose an item originally costs $50, and there is a 20% discount. To find the final price, we can use a simple equation:
- Step 1: Set up the equation: Final price = Original price – (Discount percentage × Original price)
- Step 2: Substitute values: Final price = 50 – (0.20 × 50)
- Step 3: Simplify: Final price = 50 – 10 = 40
The final price after the discount is $40. This example demonstrates how to use basic operations to solve real-world pricing problems.
Example 2: Solving a Mixture Problem
Suppose you are mixing two types of fruit juice. One juice contains 60% orange juice, and the other contains 30%. You need to create 10 liters of a mixture that is 45% orange juice. How much of each juice should you use?
- Step 1: Set up the equation for the total amount of orange juice in the mixture: 0.60x + 0.30(10 – x) = 4.5 (where x is the amount of the 60% juice)
- Step 2: Simplify and solve for x:
- 0.60x + 3 – 0.30x = 4.5
- 0.30x = 1.5
- x = 5
This example shows how algebraic equations can help solve practical problems involving mixtures and concentrations.
These examples highlight the importance of applying algebraic methods to everyday situations. By practicing these types of problems, learners can gain confidence in their ability to use math in real life.
Tips for Mastering Chapter 4 Content
To successfully master the material in this section, it’s essential to adopt effective strategies that will help reinforce key concepts and improve problem-solving skills. Whether you’re struggling with specific problems or aiming to strengthen your overall understanding, the following tips will guide you toward mastery of the content.
1. Focus on Fundamental Principles
Before diving into complex problems, ensure that you have a strong grasp of the basic principles. Building a solid foundation is crucial for tackling more advanced topics. Here are some steps to help reinforce core concepts:
- Review basic operations: Make sure you are comfortable with addition, subtraction, multiplication, and division.
- Understand key terms: Familiarize yourself with essential terminology such as variables, coefficients, and constants.
- Practice simple problems: Start with easier examples to strengthen your skills before attempting more complex problems.
2. Break Down Complex Problems
When confronted with a challenging problem, take a systematic approach to simplify it. Breaking down the steps helps prevent confusion and allows you to tackle the problem more efficiently. Here’s how to approach difficult tasks:
- Identify the unknowns: Carefully read through the problem and determine what you’re solving for.
- Set up an equation: Translate the problem into an equation or inequality that reflects the relationships between the variables.
- Solve step-by-step: Avoid rushing through the steps. Solve each part of the problem one at a time and check your work as you go.
3. Regular Practice and Review
Consistent practice is key to mastering any subject. Set aside time each day to practice problems, review mistakes, and reinforce your understanding. To track progress:
- Work through a variety of problems: Challenge yourself with problems of varying difficulty to improve flexibility in problem-solving.
- Review errors: Analyze mistakes to understand why they occurred and how to avoid them in the future.
- Test yourself: Take practice tests or quizzes to evaluate your progress and pinpoint areas that need improvement.
By applying these tips, you’ll build confidence and proficiency in mastering the content of this section. With patience, practice, and consistent effort, you can deepen your understanding and improve your problem-solving abilities.
How to Approach Word Problems
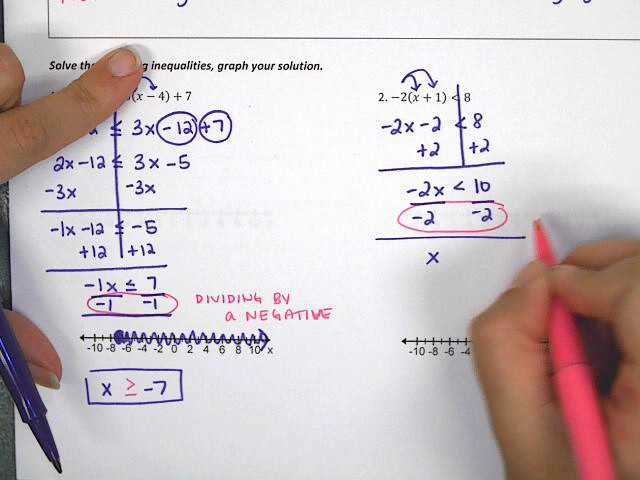
Word problems can often seem daunting at first, but breaking them down into manageable steps can make them much easier to solve. The key is to carefully read through the problem, identify the important information, and translate the words into a mathematical framework. By following a systematic approach, you can find solutions with confidence and clarity.
1. Understand the Problem

The first step in solving a word problem is fully understanding the situation. Carefully read the problem and identify what is being asked. Pay attention to the specific details and quantities mentioned, as they will help you set up your equation or inequality. Follow these guidelines:
- Highlight key information: Underline or write down the important numbers and terms that are given in the problem.
- Identify what you need to find: Clearly define the unknowns and understand what the problem is asking you to solve for.
- Look for relationships: Determine how the numbers or variables are related to each other in the context of the problem.
2. Translate the Words into Equations

Once you have a clear understanding of the problem, the next step is to convert the information into mathematical terms. This may involve setting up an equation, a system of equations, or even an inequality. To do this effectively:
- Define variables: Assign a variable to represent the unknown quantity you are solving for. For example, let x represent the number of apples in the problem.
- Write an equation: Use the relationships from the problem to create a mathematical expression. For instance, if the total cost is the sum of individual prices, write the equation for total cost as the sum of prices.
- Check for consistency: Ensure that your equation accurately represents the relationships described in the problem. Double-check that you haven’t missed any key details.
By following these steps, you’ll be able to approach word problems with a clear strategy, ensuring you understand the situation and can set up the right mathematical expressions to solve them.
Explaining Graphs and Functions
Graphs and functions are powerful tools for visualizing and understanding mathematical relationships. A graph provides a visual representation of how variables relate to each other, while a function defines the connection between inputs and outputs. By interpreting graphs and understanding the underlying functions, you can gain deeper insights into how values change and interact in a mathematical context.
1. Understanding Functions
A function is a rule that assigns each input exactly one output. This relationship can be represented algebraically, numerically, or graphically. To understand a function, focus on how the values of one variable determine the corresponding values of another. The function’s graph can help you visualize these connections. Key aspects to focus on include:
- Domain: The set of all possible input values for the function.
- Range: The set of all possible output values generated by the function.
- Graph behavior: Observe how the graph changes as you move along the x-axis, noting increases, decreases, or constant values.
2. Interpreting Graphs
Graphs provide a visual depiction of the relationships in a function, making it easier to identify trends and patterns. Each point on the graph corresponds to an input-output pair, where the x-coordinate represents the input and the y-coordinate represents the output. By analyzing the graph, you can identify key features such as:
- Intercepts: The points where the graph intersects the x-axis or y-axis, indicating when the output or input is zero.
- Slope: The steepness of the graph, showing how quickly the output changes as the input changes.
- Curvature: How the graph bends, which can indicate increasing or decreasing rates of change.
By mastering both the concept of functions and the interpretation of graphs, you can unlock a deeper understanding of how mathematical relationships work and apply this knowledge to solve real-world problems.
Use of Algebraic Expressions in Real Life
Algebraic expressions are not just abstract concepts; they play a significant role in everyday life. By representing relationships between quantities, these expressions allow us to model real-world situations and solve practical problems. From calculating expenses to predicting future trends, algebra helps us understand and navigate the world around us.
1. Budgeting and Financial Planning
One of the most common applications of algebraic expressions is in managing personal finances. For example, when planning a budget, you might use an expression to calculate your total expenses based on various categories such as rent, utilities, and groceries. If the cost of groceries changes, you can easily adjust the expression to see how it affects your overall budget.
- Example: If your monthly rent is $800, utilities cost $100, and groceries are $200, your total expenses can be represented by the expression: 800 + 100 + 200 = Total Expenses.
2. Predicting Outcomes in Business
In the business world, algebraic expressions are used to forecast future sales, calculate profits, and optimize resources. For example, a company might use an expression to calculate its expected revenue based on the number of products sold and the price per item. By using algebra, businesses can make data-driven decisions and plan for growth.
- Example: If a company sells 150 products at $20 each, the total revenue is calculated using the expression: 150 * 20 = Total Revenue.
By understanding and using algebraic expressions, we can make more informed decisions and approach daily challenges with greater confidence and efficiency.
Applying Operations in Algebraic Equations
Operations play a crucial role in solving equations and manipulating mathematical expressions. These operations–addition, subtraction, multiplication, and division–are applied to both sides of an equation to isolate variables or simplify expressions. Understanding how and when to use these operations allows for the step-by-step solving of equations and helps in understanding the relationships between different variables.
1. Simplifying Expressions
Before solving an equation, it’s often helpful to simplify expressions on both sides. This process involves combining like terms and performing basic operations to reduce the equation to its simplest form. For example, when you have an expression like 3x + 4x, you can combine the terms to make it 7x. Simplifying expressions makes it easier to isolate the variable and find its value.
- Example: If the equation is 2x + 5 = 15, subtract 5 from both sides to isolate the term with the variable: 2x = 10.
2. Solving for Variables
Once an equation is simplified, operations can be applied to solve for the unknown variable. This typically involves isolating the variable on one side of the equation by performing inverse operations. For example, if you have the equation 3x = 12, dividing both sides by 3 will give you x = 4. Using inverse operations ensures that the equation remains balanced while isolating the variable.
- Example: For the equation 5x – 3 = 22, first add 3 to both sides to get 5x = 25, then divide both sides by 5 to find x = 5.
Applying operations in a systematic way helps in solving equations step by step, ensuring the accuracy of the solution and a deeper understanding of how different mathematical elements relate to each other.
Common Formulas in Chapter 4
In the study of mathematical problems, specific formulas are used repeatedly to solve equations, simplify expressions, and understand relationships between variables. These formulas serve as tools to streamline calculations and ensure accuracy in problem-solving. Below are some of the key formulas often encountered in this section.
1. Linear Equation Formula
The formula for a linear equation helps solve for unknown variables by maintaining the balance of the equation. It is used extensively in problems that involve direct proportionality.
| Formula | Description |
|---|---|
| y = mx + b | This represents the equation of a straight line, where m is the slope and b is the y-intercept. |
2. The Distributive Property
The distributive property is frequently used to simplify expressions by distributing a number across terms within parentheses. This property is particularly helpful when dealing with expressions that involve multiplication and addition or subtraction.
| Formula | Description |
|---|---|
| a(b + c) = ab + ac | This shows how a number a is distributed to both terms inside the parentheses. |
These formulas form the foundation of many of the key concepts in this section. By mastering them, students can tackle a wide range of problems more effectively and with greater ease.
Reviewing Key Terms and Definitions
Understanding important terms and definitions is essential when working through mathematical concepts. Familiarity with key terms allows students to interpret problems accurately, apply the correct methods, and develop a deeper understanding of the subject. Below are some of the most frequently encountered terms in this section.
1. Variable
A variable represents an unknown value that can change depending on the situation. It is typically denoted by a letter, such as x or y, and plays a critical role in equations and expressions.
| Term | Definition |
|---|---|
| Variable | A symbol, usually a letter, that stands for an unknown or changing value in mathematical expressions or equations. |
2. Constant
A constant is a fixed value that does not change. Unlike a variable, which can vary, a constant remains the same in a given context. Constants are crucial for creating the structure of equations.
| Term | Definition |
|---|---|
| Constant | A number or value that does not change during the course of an equation or expression. |
3. Equation
An equation is a statement that two expressions are equal. It consists of variables, constants, and mathematical operations such as addition, subtraction, multiplication, and division.
| Term | Definition |
|---|---|
| Equation | A mathematical statement showing that two expressions are equal, often containing variables and constants. |
By reviewing these definitions, students can build a solid foundation that will help them better understand more complex topics in this section. Recognizing and applying these terms is a crucial step towards mastering the material.
How to Improve Your Math Skills
Improving your skills in mathematical problem-solving requires consistent practice, understanding fundamental concepts, and applying effective strategies. By focusing on specific techniques and methods, you can build confidence and enhance your overall ability to tackle various types of problems. Here are some tips to guide your progress.
1. Practice Regularly
Repetition is key to mastery. The more problems you solve, the more familiar you become with the methods and approaches needed to solve them. Working through a variety of problems helps reinforce concepts and improves your problem-solving speed and accuracy.
| Tip | Explanation |
|---|---|
| Regular Practice | Solving different types of problems consistently helps reinforce concepts and improve proficiency. |
2. Break Down Complex Problems
When faced with a challenging problem, try to break it down into smaller, more manageable steps. This will allow you to focus on solving one part at a time, reducing the complexity and making the problem easier to tackle.
| Tip | Explanation |
|---|---|
| Break It Down | Breaking problems into smaller parts makes complex tasks easier to understand and solve. |
3. Focus on Understanding, Not Memorizing
Instead of just memorizing formulas or steps, try to understand the reasoning behind each solution. This deeper understanding will allow you to adapt and apply your knowledge to a broader range of problems.
| Tip | Explanation |
|---|---|
| Understand, Don’t Memorize | Focusing on understanding concepts provides long-term retention and helps with solving unfamiliar problems. |
4. Use Additional Resources
Take advantage of online tutorials, practice worksheets, and textbooks. These resources provide explanations, examples, and exercises to help reinforce your learning and offer different perspectives on solving problems.
| Tip | Explanation |
|---|---|
| Use Extra Resources | Additional learning materials can provide different methods of solving problems and clarify difficult concepts. |
5. Stay Positive and Patient
Improvement takes time, so be patient with yourself. Maintain a positive attitude even when faced with difficult problems, as this mindset will help you stay motivated and continue making progress.
| Tip | Explanation |
|---|---|
| Stay Positive | A positive mindset encourages continued effort, even when encountering difficult challenges. |
By incorporating these strategies into your study routine, you can significantly improve your mathematical skills and approach problems with greater confidence. Remember, consistent effort and a focus on understanding will lead to long-term success.
Additional Resources for Learning
When striving to deepen your understanding and refine your problem-solving abilities, it’s beneficial to explore various supplementary materials. These resources can offer different approaches, clearer explanations, and additional practice to solidify your grasp on key concepts. Below are some valuable tools to enhance your learning experience.
1. Online Educational Platforms
Many websites provide interactive lessons, quizzes, and video tutorials that can help reinforce concepts. Platforms like Khan Academy, Coursera, and edX offer free or affordable courses that cover a wide range of topics and include practice problems for better retention.
2. Practice Workbooks and Worksheets

Workbooks dedicated to mathematical problem-solving are another great resource. These books typically include step-by-step solutions and additional problems that help you apply learned concepts in various scenarios. Many are available in bookstores or online.
3. YouTube Educational Channels
There are numerous educational YouTube channels that focus on breaking down complex concepts into digestible lessons. Channels like PatrickJMT and Professor Leonard offer clear explanations of mathematical procedures, making them accessible to learners of all levels.
4. Math Forums and Study Groups
Joining an online community such as Stack Exchange or Reddit’s math subreddits can provide valuable insights from peers and experts alike. Engaging in discussions and solving problems with others can broaden your perspective and clarify any doubts you may have.
5. Mobile Apps for Practice
Various mobile apps are designed to improve math skills with interactive exercises and instant feedback. Apps like Photomath, Wolfram Alpha, and Microsoft Math Solver help solve problems step-by-step and explain the process, offering a practical way to practice on the go.
6. Tutoring Services

If you’re struggling with specific concepts, personal tutoring can provide tailored guidance. Whether in-person or online, tutors can offer personalized lessons and work through complex problems with you, ensuring a deeper understanding of the material.
Utilizing a combination of these resources can significantly enhance your learning journey. The key is to engage actively with the material, practice regularly, and seek support when necessary. With the right tools, mastering mathematical concepts becomes both achievable and rewarding.