
In any advanced mathematics course, mastering complex concepts requires both practice and proper guidance. Whether tackling equations, functions, or graphs, having the right resources at hand can make a significant difference in understanding the material. This section focuses on providing essential support for students looking to strengthen their problem-solving skills in higher-level mathematics.
By working through various exercises and reviewing step-by-step solutions, learners can gain a clearer understanding of how to approach different mathematical challenges. These resources not only aid in mastering specific problems but also foster a deeper comprehension of the underlying principles that govern the subject.
Effective practice is key to success in mathematics. With the right strategies, students can improve both their theoretical knowledge and their ability to apply concepts to practical situations. Whether preparing for exams or reinforcing daily lessons, this guide is designed to help students navigate through more difficult mathematical topics with confidence.
Mathematical Problem Solutions
When tackling higher-level mathematical challenges, having access to well-structured solutions can significantly improve comprehension. By reviewing carefully worked-out examples, students can better grasp complex topics and learn effective methods for solving similar problems on their own. This section provides step-by-step walkthroughs of key exercises, offering clarity on each stage of the problem-solving process.
These solutions serve as a guide, helping learners understand the rationale behind every calculation. They provide insight into how various concepts are interconnected and how different approaches can lead to the correct result. Whether it’s simplifying equations, solving for unknowns, or interpreting graphs, these examples demonstrate practical techniques that are essential for mastering the material.
Having access to reliable resources for verification allows students to check their progress and ensure they are on the right track. Working through problems with detailed explanations not only boosts confidence but also encourages deeper engagement with the subject, helping learners build a strong mathematical foundation for future studies.
Understanding Advanced Mathematics Curriculum
The curriculum for advanced mathematics is designed to build a deep understanding of key concepts, offering students the tools they need to solve increasingly complex problems. By following a structured approach, the course progresses through various stages, helping learners develop critical thinking and problem-solving skills that will serve them in future mathematical studies and real-world applications. This section breaks down the core components of the course, explaining how each element contributes to mastering the subject.
The curriculum covers a broad range of topics, from functions and equations to statistical analysis and complex problem-solving techniques. Students are introduced to foundational principles early on, and these principles are gradually expanded upon to cover more advanced material. The goal is to provide students with a thorough understanding of the relationships between different mathematical concepts, enabling them to approach problems from multiple angles.
| Topic | Description |
|---|---|
| Equations and Functions | Exploring different types of equations and their solutions, including linear, quadratic, and exponential functions. |
| Polynomials | Learning about polynomial expressions, factoring, and solving polynomial equations. |
| Rational Expressions | Understanding how to simplify, solve, and work with rational functions. |
| Complex Numbers | Introducing the concept of imaginary numbers and their applications in solving non-real solutions. |
| Statistics and Probability | Analyzing data sets and calculating probabilities in different scenarios. |
By progressing through these topics, students are equipped to handle a wide variety of mathematical challenges, laying the foundation for future academic success in mathematics and related fields. Understanding how each concept fits within the broader curriculum is essential for making the most of the learning experience and building confidence in problem-solving abilities.
How to Use Mathematics Solutions Effectively
To truly benefit from worked-out examples, it’s essential to approach them with a strategic mindset. Simply reviewing the solutions without actively engaging with them can hinder learning. Instead, students should focus on understanding each step, questioning the reasoning behind it, and practicing similar problems to reinforce their skills. Here are some tips on how to maximize the effectiveness of these resources:
- Analyze Each Step: Take time to break down every part of the solution. Understand why each step is necessary and how it contributes to reaching the final result.
- Work Through Problems Independently: After reviewing a solution, try solving a similar problem on your own. This helps solidify the techniques used and builds confidence in your abilities.
- Focus on Conceptual Understanding: Don’t just memorize procedures. Aim to understand the underlying concepts so you can apply them in different contexts.
- Revisit Difficult Problems: If a particular step or method confuses you, revisit it multiple times until it becomes clear. This repeated exposure helps strengthen your understanding.
- Practice Regularly: Consistent practice is key. The more problems you solve, the more familiar you become with different approaches and the better equipped you are to handle challenges.
By using solutions in this way, students can enhance their understanding, develop strong problem-solving skills, and ultimately improve their performance in mathematics. The goal is not just to find the correct answer, but to understand how to get there and apply the same methods to other problems.
Key Topics in Advanced Mathematics

In any advanced mathematics curriculum, there are several foundational concepts that students must master in order to solve more complex problems and understand higher-level material. These key topics serve as building blocks, each one linking to the next and offering insights into different mathematical areas. By focusing on these essential areas, students can develop a strong foundation and gain the skills needed to tackle increasingly challenging exercises.
- Equations and Functions: Understanding how to solve various types of equations and the behavior of different types of functions is fundamental. This includes linear, quadratic, and exponential relationships.
- Polynomials: Learning how to factor, simplify, and solve polynomial expressions prepares students for more advanced topics in calculus and higher mathematics.
- Rational Expressions: Working with fractions involving variables and understanding how to simplify and manipulate them is a crucial skill.
- Systems of Equations: Mastering systems allows students to solve multiple equations simultaneously, using methods like substitution and elimination.
- Complex Numbers: A key topic in many higher-level mathematics courses, complex numbers expand the number system and are essential for solving equations with no real solutions.
- Sequences and Series: Students learn how to analyze and work with sequences, identify patterns, and calculate sums of series, which are useful in many applied fields.
- Probability and Statistics: Basic understanding of data analysis, probability, and statistical methods is critical for making informed decisions in both academics and real-world situations.
By mastering these topics, students develop the tools needed to solve more advanced mathematical problems and prepare for future studies in fields such as engineering, economics, and the physical sciences. Each concept provides a critical piece of the puzzle in building comprehensive mathematical knowledge.
Step-by-Step Approach to Advanced Math Problems
Breaking down complex problems into manageable steps is an effective strategy for solving advanced mathematical exercises. Instead of rushing through a problem, taking the time to understand each part of the process allows students to avoid mistakes and build a deeper understanding of the concepts. This approach helps to build confidence and ensures a systematic method for tackling any challenge.
1. Identify the Problem Type
The first step in any problem-solving process is to recognize what type of problem you are dealing with. This involves understanding the mathematical concepts at play, whether it’s solving for unknown variables, factoring expressions, or analyzing functions. Once the problem type is clear, you can choose the best method to proceed.
2. Break It Down into Steps

Once the problem is identified, break it down into smaller, more manageable parts. For example, if the problem involves solving an equation, start by isolating the variable, then proceed step-by-step to simplify and solve. Keeping track of each individual step ensures that you don’t overlook important details.
For example: When solving a quadratic equation, begin by setting the equation equal to zero, then use factoring or the quadratic formula to find the roots.
By following this structured approach, students can work through difficult problems more efficiently and with greater accuracy. This method not only helps in solving individual exercises but also reinforces the overall understanding of the subject matter.
Common Challenges in Advanced Math
Many students face challenges when dealing with advanced mathematical concepts. These obstacles often arise when transitioning from basic to more complex topics. Understanding these difficulties and knowing how to approach them can significantly improve performance and comprehension. Below are some of the most common issues students encounter and strategies for overcoming them.
- Complex Problem Structures: Some problems may involve multiple steps or different methods, making them hard to navigate. It is essential to break down each problem into smaller parts and focus on solving them step by step.
- Understanding Abstract Concepts: Topics like imaginary numbers or functions with multiple variables can feel abstract. Taking the time to visualize these concepts and apply them in real-world scenarios can help make them more tangible.
- Difficulty with Word Problems: Translating verbal descriptions into mathematical equations can be difficult. To overcome this, practice interpreting word problems and identify key phrases that point to mathematical operations.
- Handling Negative and Fractional Exponents: Many students struggle with operations involving negative exponents or fractions in exponents. Reviewing the basic laws of exponents and practicing various examples can help clarify these rules.
- Graphing and Interpreting Functions: Graphing complex functions and understanding their behavior, especially when they include transformations or multiple variables, can be challenging. Regular practice with graphing tools and software can help visualize these functions more clearly.
- Time Management and Exam Anxiety: Often, the pressure of exams or timed assignments can affect performance. Developing good study habits, practicing time management, and learning relaxation techniques can help alleviate this stress.
By recognizing these challenges early on, students can take proactive steps to address them. With regular practice, patience, and the right strategies, these obstacles can be overcome, leading to a deeper understanding of the material and improved problem-solving skills.
Tips for Mastering Advanced Math Concepts
Mastering advanced mathematical concepts requires more than just memorizing formulas or procedures–it involves developing a deep understanding of the underlying principles. By adopting effective strategies and practicing regularly, students can strengthen their problem-solving skills and gain confidence in tackling complex exercises. Here are some useful tips to help you master key concepts and excel in your studies.
- Practice Consistently: The more problems you solve, the more familiar you become with various techniques. Regular practice helps reinforce concepts and improves your ability to handle different problem types.
- Understand the “Why” Behind the Methods: Instead of simply memorizing steps, focus on understanding why specific methods work. This deeper comprehension allows you to apply techniques in diverse scenarios.
- Break Down Complex Problems: When faced with a complicated problem, break it down into smaller, manageable parts. This approach makes the problem less overwhelming and allows you to focus on one step at a time.
- Use Visual Aids: Graphs, charts, and diagrams can make abstract concepts more concrete. Visualizing problems helps in understanding functions, equations, and geometric relationships more clearly.
- Seek Help When Needed: Don’t hesitate to ask for help from teachers, tutors, or classmates when you encounter difficulties. Clarifying misunderstandings early on prevents them from becoming larger issues later.
- Review Mistakes: When you make errors, take time to understand where and why you went wrong. Analyzing mistakes helps to reinforce correct methods and prevents repeating the same errors in the future.
- Stay Organized: Keeping notes and formulas well-organized allows for quick reference and efficient problem-solving. An organized approach reduces confusion and saves time during study sessions and exams.
By following these tips and committing to consistent practice, you’ll develop a solid foundation in advanced math and build the confidence to solve even the most challenging problems with ease.
Why Advanced Math is Crucial for Students
Mastering higher-level mathematics plays a fundamental role in a student’s academic and professional development. This branch of mathematics is not only a requirement for graduation, but it also serves as the foundation for many future studies, including science, engineering, technology, and economics. Understanding these concepts enables students to think critically, solve complex problems, and apply their knowledge in real-world situations.
Advanced math is essential because it develops logical reasoning and analytical thinking. These skills are transferable to other subjects and fields, helping students approach challenges with structured problem-solving techniques. Moreover, many college programs and careers in high-demand sectors require a solid grasp of mathematical concepts, making it an indispensable part of a student’s education.
| Reason | Importance |
|---|---|
| Critical Thinking: | Helps students analyze problems logically and approach challenges from multiple perspectives. |
| Preparation for Future Careers: | Many high-demand jobs, particularly in technology and engineering, require strong mathematical skills. |
| Real-World Applications: | Mathematics is used in everyday problem-solving, financial planning, and decision-making. |
| Foundation for Advanced Studies: | Mastering advanced math opens the door to more specialized studies, including calculus, statistics, and more. |
By focusing on these critical concepts, students not only gain the skills needed to succeed academically but also prepare themselves for a wide range of opportunities in their future careers. Understanding the importance of advanced math is a step toward unlocking numerous paths to success and innovation.
How Advanced Math Prepares You for Calculus
The transition from basic mathematical concepts to more complex topics like calculus can be challenging, but a solid understanding of earlier mathematical principles lays the groundwork for future success. Advanced mathematics introduces key concepts such as functions, equations, and graphing techniques, which are essential when approaching calculus. Without mastering these fundamental topics, students may struggle with the more abstract ideas that calculus presents.
1. Functions and Their Behavior
In advanced math, students explore a variety of functions, including linear, quadratic, and exponential functions. Understanding the properties of these functions, such as how they change or how to manipulate them, is critical in calculus. For example, calculus builds heavily on the concept of the derivative, which measures the rate of change of a function. A firm grasp of how functions behave and interact is necessary for comprehending the derivative and other calculus concepts.
2. Solving Equations and Inequalities

In higher-level math, students also learn to solve complex equations and inequalities. These skills are crucial for calculus, where solving equations such as limits and integrals requires a solid foundation in algebraic manipulation. The ability to simplify and solve equations efficiently ensures that students can tackle calculus problems with confidence and accuracy.
For example: Solving quadratic equations in advanced math prepares students for understanding optimization problems in calculus, where finding the maximum or minimum of a function is often required.
By mastering these foundational topics, students are not only prepared for the challenges of calculus but also develop the problem-solving skills necessary for advanced studies in mathematics and other disciplines. Calculus builds on these earlier concepts, and the more familiar students are with them, the easier the transition will be.
Finding Help with Advanced Math Online
When struggling with complex math problems, it can be challenging to find the right resources. Fortunately, the internet provides numerous platforms where students can find assistance, whether it be for understanding specific concepts, solving practice problems, or preparing for exams. Online resources offer flexibility, allowing students to get help at their own pace and on their own schedule. Here are some of the best ways to find help online for mastering advanced math concepts.
- Online Tutoring Services: Many platforms offer one-on-one tutoring sessions with qualified educators. These services can provide personalized attention and tailored explanations for difficult topics.
- Interactive Learning Websites: Websites such as Khan Academy or Coursera offer free and paid courses that cover a wide range of topics. These platforms often feature video lessons, quizzes, and interactive exercises that help reinforce key concepts.
- Math Forums and Discussion Boards: Websites like Stack Exchange or Reddit’s math communities allow students to ask specific questions and receive answers from peers or experts. These forums are a great way to get help on tricky problems or discuss broader concepts.
- YouTube Channels: Many educators and math enthusiasts upload tutorials and problem-solving guides on YouTube. Channels like PatrickJMT, Professor Leonard, or 3Blue1Brown provide clear, detailed explanations on various math topics.
- Math Apps: Apps like Wolfram Alpha or Photomath can help students quickly solve problems and understand step-by-step solutions. While these tools are convenient, it’s important to understand the methods behind the solutions to fully grasp the material.
By utilizing these online tools, students can gain deeper insight into challenging concepts and improve their problem-solving abilities. Whether through interactive lessons, tutoring, or forums, the internet offers a wealth of resources that can make mastering math more accessible and manageable.
Mastering Advanced Math Word Problems
Word problems are a critical aspect of mastering complex mathematical concepts. They require students to translate real-world scenarios into mathematical expressions and equations. While they can initially seem overwhelming, breaking them down step by step can make them more manageable. The key to solving these problems is to carefully read the problem, identify the important information, and translate it into a form that can be solved with mathematical tools.
1. Understanding the Problem
The first step in solving any word problem is to fully understand what is being asked. Carefully read the problem and identify the known and unknown quantities. Pay attention to keywords that indicate mathematical operations such as “sum,” “difference,” “product,” or “quotient.” This will help you determine the types of equations or methods you need to apply.
2. Organizing the Information
Once you’ve understood the problem, the next step is organizing the information. Writing down the key details in a structured manner helps you visualize the problem and makes it easier to identify relationships between different elements. Setting up a table or diagram is often helpful in clarifying complex relationships.
| Step | Action | Example |
|---|---|---|
| Step 1: | Identify the variables and operations needed. | In a problem involving motion, define variables for speed, distance, and time. |
| Step 2: | Translate the word problem into an equation. | Use the formula distance = speed × time to set up the equation. |
| Step 3: | Solve the equation step by step. | Substitute known values and solve for the unknown quantity. |
By following this approach, students can develop a clearer understanding of the problem and apply the right mathematical tools to find the solution. Over time, practicing this method will increase speed and accuracy in solving word problems.
Common Mistakes in Advanced Math
While studying complex mathematical concepts, students often encounter common errors that can hinder their progress. These mistakes usually stem from misunderstanding key principles, misapplying formulas, or rushing through calculations. Recognizing these errors early on can help improve problem-solving skills and lead to better results. Below are some of the most frequent mistakes students make and tips on how to avoid them.
1. Misinterpreting Word Problems
Word problems often confuse students because they require translating a real-world situation into a mathematical expression. A common mistake is not identifying the right variables or operations needed to solve the problem. To avoid this, always take time to carefully read the problem and underline the key information. Create a clear outline of the steps involved and convert the problem into an equation systematically.
2. Incorrect Use of Formulas
Another frequent mistake is applying the wrong formula or misapplying a formula. For example, using the distance formula when the problem requires the area formula can lead to incorrect answers. It’s essential to review the conditions of the problem and ensure that the correct formula is used. Practice with different types of problems to reinforce your understanding of when and how to apply each formula.
3. Arithmetic Errors
Simple arithmetic mistakes, such as adding or subtracting incorrectly, often go unnoticed but can significantly affect the outcome. When solving problems, double-check all calculations before finalizing the answer. Use tools such as calculators for large numbers, and practice mental math to improve accuracy over time.
4. Skipping Steps
In an effort to solve a problem quickly, students sometimes skip intermediate steps. This can lead to confusion later on, especially if the solution path becomes complicated. Always write out each step clearly, even if it feels repetitive. This not only ensures accuracy but also helps in understanding the process more thoroughly.
5. Failing to Check Work
After completing a problem, many students overlook the importance of checking their work. A simple review can help identify errors that might otherwise be missed. After solving a problem, go back and review each step. Verify that the solution makes sense in the context of the problem.
By understanding and avoiding these common mistakes, students can improve their performance in solving complex mathematical problems and build stronger problem-solving habits.
How to Study Advanced Mathematics Efficiently
Studying complex mathematical concepts requires a focused approach that enhances understanding and retention. Efficient study techniques not only improve your problem-solving skills but also reduce frustration when tackling difficult topics. By organizing your study time, practicing regularly, and using active learning strategies, you can master even the most challenging material. Below are some key methods to study mathematics effectively.
1. Break Down the Material
One of the best ways to approach advanced mathematical topics is to break them down into smaller, more manageable chunks. Start by reviewing each concept separately, rather than trying to understand everything all at once. For instance, when studying equations or functions, focus on understanding one type of equation before moving on to another. This approach ensures a deeper understanding of each concept and reduces the feeling of being overwhelmed.
2. Practice Regularly
Mathematics is a skill that improves with practice. Set aside dedicated time each day to work through practice problems. Begin with easier problems and gradually move on to more difficult ones as your confidence grows. The more problems you solve, the more comfortable you will become with different problem types and techniques. Repetition is key to reinforcing your understanding and developing problem-solving fluency.
3. Understand the Theory Behind the Concepts
While it might be tempting to focus only on solving problems, it’s equally important to understand the theory behind the concepts. Knowing the “why” behind a formula or method helps you apply it more effectively in various situations. Take time to review the foundational principles that support the techniques you’re using, and don’t hesitate to revisit earlier lessons when you encounter difficulties.
4. Use Active Learning Techniques
Active learning involves engaging with the material in a way that deepens your understanding. Instead of passively reading through examples, try to explain the steps to yourself or even to others. Teaching a concept is one of the most effective ways to reinforce your knowledge. Additionally, use tools like flashcards or mind maps to organize and review key concepts regularly.
5. Seek Help When Needed
If you find yourself struggling with a particular topic, don’t hesitate to ask for help. Whether from a teacher, tutor, or online resources, seeking clarification early can prevent misunderstandings from snowballing. It’s better to address confusion before it becomes a bigger issue. Remember, understanding complex material often requires a collaborative approach.
6. Review and Test Yourself
After learning a new topic, take the time to review your notes and test yourself. Regularly self-assessing your knowledge helps identify areas that need further attention. Practice quizzes or old exams are excellent tools to gauge your progress and prepare for upcoming assessments.
By following these strategies, you can study efficiently and build a solid foundation for mastering even the most complex mathematical topics. Consistency, practice, and understanding the theory behind the methods will lead to success in the subject.
Using Mathematics Resources Effectively
When tackling challenging mathematical concepts, utilizing various learning tools and resources can significantly enhance understanding and problem-solving abilities. These resources range from online platforms and practice exercises to guidebooks and tutoring services. By effectively integrating these materials into your study routine, you can gain deeper insights, clarify doubts, and reinforce what you’ve learned.
Exploring Online Platforms

Online educational platforms are an excellent way to supplement your studies. Websites and apps offer interactive lessons, tutorials, and exercises tailored to various topics. Many platforms allow you to practice problems and receive instant feedback, which helps identify mistakes and understand the correct methods. Some platforms also feature video explanations, which can make complex topics easier to digest. Platforms such as Khan Academy, Coursera, or Mathway provide a wealth of resources that can support your learning journey.
Using Practice Problems and Solution Guides

Working through practice problems is a critical part of mastering any mathematical subject. Solution guides are valuable tools that can provide step-by-step explanations of how to solve different problem types. These guides help you identify patterns and strategies that work best for different kinds of exercises. Moreover, when used in conjunction with your own attempts, solution guides give you the opportunity to compare your work, correct mistakes, and refine your approach.
Incorporating resources like practice exercises, tutorials, and solution guides into your study sessions will help solidify your understanding and prepare you for more advanced topics. By diversifying your study materials, you can find new ways to approach difficult problems and enhance your overall comprehension.
How to Check Your Mathematics Solutions

After completing a math problem, it’s essential to verify your results to ensure accuracy. Checking your solutions helps identify any mistakes made during the process and reinforces your understanding of the steps involved. Several techniques can assist in validating your work, from reviewing each calculation carefully to using alternative methods to solve the same problem.
Step-by-Step Verification

One of the most effective ways to check your solution is by retracing your steps. Go through each part of the problem again, starting from the beginning. Check if you followed the correct order of operations, applied the right formulas, and made accurate calculations. If your final result matches the original steps, there’s a higher chance that your solution is correct. If discrepancies arise, locate where the error occurred and correct it.
Using Alternative Methods
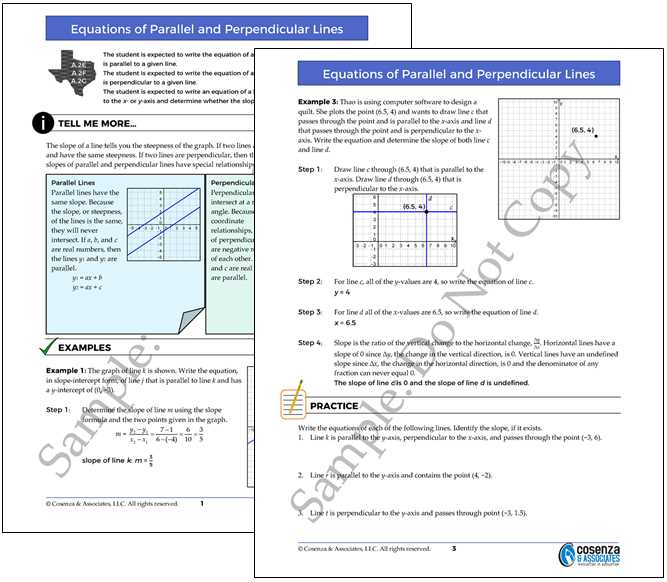
Another useful strategy is solving the same problem in a different way. For example, if you solved a problem by substitution, you can try using a graphing method or an elimination approach. When two or more methods produce the same result, you can be more confident that your solution is correct. This process not only verifies the accuracy of your work but also strengthens your understanding of various problem-solving techniques.
By applying these verification methods, you can reduce errors and improve your confidence in solving complex problems. Remember that consistency is key to mastering mathematical concepts, and regularly checking your solutions will enhance your problem-solving skills over time.
Mathematics Practice Exercises and Solutions
Practicing problems is essential for reinforcing your understanding of mathematical concepts and improving problem-solving skills. By working through various exercises, you not only apply what you’ve learned but also identify areas where further clarification is needed. The key to mastering any topic is consistent practice, and having access to step-by-step solutions can guide you in understanding the process behind each answer.
Practice exercises come in a wide range of difficulty levels, from basic operations to more advanced problem types. Each exercise provides an opportunity to explore different aspects of the subject matter. To get the most out of your practice sessions, it’s important to attempt the problems first before referring to solutions. This allows you to test your knowledge and develop a more independent approach to solving problems.
Once you’ve completed the exercises, reviewing the solutions can be incredibly helpful. By comparing your approach with the correct steps, you can identify any mistakes or misunderstandings. Solutions should not just serve as answers, but as tools to improve your reasoning and enhance your understanding of why certain methods work. They help you see the correct process and reasoning involved in each step, allowing you to replicate this logic in future problems.
Incorporating regular practice and reviewing solutions effectively will significantly enhance your ability to solve mathematical problems confidently and accurately. The more you practice, the more familiar and intuitive the concepts will become, making challenging problems easier to tackle.
Improving Your Mathematics Test Scores
Achieving high scores in math assessments requires more than just understanding the concepts; it involves developing effective study habits, mastering key strategies, and consistently practicing problem-solving techniques. While the material may seem challenging at first, with the right approach, you can greatly improve your performance on tests and exams.
Effective Study Techniques
To improve your test scores, start by organizing your study sessions and breaking down the material into manageable chunks. Focus on one concept at a time, ensuring that you fully understand each before moving on to the next. Active learning techniques such as solving problems, explaining concepts out loud, and teaching others can reinforce your understanding.
It’s also important to make use of various resources, including study guides, online tutorials, and practice tests. These tools can provide different perspectives on the same material, helping you solidify your knowledge. Reviewing notes regularly, rather than cramming the night before the test, allows the information to be stored in your long-term memory.
Test-Taking Strategies
On the day of the test, it’s important to stay calm and focused. Start by reading through the entire test to get an overview of the questions. Then, tackle the problems you feel most confident about first, leaving the more difficult ones for later. If you’re stuck on a question, move on and return to it once you’ve completed the rest of the test.
Time management during the test is crucial. Keep track of how long you spend on each section to ensure you have enough time to address every question. Double-check your answers before submitting the test to catch any errors you may have missed initially. The more you practice under timed conditions, the more comfortable you’ll become with managing your time during the actual test.
Improving test scores is a gradual process that requires consistent effort and dedication. By using effective study methods and test-taking strategies, you can increase your understanding of the material and achieve better results on your next math test.
Benefits of Reviewing Math Problem Solutions
Reviewing solutions to mathematical problems is an essential practice for mastering the subject. When students work through problems and examine the provided solutions, they can identify patterns, reinforce their understanding, and learn from their mistakes. This approach is a valuable tool for improving both conceptual comprehension and problem-solving skills.
Here are some key benefits of reviewing solutions:
- Clarifies Mistakes: Going over solutions helps students pinpoint where they went wrong in their calculations or reasoning. This reflection allows them to correct misunderstandings and prevent the same mistakes in the future.
- Strengthens Understanding: When students see a worked-out solution, they can understand the reasoning behind each step. This strengthens their grasp of the underlying concepts and techniques used to solve the problem.
- Improves Problem-Solving Skills: By regularly reviewing how problems are solved, students can enhance their ability to apply similar techniques to new, unfamiliar problems. This helps develop critical thinking and adaptability.
- Boosts Confidence: Seeing solutions clearly laid out helps build confidence. Students can understand how to approach complex problems and recognize their progress, which motivates them to tackle more challenging questions.
- Prepares for Future Challenges: Reviewing solutions also prepares students for more advanced topics. By mastering the current material, they lay a solid foundation for more difficult problems encountered later in their studies.
Ultimately, revisiting the solutions not only helps students improve their current performance but also sets them up for long-term success in mathematics. Consistent review helps students build a deeper understanding and fosters a proactive attitude toward learning.