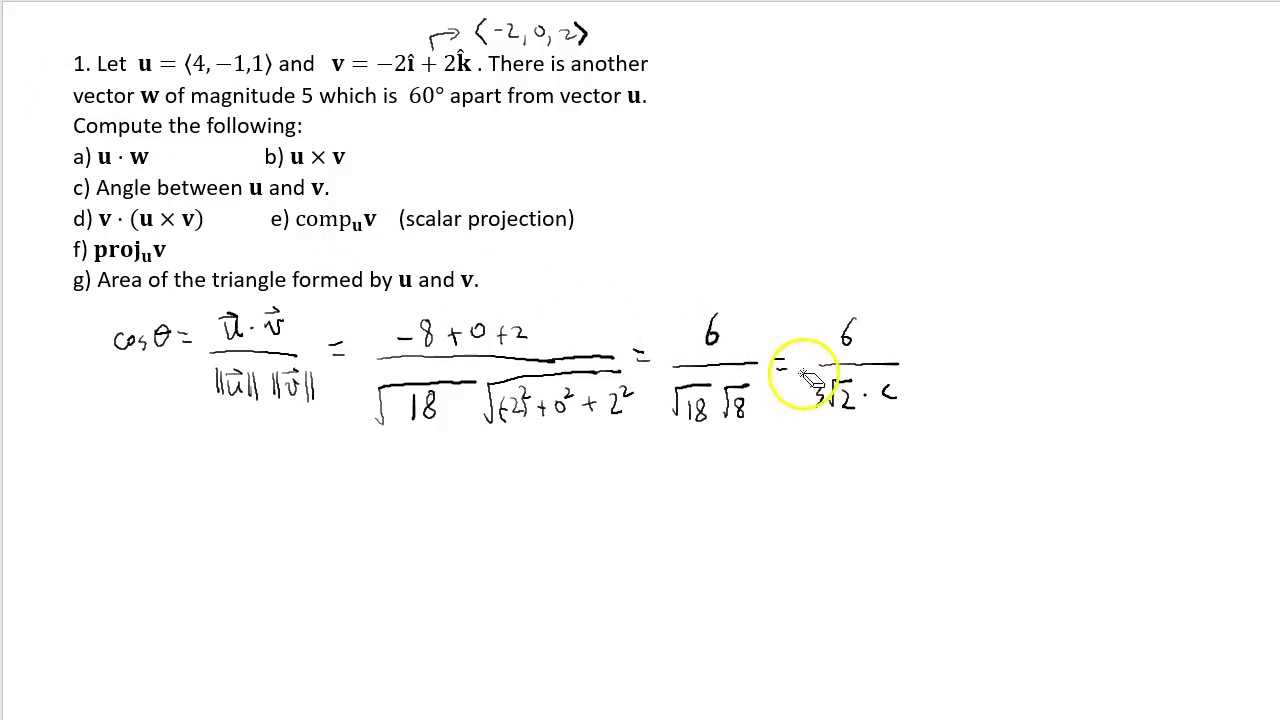
Achieving success in mathematical evaluations requires a solid grasp of key principles and the ability to apply them effectively. These assessments often challenge students to solve intricate problems, demonstrating both knowledge and analytical thinking.
This article provides clear guidance to enhance your preparation process. By exploring step-by-step explanations and practical methods, you’ll gain the confidence needed to tackle even the most difficult tasks. The material is designed to support learning through accessible techniques and proven strategies.
Whether you’re reviewing core topics or practicing advanced problem-solving, the insights shared here will serve as valuable tools for achieving academic goals. Dive into the tips and approaches that have helped countless learners excel in their studies.
Effective Strategies for Calculus Exam Success
Preparing for significant assessments in mathematical disciplines requires a thoughtful approach and careful planning. Success depends not only on understanding key principles but also on applying efficient techniques to solve complex problems under time constraints.
Organizing Study Sessions for Maximum Productivity

Structured preparation is essential for retaining essential knowledge. Divide your study time into manageable sections, focusing on specific topics during each session. Incorporate breaks to maintain concentration and reduce mental fatigue. A consistent schedule helps reinforce learning and builds a strong foundation for tackling even the most challenging problems.
Strengthening Problem-Solving Skills
Regularly practicing a variety of exercises is crucial for improving accuracy and speed. Work on problems of varying difficulty to ensure a comprehensive understanding of different scenarios. Analyze mistakes to identify weak areas and refine your approach, turning errors into opportunities for growth.
Understanding Key Concepts in Differential Calculus
To excel in mathematical assessments, it is crucial to comprehend the foundational principles that underlie the analysis of rates of change and dynamic behavior of functions. A strong grasp of these concepts allows for effective problem-solving and deeper insight into complex mathematical scenarios.
The following table outlines some of the most important topics to focus on for mastering the key principles:
| Concept | Description |
|---|---|
| Derivative | Measures the rate at which a function changes at a given point, essential for analyzing motion and trends. |
| Limits | Defines the behavior of a function as it approaches a specific point, forming the foundation for defining derivatives. |
| Continuity | Ensures that a function behaves smoothly without jumps or breaks, which is key for accurate modeling. |
| Chain Rule | Allows the calculation of the derivative of composite functions, crucial for handling more complex expressions. |
By mastering these fundamental principles, you will be better prepared to tackle both basic and advanced problems, making your approach to analysis more efficient and accurate.
How to Tackle Integration Problems Efficiently

Solving problems involving the accumulation of quantities requires a clear understanding of key techniques and an organized approach. By focusing on structured methods, complex tasks can be broken down into manageable steps, ensuring greater accuracy and efficiency.
Breaking Down Complex Expressions
Begin by simplifying the given problem as much as possible. Look for patterns or substitutions that can make the task less cumbersome. Methods like partial fractions or trigonometric identities often reduce complicated expressions into simpler forms, making them easier to integrate.
Choosing the Right Method

Selecting an appropriate strategy is essential for efficient problem-solving. Techniques such as substitution and integration by parts are powerful tools for a wide range of problems. Evaluate the problem carefully to decide which approach will yield results most effectively.
With practice and a systematic approach, solving accumulation-related challenges becomes more intuitive, allowing you to handle even the most intricate scenarios with confidence.
Common Mistakes to Avoid During the Exam
During assessments, even well-prepared individuals can make avoidable errors that hinder performance. Recognizing these pitfalls ahead of time can help you approach problems more effectively and maximize your score.
Overlooking Instructions: Always read the questions carefully to understand what is being asked. Missing key details can lead to solving the wrong problem or using inappropriate methods.
Skipping Step-by-Step Solutions: Attempting to jump to the final result without showing intermediate steps can lead to small but critical errors. Writing out each calculation helps ensure accuracy and allows you to identify mistakes quickly.
Mismanaging Time: Spending too long on a single problem can leave you with insufficient time to address other questions. Prioritize tasks by tackling simpler ones first and revisiting more complex problems later.
By staying mindful of these common errors, you can approach assessments more strategically and improve your chances of success.
Step-by-Step Guide to Solving Equations
Approaching mathematical problems methodically is crucial to finding accurate solutions. By following a clear, step-by-step process, you can break down complex problems and reach correct results more efficiently.
Understanding the Problem
The first step is always to carefully read the equation and identify what is being asked. Understanding the given variables and relationships will help you determine the appropriate method to use.
Step-by-Step Process
Follow these organized steps to solve equations effectively:
- Isolate the variable – Begin by moving terms involving the unknown to one side of the equation and constants to the other side.
- Simplify the equation – Combine like terms and eliminate any unnecessary factors to make the equation easier to solve.
- Apply appropriate operations – Use addition, subtraction, multiplication, or division to isolate the variable. If necessary, apply advanced techniques like factoring or using the quadratic formula.
- Verify your solution – Substitute your answer back into the original equation to ensure that it satisfies the equation.
By following these clear steps, you can systematically approach any equation and solve it with confidence.
Mastering Advanced Mathematical Topics with Ease
Achieving mastery in more complex areas of mathematics requires not only practice but also a deep understanding of underlying concepts. With the right approach, these advanced subjects can become more manageable and even intuitive.
Building a Strong Foundation

Before diving into advanced topics, ensure that your foundational knowledge is solid. Understanding the basic principles thoroughly will make it easier to grasp more challenging concepts and solve difficult problems effectively.
Breaking Down Complex Problems
When faced with intricate problems, break them down into smaller, more manageable parts. Identify key components and apply known techniques step by step, gradually building towards the solution. With consistent practice, even the most difficult problems will become more approachable.
By staying focused and continuously honing your skills, you will be able to navigate advanced mathematical topics with confidence and ease.
How Practice Tests Improve Your Performance
Engaging in practice tests is an essential strategy for enhancing your understanding and boosting your confidence. These mock assessments allow you to simulate real conditions, helping you prepare mentally and refine your problem-solving techniques.
By completing practice tests, you gain valuable insight into the format and structure of the questions. This familiarity reduces anxiety and helps you manage your time more effectively during the actual assessment. Moreover, practice tests highlight areas where you may need further review, giving you the opportunity to focus on weak spots and improve your overall performance.
Regularly practicing with these tests not only reinforces your knowledge but also increases your ability to think critically and adapt to different problem-solving approaches. This preparation will ensure you’re ready for any challenge that comes your way.
Tips for Managing Time During the Test
Effective time management is crucial to performing well under pressure. The ability to allocate time wisely allows you to address all questions efficiently while reducing stress. By planning ahead and following specific strategies, you can ensure that every minute counts.
Prioritize the Questions
Start by quickly scanning through the entire test to identify questions that are easier and quicker to solve. Prioritize these first, as they will help build momentum and save time for more complex problems later on. Allocate enough time to each section based on its difficulty, and make sure to leave time for reviewing your work.
Stay on Track and Avoid Getting Stuck
If you encounter a difficult question, avoid spending too much time on it. Instead, move on to the next question and return to the challenging one later. Keeping a steady pace throughout the test will prevent unnecessary pressure and help maintain focus on the tasks at hand.
By adopting these time-management strategies, you will not only enhance your efficiency but also reduce the chance of feeling overwhelmed during the test.
The Importance of Reviewing Class Notes
Reviewing class notes is an essential strategy for reinforcing understanding and retaining key concepts. Going over your notes allows you to refresh your memory, identify areas where you may need additional study, and strengthen your overall grasp of the material.
Why Review Notes?
Class notes serve as a personalized record of what was discussed during lessons, highlighting important points and methods. They act as a bridge between theoretical knowledge and practical application, helping you to recall details and connect ideas more effectively.
How to Maximize Your Review
When revisiting your notes, keep these strategies in mind:
- Focus on Key Concepts – Identify the main ideas or formulas that were emphasized in class. Pay particular attention to examples worked out by the instructor.
- Highlight Areas of Confusion – Mark sections that were unclear or require further explanation. These are areas where additional review or questions to your instructor may be helpful.
- Practice Problems – Include practice problems alongside your notes to apply the concepts. This reinforces understanding and prepares you for real-world applications of the material.
By regularly reviewing your notes, you ensure that essential concepts stay fresh in your mind and become second nature when solving problems.
Breaking Down Complex Word Problems
Word problems can often seem overwhelming, especially when they involve multiple steps or abstract concepts. However, with a clear approach, they can be broken down into manageable parts, making it easier to identify the necessary methods to solve them.
Approach to Solving Word Problems
Start by carefully reading the problem and identifying the key information. Highlight important values, relationships, or conditions mentioned in the text. Once you’ve gathered the facts, proceed with the following steps:
| Step | Description |
|---|---|
| 1. Identify the Unknowns | Determine what needs to be solved for. Label the unknowns with variables for clarity. |
| 2. Translate the Problem | Convert the word problem into a mathematical expression or equation that reflects the relationships described. |
| 3. Set Up Equations | Write out the necessary equations based on the relationships between the variables. |
| 4. Solve the System | Use algebraic methods to solve the system of equations or simplify the expression to find the solution. |
| 5. Interpret the Answer | After solving, check that the solution makes sense in the context of the original problem. Interpret it appropriately. |
By following this approach, word problems become less daunting, and you’ll be able to systematically work through even the most complicated scenarios.
Using Graphs to Solve Mathematical Questions
Visual representations can be a powerful tool when solving complex mathematical problems. By plotting functions on a graph, it is possible to gain valuable insights into their behavior, which can simplify the process of finding solutions and understanding relationships between variables.
Graphs can help illustrate critical aspects such as the function’s rate of change, maximum or minimum points, asymptotes, and areas under curves. These visual cues allow for quick identification of patterns, making it easier to apply the right methods to solve problems.
For example, the graph of a function can help identify key points where certain conditions, like tangents or intersections, are met. By observing these points, one can determine the appropriate calculations or transformations needed to find the desired result.
In addition, when working with integrals or derivatives, the graph provides an intuitive understanding of what is happening to the function’s behavior, offering a clearer picture of how to approach the problem efficiently.
Applications of Mathematics in Real-World Scenarios
The principles of mathematics extend far beyond the classroom and can be applied to a wide range of practical situations. Whether in engineering, economics, or medicine, mathematical models are used to solve problems, optimize processes, and make informed decisions.
In real-world scenarios, mathematical concepts help professionals understand complex systems, predict future trends, and analyze data. Here are some common areas where these concepts are frequently applied:
- Physics and Engineering – Mathematical models are used to study forces, motion, and energy. For example, engineers use these concepts to design structures, cars, and even spacecraft.
- Economics and Finance – Mathematical techniques help in modeling market trends, calculating risk, and optimizing investments. Economists use these tools to predict inflation, growth, and employment rates.
- Medicine and Biology – Mathematical models are employed in genetics, drug development, and population studies. For instance, they help predict the spread of diseases or optimize treatment plans.
- Environmental Science – Mathematical models are used to study climate change, environmental pollution, and resource management, allowing scientists to develop strategies for sustainability.
- Computer Science and Technology – Algorithms, data analysis, and machine learning are grounded in mathematical principles that drive innovations in technology, from software development to artificial intelligence.
These applications show that mathematical concepts are not just theoretical; they have real, tangible benefits in a variety of fields that affect everyday life. By leveraging these tools, we can better understand and influence the world around us.
Top Resources for Preparation

When preparing for an important assessment, having access to the right materials can make a significant difference. From textbooks to online platforms, the following resources are valuable tools that can help you sharpen your skills and boost your confidence.
Here are some top resources that can aid in your preparation:
| Resource | Benefits |
|---|---|
| Textbooks | Comprehensive coverage of the subject matter, detailed explanations, and practice problems. Ideal for structured study and reference. |
| Online Learning Platforms | Interactive lessons, video tutorials, and practice exercises. Allows for flexible learning and access to expert instructors. |
| Practice Papers | Helps familiarize with the format of questions, time management, and problem-solving strategies. Key to building confidence and reducing test anxiety. |
| Study Groups | Collaborative learning allows for exchange of ideas, peer explanations, and mutual support. Can clarify doubts and deepen understanding. |
| Online Forums | Access to a community of learners and instructors who can answer questions, share tips, and discuss challenging concepts. |
| Flashcards and Mobile Apps | Convenient for on-the-go review. Great for memorizing key concepts and formulas quickly. |
Utilizing a combination of these resources will help ensure a well-rounded approach to your preparation. Experiment with different tools to find which ones best suit your learning style and needs.
How to Stay Focused on Test Day

On the day of an important assessment, maintaining mental clarity and concentration is crucial to performing at your best. It’s common to feel a bit anxious or distracted, but with the right strategies, you can stay focused and make the most of your abilities. This section will explore several practical techniques to help you keep your mind sharp and your attention on task.
1. Prepare Ahead of Time
Being well-prepared is one of the best ways to reduce anxiety and improve your focus. Ensure you have all the materials you need for the test, including pens, pencils, and any necessary identification. Additionally, reviewing your notes the night before can help refresh your memory and put you in the right frame of mind.
2. Mindful Breathing and Relaxation
Taking deep breaths and practicing mindfulness can help calm your nerves and clear your mind. Before the test starts, spend a few minutes focusing on your breath, inhaling deeply and exhaling slowly. This simple technique can help lower stress levels, improve concentration, and prepare you for a productive session.
3. Time Management During the Test
Managing your time effectively during the assessment is essential to avoid feeling overwhelmed. Take a moment at the beginning to skim through the questions and estimate how much time you should spend on each section. Stay aware of the clock, but don’t let it make you rush. Prioritize questions you’re confident in first, then move on to the more difficult ones.
By following these tips and staying calm, you can ensure that you perform to the best of your ability on test day.
Benefits of Group Study for Better Understanding
Collaborative learning can significantly enhance your grasp of complex concepts. When you study in a group, you gain access to diverse perspectives that may help clarify challenging topics. Working with others allows for shared knowledge and problem-solving, making it easier to understand difficult material and reinforcing your own learning through teaching others.
1. Exposure to Different Approaches
One of the major advantages of group study is the opportunity to learn from others. Each member of the group may approach problems from different angles, offering alternative methods or explanations. This can help you discover new techniques that might be more effective than those you previously used, deepening your understanding.
2. Enhanced Motivation and Accountability

Studying in a group setting can increase motivation. Knowing that you are part of a team and that your peers rely on you can keep you accountable. This structure can encourage you to stay on track and stay consistent with your preparation, avoiding procrastination and maintaining focus throughout your study sessions.
Incorporating group study into your routine can create a dynamic and supportive learning environment, where you can collaborate, share insights, and deepen your comprehension of challenging subjects.
Building Confidence with Incremental Practice

Consistent, small steps in practice can significantly boost your self-assurance. By breaking down complex tasks into manageable pieces and progressively tackling them, you can build a solid foundation of skills and knowledge. This approach not only helps in mastering difficult material but also instills a sense of achievement as you see continuous improvement.
1. Start Small, Build Gradually
Begin with basic problems or concepts and gradually increase the difficulty as you become more comfortable. This incremental approach allows you to strengthen your skills without feeling overwhelmed. Each success, no matter how small, adds to your growing confidence.
2. Track Your Progress
Keep a record of your practice sessions, noting what you’ve learned and the challenges you’ve overcome. Reviewing your progress will highlight how far you’ve come, reinforcing your confidence in your abilities. Seeing improvement over time is one of the best motivators to continue practicing.
3. Revisit Challenging Topics
It’s natural to encounter difficult concepts along the way. However, revisiting these topics after some time allows for better understanding. Incremental practice involves repeated exposure to challenges, and with each attempt, you’ll find yourself more equipped to handle them effectively.
- Break large topics into smaller chunks.
- Review mistakes and learn from them.
- Celebrate small victories along the way.
By consistently practicing in a structured manner, you’ll find that your self-assurance grows steadily. Each session adds to your confidence, ensuring you’re well-prepared and poised for success.