To excel in your upcoming test, it is essential to have a solid grasp of the critical mathematical relationships that govern various scientific processes. Understanding these core formulas will not only help you solve problems more efficiently but also give you the confidence to tackle complex scenarios with ease.
In this section, we will explore the most vital concepts and calculations that frequently appear in the test. By mastering these principles, you will develop the analytical skills needed to approach any question methodically and accurately. These formulas cover a wide range of topics, from reaction rates to thermodynamic principles, offering a comprehensive overview of the subject’s essential components.
Grasping these fundamental tools allows you to recognize patterns and simplify intricate problems. Whether dealing with atomic interactions or energy changes, the ability to quickly apply these principles will ensure your readiness for any challenge presented during the assessment. Practice and familiarity with these key ideas will set you up for success.
Key Formulas for Success in Your Assessment
In any scientific evaluation, being able to quickly apply the correct relationships between variables is essential. These fundamental principles allow you to navigate through complex problems with ease. Understanding and mastering these vital expressions ensures that you can solve a variety of problems, whether dealing with molecular structures or energy transformations. Familiarity with these tools will give you a clear advantage when approaching different types of questions.
Thermodynamics and Energy Calculations
One of the most crucial areas of focus is the understanding of energy changes within systems. Formulas related to enthalpy, entropy, and Gibbs free energy are frequently tested. These expressions help in determining whether a reaction is spontaneous or requires external energy input. Key concepts such as ΔH (change in enthalpy) and ΔG (Gibbs free energy) are essential for predicting the direction of reactions.
Gas Laws and Stoichiometric Relationships
The behavior of gases and their interactions with other substances is another central theme. Equations like the ideal gas law PV = nRT (pressure, volume, and temperature relations) are frequently applied in problems involving gas mixtures, stoichiometry, and molecular motion. Similarly, understanding the relationships between reactants and products in a reaction is vital for solving problems related to limiting reagents, yield calculations, and concentration determination.
Mastering these expressions will enhance your ability to tackle a broad range of questions and approach them with confidence. Regular practice and application of these principles will ensure that you’re well-prepared for any scenario presented during the test.
Fundamental Concepts in Chemical Reactions
Understanding the underlying principles of molecular transformations is essential for solving problems related to reaction mechanisms and their outcomes. These concepts serve as the foundation for interpreting how substances interact, combine, and break apart under various conditions. Mastering these ideas will allow you to predict the behavior of systems and calculate the necessary parameters to describe those changes accurately.
The study of these core principles includes topics such as reaction rates, stoichiometry, and equilibrium. By analyzing the relationships between reactants and products, it becomes possible to determine factors like limiting reagents, yield, and the direction of a reaction. Furthermore, recognizing the impact of external conditions, such as temperature and pressure, is critical in understanding how they influence the speed and extent of chemical transformations.
Grasping these foundational concepts not only helps in solving typical reaction-based problems but also in applying advanced theories to new situations. Whether dealing with simple or complex reactions, these principles form the bedrock of scientific problem-solving.
Stoichiometry and Mole Relationships
In chemical processes, understanding the relationships between different substances is vital for accurately determining quantities and predicting the outcomes of reactions. This area focuses on how the amounts of reactants and products relate to one another, enabling the calculation of various quantities such as mass, volume, and molar amounts. Mastering these relationships is essential for performing precise calculations and ensuring that the required amounts of materials are used in reactions.
Converting Between Moles and Mass
The concept of the mole is fundamental in understanding the scale of chemical reactions. One mole of a substance corresponds to a specific number of particles, known as Avogadro’s number, and can be used to relate the mass of a substance to the amount of particles it contains. By using molar masses, it’s possible to convert between mass and moles, which is essential for determining how much of each reactant or product is involved in a given reaction.
Balancing Reactions and Mole Ratios

Once a reaction is balanced, the stoichiometric coefficients give the mole ratios of the reactants and products. These ratios are used to calculate how much of one substance is required to react with a given amount of another. By applying these mole ratios, you can predict the amount of product formed from a certain quantity of reactants or determine how much of a substance is needed to complete a reaction. This principle is central to solving many types of reaction-related problems.
Understanding Ideal Gas Laws
The behavior of gases is governed by a set of principles that describe the relationship between pressure, volume, temperature, and the number of particles in a given sample. These fundamental rules allow us to predict how gases will respond under various conditions. By understanding these laws, you can solve a wide range of problems related to gas behavior, from determining how much gas will occupy a space to calculating how pressure changes with temperature.
One of the most important laws in this area is the ideal gas law, which serves as a foundation for understanding how gases behave under ideal conditions. While real gases may deviate slightly from this model, the ideal gas law provides a useful approximation in most situations. Here are the key relationships to consider:
- Pressure (P): The force exerted by gas molecules as they collide with the walls of their container.
- Volume (V): The space occupied by the gas, often measured in liters or cubic meters.
- Temperature (T): The average kinetic energy of gas molecules, typically expressed in Kelvin.
- Number of Moles (n): The quantity of gas molecules present, usually measured in moles.
To better understand how these properties interact, the ideal gas law is often written as:
PV = nRT
Where R is the ideal gas constant, which varies depending on the units used for pressure and volume. This law is used to calculate any unknown property when the others are known, making it a powerful tool in solving problems related to gases.
By practicing problems involving the ideal gas law and understanding how these variables interact, you will be better prepared to tackle questions involving gas behavior in various scenarios.
Thermodynamics and Energy Calculations
Understanding the transfer and transformation of energy is essential for analyzing reactions and predicting their behavior. Thermodynamics is the branch of science that studies how energy changes form, particularly between heat and work, during chemical processes. By mastering the principles of energy conservation and flow, you can accurately determine the feasibility and direction of reactions, as well as calculate the energy required or released in these processes.
First Law of Thermodynamics
The first law, also known as the law of energy conservation, states that energy cannot be created or destroyed, only converted from one form to another. In chemical reactions, this means that the total energy before and after the process remains constant, though it may change forms, such as from potential energy to kinetic energy or from heat to work. The relationship between internal energy, heat, and work can be expressed as:
ΔU = Q - W
Where ΔU is the change in internal energy, Q is the heat added to the system, and W is the work done by the system. This principle is foundational when studying the energy changes involved in reactions.
Gibbs Free Energy and Spontaneity
Gibbs free energy is a key concept for determining whether a reaction will proceed spontaneously under constant temperature and pressure. It combines enthalpy (heat content) and entropy (disorder) to predict the spontaneity of a process. The equation for Gibbs free energy is:
ΔG = ΔH - TΔS
Where ΔG is the change in Gibbs free energy, ΔH is the change in enthalpy, T is the temperature in Kelvin, and ΔS is the change in entropy. A negative ΔG indicates a spontaneous reaction, while a positive ΔG suggests that the reaction is non-spontaneous under the given conditions.
By understanding these principles and performing the necessary calculations, you can predict whether a reaction will require external energy input or release energy, and how it will proceed under different conditions.
Equilibrium Constant Formulas
In chemical processes, reactions reach a state where the concentrations of reactants and products remain constant over time. This balance, known as equilibrium, can be described quantitatively through specific formulas that relate the concentration of substances to one another. Understanding how to calculate and interpret these constants is essential for analyzing how reactions proceed under various conditions and predicting how they will respond to changes in temperature, pressure, or concentration.
Defining the Equilibrium Constant (K)
The equilibrium constant, K, is a ratio that expresses the relative concentrations of products and reactants at equilibrium. It is derived from the balanced chemical equation of the reaction. The general form of the expression for a reversible reaction is:
K = [products]^coefficients / [reactants]^coefficients
Here, the square brackets represent the concentrations of the products and reactants, and the exponents correspond to the stoichiometric coefficients from the balanced equation. A large value of K indicates that the products are favored at equilibrium, while a small value suggests that the reactants dominate.
Relating Kp and Kc
When dealing with gaseous reactions, the equilibrium constant can be expressed in terms of partial pressures. In this case, the constant is denoted as Kp>, and the formula relates the partial pressures of products and reactants. For reactions involving gases, you can convert between the concentration-based constant Kc and Kp using the following formula:
Kp = Kc(RT)^Δn
Where R is the ideal gas constant, T is the temperature in Kelvin, and Δn is the change in the number of moles of gas between the products and reactants. This relationship is important when working with reactions that involve gases under non-ideal conditions.
By understanding these formulas and how to manipulate them, you can predict how changes in concentration, pressure, or temperature will affect the position of equilibrium in a reaction.
Acid-Base Equilibria and pH
Understanding the behavior of acids and bases in solution is fundamental for analyzing a wide range of chemical processes. The balance between acidic and basic components in a system plays a crucial role in determining properties such as reactivity, solubility, and biological functions. At the heart of acid-base behavior lies the concept of equilibrium, where the concentrations of hydrogen ions (H+) and hydroxide ions (OH–) are balanced in a solution.
One of the most important concepts in this context is the pH scale, which measures the acidity or basicity of a solution. pH is a logarithmic scale that represents the concentration of hydrogen ions in a given solution. A low pH indicates an acidic solution, while a high pH indicates a basic (alkaline) solution. Understanding how to calculate and interpret pH is crucial for determining how an acid or base will behave in different environments.
pH and pOH Relationships
The pH of a solution is directly related to the concentration of hydrogen ions, and can be calculated using the formula:
pH = -log[H+]
Similarly, the pOH of a solution is related to the concentration of hydroxide ions and is given by:
pOH = -log[OH-]
Since the sum of pH and pOH always equals 14 at 25°C, you can easily convert between them using the following relationship:
pH + pOH = 14
Buffer Systems and Their Role
Buffer systems are solutions that resist changes in pH when small amounts of acid or base are added. These systems are essential for maintaining stable pH conditions in biological and chemical processes. A buffer consists of a weak acid and its conjugate base (or a weak base and its conjugate acid). The effectiveness of a buffer is determined by its ability to absorb excess hydrogen ions or hydroxide ions without significantly changing the pH.
By understanding the principles of acid-base equilibria, pH, and buffer systems, you can predict how substances will behave in different conditions, which is crucial for solving a variety of problems in both laboratory and real-world scenarios.
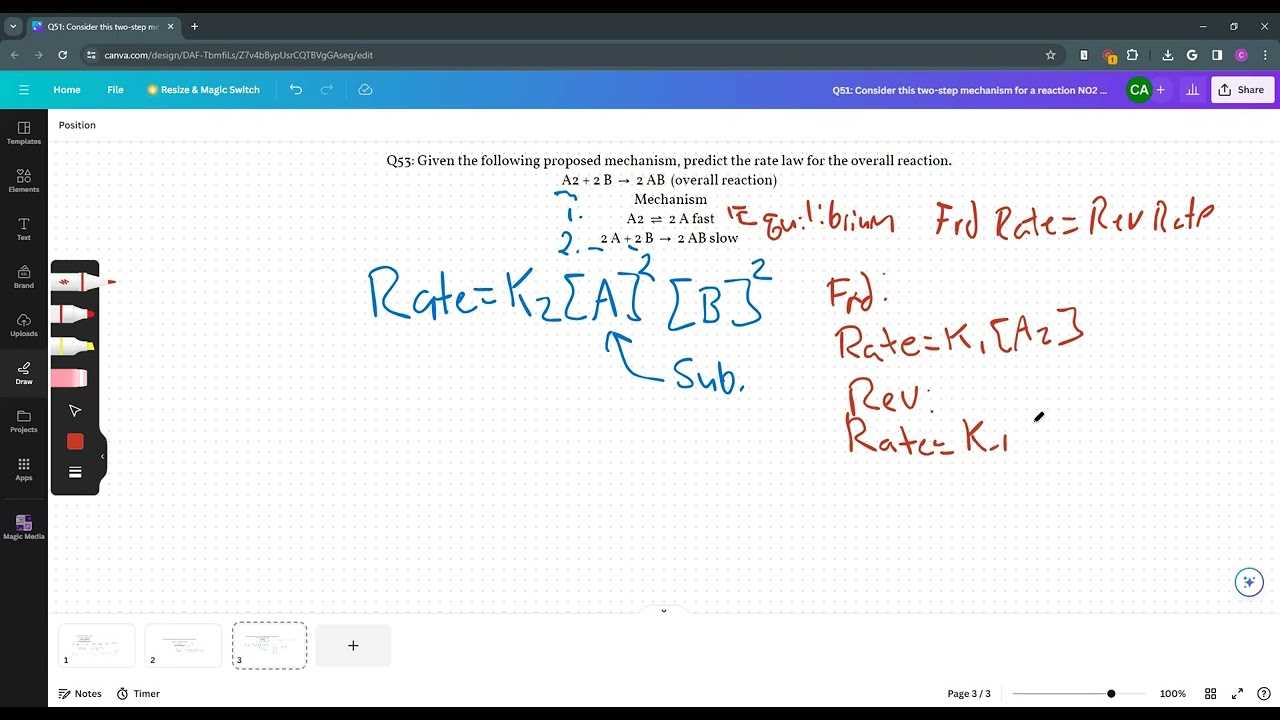
Reaction Rates and Rate Laws
The speed at which a chemical reaction occurs depends on various factors, including the concentration of reactants, temperature, and the presence of catalysts. Understanding how these factors influence the rate of a reaction is essential for predicting reaction behavior and optimizing conditions for industrial and laboratory processes. Reaction rates are typically expressed as the change in concentration of reactants or products over time.
To quantitatively describe how the concentration of reactants affects the rate of a reaction, we use rate laws. A rate law is an equation that links the rate of reaction to the concentration of reactants, with each concentration term raised to a power that represents the reaction’s order with respect to that reactant. The rate constant, k, is a proportionality factor that depends on the specific reaction and temperature.
General Form of a Rate Law
The general form of a rate law for a reaction can be written as:
Rate = k[A]^m[B]^n
Where Rate is the reaction rate, k is the rate constant, [A] and [B] are the concentrations of reactants, and m and n are the reaction orders with respect to each reactant. The reaction order is determined experimentally and indicates how the concentration of a given reactant affects the reaction rate. If m or n is 1, the rate is directly proportional to the concentration of that reactant. If it is 2, the rate is proportional to the square of the concentration.
Factors Affecting Reaction Rates
Several factors influence the rate at which reactions occur. These include the concentration of reactants, temperature, surface area, and the presence of catalysts. Below is a table summarizing how each factor typically affects reaction rates:
| Factor | Effect on Reaction Rate |
|---|---|
| Concentration of Reactants | Increasing concentration generally increases the rate of reaction (more collisions between reactant molecules). |
| Temperature | Raising the temperature typically increases the reaction rate by providing more energy for collisions. |
| Surface Area | Smaller particle size or increased surface area accelerates the reaction rate by exposing more reactant particles to collisions. |
| Catalysts | Catalysts increase the reaction rate by lowering the activation energy required for the reaction to proceed, without being consumed in the process. |
By understanding and applying rate laws, you can gain insight into how chemical reactions progress and identify ways to control reaction conditions to achieve desired outcomes efficiently.
Electrochemistry and Cell Potentials

Electrochemistry explores the relationship between chemical reactions and electrical energy. It is a field that examines how chemical energy can be converted into electrical energy and vice versa. This concept is essential in various applications, from powering batteries to understanding corrosion processes. Central to this field is the study of redox reactions, where electrons are transferred between substances, often generating an electric current in the process.
In electrochemical cells, a potential difference exists between two electrodes, which drives the movement of electrons through an external circuit. This potential difference, known as the cell potential, is a key factor in determining the cell’s ability to produce electrical energy. The voltage or electric potential of a cell depends on the nature of the half-reactions occurring at the electrodes, as well as the concentrations of ions in the solutions involved.
Standard Cell Potentials
The standard cell potential is determined under standard conditions, typically at 25°C, 1 atm pressure, and 1 M concentration for each ion involved. It is the difference between the reduction potential of the cathode and the anode. A positive standard cell potential indicates a spontaneous reaction, meaning the reaction can occur without external energy input.
The cell potential is calculated using the standard reduction potentials of the half-reactions, which are typically tabulated for various substances. The reduction potential of a substance indicates its tendency to gain electrons, and the more positive the value, the more likely it is to accept electrons in a redox process.
Relationship Between Cell Potential and Gibbs Free Energy
The cell potential is related to the Gibbs free energy change (ΔG) of the reaction. A positive cell potential corresponds to a negative Gibbs free energy, signifying that the process is thermodynamically favorable. The relationship between ΔG and cell potential is given by the following equation:
ΔG = -nFEcell
Where n is the number of moles of electrons transferred, F is the Faraday constant, and Ecell is the cell potential. This equation allows the prediction of whether a reaction will occur spontaneously based on the cell potential and the number of electrons involved.
By understanding the principles of electrochemistry and cell potentials, you can analyze and predict the behavior of electrochemical systems, which is vital in applications such as batteries, fuel cells, and corrosion prevention.
Bond Energy and Enthalpy Calculations
The concepts of bond energy and enthalpy are crucial in understanding how energy is involved in chemical reactions. Bond energy refers to the amount of energy required to break a specific bond in a molecule, while enthalpy is a thermodynamic property that represents the total heat content of a system. Both are key in determining whether a reaction is exothermic or endothermic, and they provide insight into the stability of reactants and products during a chemical transformation.
When chemical bonds are broken or formed, energy is either absorbed or released, affecting the overall heat exchange in a reaction. In this section, we explore how bond energies and enthalpy changes can be used to predict the heat involved in a reaction and to assess the energy dynamics of a system.
Calculating Enthalpy Change Using Bond Energies

The change in enthalpy (ΔH) for a reaction can be estimated by considering the bond energies of the reactants and products. The general approach is to subtract the total bond energy of the products from the total bond energy of the reactants. If the bond energy of the products is lower, the reaction is exothermic (releases energy), and if the bond energy of the products is higher, the reaction is endothermic (absorbs energy).
ΔH = Σ(Bond Energies of Reactants) - Σ(Bond Energies of Products)
In this formula, the sum of bond energies of the reactants is calculated, followed by the sum of bond energies of the products. The difference between these sums gives the enthalpy change of the reaction.
Hess’s Law and Enthalpy Calculations
Hess’s Law is another powerful tool for calculating enthalpy changes, which states that the total enthalpy change of a reaction is the sum of the enthalpy changes of the individual steps of the reaction, regardless of the pathway taken. This law allows for the calculation of enthalpy changes in reactions that cannot be measured directly, by breaking them into simpler reactions whose enthalpy changes are known.
The relationship can be written as:
ΔHreaction = ΣΔHproducts - ΣΔHreactants
By applying Hess’s Law, you can calculate the overall enthalpy change by adding or subtracting known enthalpy values for the individual steps in a reaction sequence.
Understanding bond energy and enthalpy is fundamental for predicting the direction of reactions and evaluating the energy efficiency of chemical processes. Whether considering a simple molecule or a complex multi-step reaction, these concepts provide essential insights into the energetics of chemical changes.
Gas Stoichiometry in Reactions
Gas stoichiometry is an essential concept when studying reactions involving gaseous substances. It involves understanding the relationships between the volumes, amounts, and conditions of gases participating in a reaction. By applying principles such as the ideal gas law, you can predict how gases behave during chemical processes and calculate important factors like the volume of gas produced or consumed under different conditions.
In reactions where gases are involved, stoichiometry allows you to determine the amounts of reactants and products based on the molar ratios derived from the balanced chemical equation. These relationships help in solving problems related to the volumes of gases, as well as their conversion to moles or masses, depending on the reaction conditions.
Ideal Gas Law and Stoichiometric Calculations
The ideal gas law is a powerful tool for gas stoichiometry calculations. It can be used to relate the volume of gas to its pressure, temperature, and the number of moles present. The ideal gas law is given by:
PV = nRT
Where P is the pressure, V is the volume, n is the number of moles, R is the ideal gas constant, and T is the temperature in Kelvin. By rearranging this equation, you can calculate any of these variables as long as the others are known. This law is particularly useful for solving gas-related stoichiometric problems in reactions.
Volume Relationships in Gas Reactions
In many reactions involving gases, the volume of reactants and products is directly related to their mole ratios. Avogadro’s law states that equal volumes of gases at the same temperature and pressure contain the same number of molecules. This means that in a balanced chemical equation, the volumes of gases are in the same ratio as their respective coefficients.
To perform stoichiometric calculations involving volumes of gases, follow these general steps:
- Balance the chemical equation for the reaction.
- Convert any given volume of gas to moles using the molar volume of gas at standard temperature and pressure (STP), which is 22.4 L/mol.
- Use the mole ratio from the balanced equation to find the amount of the other reactant or product.
- Convert the final result from moles back to volume, if necessary, using the ideal gas law or the molar volume at STP.
By applying these steps, you can solve a wide range of problems involving gases in reactions, whether you are dealing with simple reactions or more complex processes under varying conditions.
Kinetic Molecular Theory of Gases

The kinetic molecular theory explains the behavior of gases based on the motion of individual particles. This model assumes that gases consist of particles in constant, random motion, and that the temperature of a gas is directly related to the average kinetic energy of its molecules. Understanding this theory is fundamental to predicting how gases will react under different conditions, such as changes in pressure, volume, or temperature.
According to the kinetic molecular theory, the behavior of gases can be described by a few key postulates:
- The gas particles are in continuous, random motion and move in straight lines until they collide with other particles or the walls of the container.
- These particles are so far apart that the interactions between them are negligible except during collisions.
- The volume of individual gas particles is considered to be insignificant compared to the volume of the container.
- The average kinetic energy of gas particles is proportional to the temperature of the gas, measured in Kelvin.
- Collisions between gas particles are perfectly elastic, meaning there is no net loss of energy after a collision.
By applying these principles, it is possible to derive relationships between pressure, volume, temperature, and the number of particles in a gas, all of which are central to understanding how gases behave in different environments.
Relationship Between Temperature and Kinetic Energy
One of the key aspects of the kinetic molecular theory is the relationship between the temperature of a gas and the average kinetic energy of its molecules. As the temperature increases, the average speed of the gas particles increases, leading to higher kinetic energy. This can be mathematically expressed as:
K.E. = 1/2 mv²
Where m is the mass of a particle and v is its velocity. This relationship is crucial when analyzing the effect of temperature changes on the behavior of gases, such as their volume and pressure in various scenarios.
Application in Gas Laws
The kinetic molecular theory helps explain the ideal gas law and other gas laws, such as Boyle’s law, Charles’s law, and Avogadro’s law. For instance, when temperature rises, the kinetic energy of particles increases, which results in increased pressure if the volume is held constant. Similarly, when the volume of a gas is reduced, the particles have less space to move, causing more frequent collisions and an increase in pressure.
Understanding the kinetic molecular theory provides a deeper insight into how gases behave in real-world applications, such as in engines, weather systems, and even in the design of scientific instruments.
Limiting Reactants and Yield Calculations
In many chemical reactions, one of the reactants is consumed first, limiting the amount of product that can be formed. This is known as the limiting reactant. Understanding how to identify the limiting reactant and calculate the theoretical yield is crucial for predicting the outcome of a reaction and optimizing the efficiency of chemical processes.
When conducting a reaction, it’s essential to determine which reactant will run out first. This reactant will control how much product can be produced, and knowing this allows for accurate predictions of the amount of product formed. Additionally, the yield refers to the amount of product obtained, which is often compared to the theoretical yield to evaluate the efficiency of the reaction.
Identifying the Limiting Reactant

To determine which reactant is limiting, follow these steps:
- Calculate the number of moles of each reactant present.
- Use the stoichiometric coefficients from the balanced equation to compare the mole ratios of the reactants to determine which one will be completely consumed first.
- The reactant that is used up first is the limiting reactant.
By identifying the limiting reactant, you can determine how much product will be produced, as it dictates the maximum amount that can be formed.
Theoretical and Actual Yield
The theoretical yield is the amount of product that would be formed if the reaction proceeded perfectly, with no side reactions or losses. It is based on the limiting reactant. The actual yield, on the other hand, is the amount of product obtained in a real experiment, which may be less than the theoretical yield due to inefficiencies or incomplete reactions.
The percentage yield can be calculated using the formula:
Percentage Yield = (Actual Yield / Theoretical Yield) × 100
Understanding these concepts is crucial in lab settings and industrial applications, as it helps determine the efficiency of reactions and can guide improvements in chemical processes.
Solution Concentration and Molarity
Understanding the concentration of a solution is essential in many chemical processes. Concentration refers to how much solute is dissolved in a given amount of solvent, and it plays a critical role in determining how reactions proceed and the properties of the solution. One of the most common ways to express concentration is through molarity, which quantifies the amount of solute per liter of solution.
Knowing how to calculate and interpret solution concentration allows for precise control over reactions and is crucial for tasks such as preparing standard solutions, diluting substances, and performing titrations.
Molarity and Its Calculation
Molarity (M) is defined as the number of moles of solute per liter of solution. It is the most commonly used concentration unit in laboratories due to its simplicity and ease of application. The formula for molarity is:
M = moles of solute / liters of solution
To calculate molarity, you need to know the amount of solute in moles and the total volume of the solution in liters. This information allows you to determine how concentrated the solution is, which is crucial for accurate measurements in chemical experiments.
Changing Concentration through Dilution
When a solution needs to be diluted, it is important to maintain the relationship between the initial and final concentrations. Dilution involves adding solvent to reduce the concentration of solute in the solution. The dilution equation can be used to calculate the final concentration after dilution:
C1V1 = C2V2
In this equation:
- C1 = initial concentration
- V1 = initial volume
- C2 = final concentration
- V2 = final volume
This relationship ensures that you can prepare solutions of the desired concentration by adjusting the volume and concentration of the original solution appropriately. Proper understanding of molarity and dilution is vital for many laboratory applications, including quantitative analysis and titration experiments.
Buffer Systems and Their Equations
Buffer systems are essential in maintaining a stable pH level in various chemical environments. These systems consist of a weak acid and its conjugate base or a weak base and its conjugate acid. They are crucial for preventing significant pH fluctuations when acids or bases are added to a solution. By resisting changes in pH, buffer systems play a vital role in biological processes, laboratory experiments, and industrial applications.
In the context of acids and bases, a buffer system helps maintain the pH within a narrow range, ensuring that the conditions remain suitable for reactions to occur effectively. The effectiveness of a buffer system depends on the concentrations of the acid-base pair and the pH of the environment.
Henderson-Hasselbalch Equation
The Henderson-Hasselbalch equation provides a relationship between the pH of a buffer solution, the concentration of the acid and base components, and the pKa value of the acid. This equation is particularly useful in predicting the pH of a buffer solution when the concentrations of the acid and its conjugate base are known.
| Equation | Description |
|---|---|
| pH = pKa + log([A-]/[HA]) | This formula calculates the pH of a buffer solution, where pKa is the acid dissociation constant, [A-] is the concentration of the conjugate base, and [HA] is the concentration of the weak acid. |
In this equation:
- pKa: The acid dissociation constant, a measure of the strength of the acid.
- [A-]: Concentration of the conjugate base.
- [HA]: Concentration of the weak acid.
Buffer Capacity and pH Range

Buffer capacity refers to the ability of a buffer system to resist changes in pH when acids or bases are added. The greater the concentrations of the acid and base in the buffer, the higher the buffer capacity. Buffer systems are most effective within a certain pH range, typically within one pH unit above or below the pKa value of the acid in the system.
Understanding the relationship between the concentrations of the acid and conjugate base, along with the pKa value, allows for the preparation of buffer solutions with the desired pH. This knowledge is vital in a range of applications, from maintaining the proper pH in biological systems to controlling the pH of solutions in industrial processes.
Le Chatelier’s Principle in Action
When a chemical system is disturbed, it will naturally adjust to counteract the change and restore a state of equilibrium. This behavior, which is central to many dynamic processes, reflects how systems strive to maintain balance. Le Chatelier’s Principle provides insight into how a system will shift when subjected to changes in temperature, pressure, or concentration. By understanding this principle, we can predict how reactions will respond to various external factors, allowing for better control in laboratory and industrial settings.
This principle is crucial when dealing with reversible reactions, where the forward and reverse processes are in constant equilibrium. By manipulating the conditions surrounding these reactions, it is possible to favor one direction over the other, increasing the yield of desired products or improving reaction efficiency. The ability to anticipate and influence these shifts is a key concept in fields ranging from chemical engineering to environmental science.
Effect of Concentration Changes
When the concentration of one of the reactants or products is altered, the system will shift to favor the side that will counteract the change. If more reactant is added, the system will shift towards the product side to consume the excess reactant. Conversely, if product concentration is increased, the equilibrium will shift toward the reactant side to reduce the product concentration.
- Adding reactants: Shifts the equilibrium toward product formation.
- Removing reactants: Shifts the equilibrium toward reactant formation.
- Adding products: Shifts the equilibrium toward reactant formation.
- Removing products: Shifts the equilibrium toward product formation.
Effect of Temperature Changes
The temperature also plays a significant role in determining the direction of a reaction. In exothermic reactions, heat is released, and increasing the temperature shifts the equilibrium to favor the reverse reaction. In contrast, for endothermic reactions, heat is absorbed, and increasing the temperature will push the equilibrium toward the forward reaction, promoting more product formation.
- Increasing temperature in exothermic reactions: Shifts equilibrium toward the reactant side.
- Increasing temperature in endothermic reactions: Shifts equilibrium toward the product side.
Le Chatelier’s Principle is a powerful tool in manipulating chemical reactions. By understanding how the system responds to different changes, it becomes possible to optimize reactions and achieve the desired outcomes more efficiently. Whether in laboratory experiments, industrial applications, or natural processes, this principle provides a fundamental framework for understanding chemical equilibria.
Quantum Mechanics and Atomic Structure

The study of atomic and molecular behavior at the smallest scales is governed by the principles of quantum mechanics. This framework describes the motion and interactions of subatomic particles, such as electrons, and offers insights into the properties of atoms that classical physics cannot explain. Quantum theory not only helps in understanding atomic structure but also explains phenomena like energy quantization and the wave-particle duality of matter.
At the core of atomic structure lies the behavior of electrons, which are arranged in specific energy levels around the nucleus. These electrons do not follow simple paths, as once imagined in classical models, but rather occupy regions of space known as orbitals. The arrangement and energy of these orbitals determine the chemical properties of elements and their interactions with other atoms.
One of the foundational aspects of quantum mechanics is the concept of wave functions. These mathematical expressions describe the probability of finding an electron in a particular location at a given time. The energy levels associated with these wave functions are quantized, meaning electrons can only exist at certain discrete energy levels. This idea revolutionized our understanding of atomic stability and bonding.
To describe electron behavior more precisely, quantum mechanics uses the Schrödinger equation, a key tool that provides the wave function for a system. This equation is central to predicting electron distribution within an atom and forms the basis for understanding chemical bonding and molecular formation. Additionally, the Pauli Exclusion Principle and Hund’s Rule further refine how electrons fill atomic orbitals and interact within molecules.
As research in quantum mechanics progresses, it continues to uncover the underlying principles that govern atomic behavior. The quantum mechanical model provides the foundation for much of modern science, influencing fields like chemistry, material science, and nanotechnology.
Understanding the Nernst Equation

The Nernst relation is a crucial principle in electrochemistry that links the electrode potential of a half-reaction to the concentration of ions involved. This relationship allows us to determine the potential difference across an electrochemical cell under non-standard conditions, accounting for variations in concentration. By applying the Nernst relation, it becomes possible to predict the direction of a redox reaction and its spontaneity in a given environment.
At the heart of the Nernst relation is the balance between the chemical energy (due to ion concentration) and the electrical energy (due to charge separation). The equation adjusts the standard electrode potential to reflect the actual concentrations of the species involved, making it essential for understanding how concentration gradients impact the voltage and overall reaction behavior. This is especially important in biological systems, such as the membrane potentials in cells, where ion gradients dictate cellular functions.
The Nernst Relation Formula
The general form of the Nernst relation is expressed as:
E = E0 – (RT/nF) * ln([Coxidized]/[Creduced])
- E is the electrode potential under non-standard conditions.
- E0 represents the standard electrode potential.
- R is the gas constant (8.314 J/mol·K).
- T is the temperature in Kelvin.
- n is the number of electrons transferred in the reaction.
- F is Faraday’s constant (96,485 C/mol).
- [Coxidized] and [Creduced] are the concentrations of the oxidized and reduced species involved in the reaction.
Applications in Various Fields
This relation is not limited to theoretical studies but plays a significant role in practical applications, such as the design of batteries and fuel cells, as well as in understanding biochemical processes. In biological systems, the Nernst equation helps explain the electrochemical gradients across membranes and their role in processes like nerve signaling and muscle contraction.
By calculating the potential for reactions in real-world environments, the Nernst equation offers a valuable tool for chemists and biochemists alike, enhancing their understanding of redox processes in both synthetic and natural systems.