Core Connections Course 3 Chapter 4 Answers Guide

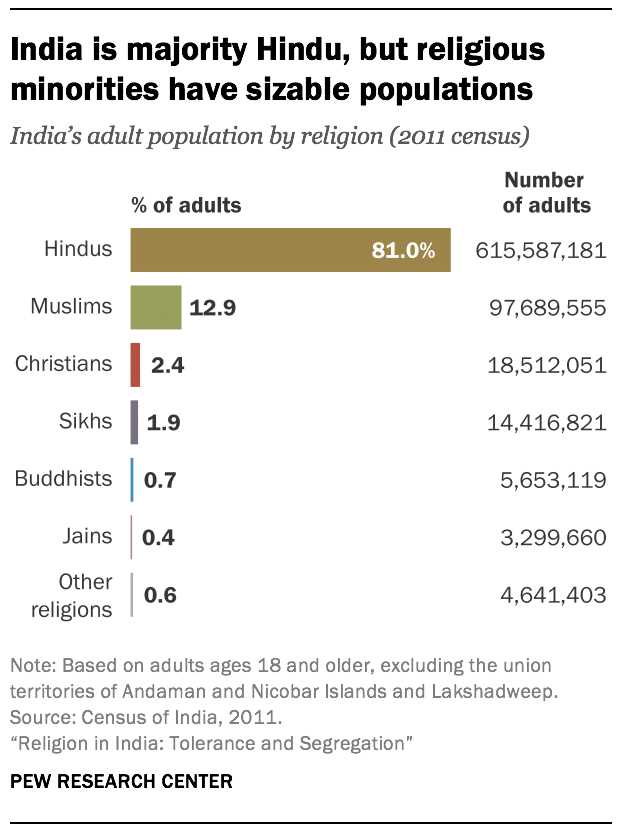
In this section, we dive into a range of mathematical concepts designed to strengthen problem-solving abilities and deepen your understanding of essential topics. With focused exercises, students will learn to apply theoretical knowledge in practical situations, enhancing their overall skill set. This part of the material emphasizes critical thinking, offering valuable insights for tackling complex problems.

Thorough practice and clear explanations are the foundation of this section, helping learners to gain confidence and improve their techniques. By breaking down problems into manageable steps, anyone can achieve mastery and prepare for more advanced topics. Each solution is approached methodically, ensuring a comprehensive grasp of the subject matter.

By the end of this section, students will not only solve mathematical problems with greater ease but also develop a deeper appreciation for the subject. The skills gained here will serve as the basis for future learning and application in various fields of study and real-world scenarios.

Core Connections Course 3 Chapter 4 Answers

This section provides a detailed breakdown of mathematical exercises aimed at enhancing problem-solving techniques and reinforcing key principles. With each task, learners are encouraged to apply their knowledge in practical scenarios, allowing them to grasp abstract concepts more effectively. The focus is on building a deeper understanding of mathematical relationships and the methods used to solve real-world problems.

Clear, step-by-step solutions are provided for each problem, ensuring that students can follow along and understand the reasoning behind each calculation. These solutions are designed to help learners master the techniques required for more complex mathematical challenges, offering a solid foundation for future topics. The goal is to ensure confidence and precision when approaching similar problems in the future.

By completing these exercises, students will improve their ability to analyze and solve problems with accuracy and efficiency. The methods explained here will not only prepare learners for more advanced subjects but also develop critical thinking skills that are essential for academic success.

Overview of Chapter 4 Key Concepts

This section focuses on essential mathematical principles designed to challenge and expand students’ problem-solving abilities. Emphasizing practical application, learners will explore how various techniques come together to tackle complex tasks. The material encourages the development of critical thinking skills while reinforcing important methods that are foundational for more advanced topics.

Exploring Key Mathematical Techniques

Students will learn to manipulate equations and apply specific strategies to find solutions. This section highlights the importance of understanding patterns and relationships within mathematics, providing a deeper understanding of both the theory and practice involved. Practice problems will allow learners to refine their approach and gain a better grasp of the underlying concepts.

Building a Strong Foundation for Future Learning

Each exercise is carefully crafted to reinforce key ideas while challenging students to think beyond the basics. By mastering these techniques, students will be well-prepared to tackle more difficult topics in the future, ensuring a well-rounded education and enhancing overall problem-solving skills. Confidence and proficiency in these methods will lead to academic success and real-world application.

Understanding the Core Connections Curriculum

This curriculum is designed to provide a comprehensive foundation in mathematics by focusing on both fundamental concepts and practical application. It aims to help students develop critical thinking skills while ensuring a deep understanding of mathematical principles. The material is structured to gradually increase in complexity, offering learners the tools they need to solve problems and apply their knowledge in real-world scenarios.

By integrating various problem-solving strategies and techniques, the program fosters a dynamic learning experience. It encourages students to not only grasp theoretical concepts but also to actively engage with exercises that challenge their reasoning and analytical abilities. This approach ensures that learners build confidence while developing a solid mathematical foundation.

Step-by-Step Solutions for Chapter 4

This section offers detailed, methodical solutions to the exercises presented in the material, guiding learners through each problem with clarity. By breaking down the steps in an easy-to-follow manner, students are able to understand the logic behind each solution and apply the same strategies to similar tasks. This approach promotes a deeper comprehension of mathematical processes and enhances problem-solving skills.

Breaking Down Complex Problems

Each solution is divided into smaller, manageable steps to help students grasp the concept behind the problem. This structured method allows learners to focus on individual parts of the task, ensuring they understand how to approach different types of questions. Whether it’s solving equations, analyzing patterns, or applying mathematical properties, this step-by-step guidance builds confidence and expertise.

Developing Problem-Solving Confidence

With clear instructions and explanations, students can improve their ability to solve problems independently. The systematic approach not only helps with immediate exercises but also prepares learners for more advanced material by reinforcing essential techniques and strategies that can be applied to future challenges.

Common Challenges in Chapter 4

As students progress through the material, several common difficulties may arise. These challenges often stem from complex problem structures, misunderstanding key principles, or misapplying learned strategies. Identifying these obstacles early on is essential for improving comprehension and mastering the skills required to tackle more advanced problems.

Below are some of the most frequent issues encountered, along with suggestions for overcoming them:

Challenge	Possible Solution
Difficulty in interpreting word problems	Carefully break down the problem into smaller, simpler components and identify key information first.
Confusion with mathematical operations	Review the basic operations and practice applying them to a variety of problems to build familiarity.
Struggles with recognizing patterns	Work through examples that highlight different types of patterns to improve pattern recognition skills.
Challenges with multi-step problems	Focus on one step at a time, ensuring each part is fully understood before moving on to the next.

By addressing these common challenges with targeted strategies, learners can build the necessary skills to improve their mathematical abilities and approach problems with greater confidence.

How to Approach Word Problems in Math

Word problems often present a unique challenge, as they require translating real-life scenarios into mathematical equations. The key to solving them is breaking down the problem into manageable steps and understanding the underlying concepts. By focusing on the important information and organizing your approach, you can solve these problems more effectively and with greater confidence.

To tackle word problems successfully, follow these steps:

Read the problem carefully: Identify the given information and what the question is asking.
Highlight key terms: Focus on numbers, operations, and words that suggest mathematical relationships.
Define variables: Assign variables to unknowns, and write down what they represent.
Set up an equation: Use the information and variables to form a mathematical model.
Solve the equation: Perform the necessary operations to find the solution.
Verify your solution: Check if the result makes sense in the context of the problem.

By following these steps, students can approach word problems with a systematic method that simplifies the process and improves accuracy. With practice, solving word problems becomes more intuitive, and learners can apply these skills to a wide range of mathematical challenges.

Key Takeaways from Chapter 4 Answers

Understanding the main lessons from this section is crucial for mastering the skills needed to solve mathematical problems efficiently. The solutions provided offer a clear view of the essential strategies and techniques that can be applied to various problems. By focusing on these key takeaways, learners can strengthen their mathematical foundation and improve their problem-solving abilities.

Breaking down problems: Approach complex tasks by dividing them into smaller, manageable steps.
Mastering mathematical operations: Ensure a strong grasp of fundamental operations, as they are critical for solving more advanced problems.
Recognizing patterns: Identifying patterns helps in predicting outcomes and simplifying solutions to problems.
Using variables effectively: Assigning variables and understanding how to manipulate them is key to solving equations accurately.
Checking your work: Always verify your solutions to ensure they align with the problem’s context and make logical sense.

By focusing on these strategies, students can build confidence and consistency in their mathematical abilities. These techniques will not only help with immediate tasks but will also serve as the foundation for more complex topics in future studies.

Strategies for Mastering Key Concepts

Mastering mathematical concepts requires more than just understanding the theory; it involves developing effective strategies to solve problems and apply knowledge in diverse situations. The following strategies can help students gain a deeper understanding and improve their problem-solving skills. By consistently practicing these techniques, learners will enhance their ability to tackle complex problems with confidence.

Practice regularly: Consistent practice helps reinforce understanding and improves problem-solving speed and accuracy.
Understand the underlying principles: Focus on grasping the fundamental concepts rather than memorizing formulas. This will help you approach problems more logically.
Work through examples: Solving sample problems and working through examples step by step will familiarize you with different methods of solving similar tasks.
Ask for help when needed: Don’t hesitate to seek clarification from instructors, peers, or online resources when you encounter difficult concepts.
Review and reflect: After completing a problem, take time to review your approach and solution. Reflecting on mistakes and understanding why certain methods work will improve your skills over time.
Stay organized: Keep your work neat and well-organized to avoid confusion, which can help prevent errors in complex problems.

By incorporating these strategies into daily study routines, students can build a solid mathematical foundation that will be crucial for their success in future challenges and more advanced topics.

Tools to Enhance Problem-Solving Skills

Developing strong problem-solving skills requires the right tools and techniques to approach mathematical tasks effectively. By leveraging a variety of resources, students can improve their analytical thinking and become more efficient in solving complex problems. These tools not only help in breaking down problems but also foster a deeper understanding of mathematical concepts and enhance overall performance.

Graphing Calculators: These tools assist in visualizing equations and functions, making it easier to identify patterns and relationships.
Online Math Platforms: Websites and apps that provide interactive exercises, step-by-step solutions, and tutorials to reinforce learning.
Study Groups: Collaborating with peers allows for the exchange of ideas and strategies, leading to a better grasp of difficult concepts.
Mathematical Software: Programs like GeoGebra and Wolfram Alpha can help in solving complex problems, creating models, and visualizing solutions.
Practice Books: A wide range of books containing practice problems with varying difficulty levels to improve problem-solving techniques.
Mind Mapping Tools: Visual aids that help organize thoughts and break down complex problems into smaller, more manageable steps.

By integrating these tools into daily practice, learners can boost their problem-solving abilities and approach challenges with a more structured and confident mindset. With time, these resources will foster greater independence and success in mathematics.

Detailed Explanation of Math Problems

Understanding the structure of mathematical problems is essential for developing effective solutions. Each problem often presents a unique challenge that requires careful analysis and a step-by-step approach to find the correct answer. By breaking down complex tasks into simpler, more manageable parts, students can learn how to tackle various types of problems with greater confidence and clarity.

In this section, we will explore common mathematical problems, explaining the key concepts involved in solving them and providing detailed steps to guide students through the process. These solutions will cover both basic operations and more advanced techniques, offering clear insights into how to approach and solve different types of questions.

Problem 1: Solving Linear Equations: We will explain how to isolate variables, manipulate equations, and check for consistency in solutions.
Problem 2: Word Problems: Learn how to translate real-life scenarios into mathematical models and use logical reasoning to find the answer.
Problem 3: Algebraic Expressions: Understanding how to simplify, factor, and expand algebraic expressions is crucial for solving many problems.
Problem 4: Geometry Challenges: We will cover topics such as area, volume, and angle relationships, explaining how to apply formulas accurately.
Problem 5: Graphing Functions: Understanding how to plot and interpret mathematical functions on a graph is vital for visualizing solutions.

By working through these problems and understanding the methods behind their solutions, students can develop the skills necessary to tackle a wide range of mathematical challenges. With practice, these concepts will become second nature, enabling learners to approach new problems with a deeper understanding and greater problem-solving ability.

How to Check Your Solutions

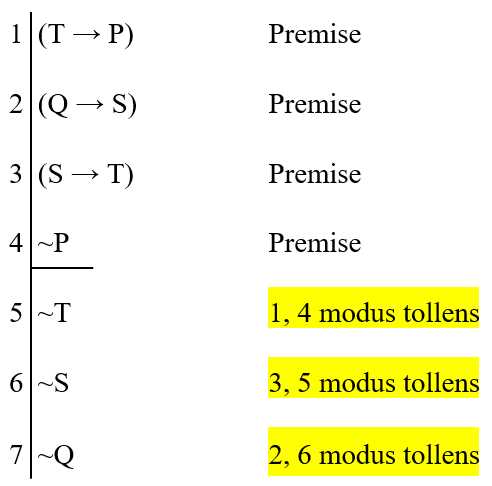
After solving a mathematical problem, it is essential to verify that your solution is correct. This process helps ensure that you haven’t made any mistakes and that your reasoning is sound. Checking your work not only boosts confidence but also sharpens your problem-solving skills. There are several techniques you can use to double-check your solutions effectively.

The first step in reviewing your work is to retrace your steps. Go through the problem again and check each calculation or logical deduction. Pay close attention to whether you followed all necessary steps and used the correct formulas. Additionally, make sure that the units of measurement and values align correctly with the problem’s context.

Revisit Your Equations: Ensure that you have set up the equations properly and solved them step by step without skipping any intermediate steps.
Substitute Values: If possible, plug the solution back into the original equation or problem to verify that it satisfies the given conditions.
Use Estimation: For complex problems, approximate your answer to see if it makes sense in the context of the question. This can help you spot any obvious errors.
Check with a Peer: Discuss your solution with a classmate or teacher. A fresh set of eyes can often identify mistakes you may have missed.
Use Technology: For some problems, tools like graphing calculators or mathematical software can quickly confirm whether your answer is correct.

By carefully reviewing your solutions using these methods, you can minimize errors and improve your problem-solving accuracy. Developing the habit of checking your work will ultimately enhance your learning experience and mathematical understanding.

Tips for Retaining Math Concepts

Mastering mathematical ideas requires more than just solving problems; it also involves retaining the concepts for long-term understanding. The ability to recall and apply mathematical knowledge is critical not only for exams but for real-world problem-solving. To retain mathematical principles effectively, students need to engage with the material in various ways, reinforcing learning through repetition, application, and review.

Here are some key strategies for strengthening your memory of math concepts:

Strategy	Explanation
Active Practice	Consistent practice is crucial. The more you work with problems, the better you’ll internalize the methods and principles behind them.
Use Mnemonics	Creating memory aids or shortcuts for formulas can make recalling key concepts easier when needed.
Teach Someone Else	Explaining concepts to others forces you to clarify your understanding and solidify your grasp of the material.
Apply Real-Life Examples	Link abstract concepts to real-life situations to make them more relatable and memorable.
Review Regularly	Revisit previous lessons periodically to ensure that foundational concepts remain fresh in your mind.
Use Visualization Tools	Graphs, diagrams, and charts can help visualize complex relationships, making them easier to understand and remember.

By incorporating these methods into your study routine, you can enhance your ability to retain and apply mathematical concepts effectively. Consistent reinforcement through a variety of techniques will help ensure that you not only solve problems correctly but also understand the underlying principles for long-term success.

Advanced Techniques for Chapter 4 Problems

As you progress in solving more complex mathematical problems, it’s essential to explore advanced techniques that go beyond basic strategies. These methods involve deeper analysis, more sophisticated problem-solving approaches, and the application of higher-level concepts that lead to faster and more accurate solutions. Mastering these techniques will not only improve your ability to tackle challenging problems but also enhance your overall mathematical understanding.

Below are some advanced strategies to effectively approach and solve difficult problems:

Technique	Application
Working Backwards	Start from the desired outcome and reverse-engineer the steps needed to reach the solution. This method is especially useful when dealing with multi-step problems where the final answer is known.
Pattern Recognition	Look for recurring patterns in numbers or structures. Identifying these patterns can simplify complex problems by reducing them to more manageable components.
Breaking Down Problems	Split larger problems into smaller, more manageable sections. This technique allows you to focus on one part at a time, ensuring you don’t overlook important details.
Use of Graphs and Diagrams	Visual tools like graphs or diagrams can provide insight into relationships and help make abstract concepts more concrete, aiding in the understanding and solving of problems.
Substitution Method	In problems with multiple variables, substitution allows you to replace one variable with its equivalent, simplifying the equation and making it easier to solve.
Dimensional Analysis	This technique is used to check the consistency of equations by analyzing the units involved. It can be particularly helpful in physics-based problems or when working with complex formulas.

By mastering these advanced techniques, you will be able to solve even the most challenging problems with greater efficiency and precision. The key is consistent practice and a willingness to explore new strategies to enhance your problem-solving toolkit.

Common Mistakes to Avoid in Chapter 4

When tackling complex mathematical problems, it’s easy to make errors that can hinder your progress. These mistakes are often subtle but can significantly affect your understanding and results. Recognizing and avoiding these common pitfalls can help ensure that you apply the correct methods and achieve accurate solutions.

Common Pitfalls in Problem-Solving

Misunderstanding the Problem Statement: Always take the time to read the problem carefully. Misinterpreting what is being asked can lead to applying the wrong methods and missing critical steps.
Skipping Intermediate Steps: Rushing through calculations or skipping intermediate steps may lead to mistakes that could be avoided with a bit more attention. Always check your work at each stage.
Overlooking Units and Measurements: When working with real-world problems, especially those involving units like distance, time, or mass, it’s crucial to track and convert units correctly. Ignoring this can cause incorrect answers.
Relying on Memorized Formulas: While formulas are important, relying too heavily on memorization can prevent you from understanding the underlying concepts. It’s better to understand how and when to apply each formula.
Not Checking for Reasonable Results: Always evaluate whether your solution makes sense in the context of the problem. If something seems off, it’s a good idea to review your work to spot errors.

Strategies to Minimize Mistakes

Double-Check Your Work: After solving a problem, go back through each step to ensure everything was done correctly. This extra review can often catch small errors that might otherwise go unnoticed.
Break Problems into Smaller Parts: If you’re feeling overwhelmed, break the problem down into smaller, more manageable sections. This way, you can focus on solving each part before combining them for the final answer.
Ask for Help or Clarification: If you’re unsure about a particular concept or step, don’t hesitate to ask for help. Sometimes discussing the problem with someone else can provide new insights and prevent mistakes.
Practice Regularly: Consistent practice is one of the best ways to avoid mistakes. The more problems you solve, the more familiar you will become with common challenges and how to avoid them.

By recognizing these common mistakes and employing strategies to avoid them, you can improve your problem-solving skills and gain a deeper understanding of the material. Keep practicing, and always approach problems with a careful and methodical mindset.

How Chapter 4 Prepares for Future Topics

The concepts and skills covered in this section play a crucial role in laying the groundwork for more advanced topics in mathematics. Mastering the material not only strengthens foundational knowledge but also helps to develop critical thinking and problem-solving strategies that will be essential in future lessons. This preparation enables students to approach more complex problems with confidence and clarity.

Building a Strong Foundation

In this section, key mathematical principles are introduced, which are often revisited in later topics. Understanding these concepts deeply will help students connect ideas across different areas of study. For example:

Algebraic Foundations: Understanding basic algebraic operations in this section will be vital as more advanced algebraic methods are explored in upcoming topics.
Problem-Solving Techniques: The strategies learned here will be applicable to solving more complex, multi-step problems encountered in future lessons.
Geometric Principles: Concepts related to geometry introduced in this section lay the groundwork for more advanced geometric proofs and applications.

Skills for Success in Future Topics

By mastering the skills in this section, students will develop the confidence needed to tackle future topics in a systematic and organized way. Some of the key skills include:

Critical Thinking: Students learn to analyze problems from different perspectives and think critically about solutions, which is an essential skill for future challenges.
Conceptual Understanding: A strong grasp of the concepts covered here prepares students for more abstract ideas in later lessons, such as complex functions or higher-order equations.
Logical Reasoning: The ability to apply logical steps to arrive at a conclusion is key for future success in mathematics, especially in areas like calculus and statistics.

In conclusion, mastering the material in this section does more than just prepare students for the next few lessons–it builds a solid foundation for future mathematical success. Whether solving algebraic equations, tackling geometry proofs, or analyzing functions, the skills developed here will be crucial in the coming lessons.

Online Resources for Chapter 4 Help

With the rise of digital learning platforms, students now have access to a wealth of online resources that can aid in mastering the material presented in this section. Whether you’re struggling with specific concepts or just want to reinforce your understanding, there are various websites, tools, and videos available that provide valuable explanations, exercises, and interactive content. These resources are designed to enhance your comprehension and help you approach problems more effectively.

Interactive Learning Platforms

Interactive platforms allow students to practice and learn in a hands-on environment. These resources offer guided exercises and real-time feedback, which can help reinforce key concepts.

Khan Academy: This platform offers free instructional videos, practice problems, and quizzes on a variety of topics. It provides a structured approach to learning with instant feedback.
Wolfram Alpha: A powerful tool for solving equations, graphing functions, and breaking down complex problems step by step. It can be useful for checking work and understanding the steps involved in problem-solving.
Desmos: An online graphing calculator that helps students visualize mathematical concepts such as functions, inequalities, and geometry.

Video Tutorials and Forums

If you prefer visual learning, video tutorials and online forums can offer step-by-step explanations of challenging topics. These resources allow you to see problems solved in real time and learn from experts and peers alike.

YouTube: Numerous math educators post detailed lessons on YouTube, breaking down complex problems and explaining key concepts in easy-to-understand formats. Channels like PatrickJMT and Professor Leonard are popular for their clear explanations.
Math Stack Exchange: This forum allows you to ask specific questions and get answers from a community of math enthusiasts and professionals. It’s a great place to find solutions to difficult problems or seek clarification on concepts.
Chegg Study: Chegg offers step-by-step solutions to problems and access to expert tutors who can help explain concepts and assist with homework questions.

By taking advantage of these online resources, students can gain a deeper understanding of the material and reinforce their learning outside of traditional classroom settings. These tools offer a variety of methods to learn, practice, and review, making them invaluable for academic success.

Working Through Exercises

Successfully navigating mathematical exercises requires both practice and strategy. Whether tackling simple problems or more complex challenges, breaking down each task into manageable steps is essential. This approach ensures that each concept is thoroughly understood and applied correctly. In this section, we explore effective methods for approaching exercises that require a deeper understanding of mathematical principles.

One of the key strategies for mastering these problems is to start by carefully reading each question. Understanding what is being asked before proceeding helps avoid mistakes and ensures that the correct solution method is used. Once the question is clear, the next step is to identify relevant formulas, methods, or theorems that can be applied to the problem. This systematic approach fosters a stronger grasp of the underlying concepts and prepares you for more complex challenges ahead.

In addition to following a step-by-step method, it’s important to review your work as you progress. Double-checking calculations, ensuring logical consistency, and verifying the final solution against the problem statement are all vital to success. If errors are detected, reviewing the steps taken will often reveal where things went wrong and how to correct them.

Practicing a variety of problem types and difficulty levels is also essential for building confidence and mastering the material. As you progress through the exercises, you will encounter different problem-solving scenarios, each reinforcing various aspects of the mathematical concepts being taught. With consistent practice, your ability to solve problems accurately and efficiently will improve over time.

Building Confidence with Solutions

Developing confidence in problem-solving comes from consistent practice and a clear understanding of the underlying principles. As you work through various problems, it’s crucial to build a solid foundation by reinforcing each concept step by step. This method not only improves accuracy but also fosters a sense of accomplishment as you progress through increasingly complex tasks.

One effective approach is to break down solutions into smaller, more digestible parts. This helps reduce feelings of overwhelm and allows for a more thorough understanding of each component of the problem. By focusing on individual steps and verifying your reasoning at each stage, you gradually build confidence in your ability to tackle more difficult questions without hesitation.

Reviewing Completed Solutions

After completing each problem, take time to carefully review your solution. Examine each step to ensure that the logic is sound and that the correct methods were applied. Verifying your approach and results helps solidify your understanding and highlights areas that may require additional attention. This process not only boosts confidence but also enhances problem-solving skills for future challenges.

Consistent Practice and Reflection

Another way to build confidence is through regular practice. The more you engage with different types of problems, the more familiar you become with common patterns and strategies. Additionally, reflecting on your progress helps you recognize how much you’ve learned and encourages a positive mindset. With persistence, you’ll develop the confidence to approach even the most challenging problems with assurance and clarity.