Geometry Review Packet Answers for Better Exam Prep

Mathematics is filled with complex concepts that often require a structured approach to fully grasp. Whether you’re preparing for an upcoming test or reviewing important material, having clear, reliable methods for tackling problems is essential. This section focuses on helping you navigate through various challenges, ensuring a deeper understanding of the core ideas that drive mathematical thinking.

Effective problem-solving is crucial for mastering this subject. By breaking down each question into manageable parts, you can uncover the underlying patterns and principles. Embracing a systematic approach not only makes tasks more approachable but also boosts confidence when dealing with unfamiliar problems.

In this guide, you’ll find strategies, tips, and clear solutions to help you conquer common issues. Whether you’re working with shapes, measurements, or algebraic expressions, the key is to stay consistent and practice regularly. By understanding the fundamental techniques, you’ll be able to tackle any mathematical challenge with ease.

Geometry Review Packet Answers

Mastering key concepts in mathematics requires more than just memorizing formulas. It’s about understanding the connections between different principles and applying them effectively to solve problems. In this section, we’ll explore various techniques and methods that will guide you in tackling mathematical exercises with confidence and precision.

One of the most important strategies for success is breaking down complex problems into simpler steps. Here are some helpful guidelines:

Identify the main components of the problem, such as shapes, measurements, and variables.
Focus on the relationships between these elements, such as angles, sides, and their properties.
Use known formulas or theorems to find missing values or make necessary calculations.
Draw clear diagrams to visualize the problem and ensure a more accurate solution.

In addition, reviewing common issues that arise in these tasks can help prevent mistakes:

Incorrectly applying formulas to unfamiliar situations.
Misinterpreting diagrams or missing key information.
Skipping important steps or assumptions that are necessary for the correct outcome.

By carefully following these techniques and understanding the patterns that emerge in problems, you’ll be able to approach tasks with greater ease and accuracy, ultimately enhancing your overall performance in mathematical challenges.

Key Concepts to Focus On

Understanding the foundational principles behind mathematical problems is essential for success. The core ideas form the building blocks for solving more complex tasks and should be prioritized in your study sessions. Recognizing these crucial elements helps in navigating different types of challenges with confidence and accuracy.

Some of the most important concepts to focus on include:

Shapes and their properties: Study the various properties of different figures, including symmetry, angles, and side lengths. Understanding these can simplify many problems.
Measurement and dimensions: Focus on how to calculate area, perimeter, and volume for different forms. Knowing the formulas for these calculations is vital.
Angle relationships: Grasp the connections between various types of angles, such as complementary, supplementary, and vertical angles.
Transformations: Understand how figures can be translated, rotated, reflected, or dilated, and how these operations affect their properties.
Proportions and ratios: Learn how to solve problems involving proportional relationships, scale factors, and similar figures.

By concentrating on these critical elements, you’ll build a strong foundation that can be applied across different types of mathematical exercises. Mastering these concepts allows for a deeper understanding and a more efficient approach to solving problems.

Common Mistakes in Geometry Reviews

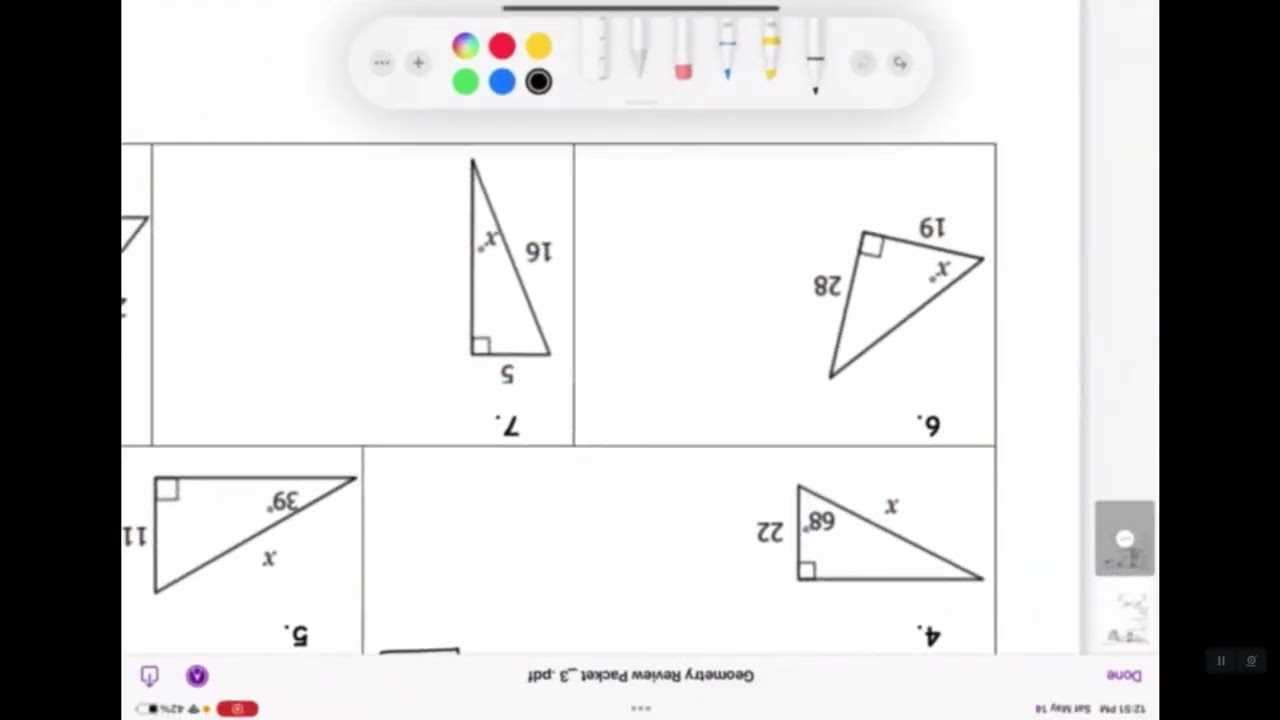
When solving mathematical problems, even small errors can lead to incorrect results and confusion. It’s crucial to be aware of frequent pitfalls so that you can avoid them and ensure your approach remains accurate. By identifying common mistakes, you can refine your problem-solving methods and improve overall performance.

Misunderstanding Formulas and Theorems

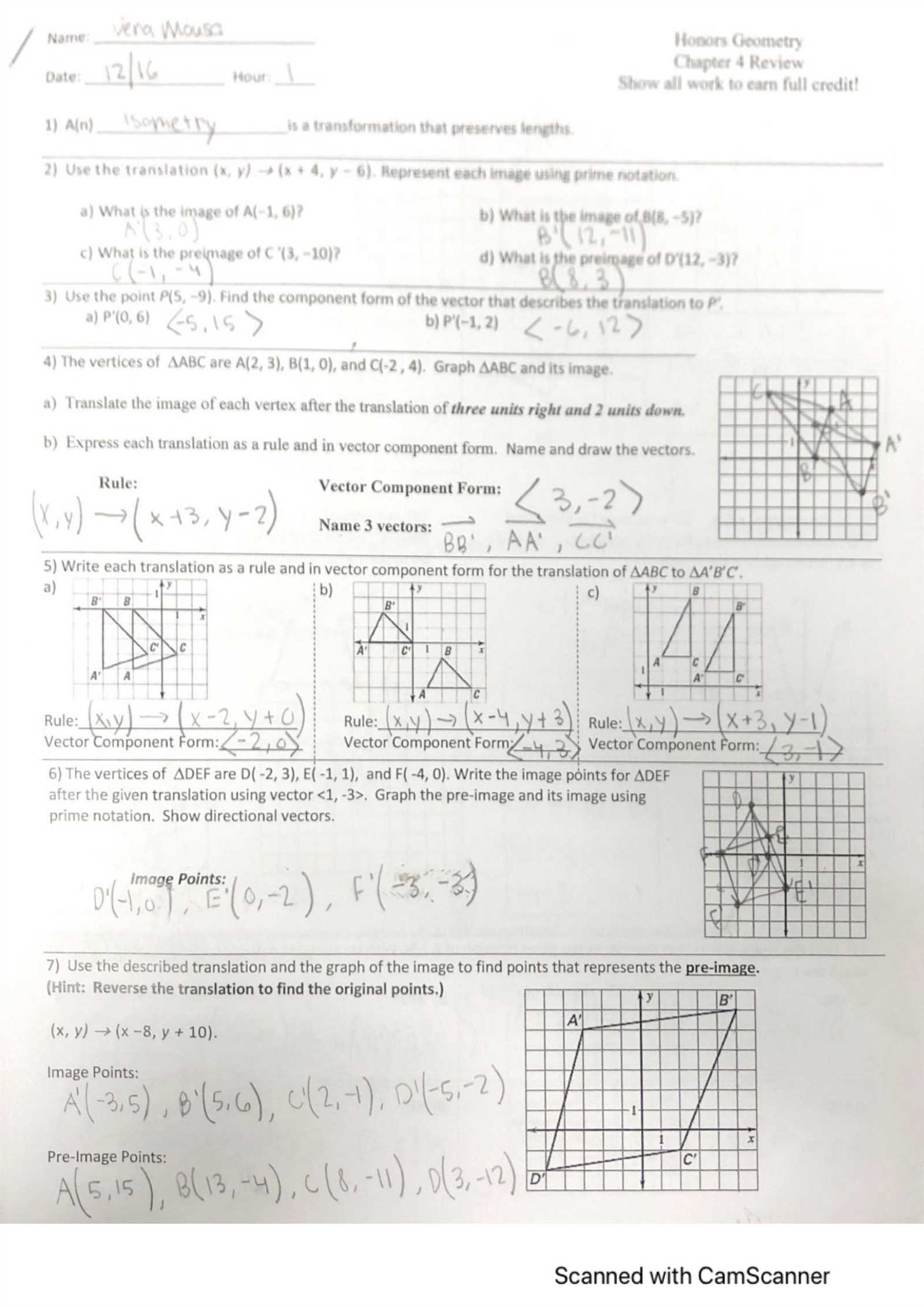
One of the most frequent errors occurs when formulas or theorems are applied incorrectly. A common mistake is using the wrong equation for a given problem or misinterpreting the relationships between different elements. It’s essential to carefully examine the context of each problem and choose the appropriate rule or formula to use. Double-checking the specific requirements of the question can help avoid this mistake.

Overlooking Key Information in Diagrams

Another common issue is failing to notice important details in diagrams or figures. These illustrations often contain essential clues about angles, side lengths, or symmetries that are crucial for solving the problem. Skipping over these visual hints can result in missed steps and inaccurate solutions. Always take time to analyze diagrams carefully and ensure you have captured all relevant information before proceeding with calculations.

By being aware of these common mistakes, you can adopt a more careful and methodical approach to problem-solving. This will help increase both the accuracy and efficiency of your work.

Step-by-Step Solutions for Geometry Problems

When faced with a complex problem, it’s important to break it down into smaller, more manageable steps. A structured approach ensures that each part of the problem is addressed systematically, reducing the risk of errors and improving accuracy. This method also helps build a deeper understanding of the underlying concepts.

Identify the Key Elements

The first step is to carefully read through the problem and identify all the relevant information. Look for known values such as side lengths, angles, or other measurable quantities. Then, recognize what is being asked for, whether it’s an area, a side length, or the measure of an angle. Highlighting these elements will provide clarity and direction as you move forward with the solution process.

Apply the Correct Formula or Theorem

Once the key elements are identified, the next step is to choose the appropriate formula or theorem to apply. This step requires a solid understanding of various relationships between shapes and figures. Whether you’re calculating the area of a triangle or determining the value of an unknown side, make sure to carefully apply the correct formula. Ensure that all units are consistent, and if needed, convert measurements before solving.

By following these steps, you can confidently approach each problem, ensuring a structured and logical path toward the solution. Consistent practice will also help reinforce your understanding and improve your problem-solving efficiency.

Understanding Geometry Theorems Clearly

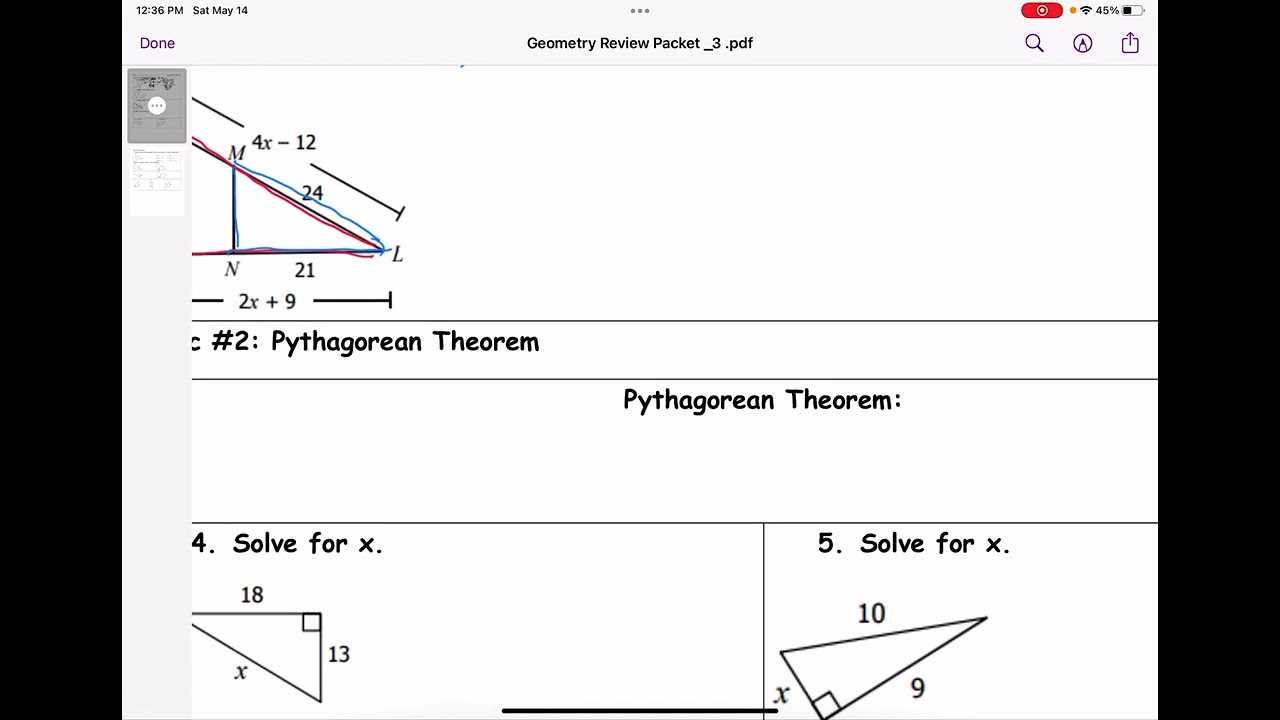
In mathematics, theorems are statements that have been proven based on previously established principles. They provide a logical foundation for solving problems and understanding relationships between different shapes and figures. To apply theorems effectively, it’s essential to first grasp their meanings and the reasoning behind them.

One key to mastering theorems is to focus on their core elements: assumptions, conditions, and conclusions. By recognizing how these aspects are connected, you can better understand the application of each theorem in various scenarios. The following table outlines some important theorems and their components:

Theorem	Key Concepts	Example of Application
Pythagorean Theorem	Right triangle, relationship between sides	Finding the length of the hypotenuse
Triangle Sum Theorem	Sum of interior angles, triangle properties	Calculating missing angles in a triangle
Angle Sum Theorem	Sum of interior angles in polygons	Finding the angle measures of a polygon

By carefully studying these concepts and their relationships, you’ll be able to approach problems with a clear understanding of how theorems can guide your solutions. With consistent practice, applying these theorems will become second nature, allowing you to solve complex problems more effectively.

Tips for Tackling Geometry Word Problems

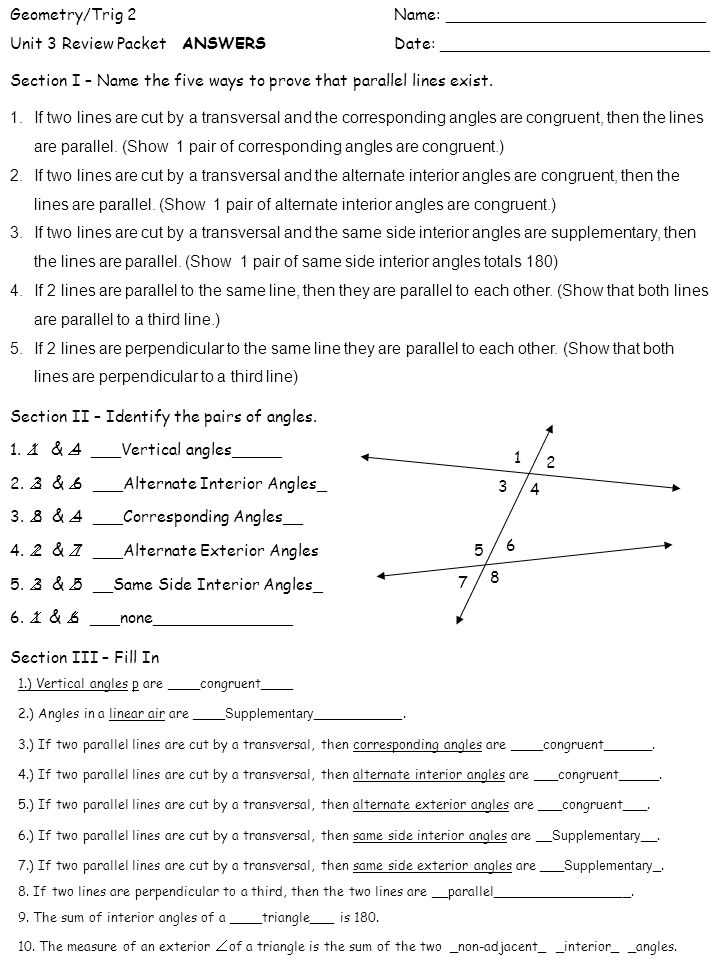
Word problems can often seem intimidating due to the detailed descriptions and multiple steps required to find the solution. However, breaking them down into smaller, manageable pieces can help clarify the process and make the task easier. The key is to focus on identifying the important details and translating them into mathematical operations.

Here are some effective strategies to approach these types of problems:

Read the problem carefully: Take your time to understand the entire scenario. Pay attention to key terms and conditions that indicate mathematical relationships.
Highlight important information: Mark relevant data such as measurements, relationships, and what is being asked. This helps in visualizing the problem more clearly.
Draw a diagram or figure: Visualizing the situation can make it easier to see relationships between different elements. It’s often helpful to sketch out shapes, angles, and distances to guide your solution.
Break down the problem into smaller steps: Simplify the task by dividing the problem into smaller, sequential steps. Solve each part one at a time to avoid feeling overwhelmed.
Check for hidden relationships: Look for indirect relationships or patterns that may not be immediately obvious. These can lead to more efficient ways of solving the problem.
Revisit the question: After working through the problem, re-read the question to ensure that your solution is directly answering what was asked.

By following these steps, you’ll find that word problems become more manageable, and you’ll gain confidence in applying mathematical reasoning to real-world scenarios.

How to Solve Geometry Equations Effectively

Solving mathematical equations requires a systematic approach that ensures both accuracy and efficiency. The key to success lies in understanding the relationships between variables and applying the right techniques at each stage. A methodical approach helps you break down the problem and avoid common pitfalls.

Understand the Variables and Relationships

The first step in solving equations is identifying all the variables involved. Carefully examine the problem to determine what each symbol represents and how the different elements are connected. Recognizing key relationships between elements, such as the Pythagorean theorem for right triangles or proportionality in similar figures, can help you choose the correct equation to apply.

Apply the Right Mathematical Operations

Once you have identified the appropriate relationships, it’s time to apply the necessary mathematical operations. Ensure you use consistent units and double-check that the formula matches the type of problem you’re solving. When performing calculations, maintain clarity by solving for one variable at a time, simplifying the equation step by step. Take your time to isolate variables before solving, and don’t rush through complex steps.

By following these strategies, you’ll improve your ability to solve mathematical problems quickly and accurately. With regular practice, the process will become more intuitive, allowing you to tackle a wider range of challenges with confidence.

Essential Geometry Formulas You Need

In order to solve a wide variety of mathematical problems, it is essential to have a solid grasp of key equations. These formulas help simplify complex tasks and provide the necessary tools for calculating areas, volumes, and other measurements. Knowing the right formulas to apply can greatly speed up problem-solving and improve accuracy.

Here are some of the most important formulas to keep in mind:

Area of a Circle: A = πr² – This formula calculates the area of a circle, where “r” represents the radius.
Perimeter of a Rectangle: P = 2l + 2w – To find the perimeter, simply add up the lengths of all sides, where “l” is length and “w” is width.
Volume of a Cylinder: V = πr²h – This equation calculates the volume of a cylinder, with “r” representing the radius and “h” the height.
Area of a Triangle: A = 1/2bh – To find the area, multiply the base (b) by the height (h) and divide by 2.
Pythagorean Theorem: a² + b² = c² – This fundamental theorem is used to find the lengths of sides in right-angled triangles.
Surface Area of a Sphere: A = 4πr² – This formula helps calculate the surface area of a sphere, with “r” being the radius.
Volume of a Rectangular Prism: V = l × w × h – The volume of a box-like shape can be found by multiplying the length, width, and height.

Familiarizing yourself with these essential formulas will provide a strong foundation for tackling a wide range of mathematical problems. With practice, these equations will become second nature, enabling quicker and more efficient solutions.

Strategies for Geometry Test Preparation

Preparing for a mathematical exam requires a well-planned approach to ensure understanding and success. The key is to review essential concepts, practice problem-solving techniques, and develop a strategy to approach questions effectively during the test. A structured study plan can help reduce anxiety and increase confidence when facing challenging problems.

Here are some practical strategies to enhance your preparation:

Review Key Theorems and Formulas: Focus on the foundational principles that govern shapes, angles, and measurements. Make sure you understand how to apply these equations in various scenarios.
Practice with Sample Problems: Work through problems from past exams or sample worksheets. This helps reinforce your problem-solving skills and familiarizes you with the types of questions you may encounter.
Focus on Weak Areas: Identify areas where you struggle and dedicate extra time to them. Whether it’s understanding certain concepts or solving specific types of problems, targeted practice will improve your ability to handle those topics.
Utilize Study Groups: Collaborate with classmates or friends. Discussing problems and solutions can lead to a deeper understanding and may provide different perspectives on how to approach a question.
Take Timed Practice Tests: Simulate exam conditions by taking practice tests within a set time limit. This helps improve time management skills and ensures you’re comfortable working under pressure.
Stay Organized: Keep all your notes, formulas, and practice problems in an organized manner. Create a cheat sheet of important formulas to quickly reference during your study sessions.

By following these strategies, you’ll develop a deeper understanding of the material and improve your problem-solving efficiency. Consistent practice, focused review, and effective time management are essential for achieving success on the test.

Common Geometry Questions in Reviews

When preparing for a mathematical exam or tackling practice problems, certain types of questions frequently appear. These questions typically focus on understanding relationships between different shapes, calculating specific measurements, and applying key concepts. Recognizing these recurring themes allows you to prioritize your study and practice more efficiently.

Here are some of the most common types of problems that you may encounter:

Area and Perimeter Calculations: Questions often ask you to find the area or perimeter of various shapes, such as rectangles, circles, triangles, and polygons. Make sure you’re familiar with the respective formulas and how to apply them correctly.
Volume and Surface Area of Solids: Another common set of questions involves calculating the volume and surface area of three-dimensional objects like cubes, spheres, and cylinders. Understanding how to break down these calculations into simpler steps is crucial.
Angle Relationships: Many problems test your knowledge of angle properties, such as complementary, supplementary, and vertical angles. These often appear in the context of parallel lines, triangles, and polygons.
Pythagorean Theorem: Problems related to right triangles are often based on this fundamental theorem. Being able to identify and apply it effectively is essential for solving distance and length-related questions.
Congruency and Similarity: Questions may involve determining whether two shapes are congruent or similar, and if so, applying the properties of these relationships to solve for unknown measurements.
Coordinate Geometry: These problems require you to find the distance between two points, the midpoint of a line segment, or the slope of a line on a coordinate plane. Mastering the basic formulas is key to solving these problems quickly.

By familiarizing yourself with these common problem types, you’ll be better prepared to handle a variety of scenarios during your study sessions or on the exam day. Regular practice with these questions ensures that you’re ready for anything that comes your way.

Using Diagrams to Solve Geometry Problems

Visual aids, such as diagrams, play a crucial role in understanding and solving mathematical problems. They help to represent abstract concepts in a tangible way, making it easier to identify relationships between various elements. By carefully analyzing and labeling these visuals, you can simplify complex problems and gain a clearer understanding of the required solutions.

Here are a few tips for effectively using diagrams to solve problems:

Label All Known Values: Mark the given measurements, such as lengths, angles, and coordinates, directly on the diagram. This will allow you to refer to them easily as you work through the problem.
Identify Key Relationships: Look for patterns and connections between different parts of the diagram. For instance, check for parallel lines, congruent angles, or symmetry, as these often provide helpful insights for solving the problem.
Break Complex Diagrams into Simpler Parts: If a diagram is complicated, break it down into smaller, more manageable sections. Solve each part individually and then combine your results to find the solution to the overall problem.
Use Different Types of Diagrams: Depending on the problem, you might need to work with various types of visual aids, such as coordinate grids, bar graphs, or 3D models. Experiment with different representations to find the most effective one.
Double-Check Your Diagram: Ensure that all information is correctly depicted. Even small errors in drawing can lead to incorrect solutions, so it’s essential to verify the accuracy of your diagram before proceeding.

By incorporating diagrams into your problem-solving process, you can visualize key concepts, making it easier to manipulate and analyze the given data. Whether you’re working with basic shapes or more complex figures, diagrams are an invaluable tool for achieving accurate solutions.

How to Approach Geometry Proofs

Solving logical problems often requires step-by-step reasoning and a solid understanding of fundamental principles. When tasked with demonstrating a relationship or property, it’s important to build a clear, logical argument that connects known facts to unknown conclusions. This process involves breaking down complex ideas into manageable steps and systematically proving each point based on previously established facts.

Here are some effective strategies for tackling proofs:

1. Understand the Problem

Before you begin writing, carefully read the statement and identify what needs to be proven. Clarify the given information and what is being asked. This will help you identify which theorems or properties you can use.

2. Plan Your Steps

Write Down Given Information: Start by noting down all the known facts and figures. Make sure these are clearly visible for reference throughout the proof.
Identify What to Prove: Clearly state what needs to be proven, as this helps guide your reasoning and ensures you’re focused on the right objective.
Look for Relationships: Recognize any key relationships or patterns, such as parallel lines, right angles, or congruent shapes, that can help support your proof.

3. Build the Proof Step-by-Step

Proofs often follow a logical sequence where each statement is supported by a reason. Each step should logically follow from the previous one, whether it’s an established property, a rule, or a previously proven theorem. Below are some useful guidelines:

Use Given Facts: Begin with the information provided in the problem statement. This forms the foundation of your reasoning.
Apply Theorems and Definitions: Use known properties or theorems that relate to the problem. Theorems like the Pythagorean Theorem or properties of parallel lines may often be helpful.
Make Logical Deductions: Each step should make logical sense and move you closer to your goal. Use previously proven facts to support your claims.
Conclude with What Was Asked: Ensure that your final step directly answers the question posed in the problem. The conclusion should be clear and based on the steps you’ve demonstrated.

By following these structured steps, you’ll build a strong logical argument that proves the desired relationship or property. With practice, solving proofs becomes a more intuitive and effective process, allowing you to confidently approach complex problems.

Breaking Down Complex Geometry Problems

When faced with a challenging problem, it’s easy to feel overwhelmed by the number of variables and unknowns. The key to solving these types of problems lies in breaking them down into simpler, more manageable parts. By isolating individual elements and addressing them one step at a time, you can gradually reconstruct the entire solution, making the task less daunting.

The process of deconstructing a difficult problem often involves the following steps:

Identify Key Information: Carefully read through the problem and highlight all given data. This includes numerical values, relationships, and any relevant figures. Knowing exactly what you have to work with is the first step in simplifying the problem.
Draw a Diagram: Visual representation can make it easier to understand the relationships between different elements. A well-labeled diagram can reveal patterns and connections that might not be immediately apparent in the text.
Break the Problem into Parts: Instead of trying to solve the entire problem at once, break it down into smaller, more straightforward tasks. Tackle one part at a time and piece the solutions together.
Look for Familiar Patterns: Many complex problems are built upon well-known principles and theorems. Look for any connections to previously learned concepts that might help simplify the solution process.
Work Backwards if Necessary: Sometimes, starting from the desired solution and working backward can offer new insights. This technique can be particularly useful when the final outcome is more apparent than the steps needed to get there.

By using these techniques, you can reduce complexity and make seemingly difficult problems easier to solve. The key is to stay organized, stay patient, and approach each part of the problem systematically.

Geometry Review Packet Practice Tips

Practicing effectively is a crucial part of mastering any subject. The goal is not just to go through problems, but to understand the process behind solving them. To make the most out of your practice sessions, it’s important to adopt strategies that enhance both comprehension and retention. Here are several useful tips that can help you improve your skills and approach challenges with confidence.

Start with the Basics: Before tackling advanced problems, ensure you have a solid understanding of foundational concepts. Mastering basic principles allows you to approach more complex problems with ease.
Practice Regularly: Consistent practice is key to improving. Set aside time each day or week to work through problems, and aim to gradually increase the difficulty level as you improve.
Understand the Why: Don’t just memorize formulas or steps–focus on understanding why certain methods work. This deeper understanding will help you solve problems more effectively and apply your knowledge in new situations.
Work Through Mistakes: When you make an error, don’t just skip over it. Take the time to identify what went wrong, understand the mistake, and correct it. This is a valuable learning opportunity that reinforces your knowledge.
Use Multiple Resources: Don’t rely on just one source of practice. Use different materials, such as online quizzes, textbooks, or interactive problem solvers, to expose yourself to a variety of problem types.
Time Yourself: If you’re preparing for a timed exam, simulate test conditions by timing yourself. This helps you get accustomed to managing your time during problem-solving and improves your efficiency.

By incorporating these strategies into your practice routine, you can build the skills and confidence needed to tackle challenges more effectively. Remember, the goal is not just to solve problems, but to truly understand the concepts and techniques that will help you excel.

Applying Knowledge in Real Life

The concepts learned in mathematics can extend far beyond the classroom, influencing various aspects of daily life. From planning projects to understanding the world around us, the principles of spatial relationships and measurement are essential tools. Understanding how to apply these skills in practical situations not only enhances problem-solving abilities but also demonstrates the real-world relevance of what might initially seem like abstract theories.

Home Design and Construction: When designing a house or renovating a space, the ability to calculate areas, volumes, and angles is invaluable. Whether you’re planning a kitchen layout, determining the slope of a roof, or creating custom furniture, these skills ensure precise measurements and proper planning.

Engineering and Architecture: In fields such as civil engineering or architecture, an in-depth understanding of shape, size, and space is crucial. Professionals rely on geometric principles to design safe structures, from bridges to skyscrapers, taking into account load distribution, angles, and materials.

Navigation and Travel: When traveling or navigating, distance, speed, and direction are often calculated using concepts of space. Whether you’re plotting a course on a map or determining the most efficient route, knowledge of shapes, angles, and measurements helps guide decisions and saves time.

Art and Design: Artists frequently use geometric principles in their work to create proportionate, aesthetically pleasing compositions. Whether working with shapes, symmetry, or perspective, geometry plays a fundamental role in visual arts, from painting to sculpture to digital design.

Sports and Athletics: Many sports, such as basketball, soccer, and tennis, require an understanding of angles and trajectories. Whether calculating the best angle for shooting a basketball or positioning oneself to intercept a ball, applying geometric concepts enhances performance and strategy.

By recognizing the connection between theoretical knowledge and practical applications, individuals can gain a deeper appreciation for the subject and its usefulness in everyday tasks. The ability to apply these skills confidently translates into better decision-making and more effective problem-solving in many areas of life.

Why This Subject Can Be Challenging

Many individuals find this area of study to be particularly difficult due to its combination of abstract concepts and practical applications. The subject often requires a shift in thinking, demanding both spatial awareness and logical reasoning. While some may grasp basic ideas quickly, others struggle with the complexity of visualizing shapes, understanding relationships between angles, and solving intricate problems.

Abstract Thinking and Visualization

A key challenge is the need to visualize problems in a two- or three-dimensional space. For some, imagining objects from different perspectives or drawing accurate diagrams can be difficult. This requires not only comprehension but also the ability to mentally rotate, manipulate, and visualize geometric shapes, which can be especially challenging without hands-on experience.

Complex Problem-Solving Techniques

Another reason this field can be difficult is the complexity of the problems themselves. The process often involves multiple steps, including identifying the correct formulas, applying them appropriately, and interpreting the results. Mistakes in any of these steps can lead to incorrect answers, which can be frustrating for students trying to find their way through complicated problems.

Additionally, the need for precision and attention to detail can make it challenging for those who are used to more straightforward or formulaic subjects. Success in this field requires a balance of mathematical skill, critical thinking, and creativity–skills that take time and practice to develop.

How This Subject Affects Other Areas of Study

This area of study plays a crucial role in shaping various academic disciplines by providing a foundation for both abstract reasoning and practical problem-solving. Its principles extend beyond the classroom, influencing fields such as physics, engineering, computer science, and even art. Understanding these concepts not only enhances one’s analytical abilities but also improves one’s approach to solving real-world challenges.

In Science and Engineering, knowledge of shapes, dimensions, and measurements is essential for understanding the physical world. Whether calculating forces, building structures, or designing machinery, the ability to apply geometric concepts ensures accuracy and efficiency. In physics, for instance, the understanding of angles, trajectories, and spatial relationships is fundamental in studying motion and forces.

In Technology and Computer Science, many algorithms and software applications rely on these principles. From creating digital graphics to developing artificial intelligence systems, the ability to manipulate shapes, dimensions, and spaces is critical. In fact, fields like computer-aided design (CAD) or 3D modeling are directly influenced by the ability to visualize and manipulate objects in virtual spaces.

In Art and Design, these concepts are just as important. Artists and designers use them to create balance, perspective, and structure in their work. Whether it’s creating architectural blueprints or painting a portrait, the knowledge of how shapes and lines interact helps artists bring their creative visions to life.

Ultimately, mastering this field empowers individuals with the skills to approach problems systematically, think critically, and find innovative solutions in a variety of other subjects. It serves as a bridge between the theoretical and the practical, making it a cornerstone of many intellectual pursuits.