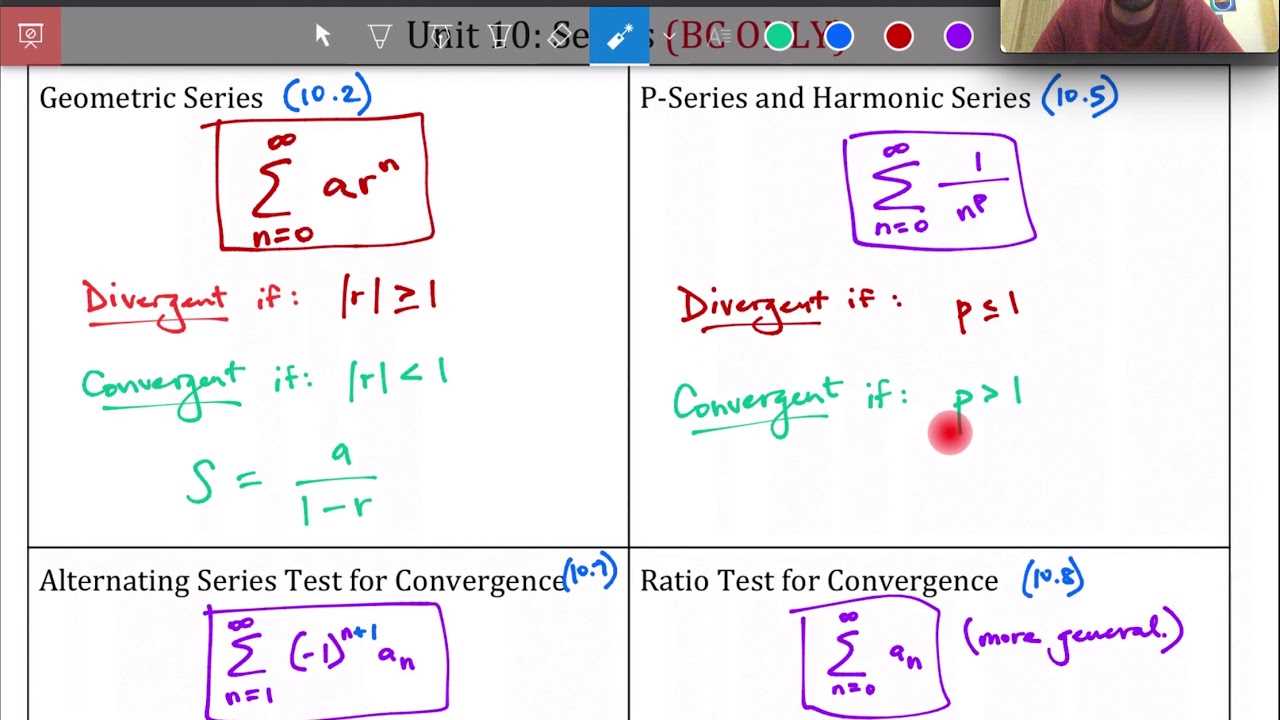
Preparing for the AP Calculus AB test can seem like a daunting task, but with the right approach, you can approach it with confidence. Success in this assessment requires a solid understanding of mathematical concepts and the ability to apply them in different scenarios. Whether you are a beginner or looking to refine your skills, proper preparation is essential to perform well.
In this section, we will explore effective strategies for mastering the necessary material. You will discover how to prioritize important topics, organize your time efficiently, and use available resources to enhance your learning. Additionally, you will learn techniques for tackling both multiple-choice and open-ended questions with ease, ensuring that you are ready for any challenge that comes your way.
AP Calculus AB Exam Study Guide
Mastering the content of this crucial assessment involves more than just memorizing formulas and theorems. It requires developing a deep understanding of the underlying principles and the ability to apply them to solve complex problems. By approaching your preparation with a structured plan, you can build the necessary skills to tackle various question types and achieve success.
Key Topics to Focus On

To effectively prepare, focus on the core areas that will appear on the test. Pay attention to fundamental concepts such as derivatives, integrals, and limits. Understanding these key areas will provide a solid foundation for solving problems and applying mathematical techniques in different contexts. Review these topics thoroughly and practice applying them in various scenarios to solidify your grasp on the material.
Effective Preparation Strategies
Developing a study plan that includes consistent practice and review is crucial for success. Use available resources, such as practice questions, sample problems, and online tutorials, to reinforce your knowledge. Break your study sessions into manageable blocks, focusing on specific areas in each one. Additionally, consider setting aside time for mock tests, which will allow you to simulate the real experience and build confidence under timed conditions.
Understanding the AP Calculus AB Exam
To perform well on this important assessment, it is crucial to understand its structure and the types of questions you will face. Knowing what to expect will help you prepare effectively and manage your time wisely. The test evaluates both your theoretical knowledge and practical application of mathematical concepts. Familiarity with its format will give you a strategic advantage on test day.
The assessment consists of two main sections: multiple-choice and free-response. Each section has its own challenges, so it’s important to approach them with different strategies.
- Multiple-Choice Section: This part of the test assesses your ability to quickly apply formulas and concepts to various scenarios. You’ll need to solve problems efficiently and accurately.
- Free-Response Section: In this section, you will be asked to show your work in detail, providing a clear step-by-step explanation of your solutions. This tests both your problem-solving skills and your ability to communicate your reasoning.
Understanding the structure and purpose of each section will allow you to focus your preparation on the right areas. Make sure to practice with both question types to ensure that you’re fully prepared for all parts of the assessment.
Key Topics to Focus On
To excel in this assessment, it is essential to focus on the most critical concepts that will be tested. Understanding the fundamental principles in these areas will provide a strong foundation for solving complex problems and performing well under timed conditions. Prioritize the topics that are central to the test, as mastering these will give you the best chance of success.
The main areas to concentrate on include:
- Derivatives: Grasping the concept of rates of change and how to apply differentiation techniques to a variety of functions is vital. Understand both the rules of differentiation and how to interpret the results.
- Integrals: Mastering techniques of integration, including area under curves and accumulation functions, is crucial for solving many problems. Practice both definite and indefinite integrals.
- Limits: Be able to evaluate limits of functions, particularly in cases of indeterminate forms or asymptotic behavior. This forms the basis for many higher-level concepts.
- Applications of Differentiation: Understanding how derivatives are used to solve real-world problems, such as optimization and motion, is key for the free-response section.
- Rate of Change and Motion: Work on problems that involve the relationship between velocity, acceleration, and position over time. These concepts are often tested in both the multiple-choice and open-ended sections.
By concentrating on these core topics, you will ensure that you are prepared for the most commonly tested material and are able to solve a wide range of problems with confidence.
How to Organize Your Study Plan
Creating a structured approach to preparing for this important assessment is essential for success. A well-organized plan will help you focus on key areas, manage your time effectively, and track your progress. Without a clear roadmap, it’s easy to become overwhelmed by the amount of material. Breaking down your preparation into manageable tasks will keep you on track and ensure you cover all the necessary topics.
Steps to Build Your Plan
- Set Clear Goals: Define what you need to accomplish each week. Set specific targets for mastering individual topics and solving a certain number of problems.
- Prioritize Key Topics: Focus on the most important concepts first, especially those that are frequently tested. This will help you build a strong foundation.
- Use a Calendar: Allocate study time in a calendar or planner. Ensure you balance practice sessions with breaks to avoid burnout.
- Include Practice Tests: Make room for practice questions and mock tests throughout your plan. These will help you assess your readiness and identify weak areas.
Time Management Tips
- Break Sessions Into Blocks: Work in focused intervals, such as 45-60 minutes, followed by short breaks. This method increases productivity and concentration.
- Review Regularly: Plan periodic reviews of material you’ve already covered. Spaced repetition will help reinforce what you’ve learned.
- Stay Flexible: While it’s important to stick to your plan, remain adaptable. If you find a topic particularly challenging, allocate extra time to it.
By following a structured plan, you’ll be able to systematically cover all topics, keep your progress on track, and increase your chances of performing well.
Time Management Tips for AP Calculus
Effective time management is crucial for success in any challenging assessment. By organizing your schedule and setting clear priorities, you can maximize your preparation without feeling overwhelmed. Proper planning allows you to balance learning new concepts with reinforcing the material you’ve already covered. This section offers practical strategies to help you manage your time efficiently and stay on track.
Key Strategies for Time Management
- Create a Study Calendar: Plan your sessions in advance by marking specific dates for each topic or skill you want to focus on. A calendar helps keep you organized and ensures you don’t miss any crucial areas.
- Set Time Limits: Allocate specific time blocks for each task. Limiting the time spent on each area helps maintain focus and prevents burnout. Try working in 45-minute intervals with 10-15 minute breaks in between.
- Break Tasks Into Smaller Chunks: Divide larger concepts into smaller, manageable tasks. Focusing on one sub-topic at a time allows for more effective learning and prevents feeling overwhelmed.
- Prioritize Difficult Topics: Tackle the most challenging concepts first, when you have the most energy. This ensures that you give yourself ample time to master difficult areas.
How to Stay on Track
- Monitor Progress: Regularly check your progress against your plan. Make adjustments if necessary to stay on track and ensure you’re meeting your study goals.
- Avoid Procrastination: Stick to your schedule and avoid putting off tasks. Starting early and staying consistent will help you avoid last-minute stress.
- Incorporate Review Time: Schedule time for periodic reviews to reinforce your knowledge. Spaced repetition is an effective technique for retaining information over the long term.
With careful planning and consistent effort, you can make the most of your study time and enter the assessment with confidence. Effective time management allows you to prepare thoroughly while reducing stress and increasing your chances of success.
Effective Ways to Learn Derivatives
Understanding how to calculate rates of change is essential for solving many problems. Derivatives play a central role in analyzing functions, from finding slopes to determining critical points. To master this concept, it’s important to focus on both the theory and practical application, practicing regularly to solidify your skills. This section provides strategies to help you learn derivatives more effectively.
One of the most important steps in learning derivatives is mastering the basic rules. Begin by familiarizing yourself with the most common differentiation formulas, such as the power rule, product rule, quotient rule, and chain rule. Once you have these formulas down, you can begin applying them to more complex functions.
- Start with Simple Functions: Begin by practicing derivatives of basic functions, such as polynomials, trigonometric functions, and exponentials. This will help you build confidence and develop a solid foundation before moving on to more complex examples.
- Understand the Geometric Interpretation: Try to visualize the derivative as the slope of a tangent line to a curve. This can help you understand the concept on a deeper level and recognize its significance in various applications.
- Practice Regularly: Consistent practice is key to mastering derivatives. Work through a wide variety of problems, ranging from simple to complex, to develop problem-solving skills and reinforce your understanding.
- Use Real-World Examples: Connect derivatives to real-life scenarios, such as velocity, acceleration, and optimization problems. This practical application will help make abstract concepts more relatable and easier to grasp.
By focusing on these strategies and practicing regularly, you will be able to confidently tackle problems involving derivatives and develop a deeper understanding of their role in mathematics.
Mastering Integrals for the Exam
To excel in solving problems that involve accumulation and area, it’s crucial to understand the fundamental techniques of integration. Whether it’s finding areas under curves or solving real-world problems, mastering this concept is essential for success. This section covers the key methods and strategies you need to fully grasp integration, so you can approach related questions with confidence.
Integration can be intimidating at first, but breaking it down into manageable steps and practicing consistently will help you develop a deeper understanding. Start by learning the core integration techniques and gradually move to more complex methods as you gain experience.
Key Techniques for Mastering Integrals
- Basic Integration Rules: Begin with the fundamental integration formulas, such as the power rule, constant rule, and sum rule. Understanding these rules will allow you to tackle simple problems efficiently.
- Substitution Method: Master the art of substitution, a powerful technique used to simplify complex integrals. Recognizing when and how to apply substitution is crucial for solving more advanced problems.
- Integration by Parts: This method is essential for integrating products of functions. Practice applying the integration by parts formula, especially for trigonometric and logarithmic functions.
- Partial Fraction Decomposition: For rational functions, learning to break them down into simpler fractions can make integration much easier. This technique is especially useful for functions that cannot be integrated directly.
Applying Integrals to Real-World Problems
- Area Under Curves: One of the most common applications of integration is finding the area between a function and the x-axis. Practice setting up and solving these problems to reinforce your understanding of definite integrals.
- Accumulation Problems: Understand how integrals can be used to model real-world accumulation, such as distance traveled over time or the total amount of a substance added to a system.
- Volume and Surface Area: Learn how to use integrals to compute volumes of solids of revolution and the surface area of 3D objects. These problems often appear in more advanced levels of study.
By mastering these integration techniques and understanding how they apply to various problems, you will be better prepared to solve integration-related questions efficiently and accurately. Consistent practice and familiarity with these methods are key to performing well when faced with integration challenges.
Common Mistakes to Avoid in Calculus
While working through mathematical problems, especially those involving rates of change and areas, it’s easy to make simple mistakes that can lead to incorrect results. Recognizing and avoiding these errors is essential for performing well. This section highlights some of the most frequent pitfalls and offers advice on how to avoid them, ensuring that your understanding remains solid and your solutions accurate.
Frequent Errors in Problem Solving
- Misapplying Rules: A common mistake is incorrectly applying differentiation or integration rules. For example, forgetting to apply the chain rule or incorrectly using the power rule for non-integer exponents can result in errors.
- Sign Errors: Pay close attention to positive and negative signs. These small mistakes often occur during the simplification process and can drastically change the result.
- Overlooking Domain Restrictions: Many problems involve functions with restrictions on their domains. Ignoring these can lead to unrealistic or undefined solutions, particularly when working with logarithmic or rational functions.
- Incorrect Substitution: In integration, substitution is a common technique, but selecting the wrong variable or forgetting to adjust the bounds of integration can lead to errors.
Common Conceptual Mistakes
- Confusing Concepts: Mixing up related concepts such as instantaneous rate of change and average rate of change can lead to incorrect interpretations of problems. Always ensure you’re clear on the definitions before solving.
- Forgetting Units: When solving real-world problems, it’s important to keep track of units throughout the entire process. Omitting or misinterpreting units can lead to nonsensical results.
- Not Checking Results: After solving a problem, it’s always a good idea to double-check your answer. Verifying through alternative methods or checking against known values can help spot errors you may have missed.
By recognizing these common mistakes and being mindful during problem-solving, you can improve your accuracy and reduce the chances of errors. Attention to detail and regular practice are key to mastering these concepts and avoiding pitfalls.
Using Practice Tests for Better Preparation

One of the most effective ways to enhance your understanding and readiness for any challenging assessment is by using practice tests. These simulated scenarios provide an opportunity to apply what you’ve learned, identify gaps in your knowledge, and refine your problem-solving techniques. By regularly practicing with test-like conditions, you can familiarize yourself with the structure and timing of the actual tasks ahead.
Incorporating practice tests into your preparation routine can also help you build confidence and reduce test anxiety. This section outlines how to maximize the benefits of practice tests and integrate them effectively into your study plan.
Benefits of Practice Tests
- Improved Time Management: Practice tests simulate real conditions, helping you get accustomed to the time limits. This allows you to refine your pacing and ensure you can complete tasks within the given time frame.
- Identifying Weak Areas: By working through a variety of practice problems, you can pinpoint areas where you might need additional review. This targeted approach ensures that your efforts are focused on the most important concepts.
- Enhanced Test-Taking Strategy: Regularly completing practice tests teaches you how to approach different types of questions strategically. You’ll learn to recognize patterns, use shortcuts, and prioritize tasks effectively.
How to Use Practice Tests Effectively

| Step | Action | Goal |
|---|---|---|
| 1 | Take Full-Length Practice Tests | Simulate actual conditions and get a sense of the timing and structure of tasks. |
| 2 | Review Mistakes | Analyze incorrect answers to understand your reasoning errors and learn from them. |
| 3 | Track Progress | Monitor your performance over time to see improvements and areas still needing attention. |
| 4 | Replicate Test Environment | Practice in a quiet, distraction-free space to mimic test conditions as closely as possible. |
By following these steps and integrating practice tests into your preparation, you’ll be better equipped to handle the challenges you face. Regular testing, combined with careful review, builds both skill and confidence, ensuring that you’re fully prepared for the tasks ahead.
Resources for AP Calculus AB Students
There are a variety of tools available to help students master complex mathematical concepts and prepare for assessments. These resources can assist in reinforcing key topics, offer practice opportunities, and provide clarity on difficult subjects. Whether you are looking for interactive tools, textbooks, or online platforms, utilizing a combination of different resources can enhance your understanding and boost your performance.
This section explores some of the most valuable resources for students aiming to strengthen their skills and knowledge in preparation for any challenge. From online courses to practice questions, these materials can help guide your learning process effectively.
Recommended Online Platforms
- Khan Academy: Offers a wide range of free video lessons and exercises on mathematical topics. The interactive exercises are particularly useful for self-assessment and reinforcing learning.
- Wolfram Alpha: An advanced computational tool that can solve mathematical problems step by step. Great for checking solutions and understanding the process behind problem-solving.
- PatrickJMT: A YouTube channel with clear, concise video tutorials on a wide variety of mathematical concepts, ideal for visual learners.
- AP Classroom: A platform provided by the College Board with practice problems, videos, and other helpful materials tailored for advanced learners.
Books and Text Resources

- AP Calculus AB and BC Prep Books: Comprehensive books with practice problems, detailed solutions, and in-depth explanations. Examples include “Barron’s AP Calculus” and “The Princeton Review’s Cracking the AP Calculus.”
- Textbook Resources: Many textbooks come with supplemental materials, including online quizzes, practice tests, and guided exercises. These can provide a more structured approach to learning.
- Practice Problem Sets: Using books or online platforms that offer large collections of problems helps build fluency in solving various problem types.
Study Groups and Tutoring Services

- Peer Study Groups: Collaborating with classmates or joining study groups can provide diverse perspectives on complex topics. Explaining concepts to others reinforces your own understanding.
- Online Tutoring Services: Websites like Chegg and Khan Academy offer tutoring services that provide one-on-one support, which can be extremely helpful for understanding difficult concepts.
By utilizing these various resources, you can create a well-rounded approach to mastering mathematical concepts. Combining video tutorials, practice problems, and personal assistance will give you the tools you need to succeed in any mathematical challenge.
How to Tackle Multiple-Choice Questions
Multiple-choice questions can often feel daunting, but with the right strategies, they can become an opportunity to demonstrate your knowledge efficiently. Understanding how to approach these questions is key to improving your accuracy and confidence. By following a methodical process, you can increase your chances of selecting the correct answer, even when you’re uncertain.
This section offers practical tips on how to effectively navigate through multiple-choice questions, ensuring that you make the best decisions under timed conditions.
Key Strategies for Multiple-Choice Success
- Read the Question Carefully: Before looking at the answer choices, make sure you fully understand the question. This helps you focus on what’s being asked and avoid getting distracted by irrelevant information.
- Eliminate Incorrect Answers: Often, multiple-choice questions have one or two choices that are clearly wrong. Cross them off to narrow down your options and increase your chances of choosing correctly.
- Look for Keywords: Pay attention to specific words like “always,” “never,” “all,” or “none,” as they can significantly impact the correct answer. These words can indicate whether the answer is likely true or false.
- Make Educated Guesses: If you’re unsure, eliminate obviously incorrect answers and make an educated guess from the remaining choices. Even if you’re not certain, narrowing it down helps you make the best possible decision.
Time Management During Multiple-Choice Questions
- Don’t Spend Too Much Time on One Question: If a question is taking too long, move on and return to it later. Spending too much time on a difficult question can waste valuable minutes.
- Mark and Skip: If you’re unsure of an answer, mark the question and come back to it after finishing the easier ones. This ensures that you don’t miss out on points you can earn easily.
- Keep Track of Time: Set a mental or physical timer to pace yourself. Aim to spend roughly the same amount of time on each question to ensure you can finish the entire set.
By using these techniques, you can approach multiple-choice questions with confidence, systematically narrowing down your options and increasing your chances of selecting the right answers.
Strategies for Free-Response Questions
Free-response questions require a different approach compared to multiple-choice. They often test your ability to think critically and explain your reasoning step by step. These questions give you the opportunity to show a deeper understanding of the material and demonstrate your problem-solving process. Mastering these types of questions is essential for maximizing your score, as they typically make up a significant portion of the assessment.
In this section, we’ll cover effective strategies that will help you tackle free-response questions with confidence, ensuring that you present your answers clearly and systematically.
Step-by-Step Problem Solving
- Understand the Question: Before jumping into the solution, read the question carefully. Break it down into smaller parts to identify exactly what is being asked. Sometimes, the question will include specific instructions like “justify your answer” or “show your work,” so it’s important to follow these guidelines.
- Organize Your Work: Start with a clean, organized approach. Write down the relevant formulas, theorems, or concepts that might help solve the problem. This will help keep you on track and avoid confusion.
- Show Every Step: Even if you’re confident in your answer, always show every step of your work. This not only helps ensure you don’t miss anything but also provides partial credit if the final answer is incorrect.
- Check for Units and Accuracy: For problems involving physical quantities, always include appropriate units in your answer. Double-check your math for accuracy, especially when solving complex problems.
Managing Time Effectively
- Start with Easier Problems: If you’re unsure about a question, don’t waste too much time on it. Start with the ones you know well to build momentum and gain confidence.
- Write Neatly and Clearly: The clarity of your handwriting and the organization of your work are critical. Neatness ensures that your solutions are understandable and that the grader can follow your thought process easily.
- Leave Time for Review: Always leave some time at the end to review your answers. Check for any mistakes or missed steps. A quick review can help catch errors that you might have overlooked in the heat of the moment.
By following these strategies, you can approach free-response questions with confidence and efficiency. A methodical approach will not only help you solve problems accurately but will also demonstrate your understanding and problem-solving skills to the grader.
Understanding the Grading Scale

Knowing how your performance will be assessed is crucial when preparing for any high-stakes evaluation. The grading scale used for these types of assessments can be complex, as it often involves multiple sections with different weightings. Understanding the breakdown of how your answers are scored can help you strategize your approach to maximize your score, allowing you to focus on areas that carry more weight or are particularly challenging for you.
In this section, we’ll explore how the grading scale is structured, what each section contributes to your overall score, and how you can interpret your results to identify areas for improvement.
Overview of the Grading Structure
- Multiple-Choice Section: Typically, this section is scored based on the number of correct answers. There’s often no penalty for incorrect answers, so it’s advantageous to guess if you’re unsure.
- Free-Response Section: This section is scored based on the completeness and accuracy of your work. Each part of the question is graded individually, and you are awarded points for demonstrating the correct process, even if your final answer is incorrect.
- Weighting of Sections: Different sections carry varying weights in determining your overall score. It’s essential to know how much each part contributes so that you can allocate your time and energy effectively during preparation.
Interpreting Your Score
- Raw Score: This is the initial score calculated from your responses. It represents your total number of correct answers in both the multiple-choice and free-response sections.
- Scaled Score: Your raw score is then converted to a scaled score, which adjusts for differences in difficulty between different test versions. This is the score that ultimately determines your performance and may range from 1 to 5.
- Score Bands: A score of 3 or higher typically signifies proficiency, while scores of 4 or 5 are considered excellent. Understanding these bands can help you set a target score and gauge your readiness.
By familiarizing yourself with the grading scale, you can better understand how your performance will be evaluated and focus your preparation accordingly. Properly interpreting your score can also provide valuable insights into your strengths and areas that may need further attention before the test.
How to Stay Motivated During Study
Maintaining focus and drive while preparing for a challenging assessment can be one of the most difficult aspects of the process. The key to long-term success lies in staying motivated and avoiding burnout, especially when the material seems overwhelming or the goals feel far off. By developing a positive mindset and using effective strategies, you can push through moments of doubt and keep moving forward.
This section explores practical tips for staying energized and focused during your preparation. By incorporating these approaches, you’ll be able to maintain a consistent work ethic and achieve your goals without losing momentum.
Setting Clear Goals
- Break Large Tasks into Smaller Steps: Instead of focusing on the entire scope of the material, break it into smaller, manageable segments. This will make the workload seem less daunting and help you track your progress more easily.
- Set Daily or Weekly Milestones: Establish short-term goals that are achievable. Meeting these milestones will give you a sense of accomplishment and keep you on track.
- Visualize Success: Think about how great it will feel when you reach your goals. Keeping the end result in mind can serve as a powerful motivator to keep going, especially when motivation is low.
Creating a Productive Environment
- Limit Distractions: Find a quiet, comfortable place to work and minimize distractions like social media, TV, or loud environments. A focused space will allow you to concentrate more effectively.
- Incorporate Variety: Alternate between different types of tasks to avoid monotony. Switch from reading to practice questions or from working on problems to reviewing notes to keep things interesting.
- Reward Yourself: Give yourself small rewards after completing tasks. Whether it’s a short break, a snack, or a favorite activity, these incentives can help you stay motivated and make studying feel less like a chore.
By implementing these strategies, you can maintain your focus, stay motivated, and feel more in control of your preparation. The journey may be long, but consistent effort and a positive mindset will make all the difference in achieving your goals.
Key Formulas to Memorize
Mastering essential mathematical equations is a fundamental step in preparing for any high-level assessment. While understanding the underlying concepts is important, having key formulas at your fingertips is crucial for solving problems quickly and efficiently. In this section, we focus on the most important formulas that you should commit to memory, ensuring you can apply them with ease during the assessment.
These formulas form the backbone of many problems and will frequently appear in various forms. Being familiar with them will help you save valuable time and improve accuracy when tackling complex questions.
Essential Derivatives and Integrals

| Formula | Description |
|---|---|
| Power Rule: ( frac{d}{dx} x^n = n cdot x^{n-1} ) | For differentiating functions of the form ( x^n ). |
| Product Rule: ( frac{d}{dx} [u(x) cdot v(x)] = u'(x) cdot v(x) + u(x) cdot v'(x) ) | Used for the derivative of the product of two functions. |
| Quotient Rule: ( frac{d}{dx} left[ frac{u(x)}{v(x)} right] = frac{v(x) cdot u'(x) – u(x) cdot v'(x)}{v(x)^2} ) | For differentiating the ratio of two functions. |
| Fundamental Theorem of Calculus: ( int_a^b f(x) dx = F(b) – F(a) ) | Relates the derivative and the integral, providing a way to compute definite integrals. |
Trigonometric and Logarithmic Functions
| Formula | Description |
|---|---|
| Derivative of Sine: ( frac{d}{dx} sin(x) = cos(x) ) | The derivative of sine is cosine. |
| Derivative of Cosine: ( frac{d}{dx} cos(x) = -sin(x) ) | The derivative of cosine is the negative sine. |
| Integral of ( frac{1}{x} ): ( int frac{1}{x} dx = ln|x| + C ) | The integral of ( 1/x ) is the natural logarithm of ( x ). |
| Product of Exponentials: ( e^{a+b} = e^a cdot e^b ) | The property of exponents for multiplying exponential functions. |
These formulas will provide the necessary tools for tackling a wide range of problems efficiently. Memorizing them and understanding their application is essential for success in any advanced mathematics challenge.
Managing Exam Stress Effectively
Preparation for a challenging test can often lead to heightened stress, which may negatively impact your performance. Understanding how to manage pressure and maintain a calm, focused mindset is crucial for optimal results. This section will explore several strategies to help you keep stress levels in check and maximize your effectiveness during the preparation and assessment phases.
By incorporating stress-relieving techniques, you can maintain mental clarity and enhance your ability to retain and apply information under pressure. Here are a few effective strategies that can help you cope with the tension commonly associated with high-stakes assessments.
Practical Stress Management Techniques

| Technique | Benefit |
|---|---|
| Mindful Breathing | Calms the nervous system, helping to reduce anxiety and increase focus. |
| Physical Exercise | Boosts endorphins, which reduce stress and improve overall mood. |
| Time Management | Prevents last-minute cramming by spreading out work over a longer period, reducing pressure. |
| Positive Visualization | Helps to build confidence by imagining successful outcomes and reducing negative thoughts. |
| Adequate Rest | Ensures the brain functions at its best, increasing memory retention and problem-solving ability. |
Maintaining Focus and Balance
Balancing study sessions with relaxation is key to staying sharp and reducing anxiety. A well-structured routine that includes breaks, exercise, and proper rest will help you sustain energy levels and focus, allowing you to approach each challenge with a clear, calm mind.
By consistently applying these strategies, you will be better equipped to handle pressure and perform at your best, ultimately reducing the impact of stress on your performance.
How to Review Calculus Concepts Quickly
When faced with a tight timeline, it’s essential to focus on the most important concepts to maximize your efficiency. Instead of trying to cover every single detail, identifying key areas and applying effective strategies can help you review quickly and effectively. This section will highlight methods for reinforcing your understanding and retaining the most crucial information in a limited amount of time.
By using focused techniques, you can streamline your revision and reinforce your comprehension, ensuring that you’re well-prepared for the upcoming assessment. Here’s a breakdown of some useful strategies to help you review efficiently.
Effective Strategies for Quick Review
| Strategy | Purpose |
|---|---|
| Focus on Core Principles | Concentrate on essential rules and formulas that form the foundation of the subject. |
| Utilize Flashcards | Quickly test your recall of key concepts and reinforce memory retention. |
| Practice with Sample Problems | Apply concepts to solve problems and identify areas where you need improvement. |
| Review Mistakes | Analyze incorrect answers to understand where you went wrong and strengthen weak points. |
| Use Visual Aids | Diagrams, graphs, and charts can simplify complex ideas and improve understanding. |
Organizing Your Revision Session
To make the most of your review, start by organizing a plan that allocates time to the most important topics. Prioritize areas where you feel less confident, but don’t neglect reviewing material you’re comfortable with, as this helps reinforce your overall understanding.
By following these approaches, you can make rapid progress in reinforcing the core ideas and improve your readiness in a short amount of time.
Final Tips Before the AP Exam
As the time to face the test approaches, it’s important to enter the assessment with a clear mindset and effective strategies. The days leading up to the event should be focused on reinforcing confidence, reviewing key areas, and managing stress. A few crucial actions can make a significant difference in how well you perform when the time comes.
This section provides practical advice for the final moments before the test, helping you stay calm, prepared, and mentally sharp. Here are the top strategies to implement as the big day arrives.
Stay Calm and Confident
It’s easy to become overwhelmed as the event nears, but maintaining a calm and focused mindset is crucial. Here are some ways to keep your confidence high:
- Review what you’ve learned rather than cramming new information.
- Trust in your preparation and progress so far.
- Take breaks when needed to avoid burnout, especially in the final hours before the test.
- Get a good night’s sleep to ensure you’re rested and alert on test day.
Prepare Logistically

Aside from mental preparation, ensuring that all logistical details are sorted will allow you to enter the room with minimal distractions:
- Double-check the test location, time, and any required materials.
- Prepare everything you’ll need in advance, such as pens, pencils, an approved calculator, and an ID.
- Plan to arrive early to avoid any last-minute stress.
By focusing on these final tips, you’ll be able to walk into the test feeling composed and ready to perform at your best. Remember, success comes from preparation, confidence, and the ability to manage stress effectively.