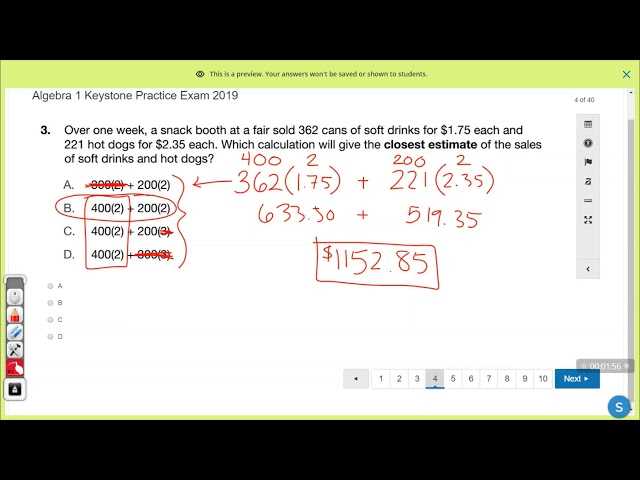
Preparing for standardized assessments requires a clear understanding of key mathematical principles and the ability to apply them effectively. Whether you’re revisiting foundational topics or strengthening your knowledge, a structured approach can help ensure success. With the right techniques and resources, you’ll be equipped to tackle even the most challenging questions with confidence.
Focused review on core problem-solving strategies, critical thinking, and efficient time management is essential. By honing these skills, you can improve both accuracy and speed, making it easier to navigate through complex problems. Developing a strong grasp of the material is not just about memorizing formulas, but also about understanding how to apply them in various contexts.
Consistent practice and careful review of previous assessments can provide valuable insight into what to expect and where to focus your efforts. By identifying common patterns and problem types, you’ll be better prepared to approach each question with a strategic mindset, ultimately increasing your chances of success.
Algebra 1 Exam Preparation Tips

Preparing for any assessment that tests mathematical reasoning requires a thoughtful approach. Developing a solid understanding of core concepts and refining your problem-solving skills can significantly enhance your performance. To improve your confidence and efficiency, it’s essential to focus on key areas that often appear in these evaluations. The following strategies will help guide you through the preparation process and ensure you’re ready for any challenge that comes your way.
Focus on Core Problem-Solving Techniques
To succeed, concentrate on mastering the fundamental problem-solving methods that are frequently tested. These include simplifying expressions, solving for unknown variables, and recognizing patterns. Understanding the logic behind each solution is crucial, as it allows you to approach similar problems more effectively. Regularly revisiting these core techniques will make it easier to tackle unfamiliar questions when the time comes.
Maximize Your Practice Time
Allocating sufficient time to review previous assessments is a powerful tool for success. Identifying recurring question types will help you predict what you might encounter and guide your study sessions. The more time you dedicate to solving various problems under timed conditions, the better equipped you’ll be to handle pressure during the actual test. Practice also helps improve your speed and accuracy, two crucial components of success.
Mastering Key Concepts for Algebra 1
Grasping essential mathematical principles is the foundation for performing well on any standardized assessment. The ability to understand and apply key concepts is what sets strong students apart. It’s not just about memorizing rules but also about developing a deeper understanding of how these concepts connect and function within different types of problems. By focusing on the core principles, you can approach even the most complex tasks with confidence and clarity.
Start by reinforcing your knowledge of basic operations, such as solving linear equations, working with inequalities, and understanding functions. These are the building blocks that will support more advanced topics and make them easier to master. Consistent review and active application of these concepts will ensure they become second nature, allowing you to solve problems efficiently and accurately under pressure.
Effective Study Strategies for the Exam
Adopting the right study techniques is essential for maximizing your performance in any assessment. A well-structured approach not only helps you absorb the material but also builds confidence and improves retention. To truly succeed, it’s important to tailor your study routine to your learning style and focus on areas where you need the most improvement. With the right strategies, you can approach the test with a sense of preparedness and calm.
Start by breaking down the material into manageable sections and set clear goals for each study session. Use a mix of review methods such as flashcards, practice problems, and summarizing key concepts to reinforce your understanding. Regular short study intervals, instead of cramming, can boost memory retention. Additionally, reviewing your mistakes and understanding why you went wrong is just as important as solving problems correctly.
Common Mistakes to Avoid in Algebra 1
When working through mathematical problems, it’s easy to make small errors that can lead to big mistakes in your final results. These mistakes often arise from misunderstandings or oversights of key concepts. Identifying and avoiding common pitfalls is crucial to improving your accuracy and efficiency. By being aware of these frequent errors, you can focus on correcting them before they affect your performance.
Common Calculation Errors
Many errors stem from basic calculation mistakes that can easily be avoided with more careful attention. Some of the most common include:
- Misplacing decimal points or missing them entirely
- Forgetting to apply the order of operations correctly
- Overlooking negative signs or incorrectly handling them
- Misinterpreting fractions or failing to simplify them
Conceptual Misunderstandings
Other mistakes occur when there is a fundamental misunderstanding of key principles. These errors may not be immediately obvious, but they can lead to incorrect answers if not addressed:
- Confusing linear and quadratic relationships
- Failing to understand the properties of functions
- Not recognizing equivalent expressions or equations
- Overlooking constraints or conditions in word problems
Understanding Algebra 1 Question Formats
Being familiar with the structure and types of questions you’ll encounter during an assessment is key to performing well. Different question formats test your ability to apply concepts in various ways, and understanding how these questions are presented can help you approach them more confidently. By recognizing the format of a problem, you can quickly identify the best strategy for solving it.
Types of Question Formats
Questions often vary in how they are phrased, but they generally fall into a few common categories. Recognizing these types can help you prepare for what to expect:
- Multiple Choice: These questions provide several possible answers, and you must choose the correct one. Practice identifying key clues in the problem to eliminate incorrect options.
- Open-Ended: These require you to solve a problem and provide a detailed solution. Pay attention to every step and ensure you show your work clearly.
- Word Problems: These questions present a real-world scenario that you must interpret mathematically. Make sure to identify the given information and decide which mathematical operation to apply.
- Fill-in-the-Blank: Here, you must supply the correct value or expression to complete an equation or inequality. Accuracy and precision are important.
Tips for Handling Different Question Formats
Different question formats require different approaches. Here are some strategies for handling each type:
- For multiple-choice questions, read all the options carefully before selecting the answer.
- For open-ended questions, break down the problem into smaller steps and ensure each part is completed correctly.
- When facing word problems, underline key terms and translate the situation into a mathematical equation.
- In fill-in-the-blank questions, double-check your work and ensure the value fits the equation or inequality.
How to Tackle Word Problems in Algebra

Word problems can be some of the most challenging questions in any math-based assessment. They require you to translate a real-world scenario into a mathematical equation, which involves careful reading and critical thinking. The key to solving word problems is breaking them down into manageable steps and understanding how to extract the relevant information from the given text.
Steps for Solving Word Problems
Follow these steps to solve word problems effectively:
- Read the Problem Carefully: Ensure you understand all the details provided in the problem. Look for keywords that indicate mathematical operations, such as “sum,” “difference,” or “product.”
- Identify What You Need to Find: Determine what the problem is asking you to solve for. It could be a number, an equation, or a specific relationship between variables.
- Translate Words into Equations: Once you’ve identified the key information, write an equation or inequality based on the problem’s details.
- Solve Step-by-Step: Carefully solve the equation, showing your work. Don’t rush, as each step is essential to getting the correct answer.
- Check Your Work: Once you’ve solved the problem, revisit the question to ensure that your answer makes sense within the context of the scenario.
Example Word Problem Breakdown
Let’s look at a sample word problem and break it down:
| Problem | A train travels 60 miles per hour for 3 hours. How far will the train travel? |
|---|---|
| Step 1: Identify Information | Speed = 60 miles per hour, Time = 3 hours |
| Step 2: Write the Equation | Distance = Speed × Time |
| Step 3: Solve | Distance = 60 × 3 = 180 miles |
| Step 4: Check the Answer | The answer is reasonable, as 60 miles per hour for 3 hours should result in 180 miles. |
Essential Formulas for Algebra 1 Exam
Understanding and memorizing key mathematical formulas is essential for success in any assessment. These formulas serve as the foundation for solving problems efficiently, whether you’re dealing with equations, functions, or inequalities. Having a solid grasp of these fundamental expressions allows you to quickly recognize patterns and apply the right approach to a wide variety of problems.
Below are some of the most important formulas you should be familiar with:
Basic Arithmetic and Operations
- Distributive Property: a(b + c) = ab + ac
- Order of Operations: Parentheses, Exponents, Multiplication/Division, Addition/Subtraction (PEMDAS)
- Negative Numbers: (-a)(-b) = ab and (-a)(b) = -ab
Linear Equations and Functions
- Slope-Intercept Form: y = mx + b, where m is the slope and b is the y-intercept.
- Point-Slope Form: y – y₁ = m(x – x₁), used when a point (x₁, y₁) and the slope m are known.
- Standard Form of a Linear Equation: Ax + By = C, where A, B, and C are constants.
Quadratic Equations
- Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a, used to solve equations in the form ax² + bx + c = 0
- Factoring: Factoring expressions of the form ax² + bx + c into two binomials.
Systems of Equations
- Substitution Method: Solve one equation for one variable and substitute it into the other equation.
- Elimination Method: Add or subtract the equations to eliminate one variable and solve for the other.
These formulas are essential tools that will help you efficiently solve problems. Make sure to practice applying them in different scenarios to enhance your understanding and speed when tackling problems in an assessment.
Breaking Down Algebra 1 Exam Sections
Understanding the structure of any assessment is key to effective preparation. By recognizing how the different sections are organized, you can tailor your study efforts to focus on areas that require the most attention. This breakdown will help you allocate your time wisely and approach each section with a clear strategy.
Typically, tests are divided into several sections that each evaluate different skills. These sections may include a variety of problem types, such as equations, inequalities, word problems, and graph interpretations. Let’s take a closer look at how each section might be structured:
Section 1: Solving Equations and Inequalities
This section usually tests your ability to solve linear equations and inequalities, both with one variable and multiple variables. You may encounter simple equations, as well as more complex ones that require multiple steps. The key here is to remember the order of operations and the methods for isolating variables.
- Linear Equations: Solve for unknown variables.
- Inequalities: Solve and graph solutions, paying attention to direction signs.
Section 2: Graphing and Functions
In this section, you’ll be asked to work with graphs and functions. You may be required to graph linear functions, interpret graphs, or find the slope and y-intercept of a given line. The ability to understand the relationship between algebraic expressions and their graphical representations is essential here.
- Graphing Linear Functions: Identify the slope and y-intercept to plot points on a graph.
- Functions: Work with function notation and solve for function values.
Section 3: Word Problems and Applications
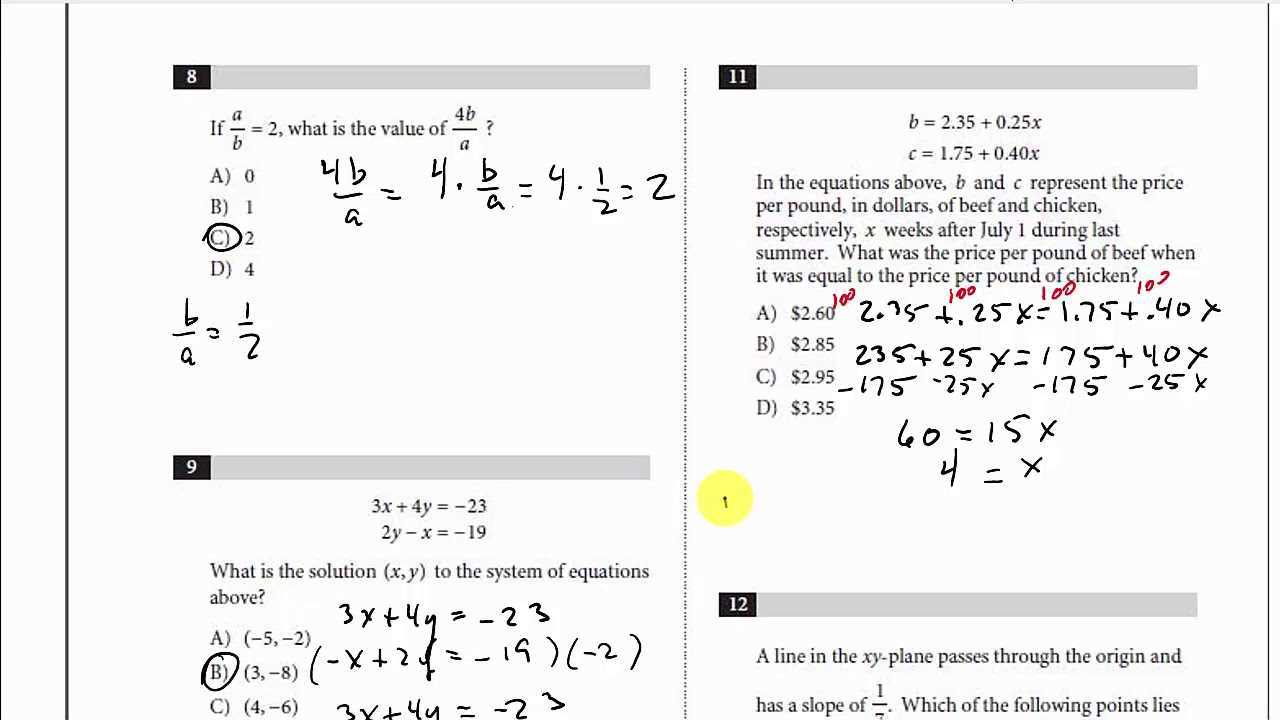
Word problems test your ability to translate real-world situations into mathematical expressions. This section typically includes problems related to ratios, proportions, and various application scenarios. The focus is on understanding how to extract relevant data and set up the correct equation to solve for the unknown.
- Translating Situations: Convert words into mathematical operations and expressions.
- Applications: Apply algebraic methods to solve problems in finance, geometry, and other areas.
Section 4: Quadratics and Higher-Level Problems
The final section often includes quadratic equations, factoring, and other advanced topics. You may be asked to solve quadratic equations using the quadratic formula or by factoring, as well as graph quadratic functions. Being comfortable with these concepts is crucial for completing this section successfully.
- Quadratic Equations: Solve using factoring, the quadratic formula, or completing the square.
- Factoring: Factor quadratic expressions and solve related problems.
By understanding the structure of each section and focusing your study efforts accordingly, you’ll be better prepared to tackle each part of the assessment. Practice each type of problem to build confidence and improve your problem-solving skills.
Time Management During the Keystone Exam
Effective time management is one of the most important skills to master when preparing for a major assessment. Having a solid plan allows you to pace yourself, avoid rushing through questions, and ensure that you complete every section to the best of your ability. Learning to manage your time efficiently during the test will reduce stress and improve your overall performance.
One key strategy is to allocate time for each section based on its complexity. Start by reading through the entire test quickly to get an overview, and then break down your time for each part. Prioritize easier sections first to gain confidence, but be mindful of the time spent on more challenging questions. Here are a few tips to help you manage your time effectively:
- Set Time Limits: Set a specific amount of time for each section or question, and stick to it. This prevents you from spending too long on any one part.
- Answer Easy Questions First: Quickly go through and answer the questions you find easiest. This will help you build momentum.
- Leave Difficult Questions for Later: If you encounter a question that seems too time-consuming, skip it and return to it once you’ve answered the rest of the test.
Additionally, remember to leave a few minutes at the end for review. Double-checking your answers ensures that you didn’t overlook any details or make simple mistakes. Practicing these time management strategies before the test can make a significant difference on the day of the assessment.
How to Use Practice Tests Effectively
Using mock assessments is one of the best ways to prepare for any high-stakes evaluation. These simulated tests provide insight into the format and types of questions you’ll encounter, helping you gauge your strengths and identify areas for improvement. However, simply taking a practice test isn’t enough–you need to approach them with a strategy to maximize their effectiveness.
1. Simulate Test Conditions
To get the most out of your mock tests, recreate the testing environment as closely as possible. Find a quiet place, set a timer, and avoid distractions. By practicing under realistic conditions, you’ll become familiar with the pressure of time constraints and improve your focus during the real assessment.
2. Analyze Your Results
After completing a practice test, review your answers thoroughly. Don’t just look at the questions you got wrong; examine why you made mistakes. Did you misinterpret the question? Were you too rushed? Did you forget to apply a certain method? Understanding the reasoning behind your errors will help you avoid repeating them in the future.
Additionally, track your progress over time. By taking multiple practice tests, you can see improvements in your speed and accuracy. This also helps you become more comfortable with the material, increasing your confidence as the test day approaches.
Remember, the key to using mock assessments effectively lies in active review. Simply completing the test isn’t enough–take the time to analyze your performance and use it as a learning tool to refine your skills.
Top Resources for Algebra 1 Prep
When preparing for a significant assessment, having the right tools and materials at your disposal can make all the difference. Whether you’re looking for practice materials, explanatory videos, or study guides, there are a variety of resources that can help you understand the concepts better and boost your readiness. These resources provide structured lessons, real-world examples, and tailored exercises that can significantly enhance your ability to perform under test conditions.
1. Online Practice Platforms
One of the most effective ways to prepare is by using online platforms that offer interactive lessons and quizzes. Websites like Khan Academy and IXL provide a wide range of content that adapts to your learning pace. These platforms allow you to practice problem-solving in a dynamic way, offering immediate feedback on your responses and highlighting areas for improvement.
2. Study Guides and Review Books
Comprehensive study guides and review books are excellent for a more structured approach to preparation. These guides typically cover every key topic in the curriculum, with clear explanations and practice questions at the end of each section. Popular review books like those from Barron’s or the Princeton Review break down complex topics into digestible chunks, making it easier to absorb and retain information.
Additionally, you can find many downloadable resources that focus specifically on problem-solving strategies, common pitfalls, and critical thinking exercises. Combining different types of study aids will provide a well-rounded preparation experience, helping you tackle a variety of challenges on the day of the test.
Improving Speed and Accuracy in Algebra
Enhancing both speed and accuracy is essential when preparing for a comprehensive test. Mastering these two skills allows you to tackle questions quickly while minimizing errors, which is crucial when time is limited. Achieving a balance between swift problem-solving and precision can be challenging, but with the right techniques, it is certainly attainable.
One effective strategy to increase speed is to focus on solving simpler problems first. This allows you to build momentum and boost confidence, which can help you when facing more complex questions. However, it’s important to maintain accuracy throughout the process. Developing a systematic approach, such as checking work as you go, can prevent careless mistakes and ensure that your answers are correct.
Another way to improve both speed and accuracy is by practicing mental math and recognizing patterns. Being able to identify shortcuts or quick methods to solve problems can save valuable time during the test. Additionally, reviewing common formulas and techniques regularly will make them second nature, allowing you to apply them quickly and correctly when needed.
Finally, consistent practice and timed drills are key. By simulating test conditions and challenging yourself to solve problems within a set time limit, you’ll become more comfortable working efficiently under pressure. Over time, you’ll notice improvements in both your speed and the precision of your answers, boosting your overall performance.
Why Reviewing Past Exams Helps
Reviewing previous assessments is one of the most powerful ways to improve your understanding and performance. By revisiting past tests, you can gain insights into the types of questions that are most commonly asked, as well as the specific areas where you may need further improvement. This process helps reinforce your knowledge and allows you to familiarize yourself with the format, ensuring you’re better prepared when it’s time for the real test.
1. Identifying Patterns and Trends
Past assessments often feature recurring themes or question types. By analyzing these tests, you can identify patterns in the types of problems presented and the common concepts that are tested. This allows you to focus your study efforts on the areas that matter most, improving your chances of success. Recognizing these trends can also help you predict what to expect and reduce the element of surprise during the actual test.
2. Understanding Mistakes and Strengthening Weak Areas
When you review previous tests, it’s crucial to focus on the mistakes you made and understand why they occurred. This gives you the opportunity to correct misconceptions and strengthen your weaker areas. By identifying where you struggled, you can tailor your preparation to address these specific gaps in your knowledge, ultimately improving both your accuracy and confidence.
Additionally, revisiting old tests allows you to track your progress over time. By comparing your performance on past assessments to your current abilities, you can see how much you’ve improved and what areas still need attention. This sense of progress can be motivating, encouraging you to keep refining your skills as the test date approaches.
Algebra 1 Practice Questions and Answers
Working through a variety of problems is one of the most effective ways to sharpen your skills and ensure you’re prepared for any assessment. By tackling a wide range of questions, you can build a solid understanding of key concepts and identify any areas that need additional attention. The more you practice solving different types of problems, the more confident and capable you’ll become when it comes time to apply your knowledge.
It’s important to focus on both the process and the final result. When solving each question, pay attention to the methods you use and double-check your work. Understanding why a solution works is just as important as finding the correct answer. By breaking down each problem step-by-step, you’ll be able to reinforce your comprehension of the material and improve your problem-solving strategies.
Additionally, reviewing the solutions to these problems can provide valuable insights into more efficient ways of tackling similar questions in the future. You’ll learn to recognize patterns and develop strategies that allow you to approach problems with greater speed and accuracy. The key to success lies not only in getting the right answer, but also in understanding how to reach it efficiently and without mistakes.
Key Algebra 1 Topics to Focus On
Mastering the foundational concepts in mathematics is essential for success in any assessment. By concentrating on the core topics, you can develop a deep understanding and apply it confidently. Focusing on the areas that are most commonly tested will help ensure you’re well-prepared. In this section, we’ll explore the most crucial topics that will sharpen your problem-solving abilities and provide a solid foundation for advanced mathematical challenges.
| Topic | Description |
|---|---|
| Linear Equations | Understanding how to solve for unknown variables in one-step, two-step, and multi-step equations. |
| Quadratic Equations | Techniques for solving quadratic equations using factoring, completing the square, and the quadratic formula. |
| Functions | How to identify, analyze, and work with different types of functions, including linear and non-linear functions. |
| Inequalities | Solving and graphing inequalities and understanding their real-world applications. |
| Polynomials | Factoring polynomials and understanding the operations involved in polynomial expressions. |
Each of these areas forms the backbone of the skills you’ll need to solve complex problems. By reviewing and practicing these topics, you’ll be able to approach various mathematical challenges with greater ease and efficiency. Make sure to allocate time to these key concepts to build a strong foundation and boost your performance in assessments.
What to Expect on the Algebra 1 Exam

When preparing for an important mathematics assessment, it’s essential to understand the format and types of questions you will encounter. Knowing what to expect can help reduce anxiety and improve performance. In this section, we will break down the key elements of the assessment and provide tips for approaching each section effectively.
Types of Questions

The test will consist of various question types, each assessing different skills and problem-solving techniques. You will be asked to demonstrate your ability to apply mathematical concepts and solve problems in a clear and organized manner.
- Multiple Choice Questions: These questions will test your understanding of key concepts. You will be given a set of options, and your task is to choose the correct answer.
- Short Answer Questions: You will need to write out your solution step-by-step, showing how you arrived at the answer.
- Word Problems: These questions will require you to interpret real-world scenarios and apply mathematical techniques to find solutions.
- Graphing Questions: Some questions will involve interpreting graphs or creating graphs based on given equations or data.
What to Focus On
It is important to focus on the topics that are most frequently covered on the assessment. Be sure to review the following areas:
- Understanding and solving linear equations
- Working with inequalities
- Factoring polynomials
- Solving quadratic equations
- Interpreting and creating graphs
By being prepared for the types of questions you’ll face and focusing on the most important topics, you can approach the assessment with confidence and a strong understanding of the material.