Big Ideas Math 6th Grade Answer Key

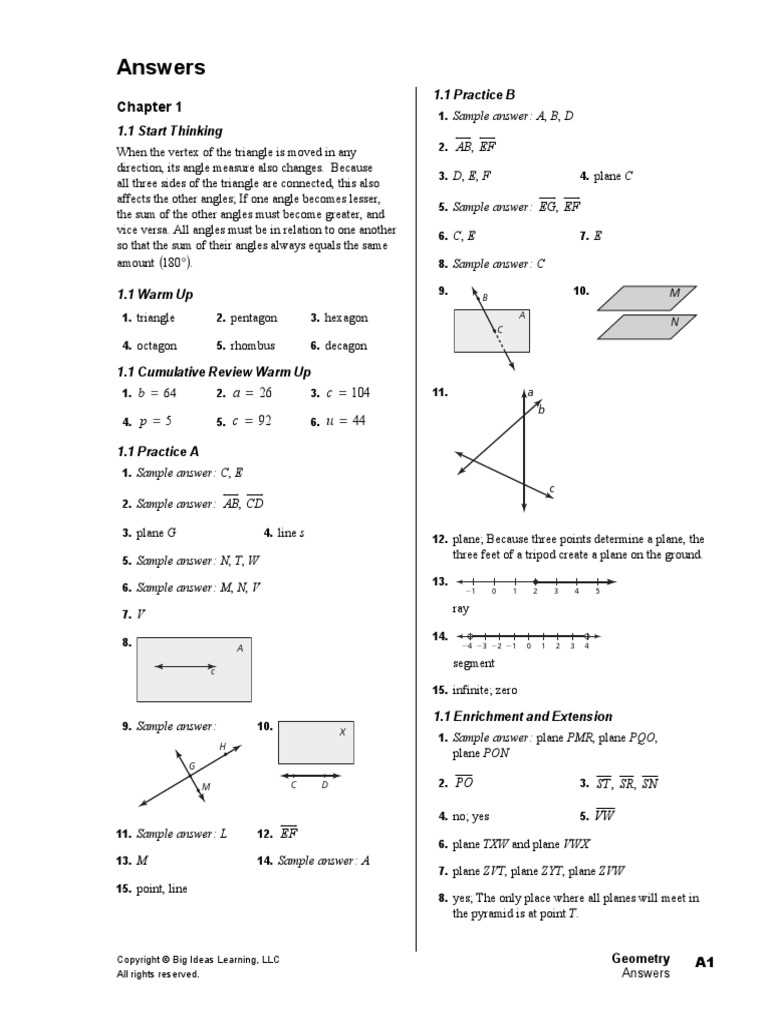
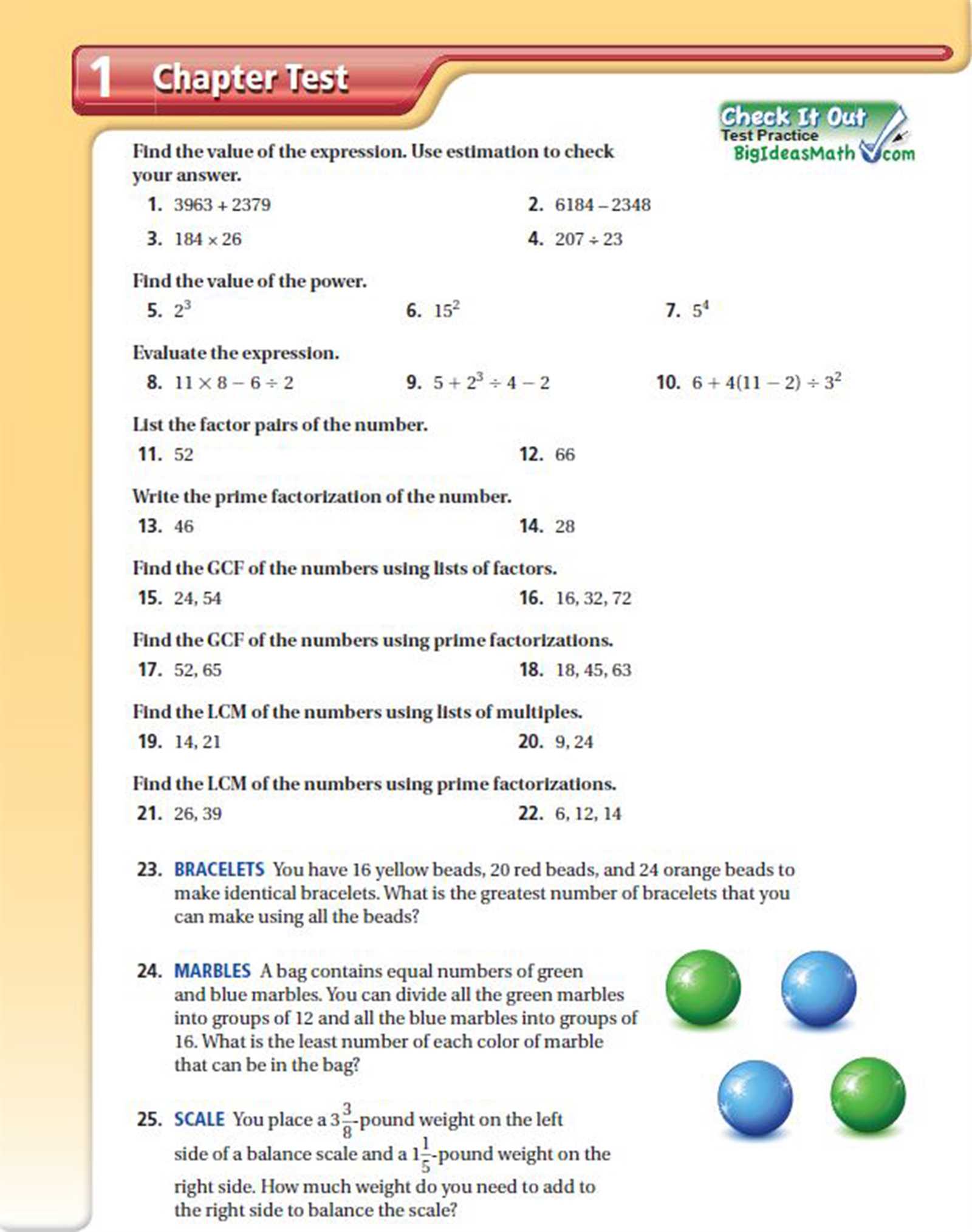
Understanding complex concepts in mathematics requires dedication and the right resources. Many students struggle with certain topics, which can make their learning journey more difficult. Having access to the right tools can significantly improve their ability to grasp new ideas and solve problems with confidence.

In this guide, we will explore effective strategies for mastering essential numerical skills. The focus is on providing support through detailed solutions that clarify common obstacles in various mathematical areas. Whether you’re tackling equations or working through geometry challenges, the resources offered here will help students develop the necessary skills to succeed.

Maximizing learning potential involves understanding the method behind every solution, not just memorizing the answers. Through practice and systematic review, students can become more confident and competent in their abilities, ultimately improving their overall performance in the subject.

Comprehensive Solutions for Learning Challenges

For students working through challenging topics in their mathematics curriculum, having access to reliable resources can make a significant difference in understanding complex concepts. Solutions that break down problems step by step can help clarify difficult tasks and provide students with the confidence needed to progress. In this section, we offer a structured approach to solving key problems encountered in the subject.

How to Effectively Use Problem-Solving Resources

One of the best ways to strengthen understanding is by reviewing worked-out examples. These guides allow students to see each problem tackled methodically, helping to reinforce their own ability to approach similar challenges. By following clear, logical steps, learners can develop a deeper comprehension of the processes involved in solving various mathematical exercises.

Common Obstacles and How to Overcome Them

Many students face specific difficulties when working through topics like fractions, decimals, or algebraic expressions. Addressing these challenges requires more than simply providing the correct solutions–it involves helping learners understand the reasoning behind each step. With consistent practice and thoughtful review of solutions, students can overcome these hurdles and gain a clearer grasp of each topic.

Overview of Comprehensive Learning Curriculum

The curriculum focuses on equipping students with the necessary skills to solve problems across various mathematical domains. With an emphasis on conceptual understanding, the program helps learners build a strong foundation in key areas such as algebra, geometry, and number operations. Through a progressive learning structure, students gain a deeper understanding of each topic, making complex concepts more approachable and easier to master.

Key Features of the Program

• Clear and structured progression through topics
• Practical applications to enhance real-world problem-solving skills
• Step-by-step instructions and examples for guided learning
• Frequent assessments to monitor progress and understanding
• Supportive resources designed to reinforce key concepts

Core Areas of Focus

1. Fractions and Decimals: Building a strong understanding of part-whole relationships
2. Equations and Expressions: Introducing algebraic thinking and problem-solving techniques
3. Measurement: Applying skills to real-life scenarios like distance, weight, and volume
4. Geometry: Exploring shapes, angles, and spatial relationships
5. Data and Statistics: Interpreting and analyzing data for informed decision-making

With its clear structure and engaging activities, this curriculum provides students with the tools to achieve mastery in foundational topics, ensuring academic success in subsequent levels of study.

Importance of Solution Guides in Learning

Having access to reliable solution guides plays a crucial role in the learning process, especially when tackling complex subjects. These resources not only provide correct results but also show the reasoning behind each step, allowing students to understand how to approach similar problems in the future. By reviewing solutions, learners can reinforce their comprehension and develop effective problem-solving strategies.

Why Solution Guides Are Essential

• Clarify the problem-solving process step-by-step
• Help identify common mistakes and avoid them in the future
• Provide a model for approaching unfamiliar or difficult tasks
• Boost confidence by offering clear, concrete solutions
• Encourage independent learning and self-assessment

How They Enhance Understanding

Reviewing how each problem is solved in detail offers valuable insight into the thought process behind the solution. This not only reinforces the material but also helps students develop a deeper understanding of the concepts. Instead of memorizing solutions, learners are guided to think critically, improving both their problem-solving abilities and their long-term retention of the material.

How to Use the Solution Guide Effectively

Using solution guides effectively requires more than simply checking your results. The true value lies in understanding the steps taken to reach each conclusion and applying those methods to new problems. By actively engaging with the provided solutions, students can enhance their comprehension, develop stronger problem-solving skills, and improve overall academic performance.

Steps for Maximizing Learning

• Attempt problems independently before consulting the guide
• Review each step in the solution, ensuring you understand the reasoning
• Compare your method with the provided solution and identify any differences
• Work through similar problems using the same approach
• Use the guide as a reference to clarify doubts but avoid dependency

Common Mistakes to Avoid

• Relying on the solution guide without attempting problems first
• Skipping steps while reviewing solutions
• Failing to understand the underlying concepts behind each solution
• Not practicing enough problems after reviewing the guide

When used properly, solution guides serve as powerful tools for learning. By ensuring you fully understand each process, you can develop the skills necessary to approach future challenges with confidence.

Common Challenges in Learning Mathematics

Students often encounter various obstacles when advancing through mathematical concepts, especially as the complexity of the material increases. These challenges can range from understanding abstract concepts to mastering problem-solving techniques. Identifying common difficulties early on can help educators and learners address them proactively, ensuring steady progress in the subject.

Key Areas of Difficulty

• Fractions and Decimals: Many students struggle with understanding the relationship between fractions and their decimal equivalents.
• Word Problems: Translating real-world scenarios into mathematical equations can be particularly challenging.
• Algebraic Concepts: Introducing variables and solving equations can seem abstract to younger learners.
• Geometry: Visualizing and calculating areas, angles, and shapes often presents a challenge.
• Multiplication and Division of Larger Numbers: Performing calculations with larger numbers requires practice and strong foundational skills.

How to Overcome These Challenges

• Practice regularly to reinforce concepts and techniques.
• Break down complex problems into smaller, more manageable steps.
• Use visual aids like diagrams and charts to clarify geometric and algebraic concepts.
• Encourage active problem-solving and critical thinking for word problems.
• Seek additional support and resources when a topic remains unclear.

By understanding these challenges and applying targeted strategies, students can gain a better grasp of the subject and overcome obstacles more effectively, building confidence and improving performance.

Strategies for Mastering Mathematical Concepts

Mastering mathematical concepts requires more than just memorizing formulas or procedures. It involves developing a deep understanding of the principles behind each concept and applying them to a variety of problems. To succeed, students need to adopt strategies that encourage active learning, critical thinking, and consistent practice. By following effective methods, learners can build a strong foundation and approach complex tasks with confidence.

Effective Learning Techniques

• Understand the Concepts: Focus on grasping the underlying ideas before attempting to solve problems. Ask questions like “Why does this work?” rather than just “How do I do this?”
• Break Down Problems: Complex tasks can be overwhelming. Break them into smaller, more manageable steps to understand the process better.
• Practice Regularly: Consistent practice is essential for reinforcing new skills. Working on similar problems regularly helps solidify knowledge.
• Use Visual Aids: Diagrams, charts, and visual representations can help clarify abstract concepts, especially in geometry and algebra.
• Seek Alternative Methods: If one approach isn’t working, try to solve the problem from a different angle. Experiment with various techniques until you find what works best for you.

Building Long-Term Mastery

• Review and Reflect: Regularly review previously learned material to reinforce your understanding and prevent forgetting.
• Teach Someone Else: Explaining a concept to another person can help solidify your own understanding and identify any gaps in knowledge.
• Stay Patient and Persistent: Mastery takes time, so remain patient and keep practicing, even when concepts seem difficult.
• Use Supplemental Resources: Use additional learning tools like online videos, interactive exercises, or study groups to deepen your understanding.

By incorporating these strategies into their study routine, students can build a solid mathematical foundation, enhancing their ability to solve problems and apply knowledge with ease.

How to Approach Word Problems

Word problems can often seem intimidating, as they require not only mathematical skills but also the ability to translate real-world situations into mathematical expressions. However, with the right approach, solving these types of problems can become a manageable and even enjoyable task. The key is to break the problem down into smaller, understandable parts and carefully identify what information is given and what needs to be found.

Steps for Solving Word Problems

• Read the problem carefully: Begin by reading the problem slowly and carefully to fully understand the situation being described.
• Identify key information: Highlight or underline important numbers, keywords, and conditions that are relevant to the solution.
• Determine what is being asked: Clarify exactly what the problem is asking you to find. Look for words like “how many,” “total,” or “difference” to understand the goal.
• Set up an equation: Based on the information you’ve identified, create an equation or mathematical expression that represents the problem.
• Solve step by step: Work through the problem systematically, solving one part at a time while keeping track of your calculations.
• Check your answer: After solving the problem, revisit the question and check if your answer makes sense in the context of the scenario.

Tips for Overcoming Challenges

• Use a visual aid: Drawing a diagram or chart can help organize the information and make relationships clearer.
• Look for patterns: Word problems often follow certain patterns. Identifying these can simplify the problem-solving process.
• Practice regularly: The more you practice, the more comfortable you will become with interpreting and solving word problems.
• Take your time: Don’t rush through the problem. Take your time to carefully read and analyze each part before moving on to the solution.

With practice and patience, approaching word problems can become a straightforward and rewarding experience. By following these strategies, students can develop the skills needed to solve even the most complex challenges with confidence.

Understanding Fractions and Decimals

Understanding fractions and decimals is essential for grasping fundamental concepts in arithmetic. Both are different ways of representing parts of a whole, and they are used in various real-world situations, such as measurements, money, and dividing items. While fractions express numbers as parts of a whole using a numerator and denominator, decimals provide a way to represent those parts in a base-10 format, making them particularly useful for calculations and comparisons.

To master these concepts, it is important to recognize how fractions and decimals are related. For instance, a fraction like 1/2 is equivalent to the decimal 0.5. Knowing how to convert between these two forms allows for easier calculations and deeper understanding. The ability to work with both fractions and decimals also improves one’s ability to solve a wide range of problems, from basic operations to more complex applications in various fields.

Solving Algebraic Expressions with Confidence

Solving algebraic expressions may seem daunting at first, but with the right approach, it becomes a manageable and rewarding skill. The key to mastering these types of problems is to understand the structure of the expression and break it down step by step. By applying rules and operations systematically, anyone can confidently solve for unknowns and simplify complex expressions.

To approach algebraic expressions effectively, it’s crucial to follow a few basic principles. First, always perform operations in the correct order, often referred to as PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction). Next, combine like terms where possible to simplify the expression. Finally, isolate the variable by reversing the operations in the equation to solve for the unknown.

With consistent practice and a clear understanding of these steps, anyone can solve algebraic problems with greater ease and accuracy, building a solid foundation for more advanced mathematical concepts.

Working with Ratios and Proportions

Understanding ratios and proportions is essential for solving real-world problems that involve comparisons and relationships between different quantities. Ratios express the relationship between two numbers, while proportions show how two ratios are equivalent. Mastering these concepts helps with tasks like scaling recipes, analyzing data, and working with measurements in various fields.

Solving Ratios

To work with ratios, begin by identifying the quantities being compared. A ratio can be expressed as a fraction, a colon, or the word “to,” depending on the context. For example, a ratio of 4 to 5 can be written as 4:5 or 4/5. Once you understand the relationship between the numbers, you can perform operations like simplifying the ratio or using it to compare with other ratios.

Understanding Proportions

Proportions are equations that express the equality of two ratios. For example, if 2/4 = 6/x, you can solve for x by cross-multiplying. Proportions are used to solve problems involving scaling, resizing, or adjusting values in a consistent manner. By understanding how to set up and solve proportions, you can apply them to a wide range of practical scenarios.

Mastering the use of ratios and proportions allows for easier comparison and analysis of quantities, and these skills are crucial for both everyday tasks and more advanced mathematical applications.

Geometry Skills for 6th Graders

Geometry is a fundamental branch of mathematics that focuses on the properties, measurements, and relationships of shapes and spaces. Developing a solid understanding of geometry is essential for students, as it helps build problem-solving skills and enhances spatial awareness. In this section, we will explore key concepts and essential skills that students need to master in geometry, such as understanding different types of shapes, measuring angles, and calculating areas and perimeters.

Key Geometry Concepts

Concept	Description
Types of Shapes	Understanding basic geometric shapes, such as squares, rectangles, circles, triangles, and polygons, and their properties.
Angles	Learning how to measure and identify different types of angles: acute, right, obtuse, and straight.
Perimeter	Calculating the perimeter of various shapes by adding up the lengths of their sides.
Area	Determining the area of shapes by applying formulas (e.g., length x width for rectangles).

Practical Applications of Geometry

Geometry is not just about solving problems on paper; it also has real-world applications. For example, knowing how to calculate the area and perimeter of a space is useful when designing rooms or planning construction projects. Additionally, understanding shapes and angles is crucial for activities like architecture, engineering, and even art. By honing these geometry skills, students will be able to approach complex problems with confidence and see how geometry is connected to everyday life.

Improving Problem-Solving Techniques

Enhancing problem-solving abilities is essential for tackling challenges across various subjects. Developing effective strategies allows individuals to approach complex questions with clarity and confidence. By understanding different methods and applying them systematically, students can improve their reasoning and analytical skills. Whether it’s breaking down a difficult equation or analyzing a real-world issue, strong problem-solving skills are critical for academic success and beyond.

One effective approach is to first thoroughly understand the problem. Identify the key information and what is being asked. Then, break the problem into smaller, more manageable parts. This will make it easier to identify patterns or connections that may not be immediately obvious. Additionally, practicing different techniques, such as drawing diagrams, making tables, or using trial and error, can help clarify complex problems.

Lastly, it’s important to reflect on the solution once the problem is solved. Reviewing the steps taken and verifying the results ensures accuracy and provides valuable insights for future challenges. Over time, consistent practice will help strengthen problem-solving skills and build a deeper understanding of the process.

Reviewing Key Math Operations

Mastering fundamental mathematical operations is essential for building a strong foundation in numerical problem solving. Understanding and applying these operations effectively enables students to tackle a wide range of challenges across various areas of study. In this section, we will review the core operations–addition, subtraction, multiplication, and division–and explore strategies for using them with confidence in different contexts.

Addition is the process of combining two or more numbers to find their total. It is one of the most basic yet essential skills in mathematics. To perform addition efficiently, it’s helpful to break down larger numbers into simpler parts, making mental calculations easier.

Subtraction involves determining the difference between two numbers. Mastering this operation requires an understanding of place value and the ability to work with negative numbers when applicable. Practicing subtraction helps students develop a clearer sense of how numbers relate to one another.

Multiplication is the repeated addition of a number and is often used to solve problems involving groups or sets. By recognizing patterns and learning multiplication tables, students can speed up their calculations and improve accuracy. Visualization methods, such as arrays, can also help reinforce multiplication concepts.

Division is the process of splitting a number into equal parts. This operation can be thought of as the inverse of multiplication. Dividing large numbers can be challenging, but breaking them down into smaller steps and practicing long division techniques can enhance precision and speed.

By reviewing and practicing these core operations regularly, students can strengthen their computational skills and gain the confidence to approach more advanced mathematical problems with ease.

Practical Applications of Math in Real Life

Mathematics is not only a subject studied in classrooms but also a powerful tool used in everyday life. From managing personal finances to planning trips, math plays a vital role in many daily activities. Understanding how mathematical concepts apply to real-world situations helps individuals make informed decisions, solve problems, and optimize their resources effectively.

Everyday Scenarios Where Math Is Useful

Here are several practical areas where mathematical skills are essential:

• Budgeting and Personal Finance: Managing money involves understanding percentages, interest rates, and calculating expenses. Whether you’re saving for a goal or tracking monthly spending, these skills are crucial.
• Shopping and Discounts: When shopping, knowing how to calculate discounts, compare prices, or determine sales tax can help you make smarter purchasing decisions.
• Cooking and Recipes: Recipes often require adjustments based on serving sizes. Understanding fractions and proportions allows you to scale ingredients accurately and achieve the best results.

Real-World Applications in Professional Fields

Mathematical concepts extend beyond personal tasks and are fundamental in various professional sectors:

• Engineering and Architecture: Engineers and architects rely on geometry, measurement, and calculations to design structures, ensure stability, and optimize resources.
• Technology and Software Development: Programmers and developers use algorithms, logic, and problem-solving techniques to create software, solve technical issues, and improve user experience.
• Healthcare and Medicine: Medical professionals use statistics and calculations to interpret test results, measure medication dosages, and analyze trends in public health data.

By understanding how math intersects with real-life situations, students can see its relevance and develop a deeper appreciation for the subject. Applying these skills daily not only enhances problem-solving abilities but also fosters confidence in navigating the complexities of modern life.

How to Prepare for Math Assessments

Preparing for assessments in any subject, especially mathematics, requires both strategic planning and consistent effort. It’s essential to understand the key topics, practice regularly, and build confidence in solving problems. With the right approach, students can perform better during evaluations and even reduce anxiety surrounding exams.

Key Strategies for Effective Preparation

Here are some proven strategies to help students prepare effectively:

• Review Core Concepts: Focus on understanding the foundational principles behind each topic. Revisit important rules and formulas, as these are often the basis for most problems.
• Practice Regularly: Consistent practice is crucial for reinforcing concepts and identifying areas that need more attention. Use worksheets, online quizzes, or practice tests to sharpen your skills.
• Identify Weak Areas: Take time to pinpoint specific concepts that you find challenging. Work on these areas with additional resources such as tutoring or instructional videos.
• Use Real-Life Applications: Relate problems to real-world situations. This helps contextualize abstract concepts and makes them easier to understand and remember.

Time Management and Stress Reduction

Managing your time and reducing stress are crucial components of successful preparation:

• Create a Study Schedule: Break down your study time into manageable chunks. Set aside specific hours for review, and stick to your plan to avoid last-minute cramming.
• Take Regular Breaks: Avoid burnout by taking short breaks between study sessions. This helps keep your mind fresh and improves focus during study time.
• Stay Calm and Confident: Keep a positive mindset. Practice relaxation techniques like deep breathing or meditation to reduce anxiety before and during the exam.

Sample Study Plan for Math Preparation

Day	Focus Area	Time Spent
Monday	Review basic operations and fractions	1 hour
Tuesday	Practice word problems and equations	1 hour
Wednesday	Study geometry concepts	1 hour
Thursday	Focus on ratios and proportions	1 hour
Friday	Take a practice test	1 hour

By following these strategies and maintaining a consistent study routine, students can improve their performance and approach assessments with confidence.

Support Resources for Struggling Students

For students facing difficulties in understanding key concepts, seeking out additional support and resources can make a significant difference in their academic progress. With the right guidance, encouragement, and tools, these students can overcome challenges and build a stronger foundation for future learning.

Effective Support Resources

Here are some helpful resources that can aid students in improving their understanding and skills:

• Tutoring Services: Personalized one-on-one sessions with a tutor can address specific challenges and provide focused help in areas of difficulty.
• Online Educational Platforms: Websites and apps offering interactive exercises, videos, and lessons can be invaluable for reinforcing learning outside the classroom.
• Peer Support: Forming study groups with classmates can provide collaborative learning opportunities, allowing students to help one another with difficult topics.
• Teacher Office Hours: Many educators offer extra time after school for students to ask questions and receive additional explanations on confusing topics.

How to Utilize Support Resources

Students should adopt a strategic approach when utilizing support resources:

• Be Proactive: Seek help early before problems escalate. The sooner a student identifies a struggle, the easier it is to find a solution.
• Practice Regularly: Consistent practice with support resources helps reinforce learning and boosts confidence over time.
• Communicate Openly: Be open with teachers, tutors, or study group members about the areas where help is needed. This ensures targeted assistance and progress.

Example of a Weekly Support Plan

Day	Resource	Activity
Monday	Tutor Session	Review challenging concepts from the previous week’s lessons
Tuesday	Online Educational Platform	Complete interactive exercises on key topics
Wednesday	Study Group	Collaborative problem-solving and discussion of difficult questions
Thursday	Teacher Office Hours	Ask questions and clarify doubts regarding upcoming lessons
Friday	Review Notes	Self-assess understanding and practice additional exercises

By effectively utilizing these resources, students can make significant strides in their learning and feel more confident in their academic abilities.

Benefits of Consistent Practice

Regular and consistent practice in solving problems is one of the most effective ways to improve proficiency in any subject. For students, dedicating time each day to practice reinforces concepts, strengthens understanding, and helps them retain information for the long term. This approach not only builds confidence but also encourages the development of problem-solving skills that can be applied across various topics.

Key Advantages of Regular Practice

Here are some of the primary benefits of incorporating daily practice into the learning routine:

• Improved Retention: Consistent practice ensures that concepts are solidified in memory, making it easier to recall them when needed.
• Enhanced Problem-Solving Speed: Regular exposure to different types of problems helps students become quicker and more efficient in finding solutions.
• Greater Confidence: As students practice more, they become more comfortable with complex tasks, leading to greater self-assurance in their abilities.
• Mastery of Concepts: With repeated practice, students are able to master key ideas and strategies, ensuring they can apply them effectively in new situations.

How to Make Practice More Effective

To maximize the benefits of regular practice, students should follow a structured approach:

• Set Specific Goals: Define what to focus on during each practice session. This could involve specific skills or problems that need attention.
• Break Down Tasks: Start with simple exercises and gradually increase the difficulty to build confidence and mastery over time.
• Stay Consistent: Make practice a daily habit, even if it’s only for a short amount of time. Consistency is key to retaining and reinforcing knowledge.
• Review Mistakes: Take the time to go over errors and understand where things went wrong. This reflective practice helps improve problem-solving strategies.

By embracing a consistent practice routine, students will not only improve their overall understanding but also develop the skills needed to tackle more advanced topics with ease and confidence.

Using Technology to Enhance Learning

In today’s educational landscape, technology plays a pivotal role in supporting and enhancing students’ learning experiences. By integrating digital tools and resources, learners can access a variety of materials, work at their own pace, and engage with interactive content that deepens their understanding. Technology offers personalized learning opportunities, fostering a more tailored approach that addresses individual needs.

Benefits of Technology in Education

Here are several key advantages of incorporating digital tools into the learning process:

• Interactive Learning: Tools such as simulations, educational games, and interactive tutorials help students visualize complex concepts and engage in hands-on learning.
• Access to Resources: Online platforms and apps provide a wealth of educational materials, from video tutorials to practice exercises, making it easier for students to find additional help when needed.
• Personalized Learning Paths: Adaptive software can adjust the difficulty and type of content based on a student’s performance, providing a more customized learning experience.
• Immediate Feedback: Many digital tools offer instant feedback on exercises, helping students identify mistakes and correct them right away, which is crucial for mastering concepts.
• Increased Engagement: Technology can make learning more dynamic, with multimedia resources keeping students interested and motivated to learn.

How to Use Technology Effectively

To make the most of digital tools, students should consider the following strategies:

• Set Clear Goals: Know what you want to achieve before using a particular tool or app. Whether it’s reviewing a concept or practicing a skill, having a clear goal makes the session more productive.
• Choose the Right Tools: Use tools that are designed for your current learning needs. Some platforms focus on practice, while others provide explanations and step-by-step guides.
• Balance with Traditional Learning: While technology is a valuable resource, it should complement traditional study methods. Combine online practice with textbooks, writing exercises, and hands-on activities for well-rounded learning.
• Track Progress: Many educational platforms offer tracking features that allow students to monitor their progress over time, which can be motivating and helpful for adjusting learning strategies.

By leveraging the power of technology, students can enhance their understanding, reinforce concepts, and develop critical thinking skills that will serve them well beyond the classroom.