
Learning mathematics can often be a challenging journey, especially when faced with complex concepts and problems. Having the right resources to guide you through each step is essential for success. With the right tools, you can enhance your understanding and gain confidence in solving even the most difficult equations.
One of the most effective ways to check your progress is by using reliable reference materials. These resources not only provide solutions but also offer explanations that help clarify the steps involved. Whether you’re a student looking to strengthen your skills or a teacher seeking additional support for your class, having access to these materials is invaluable for mastering the subject.
Mastering problem-solving techniques is a gradual process that requires practice, focus, and the right guidance. With the right approach, solving mathematical challenges becomes more manageable and less intimidating. This guide will help you navigate through the various strategies available to improve your skills and achieve your learning goals.
Big Ideas Math Blue Answer Key Overview
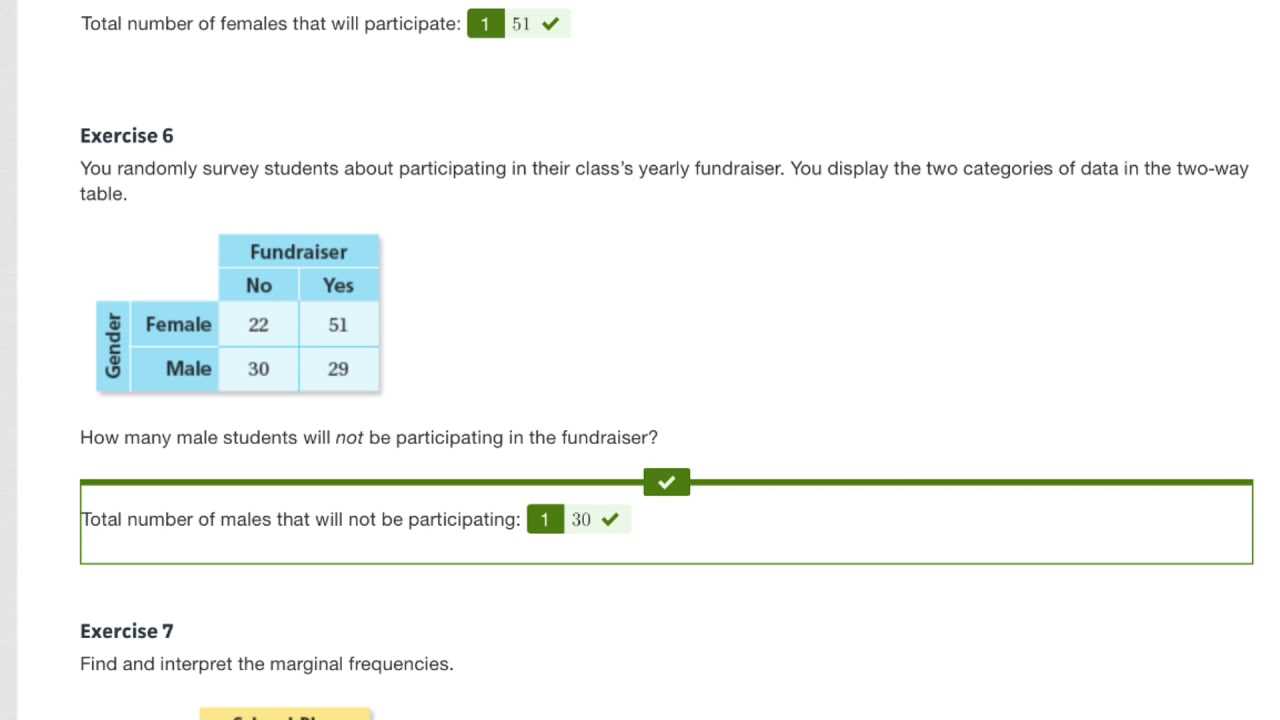
This section provides a comprehensive look at a valuable resource for anyone tackling complex problems in mathematics. It offers an organized approach to solving exercises and understanding the steps necessary for achieving the correct solutions. By utilizing this resource, students and educators alike can ensure a deeper grasp of challenging concepts while reinforcing their learning techniques.
The resource serves as an essential companion for reviewing and cross-checking work. It offers more than just solutions by breaking down the reasoning behind each step, fostering a better understanding of how to approach problems methodically.
| Topic | Description |
|---|---|
| Step-by-Step Explanations | Each problem is broken down into easy-to-follow steps, making it easier to understand the process behind each solution. |
| Concept Reinforcement | The resource helps reinforce key principles, providing a deeper understanding of mathematical ideas. |
| Solution Verification | It serves as a tool for confirming the correctness of your solutions and refining problem-solving strategies. |
| Additional Practice | Offers extra exercises to further enhance learning and test comprehension beyond the initial problems. |
In essence, this tool not only supports learning but also encourages a more structured approach to mastering the subject. It’s an indispensable aid in navigating through different levels of problem-solving. Whether used in the classroom or at home, it provides clarity and guidance every step of the way.
What is Big Ideas Math Blue?
This educational program is designed to provide a comprehensive framework for mastering essential concepts in mathematics. It combines structured lessons, engaging activities, and clear instructions to support students at various stages of their learning journey. The system offers a variety of resources that cater to different learning styles, ensuring that each student has the tools they need to succeed.
Focused on building a strong foundation, this curriculum emphasizes problem-solving and critical thinking skills. By presenting mathematical concepts in a clear and organized manner, it helps learners grasp even the most complex topics with ease. The program is often used in classrooms and as a self-study tool, allowing students to work at their own pace while receiving the necessary guidance for success.
With its wide range of interactive features, this resource not only teaches mathematical operations but also fosters a deeper understanding of how and why certain solutions work. It’s designed to promote confidence and mastery in mathematics, providing support both inside and outside the classroom environment.
How to Use the Answer Key
Using a solution guide effectively requires a strategic approach to maximize its benefits. This resource is designed to assist in checking your work and understanding the reasoning behind each step of the problem-solving process. When used correctly, it can clarify concepts and reinforce learning by showing the correct path to follow.
Start by attempting the exercises on your own before consulting the resource. This allows you to identify areas where you might need extra help and helps to strengthen your problem-solving skills. Once you’ve made an attempt, refer to the guide to verify your results and see a breakdown of the steps taken to arrive at the correct solution.
Key points to remember when using this tool:
- Review Each Step: Don’t just look at the final solution. Understand the logic and methods used to reach the conclusion.
- Focus on Patterns: Pay attention to common approaches and strategies that can be applied to similar problems.
- Learn from Mistakes: If your solution differs from the provided one, use the guide to pinpoint where your method may have gone wrong and adjust accordingly.
By following these steps, you can use this resource to enhance your understanding, correct mistakes, and build confidence in your problem-solving abilities.
Common Math Topics Covered
This resource addresses a wide range of essential mathematical concepts, ensuring that students gain a comprehensive understanding of the subject. From basic arithmetic to more advanced topics, it covers the necessary skills required to excel in the field. The program is structured to progressively build knowledge, making even complex ideas easier to grasp.
Algebraic Expressions and Equations
Algebra forms the foundation of many advanced mathematical concepts. Topics such as simplifying expressions, solving linear equations, and working with inequalities are all explored in detail. These concepts are crucial for developing logical thinking and problem-solving skills.
Geometry and Measurement
Understanding shapes, sizes, and spatial relationships is central to geometry. The program covers topics such as calculating areas, perimeters, volumes, and understanding geometric properties. Students also explore coordinate geometry and transformations, providing them with tools for solving real-world problems.
Understanding Key Concepts in Big Ideas Math
Grasping the core principles of mathematics is essential for success in solving problems and applying knowledge in real-world situations. This resource helps clarify these foundational ideas, providing a clear framework for students to understand complex topics. Whether it’s algebraic thinking, geometric principles, or data analysis, mastering these concepts is crucial for building advanced skills.
Key ideas such as the relationship between numbers, patterns in equations, and the application of formulas are consistently emphasized. By focusing on these areas, learners can develop a solid understanding that will serve as the basis for tackling more challenging problems. This approach not only improves mathematical abilities but also strengthens critical thinking and analytical skills.
Furthermore, the breakdown of concepts into manageable sections allows students to progress at their own pace. Each topic is presented in a logical sequence, ensuring that learners can build on previous knowledge and move forward with confidence.
How the Answer Key Enhances Learning
Having access to a solution guide significantly contributes to the learning process by providing clarity and insight into the methods used to solve problems. Rather than just offering the final results, this resource helps students understand the logic behind each step, deepening their comprehension and reinforcing their problem-solving skills. By reviewing each solution, learners can identify areas for improvement and refine their approach to similar challenges.
Additionally, the guide promotes self-paced learning. It allows students to attempt exercises independently, then check their progress and correct any mistakes. This encourages a proactive approach to learning, where students are not merely relying on external explanations but are actively engaged in the process of discovery and correction.
Through consistent use, the guide helps students develop critical thinking and analytical skills. By seeing the rationale behind each solution, they learn to approach problems more methodically, which not only aids in the current subject but also fosters lifelong problem-solving abilities.
Step-by-Step Guide for Students
This section provides a clear, structured approach to help students navigate through complex problems and improve their learning experience. By following a methodical process, students can break down challenges into manageable steps, making problem-solving more accessible and less overwhelming.
Follow these steps to ensure effective learning and mastery of each topic:
- Read the Problem Carefully: Begin by thoroughly understanding the question. Identify what is being asked and the key information provided.
- Make a Plan: Decide on the best approach to solve the problem. This could involve choosing the appropriate formula, method, or strategy.
- Work Through the Solution: Start solving the problem step by step. Don’t rush; take your time to ensure each part is done correctly.
- Check Your Work: Once you have a solution, double-check your calculations and the reasoning behind each step. Make sure the solution makes sense in the context of the problem.
- Use the Solution Guide: If you’re unsure about your answer or approach, refer to the solution guide for a detailed breakdown. Compare each step and correct any mistakes.
- Practice Regularly: To master the concepts, practice similar problems. Repetition is key to reinforcing learning and improving confidence.
By following these steps, students can improve their problem-solving skills, build a deeper understanding of the subject, and approach mathematical challenges with greater confidence.
Teacher’s Role with Answer Key
Educators play a crucial role in guiding students through the learning process, and the solution guide serves as an essential tool in this journey. While students can use the resource to check their progress, teachers help ensure that learners understand the reasoning behind each solution. This guidance fosters a deeper comprehension of the concepts and ensures that students are not merely memorizing steps but truly grasping the material.
Supporting Independent Learning

Teachers can use the solution guide to encourage students to think critically and independently. By allowing learners to first attempt problems on their own, instructors can focus on addressing individual challenges rather than providing direct answers. The guide offers a reference for students to verify their work, but it is the teacher’s role to help students understand why a particular approach works, reinforcing the learning process.
Facilitating Classroom Discussions
In the classroom, the resource acts as a reference for collaborative learning. Teachers can use the guide to facilitate discussions, comparing different problem-solving strategies and encouraging peer learning. This interaction helps reinforce key concepts, allowing students to see different perspectives and understand various methods of approaching problems.
Key Teacher Functions:
- Clarifying Concepts: Use the guide to explain complex topics in simpler terms, ensuring all students can follow along.
- Providing Feedback: Offer constructive feedback on students’ solutions, highlighting areas for improvement and helping them refine their problem-solving methods.
- Encouraging Critical Thinking: Encourage students to question their answers and reasoning, promoting a deeper understanding of the material.
Ultimately, the solution guide is a powerful resource that, when used effectively, enhances both teaching and learning, allowing students to develop a strong foundation in the subject while benefiting from their instructor’s expertise.
How to Verify Your Solutions
Verifying your work is an essential step in the learning process, ensuring that your solutions are accurate and the methods used are correct. Checking your answers allows you to identify mistakes, reinforce your understanding, and build confidence in your problem-solving skills. By following a systematic approach, you can evaluate whether your solution is reliable and whether the process used to obtain it is sound.
Steps to Verify Your Solutions
Follow these steps to confirm the correctness of your results:
- Recheck the Problem Statement: Before you begin verifying your solution, carefully read the original question again to ensure you understand what is being asked. Sometimes errors occur because of a misinterpretation of the problem.
- Rework the Solution: Go through each step of your solution again. Ensure that every calculation, operation, and logic follows correctly from one step to the next.
- Use Alternative Methods: If possible, try solving the problem using a different approach. This not only confirms your answer but also strengthens your understanding of different problem-solving strategies.
- Check Units and Dimensions: If the problem involves measurements, ensure that all units are consistent and properly converted, if necessary. This step can often help catch errors that are easy to overlook.
- Consult the Solution Guide: Compare your work with the reference guide to ensure that your solution matches the correct one. Focus on the steps taken, not just the final result, and note any differences in approach.
Common Mistakes to Watch For
While verifying your work, be mindful of these common issues:
- Calculation Errors: Double-check basic arithmetic, especially when working with decimals or fractions.
- Incorrect Application of Formulas: Ensure that you are using the correct formulas and applying them properly in the context of the problem.
- Sign Mistakes: Pay attention to positive and negative signs, particularly in equations and inequalities.
- Units of Measurement: Ensure that all units are consistent and converted properly if necessary.
By following these steps and being mindful of common errors, you can confidently verify your solutions and improve your overall problem-solving ability.
Benefits of Using Answer Keys
Utilizing a solution guide offers a wide range of advantages that contribute significantly to a student’s learning process. These resources not only provide correct solutions but also help students develop critical problem-solving skills, build confidence, and improve their understanding of various concepts. With a clear reference to compare their work, students can easily identify mistakes and learn how to correct them, which is essential for mastery of the material.
Here are some key benefits of using solution guides:
| Benefit | Description |
|---|---|
| Improved Accuracy | Solution guides provide the correct steps and answers, allowing students to verify their work and ensure their solutions are accurate. |
| Increased Confidence | By checking their progress against a trusted source, students gain confidence in their ability to solve problems correctly. |
| Enhanced Learning | Reviewing the solutions helps students understand the reasoning behind each step, deepening their comprehension of the concepts involved. |
| Immediate Feedback | Students can quickly identify mistakes and take corrective action, reducing the chances of reinforcing incorrect understanding. |
| Self-Directed Learning | With a solution guide, students can work independently and at their own pace, developing self-reliance and autonomy in their studies. |
By using solution guides, learners not only validate their answers but also refine their problem-solving techniques, making it a valuable tool for both independent and guided study. This resource aids in reinforcing the concepts and approaches required to excel in the subject at hand.
Effective Study Techniques for Math
To succeed in solving complex problems and mastering new concepts, it’s essential to develop a solid study routine. Effective study strategies can make all the difference in improving problem-solving skills and understanding difficult concepts. By incorporating active learning techniques, students can enhance their retention and apply what they’ve learned more effectively. Here are some proven methods to help you excel in your studies.
Key Study Techniques
- Practice Regularly: Consistent practice is the cornerstone of mastering any subject. Set aside time each day to solve problems and work through exercises to reinforce what you’ve learned.
- Break Down Complex Problems: When faced with a difficult problem, break it down into smaller, more manageable steps. Tackle each part one at a time and gradually piece everything together.
- Review Mistakes: Learn from your errors by going back over incorrect solutions. Understanding where you went wrong and why is crucial for avoiding similar mistakes in the future.
- Use Active Recall: Instead of just reading through notes, try to recall key concepts and solutions without looking at your references. This strengthens memory retention and problem-solving skills.
- Teach What You Learn: Explaining concepts to others or even to yourself reinforces your own understanding. Teaching is one of the best ways to solidify new knowledge.
- Group Study Sessions: Collaborating with classmates allows for sharing different problem-solving approaches. Group discussions can uncover new insights and clarify confusing topics.
Utilizing Resources Effectively
- Leverage Study Guides: Use solution guides to verify your work and ensure your approach is correct. Reviewing the steps can help you identify any gaps in your understanding.
- Take Advantage of Online Resources: Explore online tutorials, videos, and problem sets to reinforce difficult concepts or get additional practice.
- Stay Organized: Keep your notes and study materials well-organized. A clean, methodical study environment can help you stay focused and reduce unnecessary stress.
By adopting these strategies, you can improve your performance, boost your confidence, and approach problems with a clearer and more efficient mindset. Success comes from persistence, practice, and applying the right techniques in your study routine.
How to Approach Problem-Solving
Solving complex problems requires a clear, structured approach that helps break down the issue into manageable steps. A thoughtful method ensures that the problem is understood, all available information is considered, and a solution is found effectively. By following a strategic process, you can tackle even the most challenging tasks with confidence and clarity.
Here are key steps to guide your problem-solving process:
- Understand the Problem: Take the time to fully read and comprehend the problem. Identify what is being asked and highlight key information that will help you proceed.
- Break It Down: Divide the problem into smaller, more manageable parts. Addressing each section step-by-step prevents feeling overwhelmed and allows you to focus on one thing at a time.
- Plan Your Approach: Consider various strategies that could help solve the problem. Whether it’s working through examples, drawing diagrams, or using logical reasoning, having a clear approach can guide your work.
- Execute the Plan: Apply the chosen method carefully and systematically. As you work through the solution, check your progress to ensure you are heading in the right direction.
- Review Your Work: After reaching a solution, double-check your steps and the final answer. This helps identify any errors and ensures your reasoning is sound.
- Reflect and Learn: Once the problem is solved, take a moment to reflect on the process. Understand why the chosen approach worked and how it can be applied to similar problems in the future.
By adopting these steps, problem-solving becomes a structured, manageable task rather than an overwhelming challenge. Practicing this approach regularly strengthens your ability to think critically and develop solutions efficiently.
Finding Extra Resources for Practice
To truly master a subject, it is essential to seek out additional resources beyond the standard materials. Extra practice allows you to reinforce concepts, improve your problem-solving skills, and build confidence. Fortunately, there are many accessible tools available that can supplement your learning and provide extra exercises for deeper understanding.
Here are some effective ways to find supplementary practice materials:
- Online Educational Websites: Many websites offer free practice problems and step-by-step solutions. Websites like Khan Academy, Coursera, and other educational platforms provide interactive exercises tailored to various topics.
- Practice Books: Consider investing in books with additional exercises. These often come with varying levels of difficulty and can help reinforce foundational concepts or introduce new ones.
- Educational Apps: Mobile apps designed for learning often include practice sets and quizzes. Apps such as Quizlet or Wolfram Alpha allow for on-the-go practice and provide immediate feedback.
- Online Forums and Communities: Join online forums or study groups, where you can access shared resources and exchange practice materials with others. Reddit and StackExchange are two popular platforms with active learning communities.
- Teacher-Provided Resources: Don’t forget about the resources your instructor may offer. Many teachers provide extra worksheets, online links, or recommend specific practice materials for students to use outside of class.
- Tutoring Services: If you need more personalized help, consider tutoring services. Many tutoring centers offer additional resources or can point you toward supplementary exercises that match your level.
By diversifying your practice and seeking out additional materials, you can strengthen your understanding and develop a well-rounded skill set. With consistent effort and the right resources, success becomes more attainable.
Key Tips for Success in Mathematics
Achieving success in any subject requires a combination of effective strategies, consistent practice, and a positive mindset. In mathematics, this is especially true, as it demands both conceptual understanding and problem-solving skills. The following tips can help build a strong foundation, improve performance, and foster confidence when tackling challenges.
1. Build Strong Foundations
Understanding core concepts is essential for tackling more complex problems. Take the time to fully grasp basic principles, as they often serve as the building blocks for more advanced topics. If something seems unclear, revisit it until you feel confident in your understanding. Reinforcement of foundational knowledge will allow you to solve problems more efficiently.
2. Practice Regularly
Mathematical skills improve with consistent practice. Regularly working through problems helps solidify concepts, builds fluency, and enhances problem-solving techniques. Make a habit of solving exercises daily or weekly, depending on your schedule. It’s important to challenge yourself with different types of problems to develop versatility in your skills.
- Work on a Variety of Problems: Different types of problems help you apply concepts in different ways. This improves both flexibility and adaptability.
- Focus on Mistakes: Don’t be discouraged by errors. Mistakes are an opportunity to learn and refine your understanding.
By focusing on mastering the basics and practicing regularly, you’ll develop the skills needed to succeed. Keep a positive attitude, and remember that persistence is key to overcoming challenges.
Understanding Different Levels of Mathematics
Mathematics is a broad subject that spans a variety of topics, each with its own complexity and depth. Understanding the different levels of mathematical study is essential for students as they progress through their education. From basic arithmetic to advanced calculus, each level builds on previous knowledge and introduces new challenges. Identifying where you are in your learning journey can help you focus your efforts more effectively and tackle new concepts with confidence.
Below is an overview of common levels within the subject, each representing a different stage in the learning process:
| Level | Description |
|---|---|
| Elementary Concepts | Includes basic arithmetic operations, such as addition, subtraction, multiplication, and division, as well as an introduction to geometry and basic problem-solving strategies. |
| Pre-Algebra | Focuses on understanding variables, equations, and simple functions. Introduces concepts like exponents, fractions, and negative numbers. |
| Algebra | Explores the manipulation of algebraic expressions, solving equations, graphing functions, and understanding polynomials, inequalities, and systems of equations. |
| Geometry | Examines shapes, angles, areas, volumes, and the properties of space. Teaches how to use deductive reasoning to prove geometric theorems. |
| Trigonometry | Deals with the relationships between angles and sides of triangles. Introduces trigonometric functions like sine, cosine, and tangent. |
| Calculus | Focuses on the study of change and motion, including differentiation, integration, and limits. It also covers concepts like sequences and series. |
| Advanced Topics | Includes subjects like linear algebra, differential equations, and complex analysis. These areas are often studied at higher education levels. |
By understanding these levels, students can better navigate their curriculum, seek the necessary support, and know when to advance to more challenging material. It’s important to recognize that mathematics is a progressive field, and each level lays the groundwork for the next. Whether you’re just starting or exploring advanced topics, developing a strong understanding at each stage is key to long-term success.
Troubleshooting Common Mistakes
Errors are a natural part of the learning process, especially when it comes to solving complex problems. Identifying and correcting mistakes early on can significantly improve understanding and mastery of the material. This section highlights some common mistakes that students often encounter, along with strategies to avoid or fix them.
Common Errors in Problem Solving
Students frequently make several types of mistakes that can lead to incorrect solutions. Recognizing these errors can help you become more precise and efficient when working through problems.
- Misunderstanding the Question: Sometimes, students misinterpret what a problem is asking, leading them to solve the wrong equation or use the wrong method. It’s important to carefully read the problem and identify the key information before starting.
- Incorrect Application of Formulas: Many problems require specific formulas. Applying the wrong formula or miscalculating constants can lead to mistakes. Review formulas regularly to ensure you’re using the correct one for each problem.
- Sign Errors: Neglecting to account for negative signs is a common issue, especially when dealing with subtraction, negative numbers, or fractions. Double-check each operation to avoid this mistake.
- Skipping Steps: Rushing through problems can cause important steps to be missed, such as simplifying expressions or checking intermediate results. Take your time and work through each step methodically.
How to Fix These Mistakes

While mistakes are inevitable, knowing how to correct them is essential for improvement. Here are some techniques for troubleshooting and addressing errors:
- Review Your Work: Always go back and check your calculations. If your final answer doesn’t make sense, retrace your steps to identify where the error occurred.
- Seek Clarification: If you’re unsure about the problem or a specific concept, don’t hesitate to ask for help from a teacher, tutor, or peer.
- Practice Regularly: The more you practice, the more familiar you become with common pitfalls. This helps reduce mistakes over time and improves problem-solving accuracy.
- Break Down Complex Problems: If a problem seems overwhelming, break it down into smaller, more manageable parts. This approach can help you spot any errors along the way and solve the problem more effectively.
By being aware of these common mistakes and using these troubleshooting techniques, you can avoid many common pitfalls and improve your problem-solving skills. Remember, consistency and attention to detail are key to mastering any concept.