
The second section of your studies focuses on essential principles that help you understand how to approach various scientific tasks. It emphasizes the importance of using precise tools and methods to achieve reliable results, which is crucial in any field of research or experimentation. By mastering these foundational skills, you’ll be able to tackle a wide range of problems with confidence.
In this section, you will learn how to apply different strategies for interpreting data and solving complex questions. It involves exploring various techniques that enhance your ability to handle numbers and figures correctly. Whether you’re working with large or small values, developing an understanding of these techniques will ensure you have the necessary skills to analyze information accurately.
Efficiency and precision are the cornerstones of this section, as they allow you to understand how to convert units, handle measurements, and assess the validity of your results. Through practice and application, you will become proficient in processing scientific information, which will serve as a solid base for future learning and problem-solving tasks.
Overview of Chemistry Chapter 2
This section introduces fundamental concepts that are essential for understanding the tools and methods used to analyze scientific data. It covers the techniques that form the basis of accurate problem-solving, emphasizing how to obtain, interpret, and manipulate information effectively. These core principles are crucial for both theoretical understanding and practical application in the lab.
Throughout this unit, you’ll engage with a variety of approaches that ensure precision when working with values. The focus is on equipping students with the ability to handle different types of data correctly, from simple conversions to complex numerical relationships. Mastering these skills is a key step in advancing scientific knowledge and tackling challenges in a structured way.
The main goal of this section is to lay the groundwork for future learning. By honing your ability to work with data, you’ll develop a solid foundation that supports more advanced topics. This area of study is designed to build confidence in your problem-solving abilities, preparing you for more intricate experiments and theoretical exploration ahead.
Understanding Measurements in Chemistry
In any scientific field, the ability to accurately quantify properties is essential. This section focuses on the different techniques used to collect data, emphasizing how numbers are obtained, recorded, and interpreted in various experiments. Whether it’s the mass of a substance or the volume of a liquid, accurate quantification forms the backbone of reliable scientific inquiry.
Types of Quantities
There are various quantities in science, each requiring specific tools and methods to measure them effectively. These can be categorized into:
- Length – The measurement of distance between two points.
- Mass – The amount of matter in an object, often measured in grams or kilograms.
- Volume – The space occupied by a substance, usually measured in liters or cubic centimeters.
- Temperature – The degree of heat in a substance, typically measured in Celsius or Kelvin.
Tools for Accurate Data Collection
Different types of instruments are used to capture the needed data, each offering varying degrees of precision:
- Balance scales – Used for determining mass.
- Graduated cylinders – Commonly used for measuring the volume of liquids.
- Thermometers – Used for recording temperature in various experiments.
- Micrometers – Specialized instruments used to measure small distances or thicknesses with great precision.
By understanding the tools available and the appropriate methods for each type of data, students can ensure that their work is both accurate and reliable. This foundational knowledge will allow for the correct interpretation of experimental results, which is essential in scientific exploration.
Importance of Accuracy in Scientific Calculations

In any field of scientific inquiry, precision is essential to ensure the reliability of results. Even small errors can lead to significant discrepancies, affecting the conclusions drawn from an experiment. Whether you are analyzing data, predicting outcomes, or conducting experiments, maintaining accuracy is crucial for the validity of your work.
Accurate results are fundamental to understanding the relationships between variables and making informed decisions based on data. When values are not recorded or processed correctly, the entire analysis can be compromised. This can lead to incorrect interpretations, flawed predictions, or even failures in practical applications.
Ensuring precision in every step of the process, from initial observations to final computations, allows for consistent outcomes. This reliability helps to build confidence in the findings, making them useful for further research or real-world applications. Without accuracy, even the most advanced methods become ineffective, making the mastery of precise techniques a cornerstone of scientific work.
Types of Measurement Units Used
In scientific practice, various units are employed to quantify different physical properties. These units provide a standardized way to express the results of observations, ensuring consistency and comparability across studies. Understanding the appropriate unit for each type of quantity is fundamental for accurate data analysis and communication of results.
The International System of Units (SI) is the most commonly used system, offering a consistent framework for expressing different properties. However, depending on the context, some specialized units may be used to ensure precision and clarity.
Common Units include:
- Length: Meters (m), centimeters (cm), millimeters (mm), kilometers (km).
- Mass: Kilograms (kg), grams (g), milligrams (mg).
- Volume: Liters (L), milliliters (mL), cubic centimeters (cm³).
- Temperature: Kelvin (K), Celsius (°C), Fahrenheit (°F).
- Time: Seconds (s), minutes (min), hours (h).
In some cases, specific fields may use additional or alternative units for greater precision or convenience. For instance, in physics, the mole (mol) is often used to measure the amount of substance, while in electrical studies, amperes (A) are used for current. Understanding the units required for different types of experiments helps ensure that results are both accurate and meaningful.
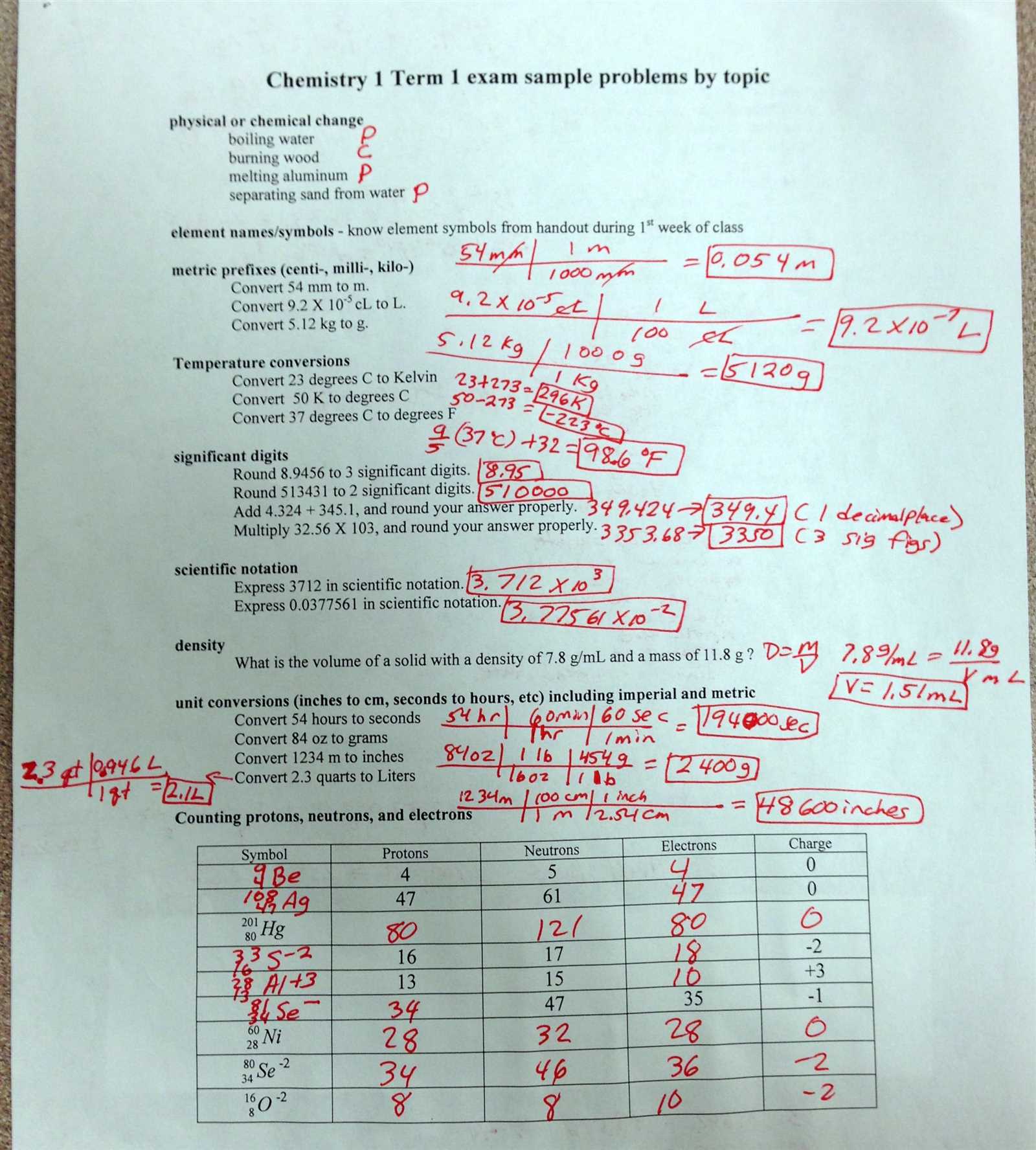
Conversion of Units in Chemistry

The ability to convert between different units is a fundamental skill in scientific work. Often, values are provided in one unit but need to be expressed in another for consistency or practical reasons. Mastering unit conversion ensures accurate comparisons, calculations, and the ability to communicate findings effectively.
Why Unit Conversion is Important

Unit conversion allows scientists to standardize data from different sources or measurements. In many experiments, values may be recorded in a variety of units depending on the tools and systems used. Being able to convert these values accurately ensures that all data can be integrated and analyzed together.
Common Conversion Methods
There are various methods to convert one unit to another, with the most common being the use of conversion factors. A conversion factor is a ratio that expresses the relationship between two units. For example:
- 1 kilometer (km) = 1000 meters (m)
- 1 liter (L) = 1000 milliliters (mL)
- 1 kilogram (kg) = 1000 grams (g)
By multiplying a given value by the appropriate conversion factor, you can easily transform the value from one unit to another. For complex conversions, dimensional analysis is often used to break down the problem step by step, ensuring accuracy at each stage.
Understanding how to convert units correctly is essential for maintaining precision in scientific experiments. It guarantees that all measurements align with the required standards and that the conclusions drawn from them are valid.
Significant Figures and Their Role
In scientific work, precision is crucial when presenting numerical data. One of the key aspects of maintaining precision is understanding how to properly represent values with the correct number of significant digits. These figures reflect the accuracy of the instruments used and ensure that results are reported in a way that conveys the correct level of certainty.
Significant figures are the digits in a number that carry meaningful information about its precision. This includes all non-zero digits, any zeros between significant digits, and any trailing zeros in the decimal portion of the number. However, leading zeros are not considered significant as they are merely placeholders.
Some basic rules for identifying significant figures include:
- Non-zero digits are always significant. For example, in the number 345.6, all four digits are significant.
- Any zeros between significant digits are also significant. For example, in 5003, all four digits are significant.
- Leading zeros are not significant. For example, in 0.0045, only the digits 4 and 5 are significant.
- Trailing zeros in a decimal number are significant. For example, in 45.00, all four digits are significant.
- Trailing zeros in a whole number without a decimal point are not considered significant. For example, 1500 has only two significant digits.
Understanding significant figures is essential for maintaining the integrity of scientific data. By adhering to these rules, you ensure that the reported values accurately reflect the precision of the measurement process and avoid overstating the reliability of results.
Scientific Notation for Large Numbers
Handling extremely large numbers can be cumbersome, especially when dealing with values that stretch over many zeros. To simplify the representation of such numbers, scientific notation provides a compact and manageable way to express them. This method is widely used in various scientific fields to facilitate calculations and improve clarity.
Understanding the Format
Scientific notation expresses large values as a product of two parts: a decimal number between 1 and 10, and a power of 10. For example, the number 7,500,000 can be written as 7.5 × 106. Here, 7.5 is the significant figure, and 106 indicates how many places the decimal point is moved.
Benefits of Scientific Notation
Using this system has several advantages:
- Compactness – Large numbers are condensed into a much shorter form, making them easier to write and read.
- Precision – The notation highlights the significant figures, ensuring that precision is maintained.
- Ease of calculation – Operations such as multiplication and division become simpler when dealing with powers of 10.
Scientific notation helps reduce the complexity of working with large numbers, making them more accessible for analysis and computation in various scientific contexts.
Dimensional Analysis in Problem Solving
When solving complex problems involving different units, dimensional analysis serves as a powerful tool to ensure consistency and correctness in your calculations. This method helps in converting units, verifying equations, and identifying errors in the process. By focusing on the dimensions or units of the quantities involved, it becomes easier to manipulate the values and solve for unknowns.
Dimensional analysis simplifies multi-step problems by breaking them down into manageable parts. It ensures that units cancel out correctly, allowing you to focus on the numerical relationships between quantities. This approach is particularly useful in fields that require precise conversions or when dealing with unfamiliar units.
Steps in Dimensional Analysis
Here are the general steps for applying dimensional analysis to a problem:
- Identify known and unknown quantities: Write down the values you are given and the unit you need to convert or calculate.
- Set up conversion factors: Use ratios between units to create conversion factors that will cancel out unwanted units.
- Multiply by conversion factors: Apply the conversion factors in a way that ensures the correct cancellation of units.
- Simplify and solve: After canceling units, perform the calculations to obtain the final answer in the desired units.
Examples of Applications
Dimensional analysis is widely applied in various fields, including:
- Unit conversion, such as converting miles to kilometers or grams to pounds.
- Verifying the correctness of equations in scientific formulas.
- Solving complex problems in physics or engineering involving different systems of units.
By mastering dimensional analysis, you can ensure that your solutions are accurate, consistent, and logical, regardless of the complexity of the problem at hand.
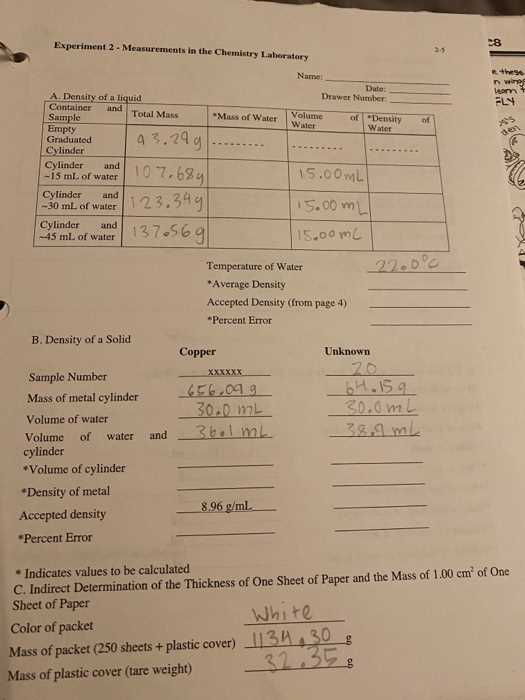
Basic Principles of Volume and Mass
Understanding the fundamental concepts of volume and mass is essential for solving a wide range of scientific problems. These two quantities play a crucial role in various experiments and calculations, helping to characterize substances and their properties. Volume describes the amount of space an object occupies, while mass refers to the amount of matter it contains.
The relationship between mass and volume allows for a deeper understanding of materials. For example, when substances are combined or manipulated, changes in these two properties can offer insights into chemical reactions, density, and the nature of the substances involved. Accurately determining both mass and volume is key to achieving reliable results in many scientific processes.
Measuring Volume
Volume is typically measured using a variety of instruments, depending on the size and shape of the object. Common methods include:
- Liquid Volume: Measured using graduated cylinders, pipettes, or burettes. Liquids conform to the shape of their container, making it easy to assess their volume accurately.
- Regular Objects: For objects with uniform shapes, volume can be calculated using mathematical formulas, such as length × width × height for rectangular solids.
- Irregular Objects: For irregular shapes, volume is often determined by displacement, where the object is submerged in water and the change in water level indicates the object’s volume.
Measuring Mass
Mass is measured using scales or balances. It is important to note that mass is constant regardless of location, unlike weight, which can change due to gravitational forces. Key instruments used for measuring mass include:
- Analytical Balance: Used to measure mass with high precision, often in laboratory settings.
- Triple Beam Balance: A traditional balance used for determining mass with moderate accuracy.
- Digital Scales: Often used for general purposes, providing quick and convenient measurements of mass.
The accurate measurement of both volume and mass is essential in determining other properties such as density, and plays a vital role in conducting precise scientific experiments.
Common Calculation Errors to Avoid
In scientific problem-solving, small mistakes can lead to incorrect results. These errors, often overlooked, can have significant impacts on the accuracy of your work. Being mindful of common pitfalls can help ensure that your results are both precise and reliable. Identifying these frequent mistakes is crucial for improving your overall approach to solving problems.
Common Mistakes in Problem Solving
Below are some of the most frequent errors people make when performing scientific computations:
| Error | Description | How to Avoid |
|---|---|---|
| Incorrect Unit Conversion | Misunderstanding or forgetting to convert between units before performing operations can lead to inaccurate results. | Always double-check unit conversions and ensure you’re using the correct factors. |
| Rounding Too Early | Rounding intermediate values before the final answer can introduce significant error. | Round only at the final step of the problem to minimize error. |
| Incorrect Use of Significant Figures | Improper handling of significant figures can lead to results that are more precise than the data allows. | Apply the rules of significant figures consistently throughout the problem-solving process. |
| Forgetting to Include All Variables | Omitting important variables or constants in equations can distort results. | Review the formula and ensure that all required variables are included in the calculation. |
| Incorrect Order of Operations | Failing to follow the correct order of operations can lead to inaccurate results. | Follow the proper sequence of operations (PEMDAS) to ensure the accuracy of your solution. |
Double-Checking Work
One of the most effective ways to avoid errors is through thorough review. After completing a problem, revisit each step, check the unit conversions, and verify the accuracy of your intermediate steps. Taking a few extra moments to double-check your work can prevent costly mistakes and improve your overall reliability in solving complex scientific problems.
Estimating Uncertainty in Measurements
In any scientific process, it’s essential to recognize that no value is ever perfectly accurate due to inherent limitations in the tools used and the environment in which experiments occur. Understanding the degree of uncertainty helps in evaluating the reliability of the results. Every observation carries a margin of error, which should be considered when reporting findings.
Uncertainty arises from multiple factors, including the precision of instruments, the method of data collection, and the skill of the person conducting the experiment. Estimating this uncertainty helps in providing a more realistic range for your results, rather than reporting a single, seemingly precise value. Here, we explore how to quantify and express uncertainty in scientific work.
| Source of Uncertainty | Description | How to Minimize |
|---|---|---|
| Instrumental Error | Errors caused by the limitations of the measuring device, such as calibration issues or inherent precision limits. | Use more accurate instruments or calibrate them properly before use. |
| Human Error | Errors caused by the person taking measurements, including misreading instruments or incorrect data recording. | Ensure consistent technique and practice, and double-check readings. |
| Environmental Factors | External factors such as temperature, humidity, or vibrations that can affect results. | Control environmental conditions as much as possible during experiments. |
| Sampling Error | Variations caused by the choice or amount of sample used in the experiment. | Use representative samples and repeat measurements to minimize random errors. |
To properly convey uncertainty, the value should typically be expressed along with its possible range. For example, reporting a length as 10.2 cm ± 0.1 cm indicates the degree of potential error, showing that the actual value lies somewhere between 10.1 cm and 10.3 cm. This approach provides a clearer understanding of the reliability of the measurement and allows for more informed conclusions to be drawn from the data.
Density and Its Application in Chemistry
The concept of density plays a fundamental role in scientific analysis, as it relates the mass of a substance to its volume. It is a crucial property that helps to characterize materials, providing valuable insights into their composition and behavior. Understanding how density influences interactions between substances is vital across numerous scientific fields, especially in processes involving matter’s physical properties.
In practical terms, density allows scientists to distinguish between substances and predict how different materials will behave under various conditions. For example, it helps explain why certain materials float on water while others sink. By measuring this property, chemists can determine the purity of a substance, its concentration in solutions, or even identify substances based on known density values.
Applications in Material Science
In material science, density is often used to evaluate the suitability of materials for specific applications. For example, metals with a higher density are often used for construction and manufacturing purposes due to their strength and durability. Conversely, lightweight materials with lower densities are preferred for products where weight is a critical factor, such as in the aerospace industry.
Density in Solutions and Mixtures

Density also plays a significant role when working with solutions or mixtures. When substances dissolve or combine, their densities change, affecting their behavior. This is particularly important in fields like pharmaceuticals, where understanding the density of drug formulations helps ensure proper dosage and effectiveness. In environmental science, knowing the density of pollutants can also help determine their movement and dispersion in water or air.
How to Interpret Answer Keys
When working with problem-solving exercises, it is essential to understand how to properly interpret the solutions provided. Answer guides are valuable tools that offer not just the final result, but also the method used to arrive at that result. Understanding the reasoning behind each step allows learners to develop their problem-solving skills, helping them identify where they may have made an error or missed a key concept.
To effectively use an answer guide, start by reading through the explanation of each step. Check the formulas or techniques applied and see if they align with what you’ve learned. Sometimes, seeing the process in action provides clarity on how to apply similar approaches in other problems. Additionally, it’s crucial to note any assumptions made or specific conditions under which the steps are valid. This can help prevent misunderstandings when applying the knowledge to different situations.
Key Components in Answer Keys
Answer keys often contain several critical elements that aid in understanding how a solution was reached:
- Step-by-step breakdown: Provides a detailed process of how the solution is derived.
- Explanations: Clarifies the logic behind each step, offering insight into the methodology.
- Formula references: Highlights which formulas are used and how they are applied in the context of the problem.
- Units of measurement: Ensures that the correct units are maintained throughout the solution, emphasizing the importance of consistency.
Using the Answer Key for Self-Assessment
After reviewing the solution guide, it’s beneficial to compare it with your own work. Ask yourself the following questions:
- Did I follow a similar approach?
- Were there any steps I missed or misunderstood?
- Did I use the correct formulas and units?
- How did the final result compare to mine?
By engaging with the answer guide in this way, you can deepen your understanding of the concepts and improve your ability to tackle similar problems in the future.
| Step | Explanation | Formula Used |
|---|---|---|
| Step 1 | Identify the given values and what needs to be solved | None |
| Step 2 | Apply the appropriate equation for solving the problem | Formula X |
| Step 3 | Substitute values and solve for the unknown | Formula X |
Practice Problems for Chemistry Review
Working through practice exercises is an essential part of mastering the fundamental principles in any scientific field. By tackling various problems, learners can apply the concepts they have studied, reinforcing their understanding while also identifying areas that need further attention. These exercises help solidify theoretical knowledge and prepare individuals for real-world applications.
Below are several problems designed to help you test your knowledge and improve your problem-solving skills. Each problem challenges you to apply different concepts, so make sure to read them carefully and approach each step methodically.
Practice Problems
- Problem 1: Convert 0.75 kilometers to meters.
- Hint: Remember the conversion factor between kilometers and meters.
- Problem 2: Calculate the volume of a cube with sides measuring 5 cm each.
- Hint: Use the formula for the volume of a cube, which is side³.
- Problem 3: Determine the density of a substance that has a mass of 12 grams and a volume of 4 cm³.
- Hint: The formula for density is mass ÷ volume.
- Problem 4: Convert 250 milliliters to liters.
- Hint: Use the correct conversion factor between milliliters and liters.
- Problem 5: Calculate the total distance traveled by an object moving at 10 meters per second for 2 hours.
- Hint: Distance = speed × time.
Steps to Approach Each Problem
- Identify the known values in the problem and what you need to solve for.
- Write down any relevant formulas or conversion factors that apply to the situation.
- Substitute the known values into the equations.
- Perform the necessary calculations, paying attention to units and significant figures.
- Check your answer to ensure it makes sense based on the given information.
By practicing regularly, you will become more comfortable with these types of problems and develop the skills needed to tackle more complex scenarios. Keep honing your abilities to ensure a strong foundation for future topics.
Tips for Efficient Study Techniques

Effective studying requires more than just reading through textbooks or notes; it involves using strategies that maximize retention, understanding, and application of the material. By adopting the right approach, learners can enhance their focus, manage their time efficiently, and retain complex information with ease. These strategies not only make learning more productive but also help reduce stress and improve overall performance.
Below are some proven techniques that can help you study more effectively and make the most out of your study sessions.
Active Learning Methods
Active learning techniques are designed to keep you engaged with the material, rather than passively reading or listening. These methods encourage critical thinking and allow you to actively apply what you’ve learned.
- Practice problems: Solving problems helps reinforce the material and identify areas that need more focus.
- Teach someone else: Explaining concepts to another person solidifies your understanding and exposes any gaps in knowledge.
- Summarize key concepts: After studying, write down the main ideas in your own words to ensure you’ve grasped the material.
Time Management Tips
Managing your time wisely during study sessions can increase efficiency and ensure you’re covering all the necessary topics without feeling overwhelmed.
- Set specific goals: Break down larger topics into smaller, manageable sections and focus on one at a time.
- Use the Pomodoro technique: Study in short, focused intervals (e.g., 25 minutes) followed by a brief break to maintain concentration.
- Prioritize difficult topics: Tackle challenging material first when your mind is fresh, and save easier topics for later.
By incorporating these strategies into your routine, you will be able to approach your studies in a more organized and efficient manner, leading to better comprehension and improved retention over time.
Using Online Resources for Learning
In today’s digital age, the internet provides an abundance of tools that can enhance the learning experience. With the right resources, students can access a wealth of information, interact with experts, and engage in various activities that deepen their understanding of complex subjects. These resources range from instructional videos to interactive simulations, offering diverse ways to grasp challenging concepts.
Online platforms can cater to different learning styles, whether you prefer visual aids, step-by-step tutorials, or community-driven discussions. By incorporating these resources into your study routine, you can strengthen your knowledge, practice more effectively, and gain immediate feedback to guide your progress.
- Educational Websites: Websites dedicated to providing structured lessons, practice exercises, and quizzes allow learners to explore topics in-depth at their own pace.
- Interactive Simulations: Platforms offering virtual labs or interactive activities can bring theoretical concepts to life, making them more engaging and understandable.
- YouTube Channels: Many educational channels provide free tutorials, problem-solving tips, and explanations that can help clarify difficult material.
- Online Forums: Community-driven platforms allow students to discuss topics, ask questions, and collaborate with peers and instructors to better grasp concepts.
By leveraging these online tools, learners can supplement traditional studying methods, stay motivated, and tackle even the most difficult subjects with confidence.
Preparing for Exams Effectively
Effective preparation is crucial for success in any exam. To ensure you approach your study sessions with the right strategies, it’s important to understand the material thoroughly and practice problem-solving techniques. Organizing your study time and using targeted methods can help you retain key information and perform confidently during your assessments.
Start by breaking down the topics into smaller, manageable sections. Focus on understanding fundamental principles before advancing to more complex content. Additionally, practicing with sample questions can help familiarize you with the exam format and identify areas that need further review.
Key Study Strategies
- Review Past Tests: Going over previous exams or practice tests can provide valuable insight into the types of questions likely to appear and help you identify any knowledge gaps.
- Active Recall: Instead of passively reading notes, test yourself regularly to enhance memory retention. This can be done through flashcards, self-quizzes, or explaining concepts out loud.
- Group Study: Collaborating with peers can be effective for discussing difficult topics and gaining different perspectives. Teaching each other reinforces your understanding.
- Stay Organized: Develop a study schedule that allocates time for each subject area. Consistent, focused study sessions yield better results than cramming the night before.
Time Management Tips
| Time Management Strategy | Benefits |
|---|---|
| Pomodoro Technique | Breaks study time into focused intervals with short breaks in between, improving focus and preventing burnout. |
| Prioritize Difficult Topics | Focus on the areas that are most challenging first, when your concentration is highest. |
| Set Realistic Goals | Having clear, achievable objectives for each study session helps keep you on track and motivated. |
By incorporating these study methods into your routine, you will be better prepared for exams and increase your chances of achieving outstanding results.