
Mastering complex concepts and solving challenging problems is key to excelling in any mathematics assessment. Whether you’re facing problems with shapes, figures, or calculations, understanding the methods to approach each question is crucial for success. By identifying patterns and applying the right strategies, you can significantly improve your results.
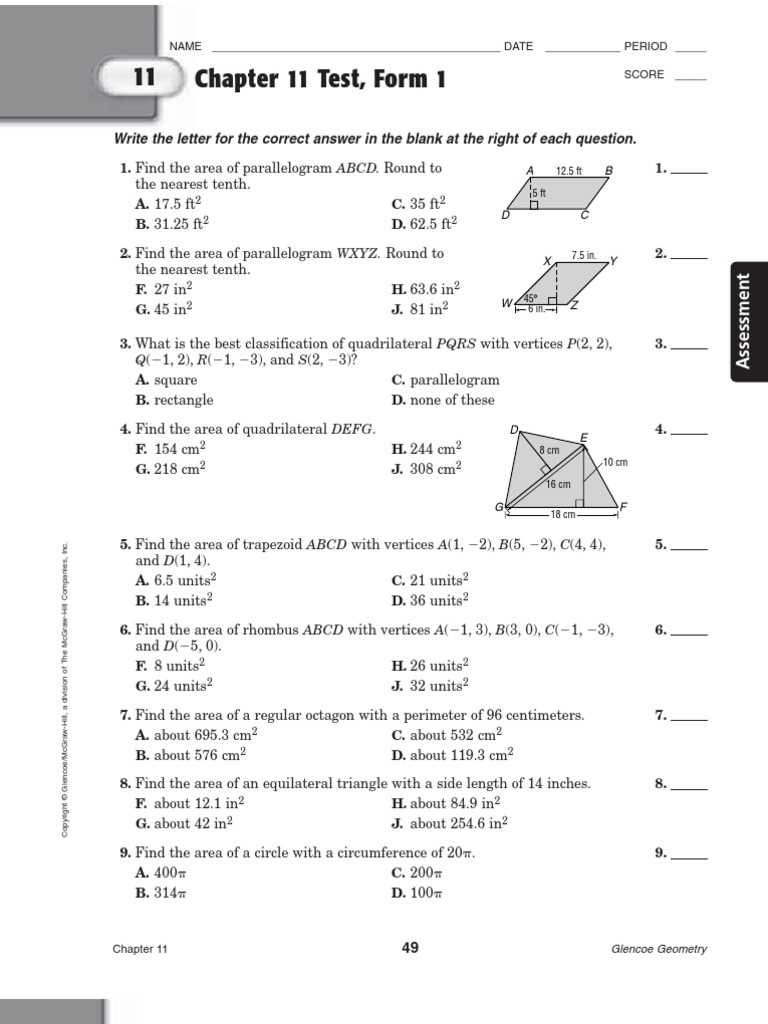
When preparing for these types of assessments, it’s important to familiarize yourself with the structure of the questions and the logical steps required to reach the correct solutions. Reviewing detailed solutions can help you understand the thought process behind each step, enhancing your problem-solving skills and boosting confidence.
Knowing how to check your work efficiently and recognize common pitfalls can make all the difference when it comes to scoring well. In this guide, you’ll find valuable insights on how to break down difficult problems and apply tested methods that will improve your overall performance.
Geometry Exam Answer Key Insights

Understanding the solutions to mathematical problems is essential for mastering any test. Knowing the correct approach to each question helps not only in identifying the right answer but also in grasping the underlying concepts. With a clear grasp of problem-solving techniques, you’ll be able to solve even the most complex tasks with confidence and accuracy.
When reviewing solutions, it’s important to focus on the process rather than just the final results. Breaking down each step allows you to see how certain methods or formulas are applied to reach the correct outcome. By internalizing these strategies, you can improve your ability to approach similar questions on future tests.
Being able to spot common errors and understanding where things might go wrong is just as important. The key to success lies not only in finding the right answer but also in avoiding common pitfalls. This insight will help you become more efficient and accurate in solving problems under time pressure.
Understanding Key Geometry Exam Topics
To perform well in any mathematics assessment, it’s essential to grasp the fundamental concepts that often appear in the questions. These core topics provide the foundation for solving a wide range of problems. Knowing which areas are frequently tested allows you to prioritize your study efforts and focus on the most important material.
Commonly Tested Concepts

Many tests cover a range of mathematical areas, such as shapes, angles, and spatial relationships. Understanding how to calculate areas, perimeters, volumes, and angles can significantly improve your ability to solve problems quickly and accurately. Additionally, recognizing patterns and how they relate to different figures is an important skill to develop.
Approaching Complex Problems
Once you have mastered the basic concepts, the next step is applying that knowledge to more complex tasks. This involves recognizing the specific approach required for each problem, whether it involves algebraic manipulation, applying theorems, or drawing accurate diagrams. Practice is key to mastering these techniques and becoming comfortable with various problem types.
How to Prepare for Geometry Exams
Effective preparation is the cornerstone of success in any mathematical test. To ensure you approach the assessment with confidence, you need a structured plan that focuses on mastering essential concepts and practicing key problem-solving techniques. The more prepared you are, the easier it will be to navigate through challenging questions.
Begin by reviewing the main topics that are frequently tested, such as properties of shapes, measurement formulas, and theorems. After that, it’s crucial to practice solving a variety of problems, focusing on both speed and accuracy. This will help you become comfortable with different question formats and identify the most efficient methods for solving them.
| Preparation Step | Focus Area | Recommended Activity |
|---|---|---|
| Review Key Topics | Shapes, angles, volume, and area formulas | Read textbooks and notes |
| Practice Problems | Solving various problem types | Work through sample questions |
| Mock Tests | Simulating the actual test environment | Take timed practice tests |
| Self-Assessment | Identifying areas for improvement | Review errors and refine strategies |
Lastly, don’t forget to revisit any mistakes or challenging problems. Analyzing your errors and understanding why certain approaches didn’t work will help you avoid similar pitfalls during the actual test.
Common Mistakes in Geometry Exams
When tackling mathematical assessments, it’s easy to make errors that can cost valuable points. These mistakes are often the result of overlooking key details or misapplying formulas. By recognizing the most common pitfalls, you can avoid them and increase your chances of achieving a high score.
One of the frequent errors is misinterpreting the problem. Sometimes, a question may involve a specific condition or restriction that requires careful attention. Failing to understand these nuances can lead to incorrect assumptions and ultimately, wrong answers.
Another common mistake is rushing through calculations without double-checking. Simple arithmetic errors can easily occur under time pressure, and they can significantly affect the final result. Always take a moment to verify your calculations before submitting your work.
Additionally, many students struggle with applying the correct formulas in the right context. While certain formulas are used for specific types of problems, using the wrong one can lead to completely inaccurate results. It’s crucial to understand when and how to apply each formula to avoid this mistake.
Breaking Down Geometry Exam Questions
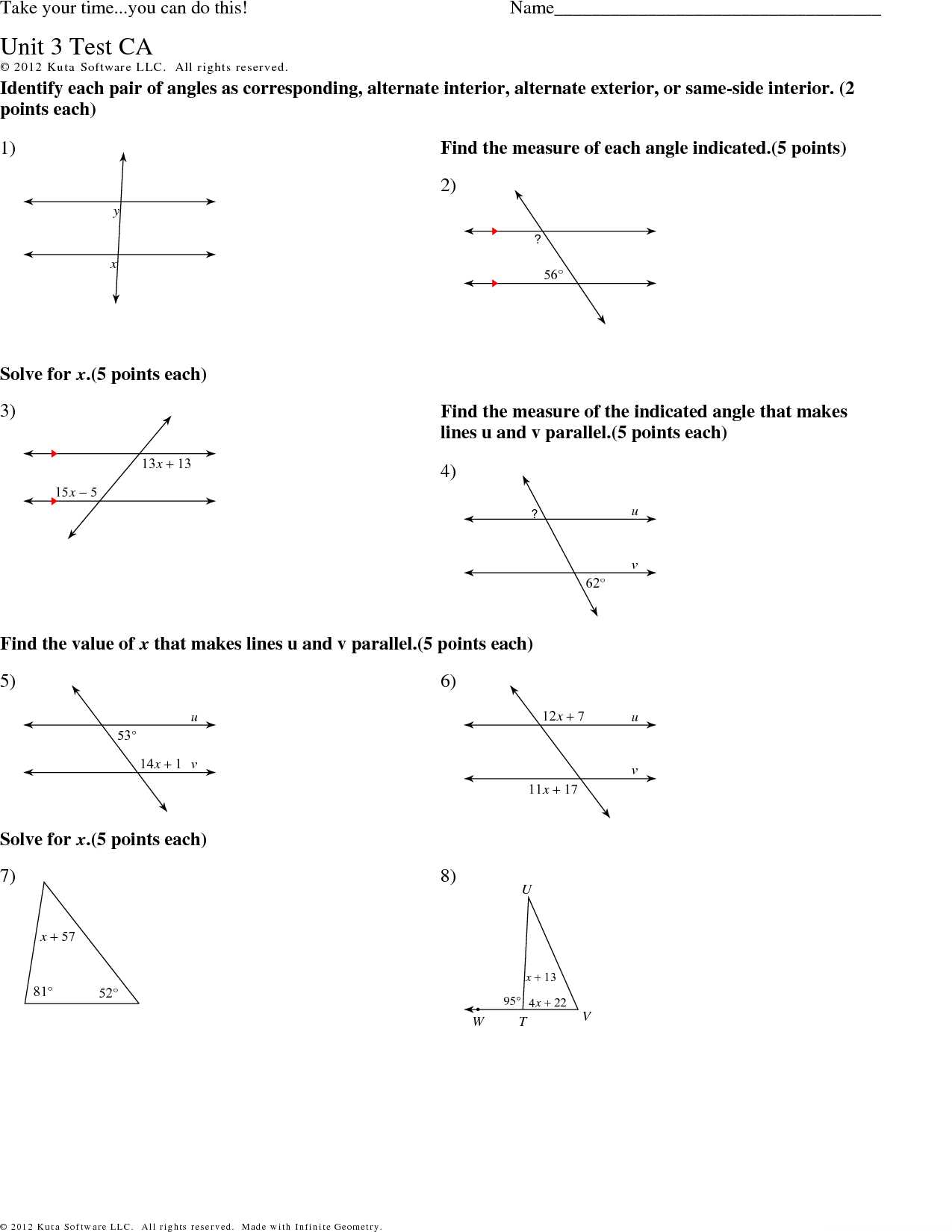
Approaching a mathematical problem can often seem overwhelming, especially under time pressure. However, breaking down each question into smaller, manageable parts can simplify the process and make it easier to find a solution. Understanding the structure of a problem and identifying the key elements is the first step toward solving it accurately.
The first thing to do is carefully read through the problem to understand what is being asked. Look for any important information or constraints, such as specific measurements, relationships between shapes, or conditions that must be met. Highlighting or underlining these key details can help focus your attention on what matters most.
Next, consider the relationships between the elements in the question. Often, problems involve multiple concepts that need to be connected. By identifying how different pieces of information relate to each other, you can determine the appropriate methods or formulas to apply.
Finally, break the problem into smaller steps. Tackle one part at a time, solving each section before moving on to the next. This systematic approach will not only make the problem feel less daunting but also reduce the likelihood of making careless errors.
Effective Time Management for Geometry Tests
Time management is a crucial skill when tackling any mathematical assessment. With limited time and a variety of problems to solve, it’s essential to approach the test with a strategic mindset. By managing your time effectively, you can ensure that each question gets the attention it deserves, and you avoid rushing through the most challenging parts.
Start by assessing the overall time you have and dividing it by the number of questions. This will give you a general idea of how long you should spend on each task. Keep in mind that some questions may take more time than others, so be prepared to adjust accordingly. Prioritize questions based on your strengths and the level of difficulty.
One effective strategy is to quickly skim through all the questions at the beginning of the test. This will allow you to identify the easier ones that you can solve quickly, giving you more time for the more complex problems. Tackle the straightforward questions first, and leave the more time-consuming ones for later.
Don’t forget to pace yourself throughout the test. Keep an eye on the clock, but avoid fixating on it. If you get stuck on a problem, move on and come back to it later. This will prevent you from wasting time on a single question and allow you to maximize your score across the entire test.
Geometry Formulas You Need to Know
When preparing for a mathematics assessment, it’s essential to be familiar with the key formulas that are frequently used in problem-solving. These formulas are the building blocks that help you find solutions to various types of problems, from calculating areas to determining volumes. Mastering these essential equations can save you time and reduce the chances of making errors during the test.
Basic Formulas for Shapes and Figures
Understanding the fundamental formulas for common shapes is crucial. From circles to triangles, knowing how to calculate perimeter, area, and other properties will allow you to solve problems quickly and accurately. Below are some of the key formulas you should know:
| Shape | Formula | Explanation |
|---|---|---|
| Circle | Area = πr² | Used to calculate the area of a circle, where r is the radius. |
| Rectangle | Area = l × w | Used to find the area of a rectangle, where l is the length and w is the width. |
| Triangle | Area = ½ × b × h | Used for calculating the area of a triangle, where b is the base and h is the height. |
| Square | Area = s² | Used for calculating the area of a square, where s is the length of a side. |
Advanced Formulas for Complex Problems
For more complex figures and multi-step problems, you may need to apply additional formulas, such as those for volume or surface area. Familiarizing yourself with these will help when you encounter three-dimensional shapes or need to calculate other advanced properties.
How to Check Your Geometry Exam Answers
Reviewing your work is an essential step before submitting any test, as it ensures that all calculations are accurate and that no mistakes have been overlooked. By following a systematic process, you can spot errors and make necessary corrections, ultimately improving your performance.
Start by reading through each question and its solution. Check if you have answered exactly what was asked, and ensure that you didn’t misinterpret the problem. A clear understanding of the question is crucial for getting the right results.
- Verify all formulas: Double-check that you applied the correct equations for each problem. Ensure that each formula is suitable for the specific type of problem you are solving.
- Recheck your calculations: Go over your arithmetic and algebraic steps carefully. Small errors in basic calculations can lead to incorrect results.
- Ensure proper units: Verify that your answers are in the correct units (e.g., centimeters, meters, square units) and that you haven’t missed any conversions.
After rechecking the formulas and calculations, it’s also helpful to verify your answers with logical reasoning. Ask yourself if the results make sense in the context of the problem.
- Look for consistency: Check if your results align with the visual or conceptual aspects of the question. For example, does the calculated area seem reasonable for the given dimensions?
- Test extreme cases: If possible, test your results using extreme or boundary values to ensure they hold true across different scenarios.
Finally, if time allows, it’s worth revisiting any challenging questions. Revising your approach can sometimes reveal overlooked details that could change your solution.
Importance of Geometry Practice Problems
Practicing a variety of problems is one of the most effective ways to solidify your understanding of mathematical concepts. Regularly solving exercises not only enhances your problem-solving skills but also helps you become more familiar with different types of challenges. The more you practice, the more confident and efficient you become in applying formulas and strategies to new situations.
Benefits of Solving Practice Problems
Working through practice problems provides several advantages that contribute to better performance. Here are some of the key benefits:
- Improves Conceptual Understanding: Repeated practice helps reinforce key principles, making it easier to recall and apply them when needed.
- Boosts Confidence: The more you practice, the more comfortable you become with different problem types, which increases your confidence in tackling similar questions during assessments.
- Identifies Weak Areas: Regular practice helps highlight areas where you may be struggling. Recognizing these weak points early allows for focused review and improvement.
How to Maximize the Benefits of Practice
To get the most out of your practice sessions, it’s important to approach them strategically. Here are some tips:
- Start with Simple Problems: Begin with basic exercises to build a solid foundation before moving on to more complex tasks.
- Vary Problem Types: Make sure to practice a wide range of problems, from simple calculations to more intricate geometric proofs or transformations. This helps you become versatile in your problem-solving approach.
- Time Yourself: Practice under timed conditions to simulate the real test environment. This will help you improve your speed and manage your time more effectively during assessments.
Ultimately, consistent practice is key to mastering the material and preparing for any challenges you may face during a test.
Tips for Solving Geometry Word Problems
Word problems can often seem intimidating, but with the right approach, they can be tackled efficiently. The key to solving these types of problems is to break them down into manageable parts and use logical reasoning. By following a systematic approach, you can translate the verbal information into mathematical equations and arrive at the correct solution.
Step-by-Step Approach

When faced with a word problem, it’s helpful to follow a structured method to ensure you don’t overlook any important details:
- Read Carefully: Start by reading the problem thoroughly to ensure you understand the given information and what is being asked. Highlight or underline key values and terms that might be important.
- Visualize the Problem: Try to draw a diagram or sketch of the situation described. Label all known values and shapes to help you see the relationships between different elements.
- Translate Words into Math: Convert the verbal information into mathematical expressions or equations. Look for phrases that indicate mathematical operations, such as “twice the length,” “sum of,” or “difference between.”
- Solve Step-by-Step: Once you’ve set up your equation, solve it step-by-step. Take your time to ensure each calculation is done correctly.
Common Pitfalls to Avoid
While solving word problems, it’s easy to make mistakes. Here are some common pitfalls and how to avoid them:
- Misinterpreting the Problem: Always double-check the wording to make sure you haven’t misunderstood the question. If something doesn’t make sense, re-read it and clarify.
- Overcomplicating the Problem: Keep things simple. Focus on what is given and what is being asked, and avoid unnecessary calculations or assumptions.
- Forgetting Units: Pay attention to the units given in the problem. Make sure you’re using the correct units for your final answer and converting when necessary.
By following these tips and practicing regularly, you’ll become more comfortable with word problems and develop a strategy for solving them effectively.
Mastering Geometry Diagrams in Exams
Diagrams are powerful tools that can help simplify complex problems and provide a visual understanding of relationships between different elements. By mastering how to interpret and use these visual aids, you can more easily grasp the underlying concepts and avoid common mistakes. Whether it’s a shape, a line, or an angle, a well-drawn diagram can offer crucial insights for solving a problem effectively.
The first step in utilizing diagrams during assessments is to always draw one if it’s not provided. A clear, precise sketch can often make abstract concepts much easier to understand. Even if a diagram is given, take the time to examine it carefully, labeling key points, lines, and angles. This step helps solidify the connections between the problem’s variables and ensures you don’t miss any important details.
- Highlight Important Information: Mark the given values on the diagram, such as lengths, angles, or any other numerical data that will be used in your calculations.
- Look for Symmetry and Patterns: Many problems rely on geometric properties such as symmetry or specific angle relationships. Identifying these patterns in the diagram can help you apply the right formulas and methods.
- Use Clear Labels: Label all key points, lines, and angles to avoid confusion later. This is particularly helpful when dealing with complex diagrams with multiple parts.
Remember that diagrams are not just for visualization–they are tools that can directly inform your approach to the solution. By practicing with different types of diagrams and familiarizing yourself with common geometric configurations, you can learn to use them efficiently to solve problems under exam conditions.
How to Study for Geometry Exams

Effective preparation is the cornerstone of success in any test, especially when it comes to mathematical subjects. A focused study plan that combines understanding fundamental concepts with regular practice can significantly improve performance. By using a strategic approach, you can tackle even the most challenging problems with confidence.
Start by reviewing all relevant concepts and formulas. Understanding the foundational principles, such as shapes, angles, and relationships, provides the necessary knowledge to approach complex problems. Use notes, textbooks, and online resources to reinforce your understanding of key concepts. It’s important to identify areas where you may need further clarification and revisit them until you feel comfortable.
- Practice Consistently: Solving problems regularly is essential. The more problems you work through, the better you will understand how to apply the concepts to real-world scenarios.
- Review Mistakes: When practicing, carefully review any mistakes you make. Understanding where you went wrong and why helps you avoid repeating the same errors in the future.
- Use Study Guides: Study guides or practice tests are useful tools to simulate test conditions. Time yourself while solving problems to get a sense of how much time you’ll need for each question during the actual test.
Finally, stay organized and manage your time effectively. Set aside regular study sessions, break down your study material into smaller sections, and focus on mastering one topic at a time. Consistency and discipline will yield great results when it’s time to sit for the test.
Essential Geometry Theorems for Success
Understanding key theorems is vital for solving mathematical problems efficiently. These fundamental principles serve as the building blocks for tackling complex questions. Mastering them allows you to apply logical reasoning and mathematical formulas to find the correct solutions quickly. A strong grasp of these concepts not only helps during practice but also boosts your confidence in tests.
Among the most important principles are those that deal with shapes, angles, and proportions. Whether you are calculating areas, solving for unknown angles, or proving relationships, knowing these theorems well enables you to approach problems systematically and with ease.
Important Theorems to Know
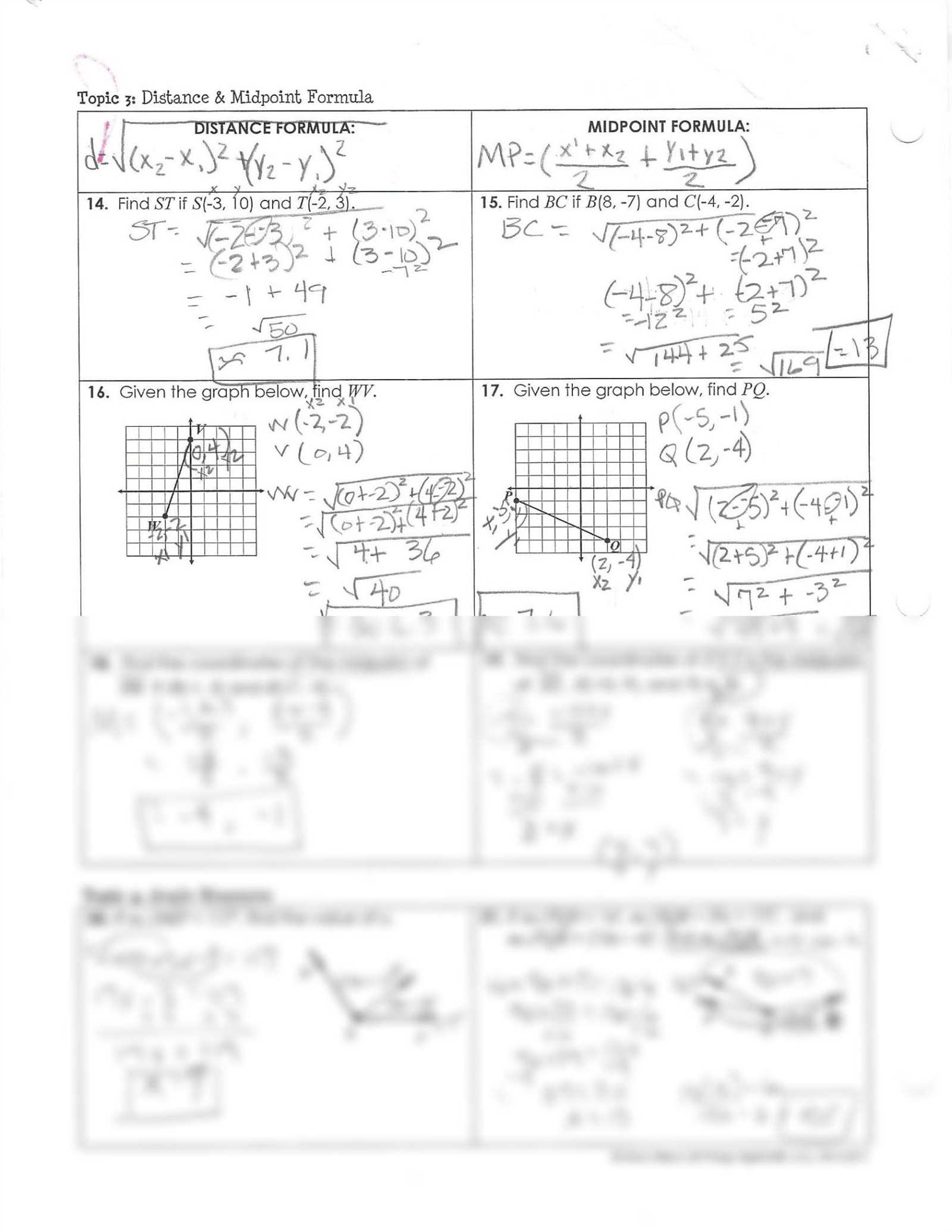
| Theorem | Application |
|---|---|
| Pythagorean Theorem | Used for finding the length of a side in right-angled triangles |
| Angle Sum Theorem | States that the sum of angles in a triangle is 180 degrees |
| Properties of Parallel Lines | Helps in determining angles when two parallel lines are intersected by a transversal |
| Similar Triangles Theorem | Used to find unknown sides or angles in triangles with proportional relationships |
Mastering these essential theorems provides a solid foundation for solving problems accurately. With practice, you can become adept at identifying when and how to apply each principle, leading to more efficient problem-solving and better results.
Geometry Problem-Solving Strategies
Effective problem-solving requires a structured approach that breaks down complex questions into manageable parts. By following specific strategies, you can streamline your thinking process, identify patterns, and arrive at solutions more efficiently. These techniques not only help improve accuracy but also save valuable time during assessments.
The key to success lies in understanding the structure of the problem, choosing the right methods, and applying logical reasoning at every step. Whether you’re working with angles, shapes, or algebraic equations, using a step-by-step approach can help clarify even the most complicated scenarios.
Key Strategies for Success
- Understand the Problem: Carefully read the problem to identify all knowns and unknowns. This helps in choosing the correct approach to solving it.
- Draw Diagrams: Visual representations make it easier to understand relationships between elements in the problem and can reveal helpful information.
- Work Backwards: In some cases, starting from the desired outcome and working backwards can help identify missing steps.
- Check for Patterns: Look for repeating patterns or similarities to simplify the problem-solving process.
- Use Theorems and Formulas: Leverage known formulas and principles that apply to the problem for a faster and more efficient solution.
Steps to Follow
- Read the problem carefully and highlight the key details.
- Sketch a diagram if applicable to help visualize the situation.
- Identify the strategy or method that best applies to the problem (e.g., algebra, geometric properties, etc.).
- Perform calculations step by step, ensuring that each step follows logically from the previous one.
- Double-check your work to verify that the solution makes sense and all steps are correct.
By following these problem-solving strategies, you can approach any mathematical challenge with confidence and clarity, ensuring that you achieve the best possible results.
Using Geometry Answer Keys Wisely
Having access to solutions is a powerful tool when preparing for mathematical assessments. However, it’s essential to use this resource strategically. Simply relying on provided solutions without understanding the reasoning behind them can hinder long-term learning and problem-solving skills. Instead, consider answer keys as a guide to reinforce your understanding and enhance your abilities.
By analyzing solutions step by step and comparing them with your approach, you can identify areas where you may have gone wrong and learn from your mistakes. The key to maximizing the benefits of answer keys is to approach them actively, using them as a learning tool rather than just a means to check your work.
How to Use Answer Keys Effectively
- Review Step-by-Step: Don’t just check the final answer. Review each step of the solution to understand the logic and methods used.
- Identify Patterns: Pay attention to recurring strategies, formulas, or methods that can be applied to other problems.
- Focus on Mistakes: If your answer differs from the key, figure out where your reasoning went wrong and correct your approach.
- Use as a Learning Aid: Use the solutions to clarify concepts you’re unsure about and reinforce your understanding of the material.
When Not to Rely on Answer Keys
- Don’t Use Them Too Early: Avoid looking at solutions before attempting the problem yourself. This defeats the purpose of independent problem-solving.
- Avoid Over-Reliance: Constantly checking the answer key can discourage independent thinking and reduce your ability to solve problems without help.
- Don’t Skip Work: Simply writing down the provided answer without understanding the solution can create gaps in your learning.
Using solutions wisely means using them to support your learning process, ensuring you can not only arrive at the right answers but also understand how to solve similar problems in the future.
Preparing for Advanced Geometry Exams
When tackling more complex mathematical assessments, preparation is key to success. The level of difficulty increases as the problems become more intricate and require a deeper understanding of concepts. Advanced assessments test not only your ability to apply formulas but also your logical reasoning and problem-solving strategies. It’s crucial to approach your preparation with a focused, structured plan that strengthens both your foundational knowledge and your ability to think critically under pressure.
To be truly prepared, one must go beyond memorization. Understanding how to approach and break down each problem is essential. By practicing a variety of problem types and applying different strategies, you can improve your ability to approach new challenges with confidence. Here’s how to begin your preparation effectively.
Building a Solid Foundation
- Master the Basics: Ensure that all fundamental concepts are well understood, as advanced topics are often built on them.
- Practice Regularly: Consistent practice of problems helps reinforce learning and builds familiarity with various types of questions.
- Use Additional Resources: Textbooks, online tutorials, and practice sets can provide extra examples and explanations.
Strategizing for Success
- Work Through Sample Problems: Familiarize yourself with the format and types of questions that are likely to appear.
- Simulate Test Conditions: Set up timed practice sessions to mimic the pressure of the real test.
- Review Mistakes: Pay attention to errors and learn from them by revisiting the concepts or strategies that caused confusion.
With focused practice and a deep understanding of core principles, you’ll be prepared to tackle even the most challenging problems. Developing both your theoretical knowledge and problem-solving techniques will give you the confidence needed to excel in any advanced mathematical test.