Geometry Exam Review Answer Key for Success

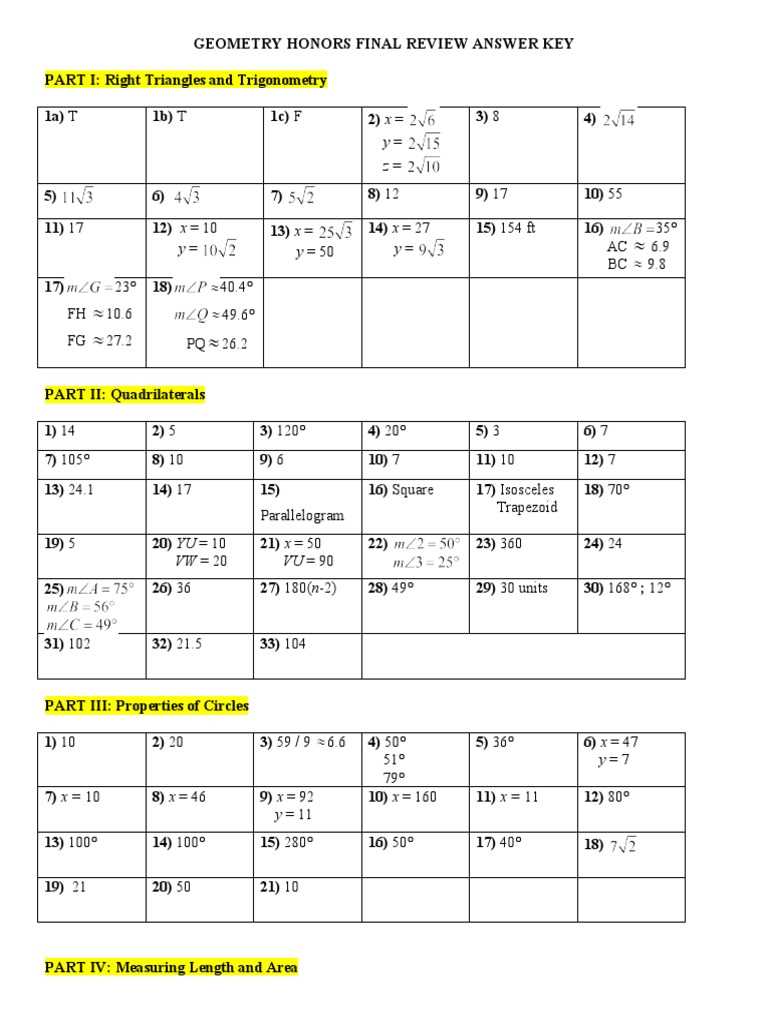
Preparing for a challenging math test requires not only understanding the material but also honing problem-solving skills and mastering key techniques. With the right approach, any student can tackle even the toughest questions with confidence. This section is designed to guide you through important steps that will improve your performance and strengthen your understanding.

By practicing with detailed solutions and focusing on the areas that require the most attention, you can significantly enhance your skills. This approach will help you identify common pitfalls, build a deeper understanding of concepts, and boost your overall readiness. Armed with the right knowledge, your success will be much more achievable.

Comprehensive Math Assessment Preparation Guide

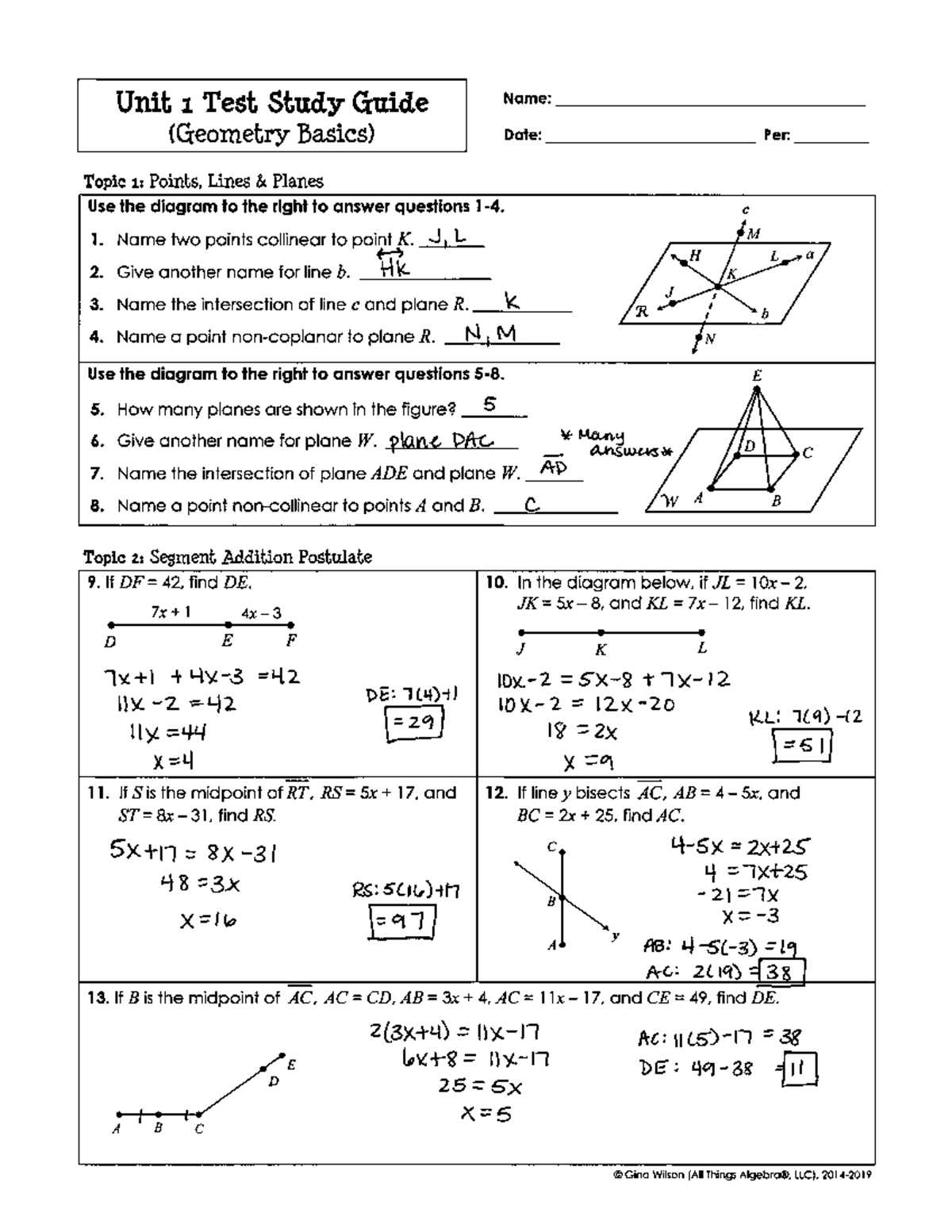
Successfully preparing for a mathematics test involves more than memorizing formulas and concepts. It requires understanding how to apply knowledge effectively, manage time, and stay focused under pressure. This guide aims to equip you with strategies to maximize your study sessions and improve performance on the test.

Begin by focusing on the fundamental principles and identifying areas where you may struggle. This will ensure that your preparation is targeted and efficient. Here are some key steps to follow:

Understand Core Principles: Break down each topic into its basic components to ensure you have a solid grasp of the core concepts.
Practice Regularly: Consistent practice with a variety of problems will help reinforce your understanding and build confidence.
Focus on Problem-Solving Techniques: Learn how to approach different types of questions with the right strategies, such as recognizing patterns and applying relevant formulas.
Review Mistakes: Analyze incorrect answers to understand where you went wrong and avoid repeating the same mistakes.
Utilize Visual Aids: Diagrams and graphs can be extremely helpful in understanding complex problems, so make sure to practice interpreting them.

Additionally, time management is essential for a successful outcome. During practice, try to simulate the time constraints of the actual test to improve your pacing. This will help you approach questions more strategically and reduce anxiety on the day of the assessment.

By integrating these strategies into your preparation routine, you’ll be well-equipped to approach any problem with confidence and efficiency. Take the time to thoroughly review and practice, and you’ll see significant improvement in your results.

Understanding Key Mathematical Concepts

Grasping the fundamental ideas that underpin mathematical problems is crucial for tackling a variety of questions. A deep understanding of the core principles allows you to approach each challenge with confidence and precision. Mastering these concepts not only helps you solve problems more effectively but also strengthens your overall mathematical reasoning.

Some of the most essential concepts to focus on include:

Angles and Their Properties: Recognizing different types of angles, such as acute, obtuse, and right angles, and understanding their relationships within various shapes.
Shapes and Figures: Familiarize yourself with common figures like triangles, circles, and polygons, and learn how to calculate their area, perimeter, and other properties.
Symmetry: Understanding the concept of symmetry in shapes and how it can simplify problem-solving by allowing for patterns and transformations.
Distance and Measurement: Master how to measure lengths, calculate distances between points, and use units of measurement accurately.
Coordinate Systems: Learn how to plot points, interpret graphs, and solve problems on the coordinate plane.
Volume and Surface Area: Grasp the formulas and methods used to calculate the volume and surface area of 3D shapes, such as cubes, spheres, and cylinders.

Once these concepts are understood, the next step is to practice applying them in various contexts. This includes solving problems that require a combination of multiple concepts, which helps you gain fluency in using your knowledge to tackle more complex scenarios.

By solidifying your understanding of these basic principles, you’ll be able to approach any mathematical question with a clear and focused mindset, improving both your accuracy and speed.

Importance of Practice for Success

To truly excel in any subject, regular practice is essential. It’s not enough to simply review theoretical concepts or memorize formulas; hands-on experience with real problems is what ultimately leads to mastery. By actively engaging with various types of questions, you can strengthen your understanding, improve speed, and reduce anxiety during assessments.

Benefits of Consistent Practice

Improves Problem-Solving Skills: The more you practice, the better you get at recognizing patterns and developing effective strategies to solve complex problems.
Builds Confidence: Frequent practice increases your familiarity with different question types, making you more confident in your ability to tackle them on the day of the test.
Enhances Retention: Repetition helps commit important concepts to memory, ensuring you recall them when needed most.
Boosts Speed and Efficiency: With time, you’ll learn to approach problems more quickly and accurately, making the most of the time available during assessments.

How to Maximize Your Practice

Start with Basics: Focus on mastering the foundational principles before moving on to more advanced problems.
Vary Your Practice: Work on a mix of question types to build adaptability and ensure you’re prepared for anything that might come up.
Simulate Real Conditions: Practice under timed conditions to replicate the pressure of the actual situation and improve your time management.
Review Mistakes: Take time to analyze incorrect answers and understand why the solution didn’t work, ensuring you don’t repeat the same errors.

By making practice a consistent part of your study routine, you’ll increase your readiness and significantly enhance your chances of success.

How to Approach Mathematical Word Problems

Word problems can often seem intimidating due to the mix of narrative and mathematical concepts. However, with a structured approach, these types of questions can be broken down into manageable steps. The key is to understand the information provided, translate it into mathematical terms, and then apply appropriate strategies to find the solution.

Step-by-Step Strategy for Success

Read Carefully: Begin by thoroughly reading the problem, making sure to highlight or underline key information such as measurements, relationships, and conditions.
Identify What Is Being Asked: Focus on understanding the main question. What is the problem seeking to solve or find? This will guide your next steps.
Visualize the Problem: Whenever possible, draw a diagram or sketch the scenario. This helps you see the problem more clearly and organize the data in a visual format.
Translate to Equations: Convert the written information into mathematical expressions. Assign variables to unknowns and write down the relationships between them.
Break It Down: Divide the problem into smaller, simpler parts if necessary. Solve step by step, rather than trying to tackle everything at once.

Tips for Overcoming Common Challenges

Look for Keywords: Certain words can indicate which operation to use. For example, “sum” suggests addition, “difference” indicates subtraction, and “product” refers to multiplication.
Check Units: Pay attention to units of measurement and make sure they are consistent throughout the problem. Converting units may be necessary.
Stay Organized: Keep track of your calculations and results in an orderly manner. This reduces the risk of making errors and helps you spot mistakes more easily.

By following these steps and practicing regularly, you’ll become more adept at approaching word problems with confidence and accuracy, ensuring that you can tackle them effectively under any conditions.

Mastering Mathematical Formulas for Efficiency

Familiarity with essential formulas is crucial for solving problems quickly and accurately. By mastering the most commonly used expressions and understanding their applications, you can streamline your problem-solving process and avoid unnecessary steps. With practice, these formulas become second nature, allowing you to focus on strategy rather than calculation.

The key to using formulas effectively is not only memorizing them but also understanding when and how to apply them. This knowledge allows for faster decision-making during tests and ensures that you don’t waste time trying to recall the right expression in the midst of a problem.

Prioritize Core Formulas: Focus on mastering the most frequently used formulas such as those for area, perimeter, volume, and surface area of common shapes.
Understand the Relationships: Be aware of how different formulas are connected. For instance, the relationship between area and volume for different shapes can help you apply the right one quickly.
Use Mnemonics and Tricks: Find creative ways to remember complex formulas. Mnemonics, visual aids, and patterns can help solidify your recall when you need it most.
Practice Application: Regularly solve problems that require different formulas to reinforce your understanding and ensure you can use them in various contexts.

By continuously refining your understanding and application of these formulas, you’ll increase both speed and accuracy, which is key to mastering any mathematical challenge.

Common Mistakes to Avoid in Mathematics

When solving mathematical problems, it’s easy to make small errors that can lead to incorrect results. These mistakes often stem from misunderstandings of concepts, misapplication of formulas, or simple calculation oversights. Recognizing and avoiding these pitfalls is essential for improving accuracy and ensuring success.

Some of the most frequent mistakes include:

Misinterpreting the Question: Failing to fully understand what is being asked can lead to solving the wrong part of the problem or applying the wrong method.
Ignoring Units of Measurement: Not keeping track of units can lead to confusing answers. Always double-check that your units match the question’s requirements.
Incorrect Formula Application: Applying a formula to the wrong shape or situation is a common error. Be sure you know which formula is appropriate for the problem at hand.
Rounding Too Early: Rounding off numbers too soon can lead to inaccurate answers. It’s best to keep values in their full form until the final step.
Skipping Steps: Rushing through problems and skipping intermediate steps can cause you to overlook key details, leading to mistakes in your final solution.
Overlooking Special Cases: Certain problems have specific conditions or exceptions. Be mindful of these exceptions and ensure they are accounted for in your solution.

By staying mindful of these common mistakes and taking care to avoid them, you’ll improve your problem-solving skills and increase your chances of achieving correct and efficient solutions.

Essential Theorems for Success

Understanding and applying fundamental principles is critical for solving complex problems. Several key theorems serve as the foundation for many types of questions, providing shortcuts and strategies for finding solutions. Familiarity with these theorems can streamline your approach and increase both accuracy and speed.

Below is a list of essential theorems you should master:

Theorem	Description	Application
Pythagorean Theorem	Relates the sides of a right triangle: ( a^2 + b^2 = c^2 ), where ( c ) is the hypotenuse.	Used to find the length of a missing side in right-angled triangles.
Triangle Sum Theorem	The sum of the angles in any triangle is always 180°.	Used to find missing angles in triangles when two angles are known.
Vertical Angles Theorem	When two lines intersect, the opposite (vertical) angles are equal.	Used to find missing angles when two lines intersect.
Isosceles Triangle Theorem	In an isosceles triangle, the angles opposite the equal sides are congruent.	Used to find missing angles in isosceles triangles.
Parallel Line Theorem	If two parallel lines are cut by a transversal, the alternate interior angles are congruent.	Used to find missing angles when parallel lines are intersected by a transversal.

Mastering these theorems will help you approach problems with confidence and make solving complex questions more manageable.

Strategies for Mathematical Proofs

Proofs are a vital part of solving problems, requiring logical reasoning to establish the truth of a statement. While they may seem daunting, developing a systematic approach can simplify the process. With the right techniques, you can break down complex problems into clear steps and arrive at solid conclusions.

Here are some effective strategies to enhance your approach to mathematical proofs:

Understand the Given Information: Before attempting to prove anything, carefully analyze the provided information. Identify known facts, properties, and any assumptions you can use.
Draw Diagrams: Visualizing the situation can help clarify relationships between elements. A well-drawn diagram can often reveal insights that are not immediately obvious from the written problem.
Work Backwards: Sometimes it’s useful to start from the conclusion and work backward. Think about what needs to be true for the statement to hold, and use that to guide your proof.
Use Logical Sequences: Structure your proof by connecting each step logically. Use definitions, postulates, and previously proven theorems to support your argument at each stage.
Be Precise with Justification: Always provide clear and accurate reasoning for every step. Make sure to reference the appropriate definitions or theorems to validate each claim you make.
Look for Patterns: In some cases, spotting patterns in the problem can lead to generalizations that simplify the proof. This is particularly useful when working with shapes or numbers that exhibit symmetry.

By following these strategies, you can improve your ability to construct well-organized and convincing proofs, making problem-solving more efficient and less intimidating.

Tips for Solving Coordinate Geometry Questions

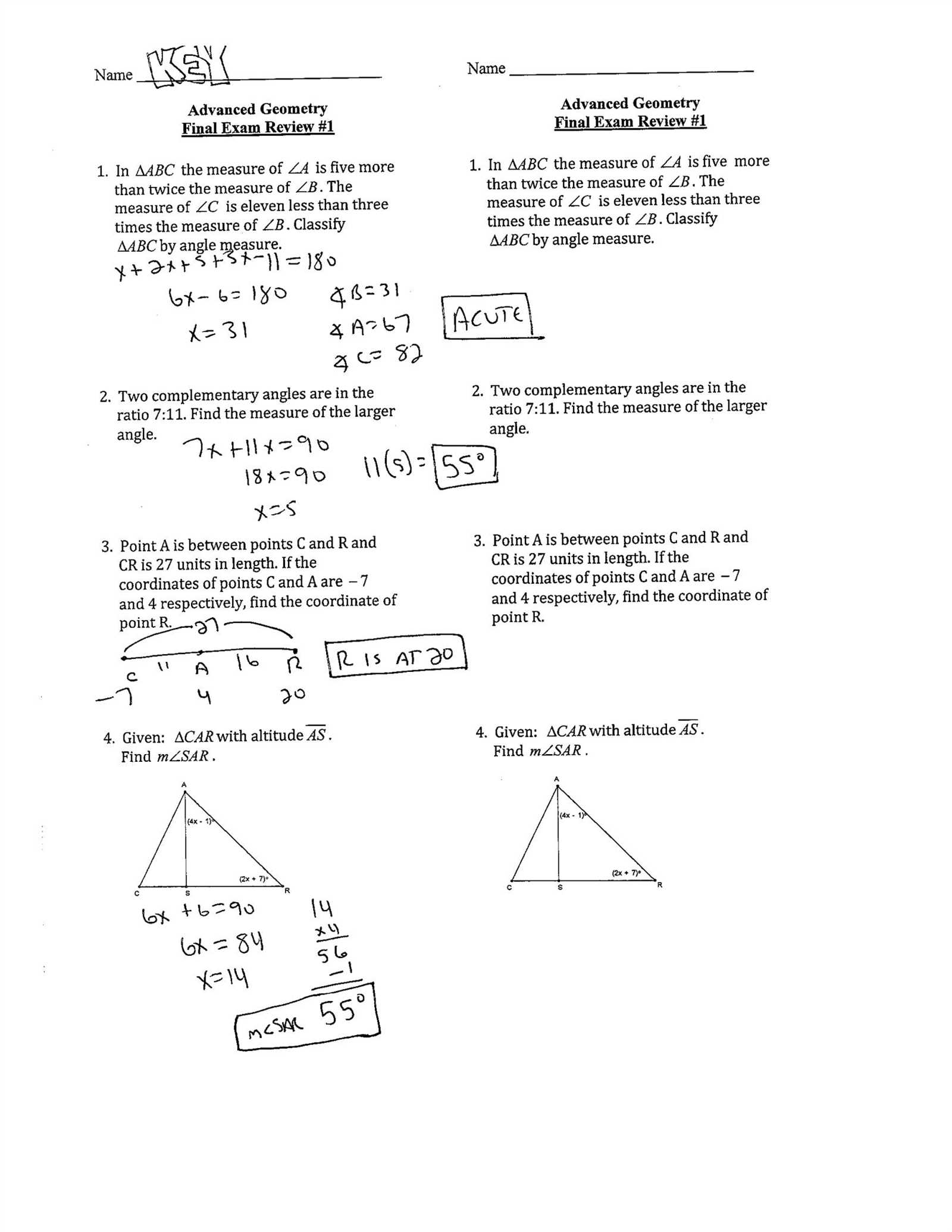
Solving problems involving points on a plane requires a good understanding of the relationships between coordinates, distances, and lines. These types of problems often involve algebraic calculations and geometric reasoning, and they can be approached systematically for more efficient solutions.

Here are some useful strategies to keep in mind when tackling coordinate-based problems:

Plot the Points: If possible, sketch the points on a coordinate plane. This visual aid helps you better understand the relationships between the points and can reveal patterns or symmetries in the problem.
Use the Distance Formula: When asked to find the distance between two points, remember the formula: (d = sqrt{(x_2 – x_1)^2 + (y_2 – y_1)^2}). This is essential for solving many problems related to length and measurement.
Apply the Midpoint Formula: If you need to find the midpoint between two points, use the formula: (left(frac{x_1 + x_2}{2}, frac{y_1 + y_2}{2}right)). This is useful for problems involving bisectors or centers of symmetry.
Equation of a Line: Know how to find the equation of a line using the slope-intercept form (y = mx + b) or the point-slope form. The slope formula (m = frac{y_2 – y_1}{x_2 – x_1}) is key for these calculations.
Understand Slopes and Perpendicularity: Lines that are perpendicular have slopes that are negative reciprocals of each other. Keep this in mind when working with perpendicular lines in problems.
Check for Special Geometries: Problems might involve specific geometric shapes like triangles or circles. Be familiar with the formulas for the area, perimeter, or equation of a circle and use them when needed.

By following these tips, you can efficiently tackle coordinate-based problems and solve them with greater accuracy and confidence.

Time Management Tips for Success

Efficient time management is crucial when solving complex problems under time pressure. Having a well-thought-out approach can help you maximize your performance and ensure that you complete all tasks effectively. By managing your time wisely, you can allocate enough attention to each question while avoiding unnecessary stress.

Here are some helpful tips to improve your time management skills:

Familiarize Yourself with the Format: Before starting, understand the structure of the test. This will help you estimate how much time you can allocate to each section or type of problem.
Prioritize Easy Questions: Begin with the problems that are easiest for you. Completing these quickly will boost your confidence and free up more time for the more challenging ones.
Set Time Limits: For each question or group of questions, set a specific time limit. This will keep you on track and prevent spending too much time on one problem.
Avoid Getting Stuck: If a question is taking too long, move on and come back to it later. Don’t let difficult problems consume the majority of your time.
Review Your Work: Reserve a few minutes at the end to review your answers. Ensure that you’ve addressed all parts of the questions and check for any simple mistakes.
Practice Under Timed Conditions: To build your speed and accuracy, practice solving problems within a set time limit. This will help you get comfortable working under pressure.

By applying these strategies, you can manage your time more effectively, ensuring that you give each question the attention it deserves while completing the entire set within the allotted time frame.

Breaking Down Complex Questions

When faced with a difficult problem, it’s easy to feel overwhelmed. However, the key to solving complex questions lies in breaking them down into smaller, more manageable parts. By taking a methodical approach, you can simplify even the most complicated scenarios and identify the steps needed to reach a solution.

Step-by-Step Approach

Start by carefully reading the question and identifying the essential information. Make a list of all the given data and what you are being asked to find. Drawing diagrams or visualizing the problem can often reveal relationships or patterns that aren’t immediately obvious from the text alone.

Decompose the Problem

Once you have a clear understanding of the question, break it down into smaller tasks. Identify each concept involved, whether it’s a relationship between points, lines, or shapes, and apply the appropriate methods or formulas to each part. Solving the sub-problems step by step can make the overall question much easier to handle.

By maintaining focus and following a structured approach, you can tackle even the most challenging problems with confidence and clarity.

Using Visual Aids to Solve Problems

Visualizing a problem can often make abstract concepts easier to understand and solve. By representing the given information with diagrams, graphs, or other visual tools, you can identify relationships between different elements and work through the solution more systematically. Visual aids serve as powerful tools for clarifying complex problems and guiding you towards the right approach.

Benefits of Visualizing Problems

Clarifies Complex Information: Diagrams help you see the structure of the problem more clearly, allowing you to identify key elements and their relationships.
Reveals Patterns: Visuals often make hidden patterns or symmetries more obvious, which can simplify the problem-solving process.
Aids in Applying Formulas: By plotting points or drawing shapes, you can more easily apply formulas related to distance, area, or angles.
Improves Accuracy: A visual representation reduces the chances of missing important details and helps ensure that you’re working with the correct information.

Effective Visual Tools

Diagrams: Drawing clear diagrams is one of the most effective ways to represent geometric problems, whether you’re working with shapes, lines, or angles.
Graphs: Use graphs to plot points and represent relationships between variables in problems involving coordinate systems.
Charts and Tables: In some cases, using charts or tables to organize given data can help break down a complex problem into manageable parts.
Models: 3D models or virtual simulations can also provide a hands-on approach to understanding spatial relationships in more advanced scenarios.

Incorporating visual aids into your problem-solving process enhances your understanding, reduces confusion, and improves your overall effectiveness in solving complex problems.

Practice Tests: Best Practices

Taking practice tests is one of the most effective ways to prepare for assessments. They help you gauge your understanding, identify areas of improvement, and build the confidence needed to perform under pressure. By simulating real testing conditions, you can develop the skills and strategies necessary for success. However, the key to benefiting from practice tests lies in how you approach them.

Key Steps to Maximize Practice Test Effectiveness

Set Time Limits: To simulate real conditions, set a time limit for each practice session. This helps you practice managing your time and ensures you stay on track during the actual test.
Review Mistakes Thoroughly: After completing a practice test, go over each question you got wrong. Understanding why you made a mistake and how to correct it is crucial for improving your performance.
Take Multiple Tests: Repetition is key. The more practice tests you take, the more familiar you become with the material and the testing format. Consistency helps reinforce concepts and strategies.
Simulate Real Test Conditions: Try to mimic the conditions of the actual test environment as much as possible. Work in a quiet space, avoid distractions, and follow the time constraints.
Focus on Weak Areas: Use the results from each test to identify areas where you struggle. Focus your study efforts on these topics to improve your overall performance.

Best Tools for Practice Tests

There are many resources available that provide high-quality practice tests. Here are some popular options:

Resource	Type of Content	Availability
Official Test Prep Materials	Realistic Practice Tests	Available for Purchase or Free
Online Platforms	Interactive Tests with Instant Feedback	Subscription-Based
Textbooks and Workbooks	Practice Questions and Solutions	Available in Stores

By following these best practices, you can enhance your test preparation and increase your chances of success. Consistent practice, focused review, and a strategic approach will allow you to tackle even the toughest questions with confidence.

Reviewing Key Figures and Shapes

Mastering the fundamental shapes and their properties is essential for solving a wide range of problems. These figures form the foundation of many concepts and calculations, so having a clear understanding of their characteristics, formulas, and relationships will significantly enhance your problem-solving abilities. By familiarizing yourself with the key types of shapes, you can more effectively recognize patterns and apply the correct methods during assessments.

Important Figures to Focus On

Triangles: Pay attention to different types, such as equilateral, isosceles, and scalene, and understand the properties of each. Key concepts include Pythagoras’ theorem and the area formula.
Circles: Understanding the relationships between radius, diameter, circumference, and area is crucial. Be sure to know how to calculate sector areas and arc lengths.
Quadrilaterals: From squares and rectangles to parallelograms and trapezoids, knowing the properties of these shapes is vital. Focus on area formulas and diagonal relationships.
Polygons: Learn the formulas for regular polygons, including the sum of interior angles and the area of regular figures.
Solid Figures: Familiarize yourself with three-dimensional shapes like cubes, spheres, cones, and cylinders. Understanding volume and surface area formulas will be beneficial.

Understanding the Relationships Between Shapes

Once you grasp the individual properties of each shape, it’s important to focus on their interrelationships. For example, the properties of triangles can be extended to the calculation of other polygons, and knowledge of circles can be applied to sectors and segments. Understanding these connections allows you to solve more complex problems with greater ease.

Being able to quickly recall these figures and apply their properties is an essential skill that will improve both speed and accuracy when tackling various problems.

How to Check Your Work

Ensuring that your calculations and reasoning are correct is essential to achieving success in any problem-solving task. When working through a set of problems, it’s crucial to have a systematic approach for verifying your results. Taking the time to double-check your work can help catch simple errors and ensure the accuracy of your solutions.

Follow these steps to verify your results:

Review the Problem Statement: Always start by carefully reading the problem again. Make sure you understand the requirements and the given information. This can prevent misinterpretation of the question and ensure you’re solving the correct task.
Recheck Your Calculations: Go through each calculation step by step. Check that all arithmetic operations are accurate, especially in formulas involving multiple variables. Sometimes a small mistake can lead to incorrect results.
Confirm Units and Labels: Ensure all measurements are in the correct units, and that you’ve labeled your results clearly. Mismatched units can lead to incorrect interpretations and answers.
Use Different Methods: If possible, try solving the problem in a different way to see if you arrive at the same result. Using an alternative method or verification tool (like a calculator or graphing software) can help confirm your answer.
Check Your Logical Reasoning: Review the reasoning behind your steps. Make sure each conclusion follows logically from the previous one, and that no assumptions were made without justification.
Look for Common Mistakes: Be aware of common errors, such as sign mistakes, misapplying formulas, or neglecting key details in a diagram. These are often easy to miss but can significantly affect the outcome.

Final Check: After verifying all the above steps, step back and ask yourself if your answer makes sense in the context of the problem. Does it align with your expectations or common knowledge of the topic? If the answer seems reasonable and consistent with all checks, it is likely correct.

By adopting this systematic approach, you can significantly reduce errors and ensure the accuracy of your work, whether it’s for practice or a formal assessment.

Handling Stress Effectively

Stress can be a major obstacle when facing a challenging task, especially when the pressure of time and performance builds up. Managing anxiety and staying focused is essential to ensure that you perform to the best of your ability. The key to handling stress is not just about avoiding pressure, but rather about developing strategies to maintain clarity and calm throughout the process.

Here are some tips to manage stress effectively:

Practice Mindful Breathing: Simple breathing exercises can help calm your nerves. Take a few deep breaths, inhale through your nose, hold for a few seconds, and exhale slowly. This technique can reduce anxiety and help you regain focus.
Stay Organized: Prepare ahead of time by creating a study schedule and sticking to it. Knowing that you’ve allocated enough time to study will help reduce feelings of being overwhelmed. Prioritize tasks based on difficulty and time required.
Break Down the Material: Large amounts of information can seem daunting. Break your preparation into smaller, manageable sections. Tackle one part at a time and give yourself breaks in between to refresh your mind.
Practice Under Timed Conditions: Simulating real conditions will help you get used to the pressure of time. Set a timer while solving practice problems to mimic the time constraints you’ll face. This will help you pace yourself during the actual challenge.
Stay Positive: Maintaining a positive mindset can help you stay calm under pressure. Focus on your strengths and remind yourself of the effort you’ve put into preparation. Positive affirmations can boost your confidence.
Take Breaks: Study in intervals and allow yourself time to relax. A quick break can clear your mind and improve your focus when you return to work. Avoid studying for long periods without rest as it can lead to burnout.

On the Day: On the day of the challenge, ensure that you get enough rest the night before. Avoid cramming or overloading your mind with new information. Stick to what you know and trust the preparation you’ve done. Manage your time effectively and take it one step at a time.

By adopting these strategies, you can reduce stress, stay focused, and increase your chances of success. Remember, it’s not about perfection, but about doing your best under pressure.

Leveraging Online Resources for Help

In today’s digital age, the internet offers an array of tools and resources to assist with learning and problem-solving. These platforms can help clarify difficult concepts, provide practice problems, and offer personalized guidance. By utilizing these resources effectively, learners can improve their understanding and performance, making complex material more accessible.

Some valuable online resources to consider include:

Tutorial Websites: Websites like Khan Academy, Coursera, or EdX offer free tutorials and video lessons on a wide range of topics. These platforms break down difficult concepts into manageable steps and provide visual demonstrations.
Interactive Problem Solvers: Tools such as WolframAlpha or Symbolab allow you to input problems and receive step-by-step solutions. These tools help you see the process behind the solution and understand where mistakes may have occurred.
Online Forums and Communities: Websites like Stack Exchange and Reddit host communities where learners and experts alike can discuss specific questions and solutions. Asking for help in these forums can provide different perspectives and explanations.
Educational YouTube Channels: YouTube has countless educational channels that explain various topics with visual aids and real-world examples. Channels like PatrickJMT or MathTheBeautiful offer in-depth explanations and tips for tackling tough problems.
Mobile Apps: There are many apps dedicated to learning and problem-solving, such as Photomath and Microsoft Math Solver. These apps allow you to scan problems and receive immediate solutions with clear, understandable steps.

Make sure to:

Verify information: While online resources can be immensely helpful, ensure that the content is from a reliable source. Cross-reference explanations to confirm accuracy.
Use resources regularly: Consistent use of online tools can help reinforce concepts and make learning feel less overwhelming.
Practice independently: Relying on online tools for guidance is helpful, but it’s important to practice and solve problems on your own to build confidence and mastery.

By incorporating online tools into your study routine, you can take control of your learning, access a wealth of information, and tackle challenges more effectively. Don’t hesitate to explore different platforms to find what works best for you.

Final Review Checklist for Assessments

Before facing any important evaluation, it’s essential to have a systematic approach to ensure you are fully prepared. The final stages of preparation should focus on revisiting key concepts, reinforcing problem-solving techniques, and checking for any weak areas. By following a structured checklist, you can ensure that no aspect is overlooked and approach the test with confidence.

Key Concepts to Revisit

Formulas: Review essential formulas for areas, volumes, and perimeters of various shapes. Ensure you can quickly recall and apply them in different scenarios.
Properties of Figures: Be familiar with the characteristics of various figures–such as angles, sides, and symmetry–and how these properties affect calculations.
Theorems and Postulates: Ensure you understand the foundational principles that guide problem-solving. Review important proofs and how they apply to specific types of questions.
Coordinate System Knowledge: Double-check your understanding of coordinate plotting, distance formulas, and equations of lines and curves.

Problem-Solving Skills

Practice with Sample Problems: Solve problems from different categories to reinforce your problem-solving techniques. Time yourself to improve your speed and accuracy.
Check for Common Mistakes: Be aware of common errors, such as misapplying formulas or forgetting unit conversions. Stay alert for small details that can lead to bigger mistakes.
Review Previous Work: Go through your past work or any practice tests you’ve taken to identify patterns in the mistakes y