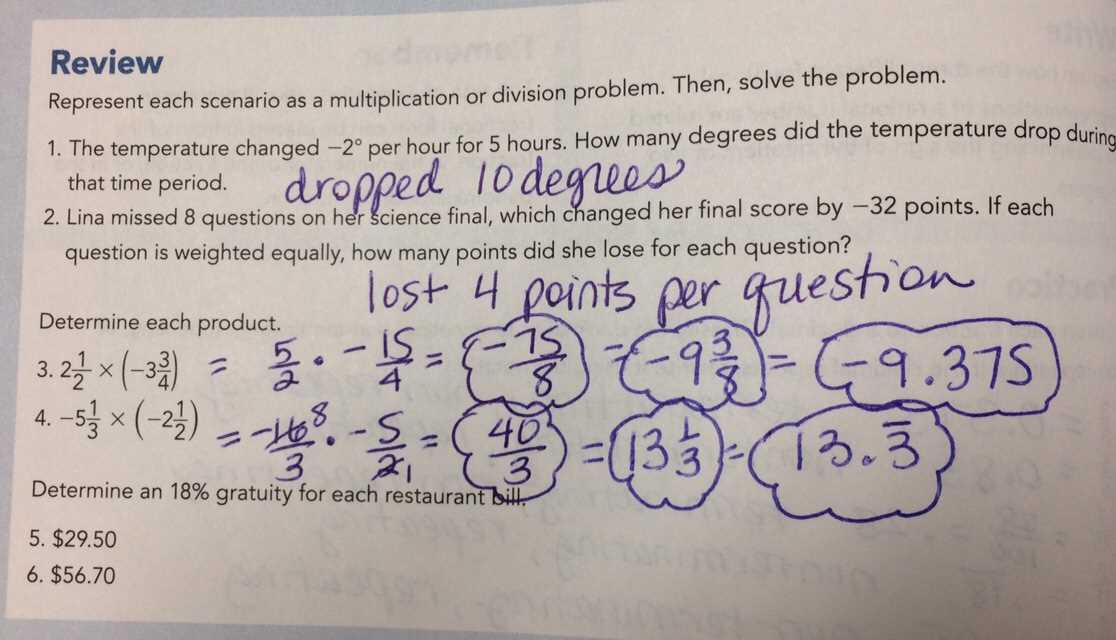
Mathematics often presents challenges for learners, especially when it comes to mastering foundational skills. Solving problems effectively requires a strong grasp of core techniques and strategies. In this section, we will explore how to navigate complex exercises and improve problem-solving abilities through clear explanations and detailed solutions.
The focus is on providing a structured approach to understanding key concepts, ensuring that students can work through problems with confidence. By breaking down each exercise, we aim to highlight the thought process behind finding the correct solutions. This method not only aids in completing individual tasks but also builds a deeper understanding of the overall subject matter.
With the right guidance and practice, anyone can enhance their mathematical skills and develop the critical thinking necessary to tackle more advanced topics. The following solutions will help guide learners through common problem types and provide clarity on the steps needed to reach accurate results.
Math Problem Solutions and Explanations
In this section, we provide detailed explanations to help solve mathematical exercises that focus on fundamental concepts. The goal is to offer clarity and insight into the steps required to successfully approach and complete each task. By breaking down the solutions, learners can gain a deeper understanding of the methods used to find accurate results.
Each exercise presents unique challenges, but with the right approach, any student can improve their skills and confidence in solving similar problems. The solutions provided here serve as a guide, outlining the necessary steps and helping to identify key patterns and techniques used in mathematics.
For those looking to strengthen their problem-solving abilities, this section offers the support needed to build a solid foundation. By practicing and understanding the rationale behind each solution, learners will be better prepared for more complex topics and will develop stronger reasoning skills.
Understanding Basic Mathematical Concepts

This section aims to provide a clear understanding of essential mathematical principles that form the foundation for more advanced topics. By mastering these core skills, learners can tackle a wide range of problems with confidence. The focus is on building a strong conceptual framework that simplifies complex calculations and enhances problem-solving abilities.
Throughout the exercises, students will encounter various techniques that help break down complicated problems into manageable steps. The goal is to demystify each challenge by explaining the reasoning behind every move. Understanding the underlying principles is key to improving accuracy and efficiency in mathematics.
By practicing these fundamental strategies and reflecting on their application, students can strengthen their skills and approach future problems with greater ease. This section encourages learners to develop a deeper understanding and to think critically about each step in the problem-solving process.
How to Use the Answer Guide Effectively
Utilizing a solution guide is an essential part of learning, as it helps clarify the reasoning behind each answer. The key to using it effectively lies in not just copying the solutions, but in understanding the process that leads to them. By carefully reviewing each step, learners can gain valuable insights into problem-solving strategies and techniques.
Instead of simply checking if your answer is correct, focus on how each step in the solution is derived. This approach will allow you to recognize patterns, identify common errors, and apply the same strategies to new problems. Revisit each solution multiple times if necessary, to reinforce your understanding and build a stronger foundation.
Remember that the guide is meant to assist your learning, not replace the problem-solving process. It should serve as a tool for checking your work and enhancing your skills, rather than a shortcut to completing exercises. By integrating this resource thoughtfully, you can accelerate your learning and gain a deeper comprehension of the material.
Overview of Exercises on This Section
The exercises in this section are designed to challenge students’ understanding of core mathematical concepts while providing a diverse range of problem types. Each problem introduces new techniques that help reinforce the skills needed to solve more complex tasks. The goal is to encourage critical thinking and allow students to apply learned strategies to different scenarios.
The exercises vary in difficulty, starting with simpler tasks and progressing to more intricate problems that require careful attention to detail. By working through these problems, students will develop a deeper understanding of the relationships between numbers and operations, enhancing their problem-solving capabilities.
Throughout the section, learners will encounter a mixture of numerical operations, word problems, and pattern recognition tasks. These are structured to build confidence, ensuring that students are gradually prepared for more advanced challenges. The focus is on applying basic principles in various contexts to strengthen overall mathematical fluency.
Common Mistakes in Basic Math
When learning fundamental mathematical concepts, students often encounter common mistakes that can hinder their progress. Identifying these errors is crucial to improving understanding and avoiding confusion. In this section, we will discuss some of the most frequent issues that arise and provide tips on how to overcome them.
Incorrect Application of Operations
A frequent mistake is the improper use of mathematical operations, such as addition, subtraction, multiplication, and division. These errors typically occur when the order of operations is ignored or misunderstood.
- Mixing up the sequence of operations, leading to incorrect results.
- Performing operations from left to right without considering parentheses or exponents first.
- Incorrectly applying distributive properties or combining terms prematurely.
Misunderstanding Variables and Expressions
Another common issue is not fully grasping the role of variables or how to manipulate algebraic expressions. Misinterpreting the relationship between terms can lead to mistakes in solving equations.
- Confusing constants and variables, leading to wrong conclusions.
- Failing to simplify expressions before solving for the variable.
- Misapplying the properties of equality when solving for unknowns.
By recognizing and addressing these typical mistakes, students can strengthen their skills and approach problems with greater accuracy. Consistent practice and reviewing solutions can help reduce errors over time, improving overall mathematical proficiency.
Step-by-Step Solutions for Exercises
This section provides a detailed breakdown of how to approach each problem, ensuring clarity and understanding at every stage. By following the step-by-step solutions, learners can easily grasp the method behind each calculation and improve their problem-solving skills. The focus is on making the process as clear and intuitive as possible, so students can replicate these techniques in future tasks.
Example 1: Solving for Unknown Variables
In this example, we will walk through the process of solving an equation for an unknown value. Follow each step carefully to see how to isolate the variable and find the correct solution.
- Start with the given equation.
- Perform the first operation, such as simplifying both sides or moving terms around.
- Continue with the next operations in the correct order to solve for the variable.
- Check your solution by substituting it back into the original equation.
Example 2: Working with Expressions
This example demonstrates how to simplify and evaluate an expression. Understanding how to combine like terms and apply the correct operations is key to solving such problems.
- Identify the terms in the expression and group them accordingly.
- Combine like terms where possible.
- Simplify the expression step by step, ensuring accuracy with each operation.
- Evaluate the final result once the expression is simplified.
By following these clear, methodical steps, students can gain a deeper understanding of how to approach and solve mathematical problems with confidence. Practice is key to mastering these techniques, and revisiting the solutions helps reinforce the concepts learned.
Tips for Improving Math Skills
Improving your mathematical abilities requires consistent practice, focus, and a strategic approach. Whether you are working through basic exercises or tackling more complex problems, there are several ways to enhance your understanding and boost your confidence. This section offers practical tips to help strengthen your skills and develop a deeper comprehension of key mathematical concepts.
- Practice Regularly: Consistent practice is essential for reinforcing concepts and improving problem-solving efficiency. Set aside time each day to work through exercises and revisit challenging topics.
- Understand the Process: Focus on understanding the steps behind each solution, not just memorizing answers. This deeper comprehension helps when facing unfamiliar problems.
- Break Problems Down: If you encounter a difficult problem, break it into smaller, more manageable parts. Solving these smaller components can make the larger problem easier to handle.
- Use Visual Aids: Draw diagrams or use visual tools to represent problems. This can help clarify relationships between different elements and make abstract concepts more tangible.
- Review Mistakes: Take the time to review errors you’ve made and understand why the correct solution works. This reflection is key to avoiding similar mistakes in the future.
- Seek Additional Resources: If a particular concept is unclear, don’t hesitate to seek out additional resources, such as instructional videos, practice sheets, or tutoring services.
By applying these strategies, learners can improve their mathematical thinking and build a stronger foundation for future studies. The key is persistence and a willingness to tackle challenges with an open mind.
Using Visual Aids for Math Problems
Visual aids are powerful tools that can simplify complex mathematical concepts, making them easier to understand and solve. By representing problems visually, students can gain a clearer understanding of relationships between numbers, variables, and operations. This approach can turn abstract ideas into something tangible and manageable.
Benefits of Visualizing Math Problems
Using diagrams, charts, or graphs can provide immediate insight into the structure of a problem. Visual representations help students break down complicated steps into simpler, more understandable parts.
- Clarify relationships: Visual tools help identify how different elements of a problem are connected, such as how variables affect one another.
- Track progress: Diagrams allow students to track their progress and visualize each step in a solution, reducing the likelihood of mistakes.
- Enhance memory retention: Seeing a concept in action can improve understanding and make it easier to recall information when needed.
Common Types of Visual Aids
There are several types of visual aids that can be used to aid in solving math problems effectively:
- Graphs: Graphs are useful for representing relationships between different variables, especially in problems involving linear equations or functions.
- Tables: Organizing data in tables can help simplify the comparison of values and highlight patterns.
- Number Lines: Number lines are especially useful for illustrating the positions of numbers or operations on a continuum, making it easier to understand inequalities and operations.
Incorporating these visual tools into problem-solving can enhance comprehension, reduce errors, and make mathematics more approachable for students. By actively using these methods, learners can improve their ability to solve complex problems and build a solid foundation for future mathematical challenges.
Why Basic Math Foundations Matter for Advanced Studies
Mastering foundational math concepts is essential for success in more advanced mathematical subjects. Without a strong grasp of the basics, students can struggle to understand more complex topics and miss key principles that build upon these early lessons. A solid understanding of fundamental operations and problem-solving strategies prepares students to tackle the challenges of higher-level mathematics with confidence.
Building Problem-Solving Skills
Learning core mathematical concepts helps develop essential problem-solving skills that are applicable across various fields. As students progress to more advanced topics, they will encounter problems that require creative thinking and a clear understanding of basic principles. Those who have a strong grasp of these skills can easily adapt to new challenges and solve complex problems efficiently.
Creating a Strong Mathematical Foundation
Advanced mathematical concepts such as calculus, geometry, and statistics all rely on the principles learned in earlier stages. The ability to manipulate variables, solve equations, and understand patterns is crucial in more advanced studies. Without these foundational skills, students may find themselves struggling to grasp new concepts, making it harder to succeed in higher math courses.
By ensuring a solid understanding of fundamental topics, students can approach advanced mathematical challenges with greater ease and continue to build on their knowledge throughout their academic journey.
Common Struggles in Basic Math
Many students face challenges when learning fundamental mathematical concepts, especially when transitioning from arithmetic to more abstract thinking. The difficulties often stem from a lack of understanding of core principles, poor study habits, or confusion about how to approach problems. Recognizing and addressing these struggles early on can help students gain the confidence they need to succeed in more advanced topics.
- Difficulty Understanding Variables: Many learners find it hard to understand how letters and symbols represent numbers. This abstract concept can be intimidating for beginners and may lead to confusion when solving equations.
- Struggling with Word Problems: Translating a word problem into a mathematical equation is a common struggle. Students often have difficulty identifying the relevant information and figuring out the correct operations to apply.
- Misunderstanding Negative Numbers: Working with negative numbers, especially when dealing with addition and subtraction, can be tricky. Many students struggle to understand the rules of signs, leading to frequent mistakes.
- Difficulty with Fractions: Fractions can be particularly challenging for those new to mathematics. Concepts such as simplifying fractions, finding common denominators, and performing operations with fractions often lead to confusion.
- Not Grasping the Order of Operations: The order in which operations should be performed is a concept that many students struggle with. Forgetting to follow the correct sequence often results in incorrect answers.
Recognizing these challenges and taking steps to address them can make a significant difference in a student’s ability to succeed in math. By practicing regularly, seeking help when needed, and approaching problems with patience, learners can overcome these common struggles and build a stronger mathematical foundation.
Breaking Down Complex Math Problems
Complex mathematical problems often seem overwhelming at first glance, but they can be made manageable by breaking them into smaller, more digestible steps. By focusing on one part of the problem at a time, students can avoid confusion and tackle each challenge methodically. Understanding the structure of a problem and approaching it in a step-by-step manner is key to finding the correct solution.
Steps to Simplify Complex Problems
When faced with a difficult problem, it’s helpful to follow a structured approach to simplify the task:
- Identify the goal: Understand what the problem is asking and what you need to find.
- Organize the information: List all given data and any relationships between numbers or variables.
- Break the problem into smaller parts: Tackle each part of the equation or expression separately, solving as you go.
- Check your work: After completing each step, verify the results to ensure accuracy before proceeding to the next part.
Example of Breaking Down a Problem
Here’s an example to illustrate how to break down a complex problem:
| Step | Action | Result |
|---|---|---|
| 1 | Identify the unknown variable in the equation | Variable x |
| 2 | Gather and organize the given data | x + 4 = 10 |
| 3 | Isolate the variable by subtracting 4 from both sides | x = 6 |
| 4 | Verify the solution by substituting x back into the original equation | 6 + 4 = 10 (True) |
By following these steps, the complexity of a problem is reduced, allowing for a clearer understanding of how to approach and solve it. This methodical approach not only improves accuracy but also boosts confidence in solving more difficult tasks.
How to Prepare for Math Tests
Preparing for math exams requires more than just reviewing notes; it’s about developing a deep understanding of the concepts and practicing problem-solving strategies. Successful test preparation involves organizing your study time, focusing on areas of difficulty, and engaging in consistent practice to strengthen your skills. The more thoroughly you prepare, the more confident you will feel on test day.
Start by reviewing the key topics that will be covered in the exam. Identify any areas where you feel uncertain and spend extra time on those concepts. It’s essential to understand both the theory behind the problems and how to apply the techniques in practice. Working through example problems and previous tests can help solidify your understanding and expose any weaknesses in your knowledge.
Next, create a study schedule to ensure you’re covering all necessary material without cramming the night before. Consistent study sessions over the course of several weeks lead to better retention and understanding. Be sure to give yourself time for breaks to avoid burnout.
Finally, make use of practice exams and review exercises. Simulating the test environment will help you manage your time during the actual exam and give you a chance to apply the techniques you’ve learned under timed conditions. Don’t hesitate to ask for help if you’re struggling with any specific topics–teachers, tutors, or study groups can provide valuable insights.
Building Confidence in Math
Developing confidence in mathematics is crucial for overcoming challenges and achieving success. When students feel confident in their problem-solving abilities, they are more likely to tackle complex tasks without fear of failure. Building this confidence involves consistent practice, understanding key concepts, and celebrating small victories along the way. With the right approach, students can transform their anxiety into empowerment and gain a stronger grasp of mathematical principles.
Start with the Basics
Confidence begins by mastering the fundamental concepts. Ensure a solid understanding of basic operations and principles, as they serve as the foundation for more complex topics. Focus on areas where you feel less confident and break them down into manageable steps. By working through simpler examples, students can gradually build their skills and increase their comfort level.
Practice Regularly and Track Progress
Frequent practice is key to reinforcing what you’ve learned and gaining confidence. Set aside dedicated time for math exercises, and track your progress to see improvements over time. Using various resources, such as practice problems or interactive exercises, can also provide additional support. The more consistent your practice, the more comfortable you will feel tackling even the most difficult problems.
Practical Applications of Math Concepts
Understanding fundamental math concepts is not just about solving academic problems; it has real-world applications that make life easier and help in various professions. Whether you are budgeting personal finances, analyzing data, or designing structures, the ability to work with numbers and equations is essential. These foundational skills serve as a stepping stone for more complex problem-solving and can be applied in various fields like engineering, technology, economics, and even in daily activities.
Everyday Situations
Mathematics is everywhere in our daily routines. Here are some examples of how basic math concepts are used in everyday life:
- Budgeting: Understanding how to manage expenses, calculate discounts, and balance a budget requires arithmetic skills and a solid grasp of mathematical principles.
- Shopping: Calculating sale prices, determining percentages, and comparing prices are common tasks that require math knowledge.
- Home Repairs: From measuring areas for flooring or wallpaper to understanding proportions when mixing materials, math plays a key role in home improvement.
Professional Uses
In many professions, math is an integral tool. Here are a few examples:
- Engineering: Engineers apply mathematical principles to design structures, machines, and systems that are safe and functional.
- Data Analysis: Statisticians and data analysts rely on mathematical concepts to interpret data, recognize patterns, and make predictions.
- Technology: Programmers and software developers use algorithms and equations to create code that powers apps, websites, and artificial intelligence.
By applying basic math concepts to these practical scenarios, students can see the importance of mastering these skills for both their academic and professional futures. Understanding these principles builds confidence and provides the foundation for further mathematical learning.
How to Double-Check Your Answers
Ensuring the correctness of your solutions is essential for success in any mathematical task. It involves reviewing each step carefully and using strategies that can help spot potential errors. By verifying your results, you can build confidence in your skills and improve your overall accuracy. Below are some helpful approaches to double-check your work effectively.
Method 1: Review Each Step Carefully
Going through the entire process again can reveal mistakes you may have missed during the initial solution. This method involves checking the logic and calculations performed in each step.
- Recheck every calculation: Confirm that each operation–whether addition, subtraction, multiplication, or division–was carried out correctly.
- Look for overlooked details: Ensure that no small details, such as negative signs or decimal places, were missed or misinterpreted.
- Confirm the problem’s requirements: Make sure that your answer matches the question’s demands, especially when dealing with word problems or multi-step operations.
Method 2: Use an Alternative Approach
Another way to verify your solution is by attempting a different method to solve the problem. This technique allows you to cross-check your answer from a different angle.
- Reversing the operations: If possible, work backwards from your result to see if you arrive at the original values or setup of the problem.
- Estimate your answer: Make a rough estimate of the expected result. If your calculated answer is far from the estimate, it’s time to review your steps.
- Check consistency with examples: Compare your solution with similar problems or known examples to ensure that your approach is valid.
Double-checking your work is a crucial step in becoming more proficient and confident in solving mathematical problems. By applying these strategies, you can identify mistakes early and improve the quality of your solutions.
Extra Resources for Algebra Practice
For anyone looking to strengthen their mathematical understanding, additional practice materials are invaluable. These tools provide opportunities to deepen knowledge, improve problem-solving abilities, and gain confidence in tackling challenging topics. Below are some excellent resources designed to enhance your learning journey.
1. Interactive Learning Websites
Several online platforms offer a wealth of practice problems and interactive lessons that can help solidify your grasp of key concepts. These resources often provide instant feedback, allowing for self-paced learning and better retention.
| Resource | Description | Link |
|---|---|---|
| Khan Academy | Free, self-paced lessons and exercises covering a wide range of topics in mathematics. | Visit Site |
| IXL | Comprehensive practice exercises with instant feedback to track progress. | Visit Site |
| Brilliant | Problem-solving based courses designed to help you think critically and deeply about concepts. | Visit Site |
2. Books and Printable Guides
In addition to online tools, printed books and guides are perfect for structured practice. They often contain a wide variety of exercises, as well as step-by-step solutions and explanations to ensure thorough understanding.
- Workbook Exercises: Printed books designed for practice, with problems and solutions at various levels of difficulty.
- Study Guides: Printed materials that break down complex topics into manageable steps for easier understanding.
- Printable Flashcards: Quick reference cards that are ideal for memorizing important concepts, formulas, and techniques.
Utilizing these resources can provide a solid foundation for mastering mathematical skills, offering flexibility and diverse methods to reinforce key concepts.