Springboard Algebra 1 Unit 4 Answer Key Guide

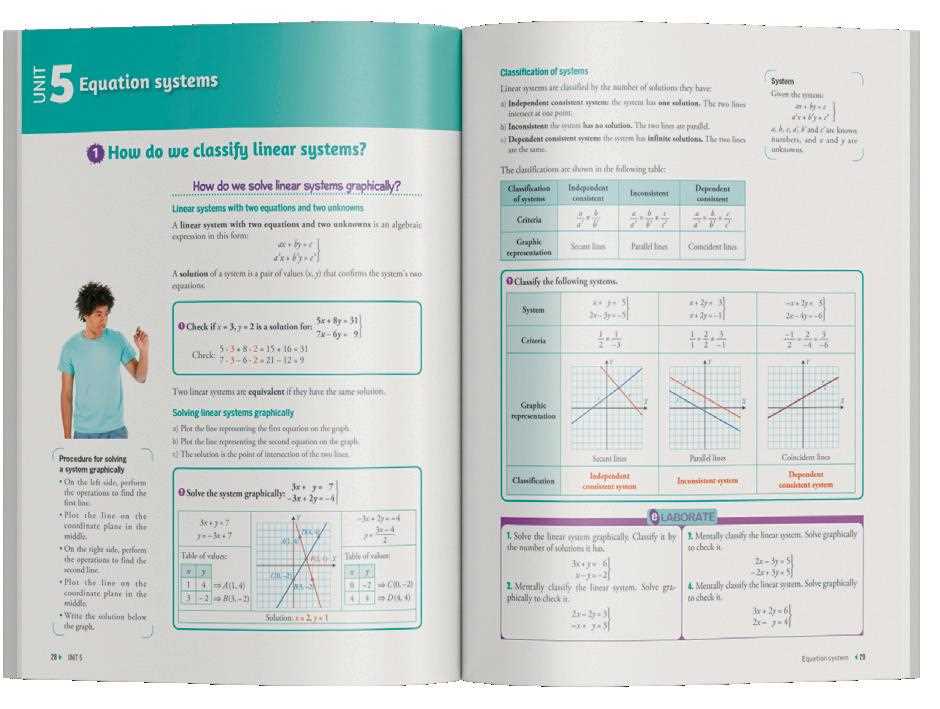
In this section, we focus on essential strategies for tackling a variety of mathematical challenges. Whether you’re working through equations, analyzing functions, or grappling with graphing techniques, mastering these concepts is crucial for advancing your understanding of mathematical principles. Clear strategies and a systematic approach can make even the most complex problems manageable.

Effective problem-solving relies on a strong foundation and the ability to apply learned methods in different contexts. Understanding the relationships between variables and honing your skills in interpreting and manipulating expressions will help you approach each challenge with confidence. By practicing regularly and reviewing solutions, you’ll be able to recognize patterns and develop a deeper comprehension of mathematical concepts.

For those seeking to refine their approach, this guide provides step-by-step explanations and examples to support your learning. With the right tools and mindset, you’ll navigate through the material efficiently and with greater ease.

Springboard Algebra 1 Unit 4 Overview

This section focuses on foundational concepts essential for solving a range of mathematical challenges. It introduces various techniques for understanding and working with numerical relationships, providing the tools necessary for tackling more complex problems. As you progress, you’ll gain deeper insight into how different mathematical principles connect and reinforce each other, making problem-solving more intuitive.

Key Mathematical Concepts

The main focus of this chapter is on manipulating expressions and understanding their graphical representations. Through practice, you’ll learn to solve equations, interpret graphical data, and recognize patterns in numbers. The methods introduced here will not only help you work through problems but also enable you to see the broader picture of how mathematical structures work together.

Problem-Solving Strategies

To ensure success in this section, it’s important to develop a structured approach to problem-solving. Breaking down complex challenges into simpler steps can significantly enhance your ability to find solutions. By practicing these strategies, you’ll gain confidence in your ability to approach new topics with clarity and precision.

Key Concepts in Algebra 1 Unit 4

This section introduces the fundamental principles that are crucial for mastering mathematical problem-solving. The focus is on understanding how variables interact within expressions and equations, as well as how these relationships can be represented and manipulated. Building a solid understanding of these concepts will lay the groundwork for tackling more complex challenges and improving overall mathematical reasoning.

One of the core elements covered is the process of solving linear equations and inequalities, including techniques for isolating variables and interpreting solutions. Additionally, you’ll explore how to graph equations and understand the significance of the slope and y-intercept in a visual context. Understanding these concepts in depth will allow you to approach problems from multiple angles and develop a more comprehensive approach to mathematics.

Understanding Linear Equations and Inequalities

Linear equations and inequalities form the foundation of many mathematical problems. These expressions involve variables that change in a predictable, consistent manner. To master these topics, it’s essential to understand how to manipulate these relationships and solve for the unknowns. By breaking down the steps, you’ll be able to approach these problems with confidence and clarity.

Solving Linear Equations

To solve linear equations, the goal is to isolate the variable on one side of the equation. This involves several key steps:

Combine like terms on each side of the equation.
Use addition, subtraction, multiplication, or division to simplify the equation.
Ensure the variable is isolated by performing inverse operations.

Once the variable is isolated, the solution can be found by simplifying the resulting equation. This process is crucial for solving real-world problems where relationships between different quantities need to be determined.

Working with Inequalities

Inequalities are similar to equations, but they describe a range of possible solutions rather than a single answer. When working with inequalities, it’s important to:

Recognize the inequality symbols: >, <, ≥, and ≤.
Apply similar steps as with equations, but remember that multiplying or dividing by a negative number reverses the inequality symbol.
Graph the inequality on a number line to visualize the range of solutions.

Understanding these concepts is vital for tackling more complex problems involving relationships between multiple variables.

Steps to Solve Algebraic Problems

Solving mathematical problems requires a structured approach that helps you organize your thoughts and work through each challenge systematically. The following steps can guide you through the process of finding solutions to a wide range of expressions and equations. Mastering these methods will improve your efficiency and confidence in problem-solving.

Step-by-Step Process

Follow these key steps when approaching any problem:

Read the problem carefully: Understand what is being asked and identify the known and unknown variables.
Rewrite the equation: Express the problem in a clear, mathematical form, ensuring all terms are correctly placed.
Simplify the equation: Combine like terms and eliminate any unnecessary operations.
Apply inverse operations: Use addition, subtraction, multiplication, or division to isolate the variable on one side of the equation.
Check the solution: Substitute the value back into the original equation to verify that it satisfies all conditions.

Helpful Tips for Success

Be patient and take your time to ensure all steps are followed correctly.
Double-check your calculations to avoid simple errors.
If you’re stuck, try breaking down the problem into smaller, more manageable parts.

By following these steps, you’ll improve your ability to solve even the most challenging mathematical problems efficiently and accurately.

Exploring Graphing Techniques in Algebra

Graphing is an essential skill that allows you to visually represent relationships between variables. By plotting equations and inequalities on a graph, you can better understand how different elements interact and gain insights into the behavior of mathematical systems. Mastering graphing techniques is key to solving many problems and interpreting results accurately.

Basic Graphing Techniques

To begin graphing, it’s important to understand the key components of a graph and how to plot points based on the given information. Here are the general steps to follow:

Identify the equation: Make sure the equation is in a form that can be easily graphed, such as slope-intercept form (y = mx + b).
Plot the y-intercept: Locate the point where the line crosses the y-axis, represented by the value of b.
Determine the slope: Use the slope (m) to find other points on the graph by moving from the y-intercept.
Draw the line: Connect the points to form a straight line, extending it in both directions.

Graphing Inequalities

Graphing inequalities follows a similar process, but with a few key differences. To graph an inequality:

Plot the boundary line: First, graph the related equation as if it were an equality. Use a dashed line if the inequality is strict (e.g., > or
Shade the correct region: After plotting the boundary, shade the area that satisfies the inequality. Use a test point (like (0,0)) to determine which side to shade.

These graphing techniques not only help visualize the relationships between variables but also provide a powerful tool for analyzing the solutions to different mathematical scenarios.

How to Tackle Word Problems Efficiently

Word problems can often seem overwhelming due to the abundance of information and the need to translate a real-world scenario into a mathematical format. However, with a structured approach, you can break down these problems into manageable steps. The key is to carefully read the problem, identify the important details, and methodically apply mathematical principles to find a solution.

Steps to Solve Word Problems

Follow these steps to approach word problems effectively:

Read the problem thoroughly: Make sure you understand what is being asked. Highlight or underline key details such as quantities, units, and relationships between them.
Identify the unknown: Determine what you’re solving for and introduce a variable if necessary.
Translate into a mathematical expression: Convert the words into an equation or inequality based on the relationships described in the problem.
Solve the equation: Use appropriate techniques to find the value of the unknown. Ensure you follow proper procedures for simplifying and solving.
Check your solution: Verify that your answer makes sense in the context of the problem and that all conditions are met.

Tips for Success

Take your time reading through the problem and avoid rushing.
Don’t be afraid to break the problem down into smaller, easier parts.
Draw diagrams or use tables to visualize relationships and organize information if necessary.
Practice regularly to improve your confidence and speed in solving word problems.

By following these strategies and practicing consistently, you’ll find that word problems become much easier to manage and solve efficiently.

Essential Strategies for Unit 4 Success

Achieving success in this section requires a combination of solid preparation, effective problem-solving techniques, and consistent practice. By mastering key concepts and developing efficient strategies, you’ll be able to tackle the material with confidence and precision. Here are some essential strategies to help you perform well and deepen your understanding of the topics covered.

Focus on Core Concepts

To excel, it’s important to build a strong foundation by focusing on the core principles. Make sure you thoroughly understand the following:

Identifying and solving equations: Ensure you are comfortable with solving both simple and complex equations.
Graphing relationships: Practice plotting equations and interpreting their graphical representations.
Applying inequalities: Understand how to work with and graph inequalities in a variety of contexts.

Effective Problem-Solving Techniques

In addition to understanding the concepts, apply these problem-solving techniques to improve your accuracy and speed:

Break problems down: Start with simple steps and gradually build towards more complex solutions.
Use diagrams or graphs: Visualizing relationships can often make it easier to solve a problem.
Check your work: After solving, always review your solutions to ensure they make sense in the context of the problem.

Regular Practice and Review

Work through practice problems to reinforce concepts and improve your problem-solving skills.
Review any mistakes and make sure you understand why they occurred.
Regularly revisit difficult concepts to strengthen your understanding and recall.

By applying these strategies, you’ll not only improve your skills but also gain confidence in tackling more challenging material. Consistent effort and a clear focus on the fundamental techniques are key to achieving success in this section.

Common Mistakes to Avoid in Algebra

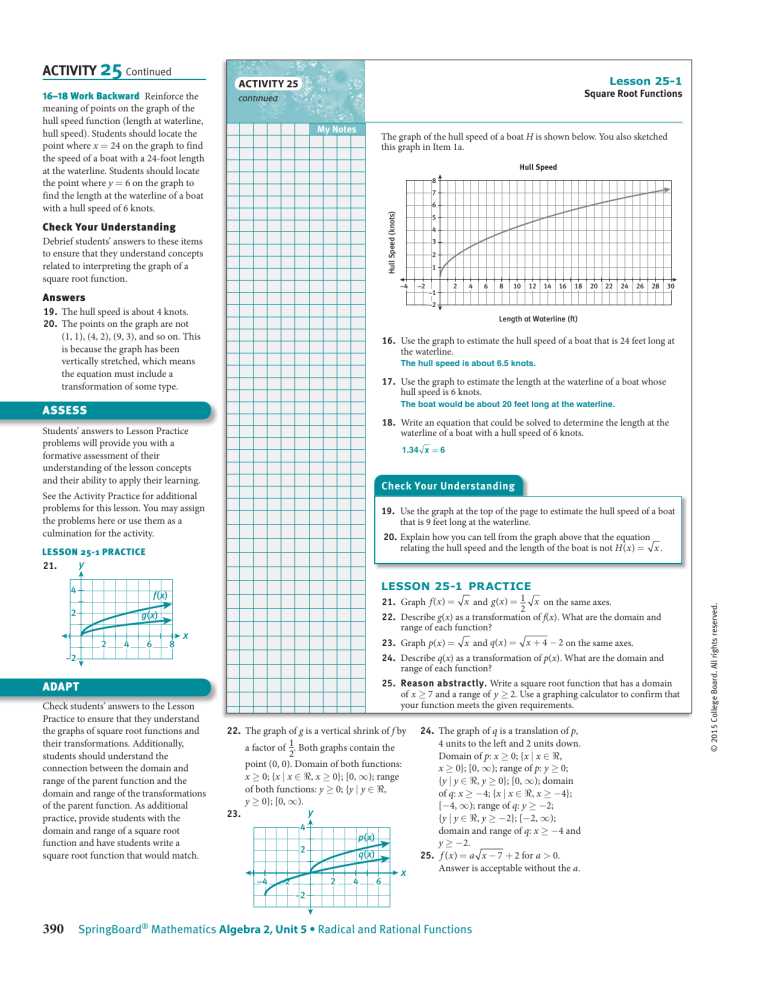
While working through mathematical problems, it’s easy to make errors that can lead to incorrect results. Recognizing and avoiding these common mistakes can help ensure accuracy and save time. Many of these errors are due to misunderstandings of key concepts or simple oversights. By being aware of these pitfalls, you can refine your approach and improve your problem-solving skills.

Here are some of the most frequent mistakes to watch out for:

Misunderstanding operation order: Failing to follow the correct order of operations (PEMDAS) often leads to incorrect results. Always handle parentheses, exponents, multiplication/division, and addition/subtraction in the correct sequence.
Incorrectly distributing terms: When multiplying a term by a sum or difference, remember to distribute each term correctly. For example, when solving a(b + c), ensure you multiply both a by b and a by c rather than applying the operation to just one part of the expression.
Sign errors: One of the most common mistakes is incorrectly handling positive and negative signs. Be especially careful when adding or subtracting negative numbers, as a small oversight can lead to a completely wrong answer.
Ignoring variable isolation: When solving for an unknown, always isolate the variable properly. Forgetting to do so can make it difficult to arrive at the correct solution.
Not checking the solution: After solving, it’s crucial to substitute the solution back into the original equation to verify that it satisfies all conditions. Failing to do so can leave small errors unnoticed.

By avoiding these mistakes and carefully working through each problem, you’ll be able to increase your accuracy and confidence in solving mathematical expressions.

Tips for Practicing Algebra 1 Skills

To master mathematical concepts, consistent practice is essential. Working through a variety of problems helps reinforce what you’ve learned and builds your problem-solving abilities. By following a structured approach and staying committed to improving, you’ll gradually become more proficient and confident in handling complex challenges.

Here are some effective tips to make your practice sessions more productive:

Start with the basics: Before diving into more challenging problems, ensure you have a solid understanding of foundational concepts. Revisiting simpler exercises will help you reinforce your skills and build confidence.
Practice regularly: Consistency is key to improvement. Set aside time each day or week to work through practice problems. This will help you retain what you’ve learned and apply it more effectively.
Work on a variety of problems: Don’t just stick to one type of problem. Expose yourself to a range of exercises that cover different concepts and difficulty levels. This will help you develop a broader skill set and adapt to different challenges.
Break problems into smaller steps: If you encounter a difficult problem, break it down into smaller, manageable parts. This makes it easier to identify your next steps and ensures that you stay on track.
Check your work: After solving each problem, review your solution to make sure it’s correct. This habit will help you catch any mistakes and improve your accuracy.
Seek feedback: If you’re unsure about a solution or a concept, ask for help. Whether from a teacher, a tutor, or an online resource, getting feedback will clarify misunderstandings and improve your learning.

By following these tips and practicing regularly, you’ll not only reinforce what you’ve already learned, but also enhance your ability to approach and solve more complex problems in the future.

Using the Answer Key Effectively

Having access to solutions can be incredibly helpful in your learning process, but it’s important to use them strategically to maximize their value. Simply copying answers without understanding the steps behind them won’t help you improve. Instead, view the solutions as a tool to guide your understanding and reinforce the concepts you’ve learned.

Here are some effective ways to utilize the provided solutions:

Understand each step: Rather than just looking at the final result, carefully analyze each step in the solution. This will help you learn the process and identify where you may have made a mistake.
Compare your work: After attempting a problem, compare your solution with the provided one. Look for differences and try to pinpoint where your approach diverged.
Focus on patterns: As you go through multiple problems, take note of any patterns or strategies that appear. This will allow you to recognize the best methods to apply in future problems.
Identify common mistakes: If you’ve made errors, use the solutions to understand why the mistake occurred. This can help you avoid repeating the same mistakes in the future.
Rework difficult problems: If a particular problem stumps you, try to solve it again after reviewing the solution. This will reinforce your understanding and help you internalize the steps for next time.

By using solutions as a learning tool, rather than just a way to check your work, you can deepen your understanding and improve your problem-solving abilities over time. Make sure to actively engage with the material and learn from each solution to achieve the best results.

Breaking Down Algebraic Expressions

Understanding complex expressions requires breaking them down into simpler parts. By isolating individual components, you can better comprehend how each term interacts with the others. This process is essential for solving equations and manipulating expressions effectively. It’s not just about finding the right answer, but also about understanding the structure and logic behind each step.

When dealing with expressions, the key is to identify terms, coefficients, variables, and operations. Each part of the expression plays a specific role in the overall equation, and breaking it down helps you focus on one aspect at a time.

Identifying Components of an Expression

The first step in simplifying expressions is recognizing their components. Here’s a breakdown of a simple example:

Component	Description	Example
Variable	Represents an unknown value	x, y
Coefficient	Number multiplying the variable	3 in 3x
Constant	A number without a variable	5 in 3x + 5
Operation	The mathematical action performed between terms	+ (addition), – (subtraction), * (multiplication), ÷ (division)

Simplifying Expressions

Once you’ve identified the individual parts, the next step is to simplify the expression by combining like terms or applying distributive properties. For example, in the expression 3x + 5x, the like terms 3x and 5x can be combined to form 8x.

Breaking down expressions into smaller parts allows you to approach each component methodically. This helps you identify patterns, reduce complexity, and make the entire process of solving equations more manageable.

Analyzing Functions in Algebra 1

To solve problems involving relationships between variables, it’s crucial to understand how different quantities interact within a given function. A function defines a specific relationship where each input corresponds to exactly one output. By analyzing these relationships, you can identify patterns, make predictions, and solve equations more efficiently. This process involves breaking down the function’s components and interpreting their meaning within the context of the problem.

Key Concepts in Function Analysis

When analyzing functions, it’s important to focus on several core concepts that help clarify the behavior and structure of the relationship:

Domain: The set of all possible input values (independent variable) for the function.
Range: The set of all possible output values (dependent variable) produced by the function.
Intercepts: Points where the function crosses the axes, often providing important insights into the function’s behavior.
Slope: The rate of change of the output with respect to the input, which helps in understanding the function’s steepness or direction.
Behavior: The way the function behaves as the input values increase or decrease (e.g., increasing, decreasing, or remaining constant).

Steps to Analyze a Function

To effectively analyze a function, follow these steps:

Identify the type of function: Determine if the function is linear, quadratic, e

Solving Systems of Equations

When dealing with multiple relationships between variables, solving systems of equations becomes essential. A system consists of two or more equations that share common variables. The goal is to find the values of these variables that satisfy all the equations in the system simultaneously. This can be achieved using various methods, each suited to different types of problems. By solving systems efficiently, you can uncover the intersection points of the equations, which represent the solutions to the system.

Methods for Solving Systems

springboard algebra 1 unit 4 answer key

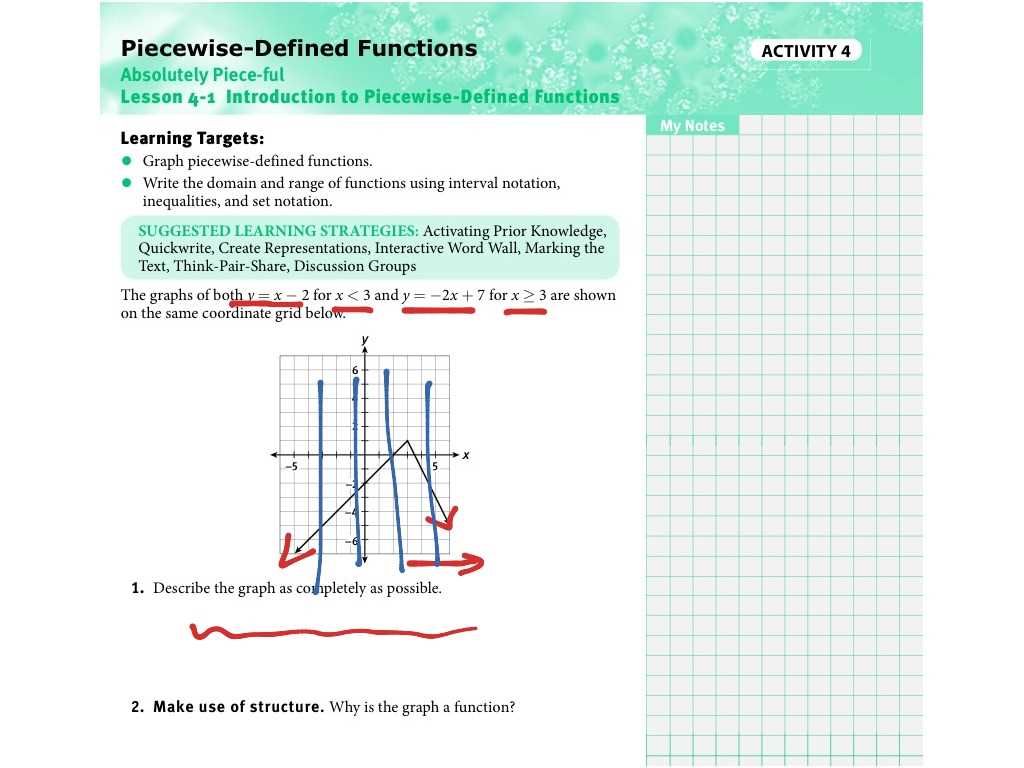
There are several approaches to solve systems of equations, depending on the complexity of the equations involved:

Graphing: This method involves plotting both equations on a graph and finding the point(s) where they intersect. The coordinates of the intersection point(s) are the solution(s) to the system.
Substitution: This technique is useful when one equation can be easily solved for one variable. Substitute this expression into the second equation to find the value of the other variable.
Elimination: Also known as the addition method, this approach involves adding or subtracting the equations to eliminate one variable, making it easier to solve for the other variable.

Step-by-Step Approach

To solve a system, follow these steps:

Choose a method: Based on the structure of the equations, decide whether graphing, substitution, or elimination is the most efficient approach.
Solve for one variable: If using substitution or elimination, isolate one variable and substitute or eliminate it in the other equation.
Solve for the second variable: After finding the value of the first variable, substitute it back into one of the original equations to find the second variable.
Check the solution: Substitute both values back into the original equations to verify they satisfy both equations. If they do, the solution is correct.

By mastering these methods, you can effectively solve systems of equations and find the correct solutions that satisfy all the conditions of the problem.

Applying Algebra to Real-Life Scenarios

Mathematics is not just confined to theoretical concepts; it plays a significant role in solving practical problems we encounter every day. Using mathematical reasoning, we can model and solve real-world situations by translating them into mathematical expressions or equations. This helps in making informed decisions, whether you’re calculating a budget, determining travel times, or analyzing the growth of investments. The ability to apply mathematical techniques effectively can make everyday tasks easier and more manageable.

One common example of applying mathematical reasoning is calculating expenses or profits. Whether managing household expenses or planning for a business, understanding how to manipulate variables and solve for unknowns can provide insights into budgeting and financial planning.

Scenario	Equation	Solution
Budgeting for a monthly expense	Income – Expenses = Savings	Determine how much you can save based on the difference between income and expenses.
Calculating the distance traveled	Distance = Speed × Time	Find how far you travel by multiplying speed by the time spent traveling.
Determining the cost of groceries	Cost = Price per item × Quantity	Multiply the price of each item by the number of items to calculate the total cost.

By framing problems in this way, we can simplify complex tasks and better understand the variables at play. Whether you’re managing finances, planning a trip, or making business decisions, applying mathematical principles can enhance your ability to navigate various situations efficiently.

How to Check Your Algebraic Solutions

Ensuring the accuracy of your solutions is a crucial part of solving mathematical problems. Once you’ve found a potential solution to an equation, it’s important to verify whether it truly satisfies the original problem. There are several methods you can use to check your work and ensure that you haven’t made any errors during the process. Verification not only boosts your confidence in the correctness of your solution but also helps in identifying any mistakes early on.

One of the most common techniques is substituting your solution back into the original equation. If both sides of the equation are equal after substitution, the solution is correct. If not, you’ll need to revisit your calculations.

Step	Description	Example
Substitution	Substitute the solution into the original equation and check if both sides are equal.	If the equation is 2x + 3 = 7, substitute x = 2: 2(2) + 3 = 7. Since both sides equal 7, the solution is correct.
Graphical Check	For linear equations, plot the equation on a graph and check if the solution lies on the line.	If the equation is y = 2x + 1, plot the points and verify that the solution fits the graph.
Use of Inverse Operations	Apply inverse operations to reverse the steps you took to solve the equation and check for consistency.	For x + 5 = 12, subtract 5 from both sides: x = 12 – 5 = 7. Verify by plugging x = 7 back into the original equation.

By using these methods, you can effectively verify the accuracy of your solutions. It’s a good habit to check your work before finalizing your answers, especially when solving complex problems or preparing for assessments.

Reviewing Key Formulas for Unit 4

In any mathematical study, understanding the essential formulas is crucial to solving problems efficiently. For the current topic, there are several fundamental equations that you’ll need to remember and apply in different scenarios. These formulas serve as the building blocks for tackling various types of problems, from solving for variables to working with different types of functions. Mastering these expressions will help you navigate through the material with greater ease and confidence.

Here’s a look at some of the most important formulas to remember:

Slope-Intercept Form: y = mx + b
- m represents the slope of the line, and b is the y-intercept, or where the line crosses the y-axis.
Point-Slope Form: y – y1 = m(x – x1)
- This formula allows you to write the equation of a line when you know the slope and a point on the line.
Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a
- Used to solve quadratic equations of the form ax² + bx + c = 0.
Standard Form of a Linear Equation: Ax + By = C
- This formula expresses linear equations in a way that is useful for determining the relationship between two variables.

By familiarizing yourself with these key formulas, you’ll be better equipped to solve problems effectively and accurately. Make sure to practice applying these formulas in various contexts, as this will reinforce your understanding and ensure that you’re prepared for more complex challenges ahead.