Grasping the essential principles behind physical characteristics is crucial for solving scientific problems. These concepts are vital not only in the classroom but also in real-world scenarios where accuracy in measurements is required. Being able to apply these principles effectively helps in determining various aspects of matter and its interactions in different environments.
In this section, we explore practical techniques to solve typical exercises related to the measurement of physical properties. Through detailed examples, we aim to clarify how to approach these types of challenges systematically, ensuring correct results. Mastering these skills will enhance your understanding of matter and its behavior under various conditions, enabling you to tackle even more complex problems with confidence.
Accurate problem-solving requires a solid foundation in key formulas and approaches, which we will break down and explain in simple terms. By the end of this guide, you will be equipped to handle such tasks efficiently, reinforcing your learning and building on essential scientific knowledge.
Volume and Density Worksheet Answer Key
In this section, we will walk through the steps involved in solving problems related to the measurement of physical properties, offering solutions to common challenges. By working through these examples, you’ll gain a deeper understanding of how to apply essential formulas to determine the mass-to-space ratio of objects and other related attributes. This guide will help you check your solutions and clarify any doubts that might arise while tackling similar exercises.
Each problem focuses on different aspects of calculating physical characteristics, including identifying the correct method for finding the value and understanding the necessary units. With each solved example, you will be able to verify your work and strengthen your grasp on these fundamental concepts. The goal is to empower you to solve complex problems with accuracy and confidence, ensuring you master key scientific principles.
Understanding the Basics of Volume and Density
To effectively solve problems related to physical characteristics, it’s essential to first grasp the basic concepts that underpin these measurements. These principles help us understand how objects interact with space and mass, which are fundamental to many scientific calculations. Mastering these foundational ideas is the first step toward solving more complex problems with precision.
Core Concepts in Measurement

Understanding the relationship between an object’s mass and the space it occupies is critical. This ratio is central to numerous scientific disciplines, from physics to chemistry. By learning how to measure this ratio accurately, you can determine essential properties that influence material behavior in various situations.
Units of Measurement
Various units are used to express these characteristics, and it’s important to know how to convert between them for accurate calculations. The most common units for mass and space include grams, kilograms, cubic centimeters, and liters. Below is a table summarizing some of these key units and their conversions:
| Quantity | Unit | Conversion |
|---|---|---|
| Mass | Grams (g), Kilograms (kg) | 1 kg = 1000 g |
| Space (Volume) | Cubic Centimeters (cm³), Liters (L) | 1 L = 1000 cm³ |
| Ratio (Mass to Space) | Grams per Cubic Centimeter (g/cm³) | 1 g/cm³ = 1000 kg/m³ |
With a solid understanding of these basic principles and units, you will be prepared to approach more complex calculations with confidence. This knowledge lays the groundwork for deeper exploration into material properties and their practical applications.
How to Calculate Volume and Density
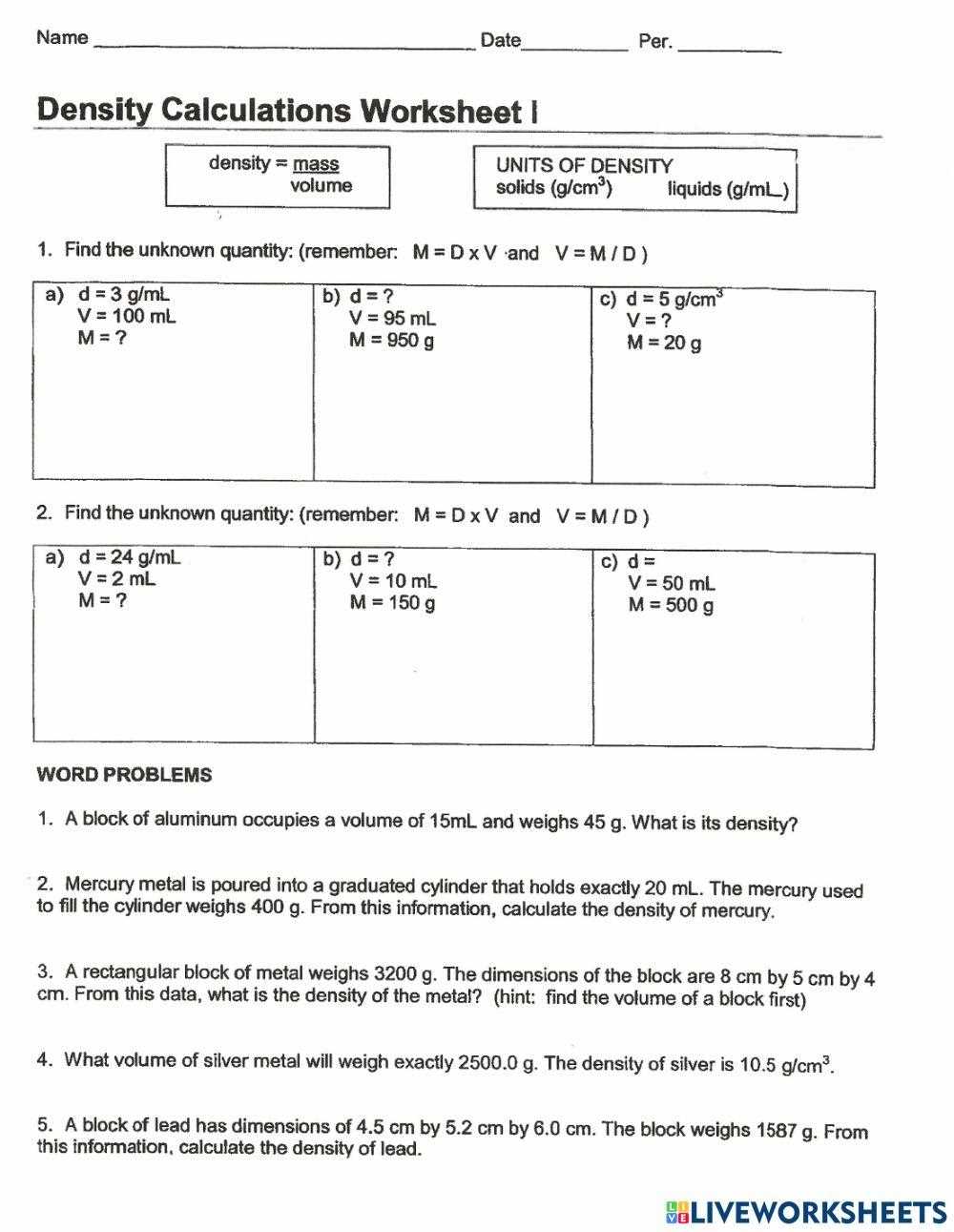
To solve problems related to the mass-to-space ratio of an object, it’s important to understand the methods used for calculating both mass and the space it occupies. These calculations are key in determining essential properties that describe the material behavior. The process involves using well-established formulas and understanding the units of measurement that correspond to each quantity.
To calculate these properties accurately, you need to know the formulas and the steps to apply them based on the given information. Below is a table outlining the common formulas used for these calculations:
| Property | Formula | Explanation |
|---|---|---|
| Space (Volume) | Length × Width × Height | This formula is used for rectangular objects, where you multiply the three dimensions to find the total space the object occupies. |
| Mass-to-Space Ratio | Mass ÷ Space | By dividing the mass of the object by the space it occupies, you can determine the ratio that describes its material composition. |
| Irregular Objects | Water Displacement Method | For irregularly shaped objects, you can use water displacement to measure space by observing the volume of water displaced when the object is submerged. |
Once you have the necessary measurements, simply apply the formulas to calculate the required values. It’s important to use consistent units throughout the process to ensure accuracy. By practicing these steps, you will be able to solve various problems effectively and understand the physical characteristics of different materials.
Common Mistakes in Volume and Density Problems

When solving problems related to the mass-to-space ratio, many learners encounter errors that can lead to incorrect results. These mistakes often arise from misinterpreting the problem, using wrong units, or applying incorrect formulas. Identifying and correcting these common errors is key to improving accuracy and understanding.
One frequent mistake is neglecting to convert units before performing calculations. For example, mixing grams with kilograms or cubic centimeters with liters can lead to significant errors in the final result. It is crucial to always check that the units match and, if necessary, convert them before proceeding.
Another common error is applying the wrong formula or using it incorrectly. For instance, using the wrong equation for irregularly shaped objects instead of the water displacement method can skew results. It’s essential to select the appropriate method based on the shape and properties of the object being measured.
Inaccurate measurements are also a major source of mistakes. Using imprecise tools or misreading values on measuring instruments can lead to slight, but impactful, errors. Always ensure that you measure with care and double-check your values to avoid these pitfalls.
By being mindful of these common mistakes and following the proper steps carefully, you can significantly improve your ability to solve such problems accurately and efficiently.
Units of Measurement for Volume and Density
Understanding the correct units of measurement is essential when working with physical properties, as using the wrong units can lead to inaccurate calculations. Different units are used to express the space occupied by an object and the mass it contains. Being familiar with these units ensures that calculations are performed correctly and consistently across various problems.
Common Units for Mass

Mass is often measured in units such as grams, kilograms, or pounds, depending on the scale and context of the measurement. Here are some of the most commonly used units:
- Grams (g): Typically used for measuring small amounts of mass.
- Kilograms (kg): Used for larger masses, especially in scientific and everyday measurements.
- Pounds (lbs): A unit commonly used in the United States for measuring mass or weight.
Common Units for Space
The space an object occupies is measured using various units. These include both metric and imperial units, depending on the system being used. Below are some of the key units:
- Cubic centimeters (cm³): Used for measuring smaller volumes, particularly in scientific contexts.
- Liters (L): A standard unit for measuring liquid volumes, commonly used in everyday situations.
- Cubic meters (m³): Typically used for measuring large volumes, especially in industrial and scientific applications.
Understanding Unit Conversions
When working with different units, it’s crucial to convert between them when necessary. For instance, to convert grams to kilograms, you must divide by 1000, while to convert from cubic centimeters to liters, you divide by 1000 as well. Ensuring proper conversions will help maintain the accuracy of your calculations.
- 1 kg = 1000 g
- 1 L = 1000 cm³
- 1 m³ = 1000 L
Being familiar with these units and their conversions is essential for solving problems related to physical properties accurately. Always double-check the units you are using and make sure they are consistent throughout your calculations.
Real-World Applications of Volume and Density
The concepts of space occupation and mass-to-space ratio are not just theoretical; they play an important role in many practical fields. These principles help us understand how materials behave under different conditions and are essential in industries ranging from engineering to medicine. By applying these measurements, professionals can make informed decisions that affect everything from material selection to product design.
In engineering, understanding these principles is crucial for designing structures and machines. For example, architects need to calculate how much space a material will occupy to ensure the stability and safety of a building. Similarly, engineers use these concepts when selecting materials for various applications, ensuring that the materials are appropriate for their intended purpose, whether for lightweight structures or for items requiring heavy-duty strength.
In the transportation industry, these measurements are used to improve fuel efficiency and reduce waste. By knowing the mass-to-space ratio of materials used in vehicles, manufacturers can optimize design, reduce the weight of cars, and improve fuel consumption. This also extends to shipping and logistics, where understanding the properties of goods ensures that transportation methods are cost-effective and efficient.
Another area where these calculations are applied is in product development. In industries such as pharmaceuticals, food production, or cosmetics, understanding how ingredients interact in terms of their mass and the space they occupy can lead to better formulations and packaging solutions. This ensures products are both effective and efficient in their usage and storage.
Ultimately, the practical applications of these principles are vast and varied. From creating sustainable materials to optimizing designs and ensuring safety, the ability to calculate these properties accurately has real-world implications that go beyond academic exercises.
Step-by-Step Guide to Solving Problems
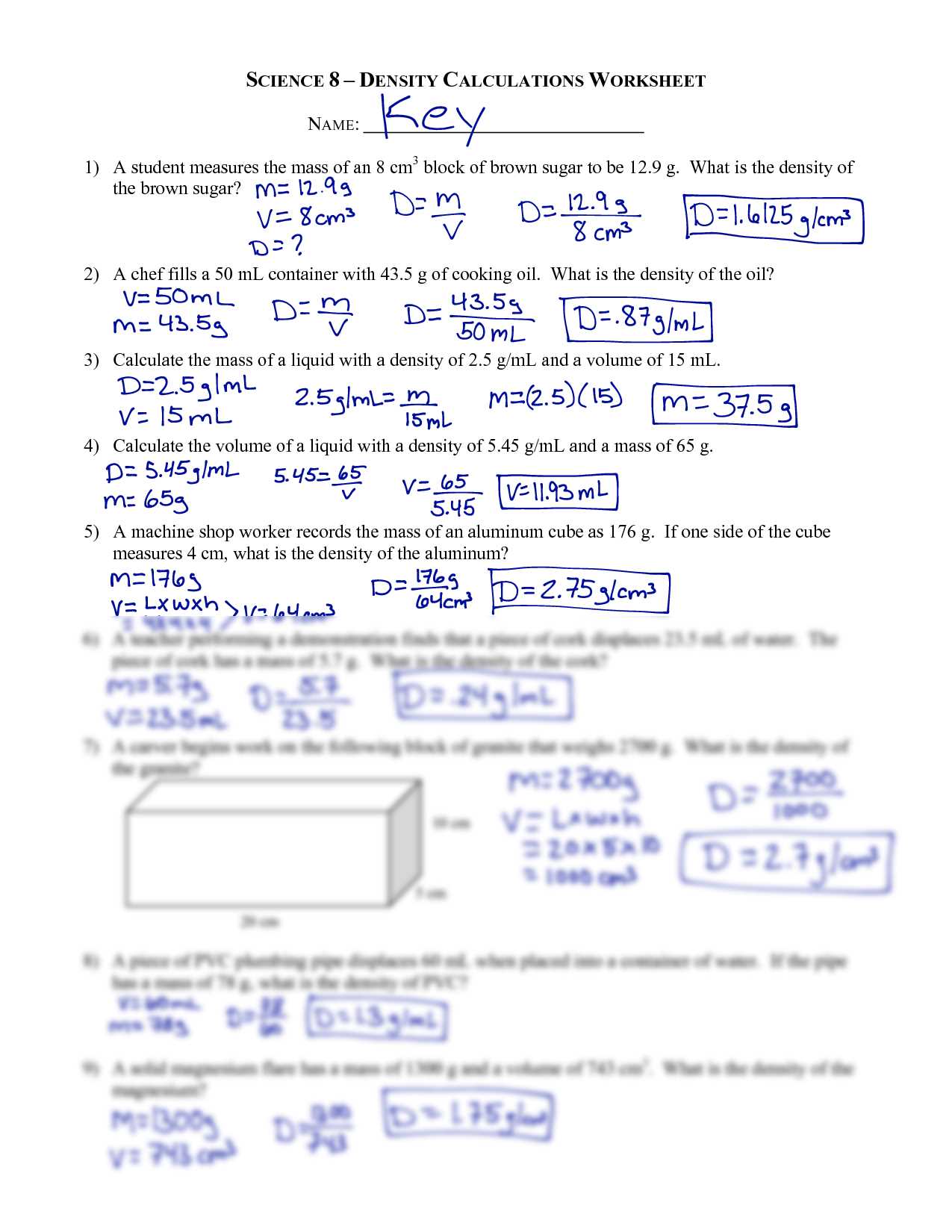
When tackling problems related to physical properties, following a systematic approach ensures accuracy and clarity in your calculations. By breaking down each task into smaller, manageable steps, you can avoid common mistakes and arrive at the correct solution more efficiently. Here is a simple guide to help you solve these types of problems with confidence.
- Understand the Problem: Read the problem carefully to determine what information is given and what is being asked. Identify the key quantities involved, such as mass, space, or related properties.
- Identify the Formula: Based on the given information, choose the appropriate equation. Make sure the formula matches the type of problem you’re solving, whether it’s for regular or irregular shapes.
- Check Units: Ensure that all measurements are in compatible units. If necessary, convert units before proceeding with the calculation. For example, convert grams to kilograms or cubic centimeters to liters.
- Plug in Values: Substitute the known values into the equation. Be precise with your numbers to avoid errors later on.
- Calculate: Perform the necessary arithmetic operations to solve the equation. Double-check each step to avoid calculation mistakes.
- Interpret the Result: Once you have a result, make sure it makes sense in the context of the problem. Check the magnitude of the result to see if it’s realistic given the values you started with.
- Verify Units: After solving, ensure the final units are correct. If they aren’t, revisit your unit conversions and calculations.
By following this step-by-step method, you can tackle a wide variety of problems with confidence, knowing that you’ve addressed all the necessary aspects for accurate results.
Importance of Accurate Measurements
In scientific calculations, precision plays a crucial role in obtaining reliable results. Whether you’re measuring the mass of an object, the space it occupies, or the relationship between the two, accurate measurements ensure that your conclusions are correct and applicable. Small errors can lead to significant discrepancies, affecting the validity of your findings in real-world applications.
Why Precision Matters

Accurate measurements are essential for several reasons. They provide a foundation for calculations, enable consistency in experiments, and ensure safety in practical scenarios. Without precise values, even the most well-thought-out plans can fail to achieve their intended outcomes.
- Reliability: Precise measurements allow others to replicate experiments or calculations with confidence, leading to consistent results.
- Cost-effectiveness: In industries like manufacturing, using incorrect measurements can result in wasted materials or products that don’t meet quality standards, leading to higher costs.
- Safety: In fields like engineering or medicine, accurate measurements are necessary to ensure that designs or treatments are safe and effective.
Common Sources of Error
While accurate measurements are crucial, several factors can introduce errors. Understanding these sources of inaccuracy is key to improving your methods and reducing mistakes:
- Inaccurate Instruments: Low-quality or poorly calibrated tools can give incorrect readings.
- Human Error: Misreading instruments or miscalculating values is common and can easily distort results.
- Environmental Factors: Changes in temperature, pressure, or humidity can affect measurements, especially in sensitive calculations.
Ensuring precision in all steps of measurement and calculation will yield reliable results that can be trusted for further analysis and practical use.
Key Formulas for Volume and Density
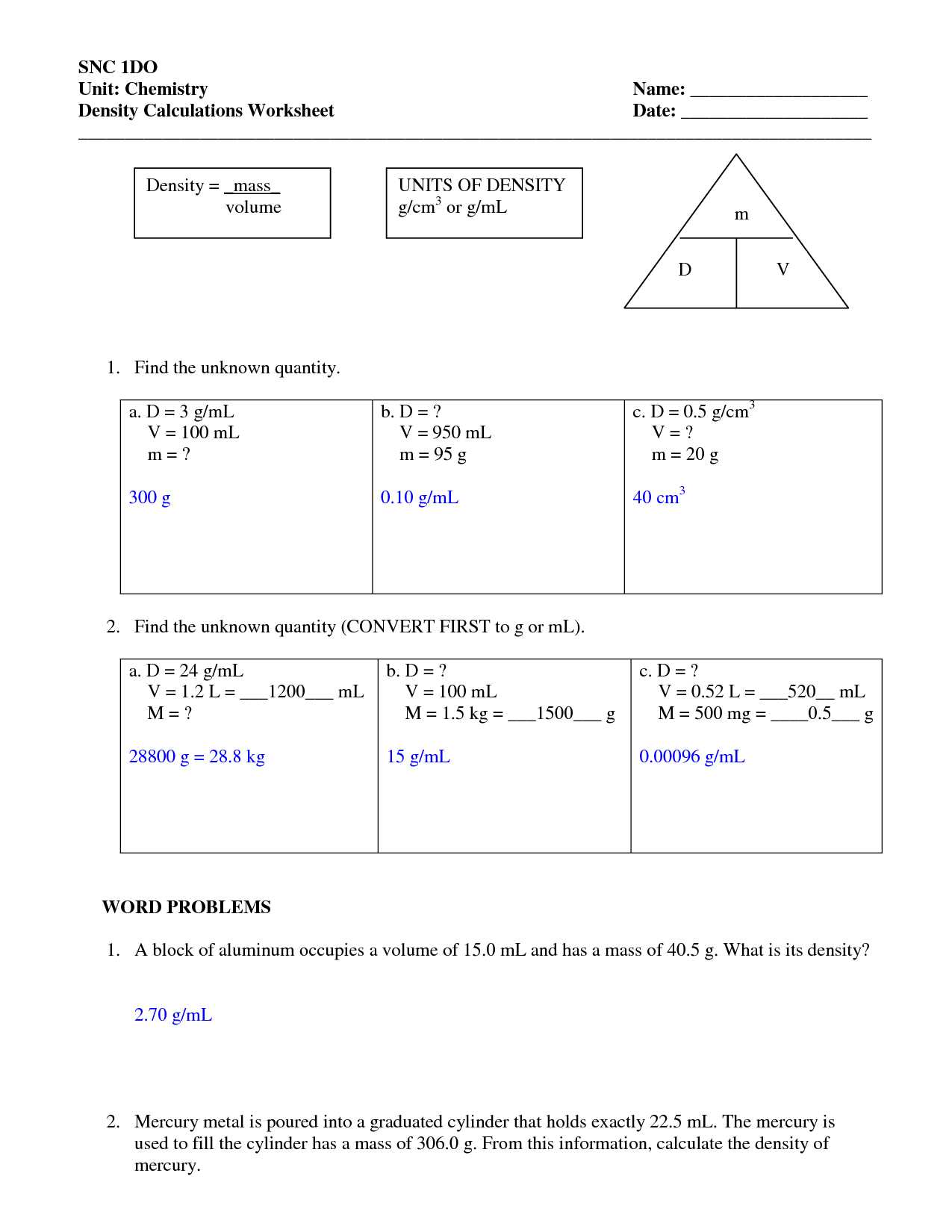
In any scientific calculation related to physical properties, knowing the right formulas is crucial. These formulas allow you to relate different characteristics of substances, such as their mass, space occupation, and ratio between them. Understanding the fundamental equations is the first step in solving problems accurately and efficiently.
Essential Formulas for Space Occupation
When working with objects and their occupied space, different shapes require distinct formulas. Below are some of the most important equations for determining how much space an object takes up:
- Rectangular Objects: Length × Width × Height
- Cylindrical Objects: π × Radius² × Height
- Spherical Objects: (4/3) × π × Radius³
Formulas for Mass-to-Space Ratio
Once the space occupied by an object is calculated, the next step is to determine its mass-to-space ratio, which is commonly referred to as the material’s characteristics. This helps in comparing the compactness of different materials.
- Basic Ratio Formula: Mass ÷ Space Occupied
- For Irregular Objects: Water Displacement Method: Mass of Displaced Water ÷ Volume of Displaced Water
By applying these essential formulas, you can easily calculate important properties for a wide range of objects and substances. It is important to always use the correct units and perform unit conversions when necessary to ensure accurate results.
How to Check Your Answers for Accuracy
Verifying the correctness of your results is a critical part of problem-solving. It’s easy to make small mistakes during calculations, but a systematic approach to checking can help ensure that your answers are accurate. Here are several strategies you can use to confirm that your results are reliable and error-free.
- Recheck Your Calculations: Carefully go through each step of your process again. Look for arithmetic mistakes, such as adding or multiplying incorrectly, or errors in applying formulas.
- Check Units: Ensure that the units in your final result match the expected units for the type of calculation you’re performing. Convert units where necessary to maintain consistency.
- Estimate Reasonableness: Take a moment to consider whether the result makes sense given the values you started with. If the answer seems too large or too small, revisit your work.
- Use Alternative Methods: If possible, try solving the problem using a different approach or formula. If the results match, it’s likely your initial calculations were correct.
- Ask for a Second Opinion: Don’t hesitate to ask someone else to look over your work. A fresh set of eyes can often spot mistakes you might have missed.
By following these strategies, you can confidently verify your results and ensure that your answers are accurate. Accuracy is essential, especially when working with scientific data, so taking the time to check your work is always worthwhile.
Exploring Different Methods for Volume Calculation
There are various techniques available to calculate the space occupied by objects, each suited to different shapes and conditions. The method you choose depends on the nature of the object you are measuring. Understanding these approaches allows for greater accuracy and versatility when solving problems in physical sciences or everyday situations.
- Geometric Formulas: For regular shapes like cubes, spheres, or cylinders, the most straightforward method involves applying specific mathematical formulas. These equations are derived from the geometric properties of the shape.
- Water Displacement: For irregularly shaped objects, the water displacement method is often used. By submerging an object in a container of water, you can measure the volume of displaced liquid, which corresponds to the object’s space.
- Approximation Method: When precise measurements aren’t required, approximating the shape and using nearby geometric formulas can provide a quick estimate. This method is particularly useful for objects that are not easily categorized into a single geometric shape.
- Integration Techniques: In more advanced applications, such as in calculus, integration can be used to calculate the space occupied by complex objects, especially when the shape has curves or irregular surfaces.
By selecting the appropriate method for the object at hand, you can ensure that your calculation is both accurate and efficient. Whether you are working with simple geometric shapes or more complicated forms, understanding the variety of techniques available is key to mastering space measurement.
Interactive Volume and Density Exercises

Hands-on practice is an effective way to deepen your understanding of the relationship between mass, space, and the properties of materials. Interactive exercises allow you to engage with the concepts in a dynamic way, enhancing both comprehension and retention. These activities can be tailored to different levels of difficulty, providing an opportunity to explore various aspects of scientific measurements.
- Online Simulations: Many websites offer interactive tools where you can manipulate objects, change their dimensions, and observe the effects on their properties. These simulations help visualize abstract concepts and encourage experimentation.
- Interactive Quizzes: Quizzes that require inputting values or selecting the correct method to calculate certain properties can offer instant feedback. This immediate response helps reinforce the correct application of formulas and techniques.
- Virtual Lab Activities: Some platforms provide virtual lab setups where users can conduct experiments, measure materials, and analyze the data. These exercises mimic real-world scenarios, making the learning process more engaging.
- Problem-Solving Scenarios: Solving practical problems based on real-life situations allows you to apply theoretical knowledge. These exercises may include challenges like determining the amount of material needed for a project or calculating the properties of unknown substances.
By using interactive exercises, learners can experiment with different scenarios, test their understanding, and gain confidence in solving complex problems. These tools are especially valuable for students and professionals who want to reinforce their knowledge through applied practice.
How to Approach Complex Density Problems

When faced with complicated problems involving the relationship between mass and space, a structured approach is crucial for clarity and accuracy. These challenges often require multiple steps, careful attention to detail, and a solid understanding of both theoretical concepts and practical applications. Breaking down the problem into manageable parts will help you tackle it more effectively.
Step 1: Understand the Problem
The first step is to carefully read the problem and identify the given values. Make sure you know which measurements are provided and which ones need to be calculated. Clarifying the objective of the problem will guide you toward the appropriate method for solving it.
Step 2: Identify the Relevant Formulas
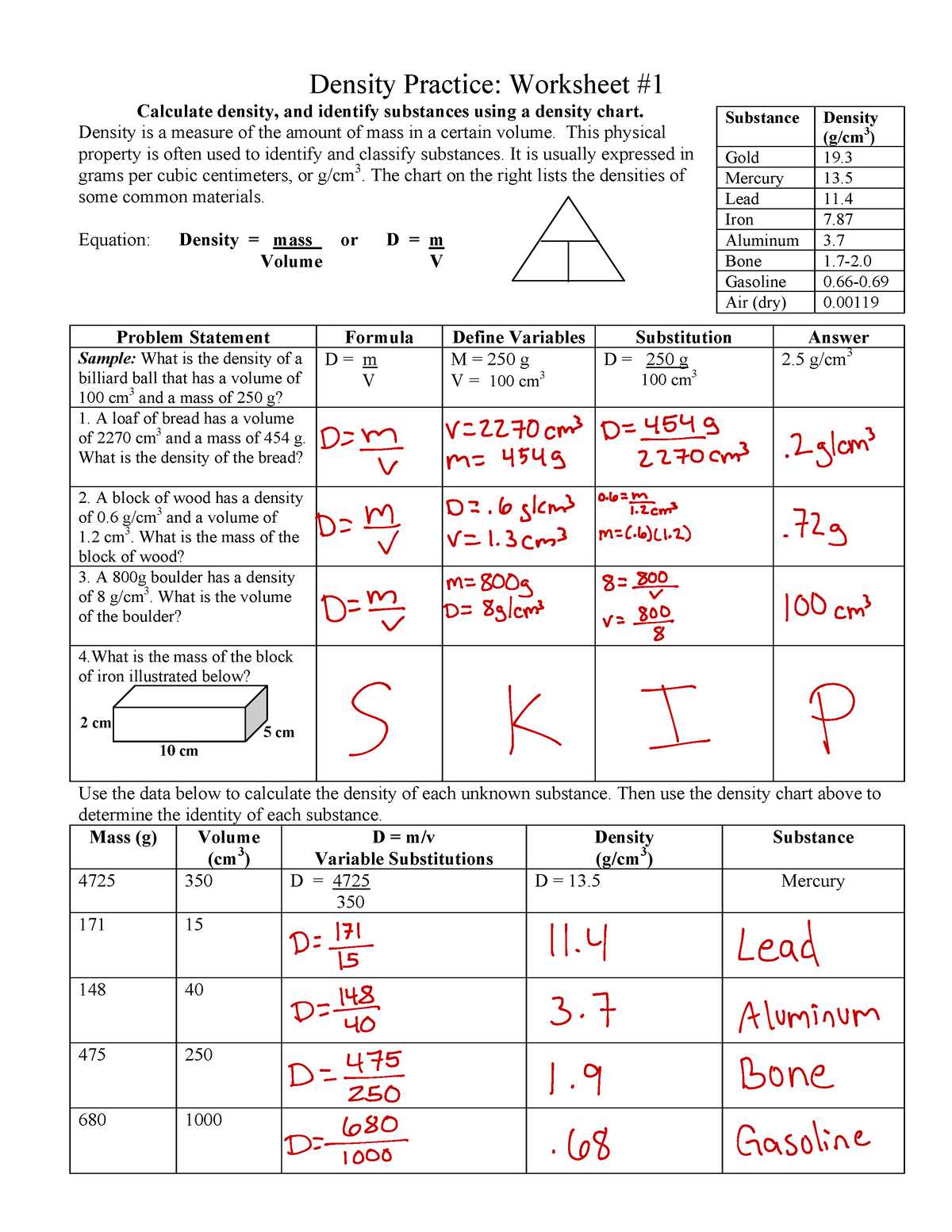
Once you understand the problem, determine which formulas are needed to solve it. Depending on the given data, you may need to use equations that relate mass, space, and the material’s characteristics. Remember that some problems may require modifications to standard formulas or even the combination of different methods.
- Isolate Variables: If you’re dealing with a formula that involves multiple variables, isolate the unknown variable before solving for it.
- Check Units: Ensure all measurements are in the correct units before plugging them into the formula. Converting units is often necessary for consistency.
- Calculate Step by Step: Break down the calculations into smaller steps, solving one part at a time to avoid mistakes.
By following these steps, you can systematically approach complex problems and find accurate solutions. Don’t rush through the process; take the time to check each calculation and ensure that all steps are logically connected.
Practical Tips for Solving Worksheet Questions
When tackling problems related to material properties, effective problem-solving strategies can make a significant difference in achieving accurate results. By staying organized, following clear steps, and paying attention to the details, you can improve both speed and precision in solving these types of exercises. Here are some useful approaches to help you navigate through them more efficiently.
Step 1: Break Down the Problem
The first step in solving any challenging exercise is to understand what is being asked. Take the time to read the problem thoroughly and break it down into smaller, more manageable parts. Identify what information is provided, what needs to be calculated, and what formulas might be relevant. This will help you organize your approach and ensure you don’t miss any critical steps.
Step 2: Use Tables for Organization
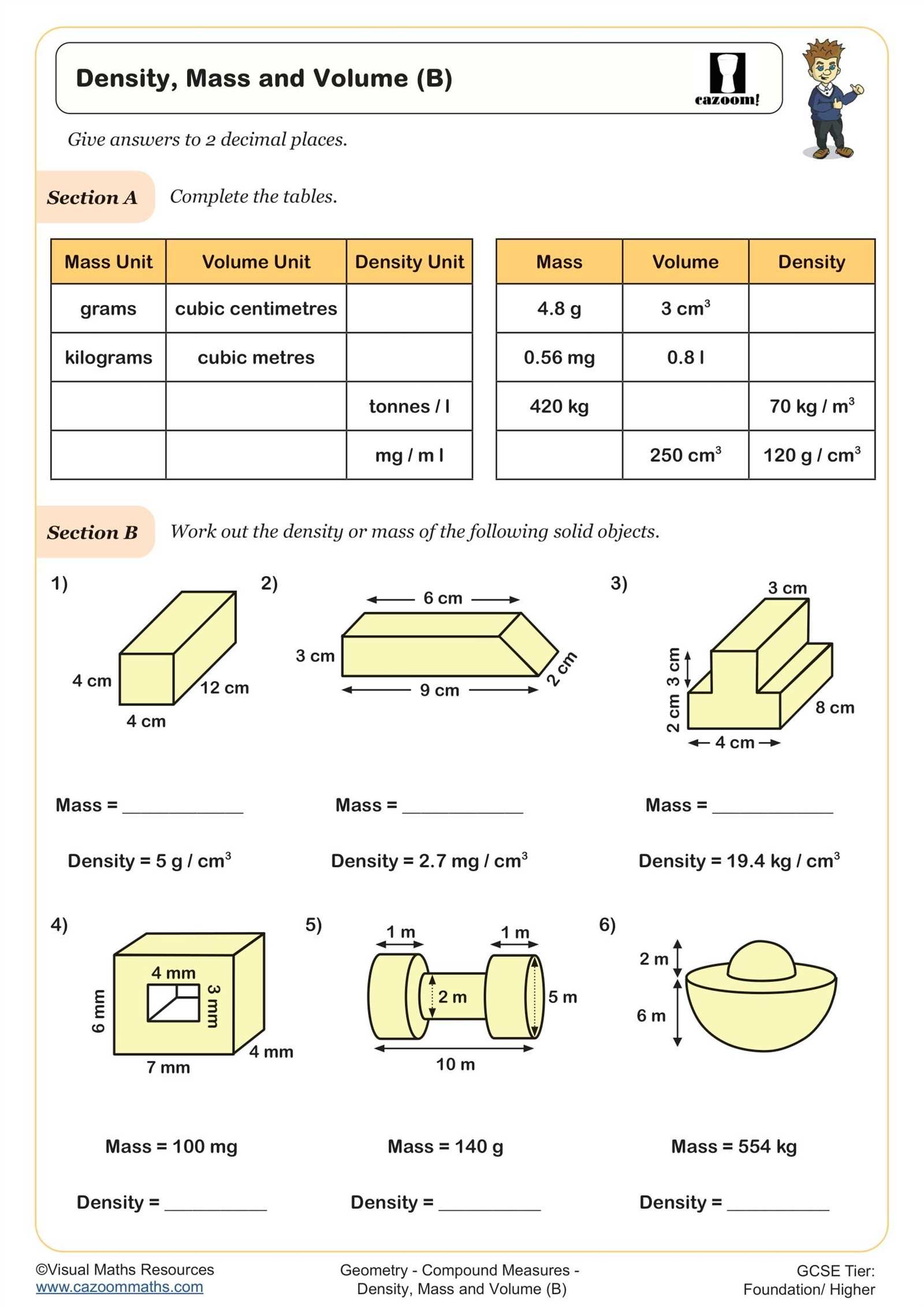
Using tables can significantly improve your ability to organize the given data and calculated values in a clear manner. Here’s an example of how a table can be helpful:
| Parameter | Value | Units |
|---|---|---|
| Mass | 50 | kg |
| Space | 10 | m³ |
| Material Property | 5 | kg/m³ |
By clearly listing all relevant parameters and their units, tables help to minimize confusion and ensure that no values are overlooked. This method also aids in cross-checking the calculations later on.
Step 3: Double-Check Your Units
One common mistake when solving these types of problems is overlooking the units. Always ensure that the units of measurement are consistent throughout your calculations. If necessary, convert units before performing any calculations to avoid errors in the final result.
By following these steps–breaking down the problem, organizing data with tables, and checking units–you can approach problems with confidence, reducing mistakes and improving your ability to find the right solutions quickly.
Understanding Different Types of Matter
In order to understand the fundamental properties of substances, it is crucial to recognize the different forms matter can take. These forms exhibit unique characteristics that can affect how they behave under various conditions. From solid to liquid and gas, each state has its own set of features that influence how substances interact with one another and with their surroundings.
Solid State
In the solid state, particles are tightly packed and vibrate in place, creating a stable structure. This state has a defined shape and volume, making it easy to identify. The arrangement of particles in solids allows them to maintain their structure and resist changes in shape under normal conditions. Metals, wood, and most rocks are common examples of substances in this state.
Liquid State

Unlike solids, liquids have particles that are loosely packed and able to move around each other. This mobility allows liquids to flow and take the shape of their container, though they still maintain a constant volume. Water, oil, and mercury are typical examples of materials in a liquid state. The ability of liquids to adapt to different shapes while retaining volume plays a key role in many physical processes.
Gaseous State
In the gaseous state, particles are widely spaced and move freely in all directions. Gases do not have a fixed shape or volume; they expand to fill any container. The molecules in a gas have high energy and move rapidly, which causes them to spread out. Common examples of gases include air, oxygen, and carbon dioxide. The behavior of gases is heavily influenced by factors like temperature and pressure.
Understanding the various forms of matter is fundamental in studying their behaviors and interactions. By recognizing the properties of each state, you can better predict how different substances will respond in diverse situations, which is crucial for scientific applications and everyday life.
Improving Your Problem-Solving Skills
Enhancing your ability to solve complex challenges requires a combination of practice, strategy, and a clear understanding of the core concepts involved. By adopting a structured approach, you can increase your efficiency and confidence in tackling various problems, particularly those involving calculations and measurements.
One of the first steps in improving your problem-solving abilities is to break down each task into manageable parts. Instead of being overwhelmed by the entire problem, focus on solving one piece at a time. Identify the key information provided, the unknown variables, and the relationships between them. This approach allows you to address each element systematically, reducing the risk of overlooking important details.
Practice Regularly
Consistent practice is essential to reinforcing problem-solving techniques. By regularly working through challenges, you become more familiar with different methods and gain a deeper understanding of the processes. Over time, this will improve your ability to recognize patterns, identify efficient strategies, and apply them effectively.
Use Visual Aids
Diagrams, charts, and other visual tools can be incredibly helpful in clarifying complex situations. When you encounter a problem, drawing a simple sketch or creating a table can help you visualize the relationships between variables. This visual approach can often reveal connections or insights that might not be immediately apparent through text alone.
Evaluate Your Mistakes
Mistakes are an invaluable part of the learning process. When you encounter errors, take the time to analyze where you went wrong. Did you misinterpret a piece of information? Were your calculations off? By understanding the root cause of your mistakes, you can refine your approach and avoid repeating the same errors in the future.
Stay Patient and Focused
Patience is key when solving challenging problems. It is easy to become frustrated when things don’t go as planned, but taking a step back and re-evaluating the problem with a fresh perspective can often lead to a solution. Staying calm, focused, and persistent is essential for success.
By adopting these strategies and consistently practicing, you can improve your problem-solving skills over time, making it easier to tackle even the most complex scenarios with confidence and clarity.