In this section, we explore essential concepts and provide thorough explanations to help you navigate through the challenges presented in this particular topic. With a focus on developing strong problem-solving skills, we aim to clarify the steps required to achieve the correct solutions and enhance your understanding of the material.
Step-by-step guidance will lead you through various examples, breaking down each problem into manageable parts. By approaching each challenge systematically, you will be better equipped to tackle similar questions on your own and deepen your mathematical insight.
It’s important to grasp the underlying principles that govern these problems, as they form the foundation for more complex ideas. Whether you’re revisiting foundational knowledge or encountering new topics, a clear understanding of the core concepts will pave the way for greater success and confidence in your studies.
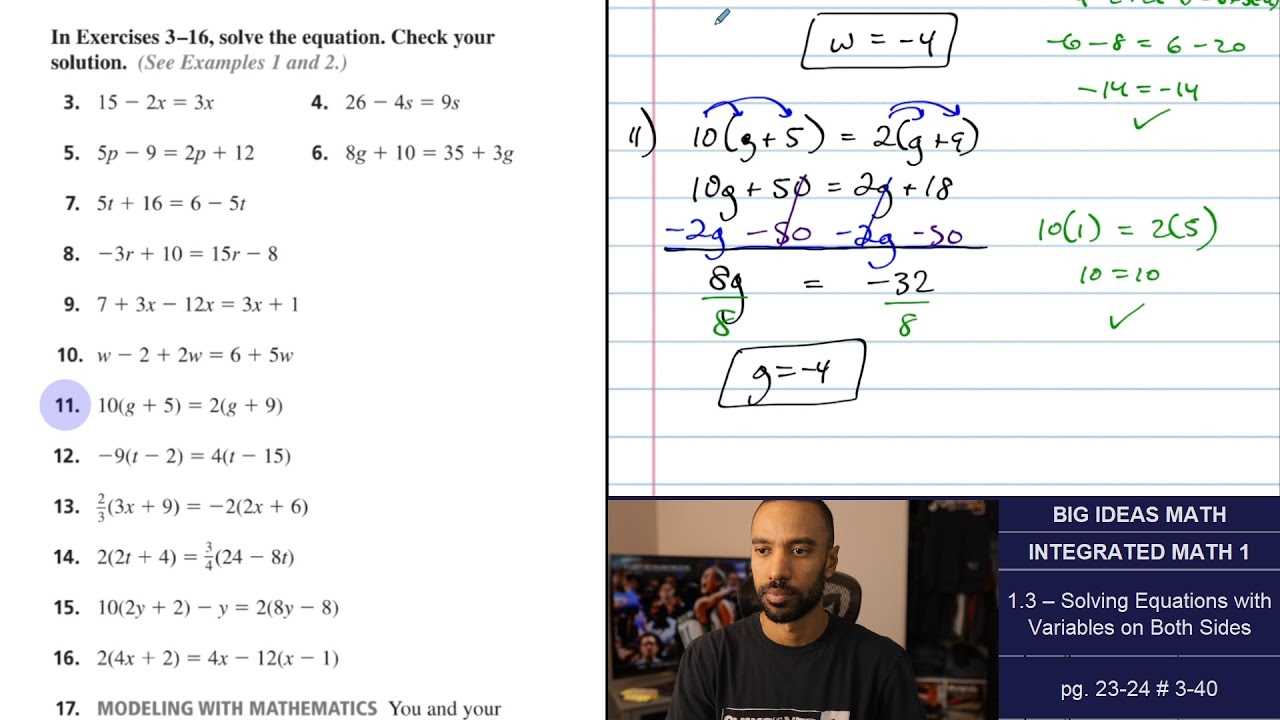
Big Ideas Math 1.3 Answers
This section focuses on providing clear and detailed solutions to help you better understand the challenges presented in this part of the curriculum. By following structured explanations, you will gain insight into the methods required to solve various types of problems and solidify your grasp on key concepts.
Each problem is approached in a logical, step-by-step manner, ensuring that you can easily follow along and replicate the process. The goal is to strengthen your problem-solving skills and enhance your ability to work through similar tasks independently.
Mastering these solutions is not just about reaching the right answer, but also about understanding the reasoning behind each step. This foundational knowledge will serve as a stepping stone for more advanced topics, equipping you with the tools to tackle increasingly complex material with confidence.
Overview of Big Ideas Math 1.3
This section introduces important concepts that form the core of this particular learning module. The focus is on building a strong foundation in problem-solving techniques and understanding essential mathematical principles. By carefully examining each topic, you will gain the skills necessary to approach and solve a variety of challenges with confidence.
Key Topics Covered
- Understanding fundamental operations
- Applying mathematical principles to real-world problems
- Exploring patterns and relationships in equations
- Mastering step-by-step problem-solving strategies
Approach to Learning
Each problem is designed to encourage critical thinking and deepen your understanding of core concepts. The structured approach ensures that you are not only solving for answers but also developing a deeper comprehension of the underlying techniques. This way, you can build upon your knowledge progressively, preparing you for more complex topics ahead.
Key Concepts in Section 1.3
This section introduces several fundamental principles that are essential for solving the problems presented. Understanding these core ideas is crucial for developing a deeper grasp of the material and mastering the techniques required to approach various questions.
Essential Ideas to Grasp
- The relationship between variables and constants
- Techniques for simplifying expressions and equations
- Identifying patterns and their applications
- Strategic approaches to solving multi-step problems
Building Strong Foundations
Each of these concepts plays a significant role in solving complex tasks. Mastering them will not only help you complete individual problems but will also strengthen your ability to tackle more advanced topics in future lessons. By practicing these principles, you will gain both accuracy and confidence in your problem-solving abilities.
Step-by-Step Solution Process
Breaking down each problem into smaller, manageable steps is crucial for finding the correct solutions. This process not only helps you understand how to arrive at the answer, but also reinforces the logical reasoning behind each decision. A clear, structured approach ensures that you tackle each challenge systematically and avoid common mistakes.
General Approach
- Read the problem carefully and identify key information.
- Determine the steps needed to solve the equation or expression.
- Apply the correct mathematical rules to simplify or manipulate the given values.
- Double-check your work at each step to ensure accuracy.
- Verify the final solution by substituting it back into the original problem.
Example Problem Breakdown
- Start by identifying the given variables and constants.
- Perform necessary operations step by step, following mathematical rules.
- Check the intermediate results and ensure that each step is logically sound.
- Once the final result is obtained, evaluate if it aligns with the context of the problem.
By following this methodical process, you will improve both your understanding and ability to solve problems efficiently. Each step serves as a building block that contributes to the overall solution and helps reinforce key concepts.
How to Approach Problem Sets
Effectively solving problem sets requires a focused and organized strategy. Breaking down the tasks and understanding each component will lead to clearer solutions and improved performance. It’s important to approach each set with a plan, rather than jumping straight to solving, as this ensures you don’t overlook key steps or concepts.
Start by analyzing the problem: Carefully read the instructions and identify what is being asked. Understand the context and note any given information. This foundational step will guide you throughout the process.
Next, break the task into smaller parts: Divide the problem into manageable sections or steps. This allows you to tackle one thing at a time, reducing the complexity and avoiding mistakes.
Finally, implement the correct methods: Apply the appropriate rules or formulas, keeping track of every step. Make sure to verify your work periodically to ensure consistency. After reaching a solution, review it to confirm its accuracy and relevance to the problem.
Common Mistakes to Avoid
When solving mathematical problems, it’s easy to make errors that can lead to incorrect answers. Identifying and understanding these common mistakes is crucial for improving accuracy and avoiding frustration. Being aware of potential pitfalls will help you refine your approach and ensure better results.
Frequent Errors to Watch For
- Misinterpreting the problem’s requirements or missing key details.
- Skipping steps in the calculation process, leading to incomplete or incorrect results.
- Incorrectly applying formulas or mathematical rules.
- Failing to check work after completing a problem, leading to overlooked mistakes.
- Confusing similar concepts or operations, such as addition and multiplication.
How to Prevent These Mistakes
- Read each problem carefully to ensure you understand the context and what is being asked.
- Work through each step methodically and double-check your calculations along the way.
- Review formulas and rules to ensure you are applying them correctly to each situation.
- Always verify your final solution by substituting it back into the original problem.
By staying mindful of these common errors and taking the time to carefully check your work, you will improve both your problem-solving skills and your overall performance.
Explaining Example Problems in Detail
Working through example problems is an essential part of mastering any subject. By examining each step in a problem-solving process, you can gain a deeper understanding of the techniques and methods used to reach the correct solution. Breaking down these examples allows you to identify key concepts and apply them to new, similar challenges.
In this section, we will explore several example problems in depth. Each problem will be dissected into smaller steps, highlighting the rationale behind every move. This approach ensures that you not only solve the problem correctly but also understand the reasoning behind each operation and decision.
By following these detailed explanations, you will be able to replicate the process in your own practice. Each example serves as a guide to help you build your skills and confidence, reinforcing the principles that will support your success in more complex tasks.
Tips for Solving Complex Questions
Tackling complex questions requires a strategic approach, patience, and a methodical breakdown of the problem. When faced with challenging tasks, it’s important to stay organized and focused. Following a series of well-planned steps can help you make sense of the most intricate problems and ultimately find the correct solution.
Effective Problem-Solving Strategies
- Start by identifying all the information provided in the problem.
- Break down the problem into smaller, more manageable parts.
- Look for patterns or relationships that could simplify your approach.
- Work through each step logically and methodically, ensuring no detail is overlooked.
- Check your work regularly to avoid making errors in calculations.
Tools to Aid in Problem Solving
Below is a helpful guide to assist you in organizing your approach to complex problems:
| Step | Action | Tip |
|---|---|---|
| Step 1 | Read the problem carefully | Highlight key information to avoid missing important details. |
| Step 2 | Break the problem into parts | Address each part separately to reduce complexity. |
| Step 3 | Apply relevant formulas or rules | Ensure you’re using the correct method for each step. |
| Step 4 | Check your solution | Substitute your final answer back into the problem to verify it. |
By following these strategies and using the tools provided, you will be able to confidently approach even the most challenging problems and solve them with accuracy and precision.
Visual Aids to Understand Solutions
Visual aids are powerful tools that can help clarify complex problems and make abstract concepts easier to grasp. By presenting information in a visual format, you can better understand the relationships between different elements and see how each step contributes to the final solution. Diagrams, graphs, and charts provide a clear representation of mathematical ideas, making them an invaluable resource for solving problems more effectively.
Charts and graphs: These tools are excellent for representing data and trends. They can visually highlight important patterns, making it easier to analyze relationships between variables.
Diagrams and flowcharts: Diagrams are helpful when dealing with geometric or spatial problems, while flowcharts can be used to map out logical steps in a problem-solving process. These visual tools help you understand the sequence of operations and their connections.
Using these visual aids: Whenever you are stuck or feeling uncertain about the problem, consider drawing a diagram or plotting data on a graph. This will often give you a fresh perspective and help you see the problem in a new light.
By incorporating visual tools into your problem-solving approach, you will not only improve your understanding but also enhance your ability to solve questions with greater confidence and clarity.
Using Practice Problems for Mastery
Practicing regularly with a variety of problems is essential for mastering any skill. It allows you to solidify your understanding, identify weaknesses, and reinforce the techniques needed for problem-solving. By consistently working through exercises, you develop a deeper grasp of concepts and improve your ability to tackle challenges efficiently.
Why Practice is Crucial
Repetition is key to retention and skill development. The more you practice, the more familiar you become with different types of problems and their solutions. Each problem you solve contributes to building confidence and improving your approach to new challenges.
Effective Ways to Use Practice Problems
To get the most out of practice exercises, consider the following approach:
| Step | Action | Benefit |
|---|---|---|
| Step 1 | Start with simpler problems | Build confidence and understanding before tackling harder questions. |
| Step 2 | Gradually increase difficulty | Expand your skills by working on more complex tasks once basic concepts are clear. |
| Step 3 | Review your mistakes | Learn from errors to avoid repeating them and deepen your understanding of the material. |
| Step 4 | Test under timed conditions | Simulate real exam situations to improve your speed and accuracy. |
Incorporating these strategies into your practice routine will not only help you master each concept but also build the skills necessary to solve even the most challenging problems with ease and confidence.
Understanding Mathematical Terminology
In any subject, understanding the language used is essential for grasping the concepts. In mathematics, specific terms are used to describe operations, relationships, and properties. Mastering this terminology allows you to communicate ideas clearly and apply them effectively in solving problems. Each term has a precise meaning, and understanding it is key to unlocking deeper insights into the subject.
Key Terms: Terms like “variable,” “equation,” and “function” are fundamental in building a strong foundation. Knowing their exact definitions and how they relate to one another helps prevent confusion and ensures accuracy in solving tasks.
Importance of Clear Definitions: Mathematical terms often have specific meanings that differ from everyday usage. For example, the word “sum” refers to the result of adding two numbers, while in other contexts, it might mean a total amount. Understanding these distinctions helps to avoid misinterpretation when working with equations and problems.
Building a Terminology Foundation: The more familiar you become with the language of mathematics, the easier it will be to approach new topics and solve problems. Regularly reviewing and using terms correctly will enhance both your understanding and application of mathematical concepts.
Best Study Techniques for Section 1.3
Effective studying is crucial for mastering complex topics and building a strong foundation. To fully understand and retain the material in any section, it’s important to use strategies that not only enhance comprehension but also improve recall during tests. By breaking down the content into manageable pieces and focusing on active learning, you can approach each concept with clarity and confidence.
Active Learning Methods
Rather than passively reading or reviewing notes, engage with the material actively. This can be achieved through various techniques such as:
- Practice Problems: Solving problems regularly reinforces key concepts and helps identify areas that need more attention.
- Summarization: Writing concise summaries of each topic can solidify your understanding and ensure you grasp the main ideas.
- Self-Quizzing: Test yourself on the material to boost retention and gauge your understanding of the topic.
Time Management and Consistency
Studying consistently, rather than cramming, is essential for long-term retention. Create a study schedule that breaks the section into smaller, more focused study sessions. This approach helps to reduce overwhelm and allows for better absorption of the material.
Incorporating these techniques into your study routine will not only improve your understanding but also enhance your ability to recall the information when needed. Stay disciplined and keep practicing, and the material will become much more manageable over time.
Review of Key Formulas and Theorems
Understanding the fundamental principles of any subject is essential for solving problems effectively. In mathematics, formulas and theorems serve as tools that simplify complex calculations and provide structured solutions. Reviewing these key concepts regularly can strengthen your problem-solving skills and help you apply them with confidence in various scenarios.
Important Formulas to Remember
Several formulas are frequently used across different topics and can be applied in a variety of problems. Here are a few core formulas to keep in mind:
- Quadratic Formula: The solution to quadratic equations is given by x = (-b ± √(b² – 4ac)) / 2a, where a, b, and c are coefficients from the quadratic equation ax² + bx + c = 0.
- Pythagorean Theorem: For right triangles, the relationship between the sides is a² + b² = c², where c is the hypotenuse and a and b are the legs of the triangle.
- Area of a Circle: The area is given by A = πr², where r is the radius of the circle.
Key Theorems and Principles
In addition to formulas, understanding important theorems is vital for solving more complex problems. Some essential theorems include:
- Fundamental Theorem of Algebra: Every non-constant polynomial equation has at least one complex root.
- Law of Sines and Cosines: These laws are used to solve triangles, particularly non-right triangles. They establish relationships between the sides and angles of a triangle.
- Properties of Exponents: Rules such as x^a × x^b = x^(a+b) and (x^a)^b = x^(ab) are essential for simplifying expressions involving exponents.
Mastering these formulas and theorems is crucial for gaining proficiency in mathematical problem-solving. By reviewing them regularly and practicing their application, you can build a solid foundation for tackling more complex topics with ease.
How to Check Your Answers
Verifying the accuracy of your solutions is an essential part of problem-solving. It ensures that your results are correct and that you’ve applied the right methods throughout the process. By using systematic approaches, you can efficiently check your work and identify any errors before finalizing your solutions.
Here are several methods you can use to check your results:
- Revisit the Problem Statement: Always start by reviewing the original problem. Ensure you understand what is being asked and that you’ve followed all the instructions correctly.
- Substitute Your Solution: For equations or functions, substitute your answer back into the original equation. If both sides match, your solution is correct.
- Use Estimation: If your problem allows, estimate the expected result. Comparing the estimated value to your final answer can help identify any glaring inconsistencies.
- Check Units and Dimensions: For problems involving measurements, ensure that your units are consistent throughout the solution. Mismatched units often signal an error in the calculation.
- Work Backwards: Try solving the problem in reverse to see if you arrive at the same starting point. This is particularly useful for more complex problems or those involving multiple steps.
By incorporating these techniques into your routine, you can significantly reduce errors and improve your overall problem-solving accuracy. Checking your answers is not just about finding mistakes but also about reinforcing your understanding and ensuring that you’re on the right track.
Importance of Conceptual Understanding
Building a deep understanding of fundamental principles is crucial for mastering any subject. This conceptual grasp helps learners see the connections between different ideas and apply them effectively to solve various problems. Without a strong foundation in the key concepts, it’s easy to rely on rote memorization or shortcuts, which can limit one’s ability to tackle more complex challenges.
When you focus on understanding the underlying concepts, you gain the flexibility to approach problems from different angles. This flexibility is especially important when faced with unfamiliar situations or problems that don’t follow a standard pattern. Conceptual understanding allows you to adapt your approach and think critically, rather than simply following a set of instructions.
Benefits of Strong Conceptual Knowledge
- Improved Problem-Solving Skills: A deep understanding enables you to break down complex problems into smaller, more manageable steps.
- Better Retention: Concepts are easier to remember when they are understood on a deeper level, as opposed to memorized facts.
- Enhanced Critical Thinking: When you understand the reasoning behind a method, you are better equipped to assess different solutions and choose the most appropriate one.
In essence, conceptual understanding forms the backbone of long-term success in learning. It goes beyond memorizing formulas or steps and allows learners to develop a genuine comprehension of the material. This foundation is essential for progressing to more advanced topics and for applying knowledge in real-world situations.
Resources for Additional Practice
To truly master any topic, additional practice beyond the classroom or primary materials is essential. Engaging with supplementary resources allows learners to reinforce their understanding, gain exposure to a variety of problems, and build confidence in applying concepts. Whether you’re looking for exercises, tutorials, or interactive tools, there are many resources available that can help you solidify your knowledge.
Below are some of the best resources for further practice, ranging from online platforms to printed materials, all designed to enhance your learning experience:
| Resource Type | Description | Link |
|---|---|---|
| Online Practice Websites | Interactive websites that offer practice problems, solutions, and step-by-step explanations. | Khan Academy |
| Video Tutorials | Educational videos explaining complex concepts in a clear and engaging way. | YouTube Educational Channels |
| Study Guides | Comprehensive guides that break down topics into manageable sections for deeper understanding. | SparkNotes |
| Interactive Apps | Mobile apps that provide on-the-go practice and quizzes. | Photomath |
By utilizing these resources, learners can expand their practice options, get immediate feedback, and continue refining their skills. Consistent use of these tools will lead to better retention and mastery of the material over time.