Grasping advanced concepts can feel like a significant challenge, especially when navigating through intricate exercises. With structured guidance and clear methods, learning becomes not only more accessible but also deeply rewarding. This section focuses on simplifying complicated ideas and breaking them into understandable steps.
Through detailed examples and well-explained solutions, learners can enhance their understanding and build confidence in approaching various tasks. These techniques are designed to foster critical thinking and logical reasoning, which are essential for mastering any topic.
Whether you’re preparing for an upcoming assessment or seeking to strengthen your grasp on foundational skills, the strategies provided here aim to make the learning process engaging and efficient. Explore practical tips and insights that will help transform challenges into opportunities for growth.
Comprehensive Guide to Math 5.2 Concepts

Understanding advanced topics requires a clear approach that breaks down complex ideas into manageable parts. This section offers a roadmap to navigate challenging exercises, providing strategies that simplify learning and make problem-solving more intuitive.
Breaking Down Key Components
The foundation of success lies in identifying and analyzing the essential elements of each problem. By focusing on these core aspects, learners can develop a deeper understanding and effectively tackle tasks step by step. Techniques such as grouping similar elements and applying logical reasoning are emphasized to ensure clarity.
Practical Applications and Problem-Solving
Real-world examples often help illustrate theoretical concepts, making them easier to comprehend. Applying learned strategies to practical scenarios enhances retention and confidence. This section highlights scenarios where these methods can be used, ensuring a well-rounded approach to mastering any exercise.
Breaking Down Problem-Solving Techniques

Approaching complex challenges becomes significantly easier with a clear and structured methodology. Breaking tasks into smaller, manageable steps allows for a focused and efficient resolution, ensuring no critical details are overlooked. This approach helps transform seemingly difficult problems into achievable goals.
The first step involves understanding the requirements and identifying the key components of the task. Careful analysis ensures clarity in recognizing patterns or relationships that simplify the process. Next, applying logical reasoning and tested strategies aids in systematically working through each part of the challenge.
By practicing these techniques consistently, learners can enhance their confidence and develop a solid framework for tackling diverse problems. This method not only improves accuracy but also nurtures critical thinking skills essential for long-term success.
Effective Methods for Simplifying Expressions
Simplifying mathematical expressions is an essential skill that enables quicker problem solving and clearer understanding. By recognizing patterns and applying specific techniques, complex equations can be reduced to their simplest forms, making them easier to work with and solve.
Combining Like Terms

One of the most powerful methods for simplifying expressions is combining like terms. This involves grouping terms that share the same variables and exponents. By consolidating these terms, the expression becomes more concise and manageable, eliminating unnecessary complexity.
Factoring and Distributive Property

Another approach to simplifying expressions is factoring, where common factors are extracted from terms. This can lead to more streamlined equations and reveal patterns that might not be immediately apparent. The distributive property also plays a key role in breaking down and simplifying expressions by distributing terms across parentheses.
Mastering Basic Operations with Confidence

Building a solid foundation in fundamental operations is crucial for tackling more advanced topics. By strengthening your understanding and skills in basic arithmetic, you can approach more complex problems with ease and confidence. Mastering these essential skills creates a strong base for all future mathematical challenges.
Key Operations to Focus On

- Addition: Combining numbers to find a total.
- Subtraction: Finding the difference between two values.
- Multiplication: Repeated addition of a number.
- Division: Splitting a number into equal parts.
Practicing with Real-World Scenarios

To truly master basic operations, it’s important to practice them in a variety of contexts. Using everyday examples, such as budgeting or cooking, helps reinforce these concepts and makes them more relevant and accessible. These practical applications enhance problem-solving skills and ensure that the fundamentals become second nature.
Understanding Algebraic Equations in Depth
Grasping the principles behind algebraic expressions and equations is essential for solving a wide range of mathematical problems. An equation is not just a string of symbols but a balanced relationship that requires manipulation to uncover unknown values. Deepening your understanding of these relationships makes it easier to apply these concepts in various contexts.
Key Components of Algebraic Equations

- Variables: Symbols that represent unknown values.
- Constants: Fixed values that do not change.
- Coefficients: Numbers that multiply the variables.
- Operators: Symbols representing operations such as addition, subtraction, multiplication, and division.
Solving Equations Step by Step
Solving algebraic equations involves isolating the variable on one side of the equation. This process typically follows a sequence of steps:
- Identify and simplify both sides of the equation.
- Move terms involving the variable to one side.
- Apply inverse operations to isolate the variable.
- Check the solution by substituting it back into the original equation.
Strategies to Solve Word Problems Quickly
Word problems can often seem overwhelming due to the need to translate real-world scenarios into mathematical terms. However, with the right approach, you can simplify the process and solve them efficiently. The key lies in breaking down the problem, identifying important information, and applying logical steps to reach the solution.
Approach to Solving Word Problems
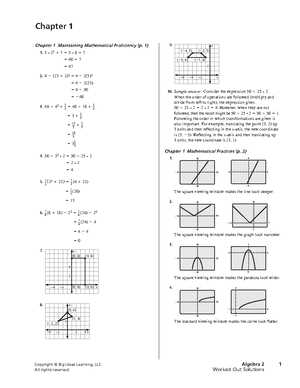
The following table outlines an effective strategy for tackling word problems:
| Step | Action |
|---|---|
| 1 | Read the problem carefully and identify key information. |
| 2 | Define what you are solving for, i.e., the unknown. |
| 3 | Translate the words into a mathematical equation. |
| 4 | Solve the equation using appropriate methods. |
| 5 | Double-check your solution to ensure accuracy. |
Helpful Tips for Speed and Accuracy
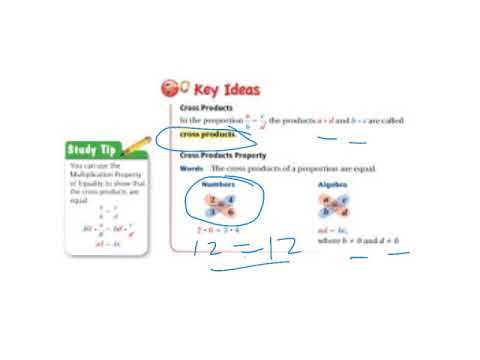
- Underlining key numbers and keywords can make the problem easier to navigate.
- Draw diagrams or use charts to visualize the situation when necessary.
- Break complex problems into smaller, more manageable steps.
- Practice regularly to develop familiarity with common word problem types.