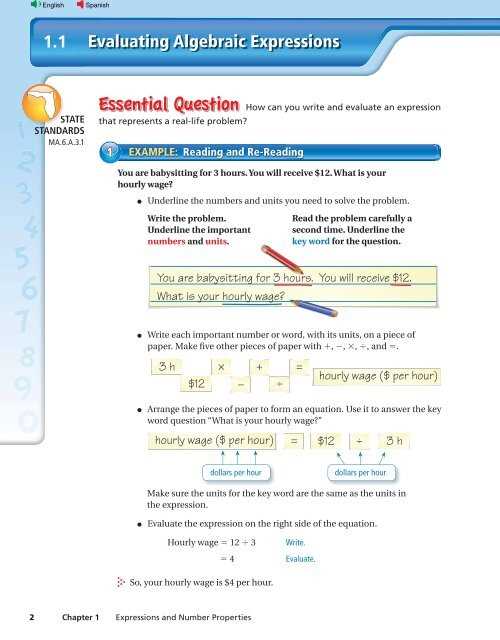
In this section, we focus on providing clear explanations and practical solutions for complex mathematical challenges. Whether you’re struggling with intricate formulas or need help navigating through difficult equations, this guide is designed to make the learning process smoother and more accessible.
By breaking down key concepts and offering step-by-step guidance, we aim to help you build a strong foundation and gain confidence in your ability to solve problems. With careful explanations and targeted examples, you’ll be able to tackle any equation, no matter how challenging.
Understanding the core principles is essential for mastering higher-level concepts. This resource provides the tools you need to unlock your full potential, from solving quadratic equations to mastering complex functions. Keep reading to discover methods that can elevate your problem-solving skills and enhance your mathematical understanding.
Big Ideas Math Algebra 2 Answers
This section provides detailed solutions to some of the most challenging exercises in your advanced coursework. The focus is on offering practical methods and clear strategies for tackling a variety of complex mathematical problems. With a structured approach, each problem is broken down into manageable steps to help you better understand the underlying concepts.
By exploring key techniques and offering helpful hints, the goal is to enhance your problem-solving abilities. Each example is chosen to reflect common difficulties students face and to provide the tools needed to overcome these obstacles efficiently. Whether you’re working through quadratic expressions, rational functions, or systems of equations, the solutions provided aim to guide you through every stage of the process.
Success in this subject relies on the ability to not only solve equations but to also comprehend the logic behind the operations. With these detailed step-by-step solutions, you’ll gain a deeper understanding of each topic, ultimately improving your mathematical skills and boosting your confidence in applying what you have learned to real-world scenarios.
Overview of Algebra 2 Key Concepts
This section provides an in-depth look at the fundamental ideas that form the backbone of higher-level mathematics. Understanding these concepts is essential for advancing to more complex topics and solving intricate problems with confidence. The focus here is on providing a clear structure for learning, so you can master each core element step by step.
The key topics covered include equations, functions, graphing, and systems of variables. These concepts are interconnected and build upon one another, allowing you to approach more advanced challenges as you progress. Here, we will outline some of the most important topics you’ll encounter, breaking them down into their core components for easier understanding.
| Topic | Key Concepts |
|---|---|
| Equations | Linear, quadratic, and polynomial equations; solving techniques and methods |
| Functions | Types of functions, domain and range, function notation |
| Graphing | Coordinate plane, graphing equations, transformations, and asymptotes |
| Systems | Solving systems of equations, substitution, and elimination methods |
| Exponents & Logarithms | Properties of exponents, logarithmic functions, and their applications |
Mastery of these concepts provides the foundation for solving more advanced mathematical problems and tackling real-world applications in fields such as engineering, economics, and computer science. Understanding how to manipulate equations and functions is the key to unlocking further knowledge in this area.
How to Use Big Ideas Math Effectively
To make the most of your learning experience, it’s essential to approach your studies with a structured and methodical plan. Effectively using resources for advanced problem-solving requires understanding how to access and apply the material in a way that reinforces key concepts and enhances your comprehension.
Here are a few strategies to help you use the available resources efficiently:
- Review the Introduction to Each Chapter: Start by reading the introductory sections. They provide a broad overview of the concepts you’ll be learning and give context to the exercises.
- Break Down Problems Step-by-Step: Don’t rush through problems. Tackle each step methodically to ensure you understand every component of the solution.
- Use Practice Problems for Reinforcement: Regularly work through example problems to reinforce your understanding. Try different types of problems to ensure mastery of the material.
- Refer to Solutions for Clarification: When you encounter challenges, look at the solutions to help clarify where you may have gone wrong. Understanding the reasoning behind each step is crucial.
- Ask Questions: If something isn’t clear, don’t hesitate to ask for help. Use online resources or ask teachers and peers for additional explanations.
By following these steps, you can maximize your understanding and retention of complex concepts, making it easier to apply them to new problems. Consistency and practice are key when it comes to mastering advanced topics.
Step-by-Step Solutions for Common Problems
This section provides detailed, easy-to-follow solutions for some of the most frequently encountered challenges in advanced coursework. By breaking down each problem into manageable steps, we aim to make the learning process more accessible and clear, allowing you to understand the reasoning behind each solution.
Below are a few examples of common problems, with step-by-step instructions to guide you through the process. These examples cover a variety of topics, ensuring that you are well-prepared to tackle similar questions on your own.
| Problem Type | Step-by-Step Solution |
|---|---|
| Solving Quadratic Equations |
|
| Graphing a Linear Equation |
|
| Solving Systems of Equations |
|
These step-by-step solutions provide a structured approach to solving a variety of problems. By following these methods, you can gain a deeper understanding of the concepts and build the confidence needed to tackle even the most challenging exercises.
Mastering Polynomial Equations
Polynomial equations are fundamental in advanced mathematics and often appear in a variety of topics. Mastering these expressions is key to solving more complex problems, as they provide the foundation for understanding higher-level concepts such as functions, graphing, and factorization. This section will guide you through essential techniques for handling polynomials efficiently and effectively.
Understanding Polynomial Structure
A polynomial consists of terms that are combined using addition, subtraction, and multiplication. The highest exponent in the expression determines its degree, and each term includes a coefficient. The primary goal is to understand the structure of these expressions, as this knowledge helps with identifying their roots and simplifying the equation. Recognizing the degree and the leading term is crucial for determining the behavior of the polynomial as you solve it.
Techniques for Solving Polynomial Equations
There are several methods for solving polynomial equations, depending on the specific problem at hand. One of the most commonly used techniques is factorization, where the polynomial is expressed as a product of binomials or other polynomials. Another method is using the quadratic formula, which can also be applied to quadratic components within the larger equation. Additionally, synthetic division and the Rational Root Theorem can help identify possible solutions, streamlining the process of solving more complex expressions.
By practicing these techniques and understanding the structure of polynomial equations, you’ll be able to approach more advanced problems with confidence and ease. These foundational skills are essential for success in higher-level mathematics.
Understanding Rational Functions
Rational functions are a key component in advanced mathematical study, as they represent the ratio of two polynomials. These functions can describe a wide range of real-world phenomena, from rates of change to optimization problems. Understanding how to work with rational functions is essential for solving more complex equations and analyzing their behavior effectively.
At their core, rational functions are expressions where one polynomial is divided by another. The key to mastering them lies in identifying their domain, simplifying the expressions, and analyzing the asymptotes–both vertical and horizontal–that define their behavior. A deep understanding of these functions allows you to solve complex problems involving rates, proportions, and limits with ease.
By learning how to manipulate and simplify rational functions, you gain the ability to solve higher-level problems that require a nuanced understanding of relationships between variables. Whether dealing with graphing, solving equations, or analyzing limits, mastering these concepts is a fundamental step in advancing your mathematical knowledge.
Key Strategies for Solving Inequalities
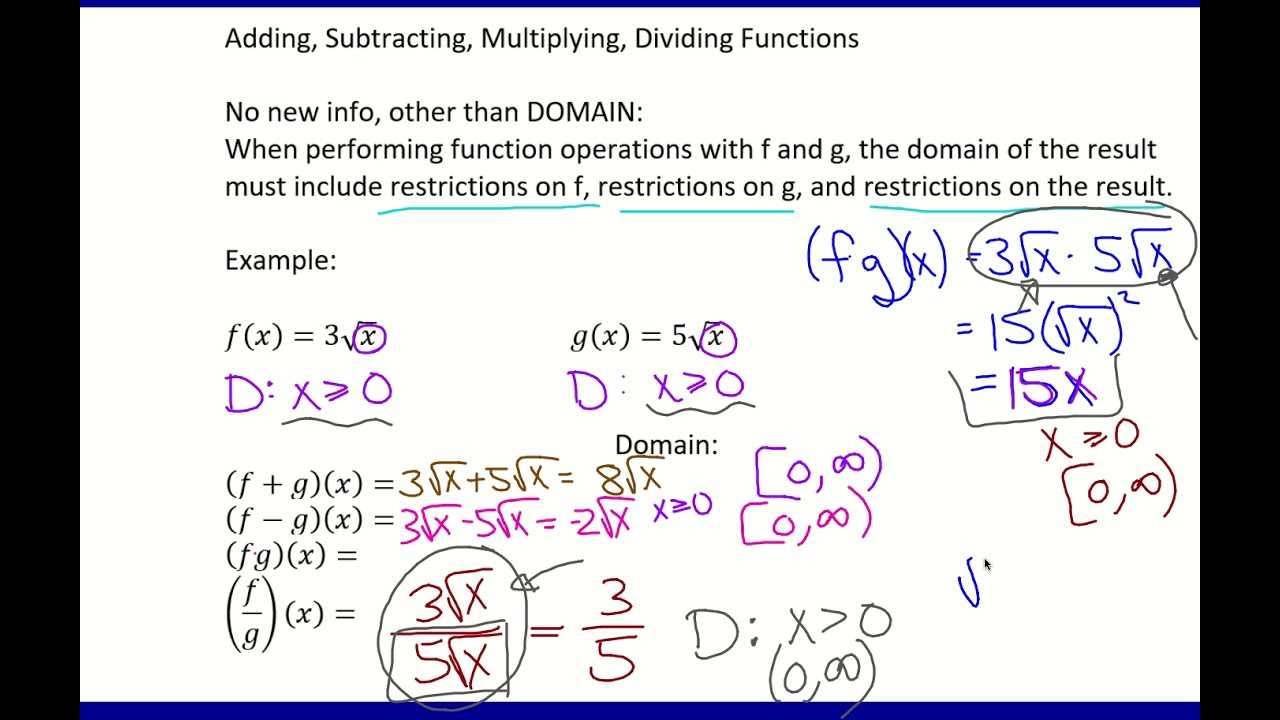
Solving inequalities requires a systematic approach, as it involves finding a range of values that satisfy the given conditions, rather than just one specific solution. Understanding the different types of inequalities and the strategies for solving them is crucial for developing problem-solving skills in advanced mathematical topics. These techniques provide the foundation for approaching more complex problems that arise in real-world applications.
One of the most important strategies is to treat inequalities similarly to equations, but with some key differences. When solving, it’s essential to pay close attention to the direction of the inequality symbol, especially when multiplying or dividing both sides by a negative number. This small adjustment can change the entire solution set and should be done with caution.
Other useful strategies include isolating the variable, combining like terms, and using test values to determine which solutions satisfy the inequality. Graphing can also be a helpful tool for visualizing the solution set, particularly when dealing with compound inequalities or systems. By practicing these methods, you will be well-equipped to solve a wide range of inequality problems with confidence.
Breaking Down Exponential and Logarithmic Functions
Exponential and logarithmic functions are two critical concepts in higher mathematics, often used to model a variety of real-world phenomena. These functions are interrelated and understanding how they work can provide valuable insights into complex problem-solving. By breaking down their components, you can gain a deeper understanding of their behavior and applications.
Exponential functions are characterized by a constant base raised to a variable exponent. These functions grow or decay rapidly depending on the base. In contrast, logarithmic functions are the inverse of exponential functions and focus on determining the exponent needed for a given base to produce a specific number.
To master these concepts, here are a few key points to keep in mind:
- Exponential Functions: Identify the base and the exponent. The growth or decay rate depends on the base of the function.
- Logarithmic Functions: Understand that logarithms answer the question, “To what exponent must the base be raised to produce this number?”
- Inverse Relationship: Recognize that exponential and logarithmic functions are inverses of each other, which can simplify solving equations.
- Properties: Familiarize yourself with the properties of both functions, such as how logarithms can be used to simplify multiplication and division in exponential equations.
With these strategies in mind, you can approach exponential and logarithmic functions with a solid understanding of their behavior and how to manipulate them in various problem-solving contexts. These functions are fundamental in many areas, including finance, population modeling, and physics.
Solving Systems of Equations with Ease
Systems of equations can initially seem challenging, but with the right strategies, solving them becomes a straightforward task. A system consists of two or more equations that share common variables, and the goal is to find values for those variables that satisfy all equations simultaneously. Mastering the methods for solving these systems will give you the tools to approach a wide variety of problems with confidence.
There are several techniques for solving systems, each suited to different types of equations. The most common methods include substitution, elimination, and graphing. Each of these approaches allows you to isolate variables and simplify the system, ultimately leading to the solution.
Substitution Method: This technique involves solving one equation for a single variable and then substituting that expression into the other equation(s). It’s particularly useful when one of the equations is easy to solve for a variable.
Elimination Method: In this approach, you manipulate the equations to eliminate one variable, making it easier to solve for the remaining variables. This is often the quickest method when both equations are in standard form.
Graphing Method: Graphing involves plotting both equations on a coordinate plane and identifying the point(s) where they intersect. While this method is visually intuitive, it’s often less precise for complex systems unless using technology to aid the process.
By applying these methods appropriately, you’ll be able to solve systems of equations more efficiently and gain a deeper understanding of the relationships between variables. These skills are crucial for tackling more advanced problems and scenarios that involve multiple conditions or constraints.
How to Approach Quadratic Equations
Quadratic equations are fundamental in mathematics and appear in a wide range of applications, from physics to economics. These equations, which involve a variable raised to the second power, often require specific methods to solve effectively. Understanding how to break down and approach them can make solving even the most complex problems much simpler.
There are several strategies for solving quadratic equations, each suited to different situations. The choice of method often depends on the form of the equation and the available information. Common approaches include factoring, completing the square, and using the quadratic formula.
Factoring: This method involves rewriting the quadratic equation as a product of two binomials. It works best when the equation is easy to factor. Once factored, the solutions can be found by setting each factor equal to zero.
Completing the Square: This technique is useful when the equation doesn’t easily factor. It involves manipulating the equation so that one side becomes a perfect square trinomial, allowing for easier solving by taking square roots.
Quadratic Formula: The quadratic formula is a universal method that can be applied to any quadratic equation. It uses the coefficients of the equation to find the solutions directly, making it a reliable choice when other methods are difficult to apply.
By familiarizing yourself with these approaches and knowing when to apply each one, you can tackle quadratic equations with confidence. These strategies form the basis for understanding more advanced concepts and solving a wide variety of mathematical problems.
Finding Roots of Complex Functions
Identifying the roots of complex functions is a critical skill in advanced mathematics. Roots represent the values of variables that satisfy the equation, essentially “zeroing” the function. These solutions are often more difficult to find when dealing with complex functions, but with the right methods, they can be determined with precision.
When working with complex functions, it’s essential to recognize that the roots may involve complex numbers, including both real and imaginary components. To simplify the process, here are several methods that can be applied:
- Factoring: For polynomial functions, factoring the equation into simpler expressions is a common technique. This may reveal the roots more directly, especially when the function is quadratic or cubic.
- Graphing: By plotting the function on a graph, you can visually estimate where the function crosses the x-axis. This method provides a useful starting point, particularly when exact algebraic solutions are difficult to obtain.
- Numerical Methods: For more complex functions, numerical methods such as Newton’s method or the bisection method can be employed to approximate the roots. These techniques are especially helpful when an exact analytical solution is not readily available.
- Using the Complex Plane: Complex roots can be found by considering the function in the complex plane. This requires understanding polar coordinates and the argument of complex numbers, which helps in identifying the exact location of the roots in a two-dimensional space.
By applying these strategies, you can effectively find the roots of complex functions, which is an essential skill for tackling more advanced topics in mathematics and science. Understanding how to approach these problems will provide a strong foundation for solving a variety of real-world challenges.
Understanding Sequences and Series
Sequences and series are fundamental concepts that appear frequently in various mathematical contexts. A sequence represents an ordered list of numbers, while a series is the sum of the terms in a sequence. Understanding how to work with these concepts is essential for solving problems in both theoretical and applied mathematics.
Sequences can follow different patterns, such as arithmetic, geometric, or even more complex recursive patterns. Each type has specific properties that make them easier to handle and analyze. Series, on the other hand, require a summation of terms, and the focus is on determining their total value or the behavior of their sum as the number of terms increases.
- Arithmetic Sequences: These are sequences where the difference between consecutive terms is constant. The nth term can be calculated using a simple formula: an = a1 + (n-1)d, where a1 is the first term and d is the common difference.
- Geometric Sequences: In these sequences, each term is found by multiplying the previous term by a fixed number, called the common ratio. The nth term of a geometric sequence can be expressed as an = a1 * r^(n-1), where r is the common ratio.
- Series Summation: When summing the terms of a sequence, you are working with a series. Arithmetic and geometric series have well-established formulas for finding their sums. For example, the sum of the first n terms of an arithmetic series is given by S = n/2 * (a1 + an), while the sum of a geometric series can be calculated using the formula S = a1 * (1 – r^n) / (1 – r) for r ≠ 1.
- Convergence and Divergence: Not all series have a finite sum. A series that approaches a finite value as the number of terms increases is said to converge, while one that grows without bound is considered to diverge. Understanding convergence is particularly important in calculus and advanced mathematics.
By mastering sequences and series, you can solve a wide range of problems and develop a deeper understanding of mathematical structures. These concepts form the basis for more advanced topics in analysis, combinatorics, and even real-world applications such as finance and physics.
Graphing Techniques for Algebra 2
Understanding how to graph various types of equations is a vital skill in advanced mathematics. Graphing helps visualize relationships between variables and provides insight into the behavior of functions. This section explores key techniques that will help you graph equations accurately and efficiently.
Each type of equation or function requires a specific approach to graphing. The strategies vary depending on whether you are working with linear, quadratic, exponential, or rational functions. By following systematic methods, you can quickly create accurate graphs and interpret their features.
- Plotting Linear Equations: To graph a linear equation, begin by identifying the slope and y-intercept. Using the formula y = mx + b, where m is the slope and b is the y-intercept, you can easily plot the graph by starting at the y-intercept and using the slope to find additional points.
- Graphing Quadratic Functions: For quadratic functions, recognize the standard form y = ax^2 + bx + c. The graph of a quadratic function is a parabola. Begin by finding the vertex and axis of symmetry, then plot points to the left and right of the vertex to draw the curve. You can also determine whether the parabola opens upward or downward based on the value of a.
- Exponential Functions: Exponential graphs grow or decay rapidly, depending on the base of the exponential. To graph an exponential function, identify the base and vertical asymptote. Then, plot key points by evaluating the function for various x-values, keeping in mind that exponential functions increase or decrease exponentially.
- Rational Functions: Rational functions are fractions of polynomials. When graphing these functions, find vertical and horizontal asymptotes, as well as any holes in the graph. These features will guide you in sketching the general shape of the graph. Check for intercepts by setting the numerator and denominator equal to zero separately.
- Using Transformations: Transformations help manipulate graphs of functions. By applying shifts, reflections, stretches, and compressions, you can modify the graph of any function. For example, a vertical shift occurs when you add or subtract a constant from the function, and a horizontal shift occurs when you add or subtract from the x-variable.
By mastering these graphing techniques, you can efficiently represent functions and equations, which is essential for understanding their properties and behavior. A well-plotted graph is a powerful tool for solving problems and analyzing mathematical relationships.
Learning to Factor Complex Expressions
Factoring is an essential technique in simplifying expressions and solving equations. When dealing with more intricate mathematical problems, knowing how to break down complex expressions into their factors can make a significant difference. This process involves identifying common factors and applying specific methods to simplify the given expression into a product of simpler terms.
The goal of factoring is to rewrite an expression as the product of two or more simpler expressions, which makes solving equations easier and more efficient. It also helps in identifying key features of functions, such as their roots or intercepts. In this section, we will explore various strategies to factor complex expressions step by step.
Key Techniques for Factoring
To master factoring, there are several methods to consider. Below are some of the most commonly used techniques:
- Factoring by Grouping: This method involves rearranging terms in the expression to group them in pairs, from which common factors can be extracted. Once the terms are grouped, factor each group separately and look for a common binomial factor that can be factored out.
- Factoring Quadratic Expressions: For quadratic expressions of the form ax^2 + bx + c, the goal is to find two numbers that multiply to ac and add up to b. These numbers are used to split the middle term, and then the expression can be factored by grouping.
- Difference of Squares: The difference of squares is a special factoring formula: a^2 – b^2 = (a + b)(a – b). Recognizing when an expression fits this pattern allows you to quickly factor it as the product of two binomials.
- Perfect Square Trinomial: A perfect square trinomial is an expression of the form a^2 + 2ab + b^2, which can be factored into (a + b)^2. Recognizing this form makes factoring more efficient.
- Sum or Difference of Cubes: For cubic expressions like a^3 + b^3 or a^3 – b^3, use the formulas a^3 + b^3 = (a + b)(a^2 – ab + b^2) or a^3 – b^3 = (a – b)(a^2 + ab + b^2) to factor the expressions.
Practical Example
Let’s look at an example of factoring a complex expression using the methods described above. Consider the following expression:
| Expression | Factoring Steps |
|---|---|
| 6x^2 + 11x + 3 |
|
By following these steps, you can factor complex expressions into their simplest form, making them easier to solve or analyze. This process is crucial for understanding the structure of equations and can greatly simplify your problem-solving approach.
Trigonometric Functions and Their Applications
Trigonometric functions play a crucial role in many areas of mathematics, especially when dealing with angles, waveforms, and periodic phenomena. These functions allow us to model real-world situations that involve cyclical patterns, such as sound waves, light waves, and even the movement of celestial bodies. Understanding how these functions work and how they can be applied in practical scenarios is essential for solving complex problems in both theoretical and applied mathematics.
These functions are typically defined based on the relationships between the sides of a right triangle or through unit circle concepts. The primary trigonometric functions include sine, cosine, tangent, secant, cosecant, and cotangent. Each of these functions has distinct characteristics and can be used in various contexts, from simple geometry to advanced calculus.
Common Trigonometric Functions
Each trigonometric function is derived from the ratio of different sides in a right triangle. Here’s a brief overview of the most common ones:
- Sine (sin): Defined as the ratio of the opposite side to the hypotenuse.
- Cosine (cos): Defined as the ratio of the adjacent side to the hypotenuse.
- Tangent (tan): Defined as the ratio of the opposite side to the adjacent side.
- Secant (sec): The reciprocal of cosine.
- Cosecant (csc): The reciprocal of sine.
- Cotangent (cot): The reciprocal of tangent.
Applications in Real-World Scenarios
Trigonometric functions are essential in various fields, from engineering to physics to economics. Some key applications include:
- Waveforms and Oscillations: Sine and cosine functions are used to describe wave patterns in sound, light, and electricity.
- Engineering: Trigonometric functions are used to design structures, analyze forces, and calculate angles in various engineering projects.
- Astronomy: Trigonometry helps astronomers calculate distances and angles between celestial bodies.
- Navigation: Trigonometric principles are used in GPS technology and to determine distances and bearings on maps.
Example of Trigonometric Applications
Consider a situation where you need to calculate the height of a building using a simple trigonometric model. If you know the distance from the building and the angle of elevation, you can use the tangent function to determine the height:
| Known Information | Value |
|---|---|
| Distance from the building | 50 meters |
| Angle of elevation | 30° |
| Height of the building | Using tan(30°) = height / 50 meters |
By rearranging the equation, you can solve for the height of the building. This example illustrates how trigonometric functions provide a straightforward way to solve practical problems involving angles and distances.
Understanding the fundamental concepts of trigonometry and their applications allows you to model real-world situations and solve problems across many disciplines. Whether you’re analyzing the motion of a pendulum or calculating the height of a mountain, these functions are powerful tools in mathematical analysis.
Using Matrices to Solve Problems
Matrices are a powerful tool for solving complex problems across various fields, including systems of equations, transformations, and data analysis. By organizing data in rows and columns, matrices provide a compact and efficient way to represent and manipulate information. Whether you’re working with linear equations, computer graphics, or network analysis, understanding how to use matrices can significantly simplify problem-solving processes.
Basic Matrix Operations
There are several key operations that can be performed on matrices to solve problems. These operations allow for the manipulation of matrix data in ways that make complex calculations easier. Some of the most common matrix operations include:
- Matrix Addition: Adding two matrices by adding their corresponding elements.
- Matrix Multiplication: Combining two matrices by taking the dot product of rows and columns.
- Matrix Inversion: Finding the inverse of a matrix, which is particularly useful for solving systems of equations.
- Determinants: Calculating the determinant of a matrix to understand its properties and behavior.
Applications of Matrices
Matrices have numerous real-world applications in fields ranging from engineering to economics. Here are some key examples of how matrices are used:
- Solving Systems of Linear Equations: Matrices are commonly used to solve systems of linear equations using techniques such as Gaussian elimination or matrix inversion.
- Computer Graphics: Matrices are used to perform transformations like rotations, scaling, and translations in graphics programming.
- Network Analysis: In network theory, matrices help represent and analyze connections between nodes, making it easier to study relationships in complex networks.
- Economics and Finance: Matrices are used to model and solve problems related to optimization, supply-demand analysis, and market equilibrium.
Example: Solving a System of Equations Using Matrices
Consider the following system of equations:
2x + 3y = 5 4x - y = 3
This system can be represented in matrix form as:
[ 2 3 ] [ x ] = [ 5 ] [ 4 -1 ] [ y ] = [ 3 ]
To solve this system, you can use the inverse of the coefficient matrix. First, find the inverse of the matrix:
Matrix A = [ 2 3 ] [ 4 -1 ] Inverse of A = 1/(2 * -1 - 3 * 4) * [ -1 -3 ] [ -4 2 ]
Once you have the inverse matrix, multiply it by the constants matrix to find the values of x and y. This method allows you to quickly and efficiently solve the system of equations.
By using matrices to organize and solve problems, you can simplify complex tasks and handle large datasets with ease. Mastering matrix operations enhances your ability to solve a wide variety of real-world challenges across different disciplines.
Practice Problems for Algebra 2 Mastery
Regular practice is essential to mastering the concepts and techniques needed to solve advanced mathematical problems. By working through various types of exercises, you can develop a deeper understanding of how to approach and solve problems. This section provides a variety of practice problems that cover key topics, helping you strengthen your skills and improve problem-solving efficiency.
Each problem is designed to challenge your understanding of different concepts and encourage you to apply different strategies. Solving these exercises will provide valuable practice that is necessary for building confidence and proficiency in solving complex equations and expressions.
Problem Set 1: Quadratic Equations
1. Solve the quadratic equation using the quadratic formula:
3x² + 7x - 4 = 0
2. Factor the following quadratic expression:
x² - 5x + 6
3. Complete the square to solve the quadratic equation:
x² + 8x - 20 = 0
Problem Set 2: Rational Expressions
1. Simplify the following rational expression:
(4x² - 9) / (2x + 3)
2. Solve for x in the equation:
(3 / x) + 2 = 7
Problem Set 3: Exponential Functions
1. Solve the following equation involving an exponential function:
5^(2x) = 25
2. Simplify the expression:
3^(x + 2) / 3^(x - 1)
Problem Set 4: Systems of Equations
1. Solve the system of equations using substitution:
2x + y = 10 3x - y = 5
2. Solve the system of equations using elimination:
4x - 3y = 12 2x + y = 10
Problem Set 5: Rational Functions
1. Simplify the rational function:
(2x - 3) / (x² - 9)
2. Find the vertical asymptotes of the rational function:
f(x) = (x + 1) / (x² - 4)
By consistently working through these problems and reviewing solutions, you will reinforce your understanding and improve your ability to tackle similar challenges with confidence. Mastery comes with time, practice, and persistence.
Preparing for Algebra 2 Assessments
Effective preparation is key to excelling in any evaluation. When it comes to advanced mathematical assessments, understanding the core concepts and practicing problem-solving techniques are crucial steps towards success. The goal is to build both proficiency and confidence, ensuring that you can tackle questions with clarity and efficiency.
Reviewing Key Concepts: Focus on mastering foundational topics such as polynomial functions, rational expressions, and quadratic equations. Ensure that you are familiar with various methods like factoring, using the quadratic formula, and simplifying rational expressions. Recognizing the underlying principles behind each type of problem will make it easier to solve them under timed conditions.
Practice Consistently: The more problems you solve, the more comfortable you will become with the techniques and strategies needed to answer questions quickly. Consider practicing problems from previous exams, or using problem sets from different resources. The key is repetition and familiarity with various question types.
Time Management: During the assessment, it’s important to pace yourself. Start with the easier questions to build confidence, and then move on to the more challenging ones. Allocating time to review your answers at the end can help catch any small errors before submission.
Test-Taking Strategies: When approaching each problem, read the instructions carefully and take note of any specific requirements. For complex problems, break them down into smaller, more manageable steps. Don’t rush–take the time to understand what each question is asking before diving into calculations.
Utilizing Resources: Use textbooks, online practice tools, or even study groups to reinforce your understanding. Discussing tricky concepts with peers or instructors can offer new insights and help clarify difficult topics.
By combining a solid review of essential topics with effective practice, time management, and strategic test-taking techniques, you’ll be well-prepared to succeed in any assessment related to advanced mathematical subjects.