Understanding the principles behind various figures and spatial reasoning is essential for developing strong problem-solving skills. Whether it’s finding angles, calculating areas, or understanding relationships between different shapes, mastering these concepts builds a solid foundation for more advanced studies. With the right approach and practice, tackling even the most complex problems becomes more manageable.
In this guide, we break down essential techniques and strategies for navigating through exercises that focus on space, structure, and measurement. Each section aims to provide clarity and practical tips for solving various challenges that may arise while studying. From basic formulas to intricate relationships, the goal is to equip learners with the tools they need for success.
Effective learning requires patience and persistence. Embracing these core strategies will not only help with immediate tasks but also foster a deeper understanding of mathematical thinking and problem-solving that can be applied in everyday situations.
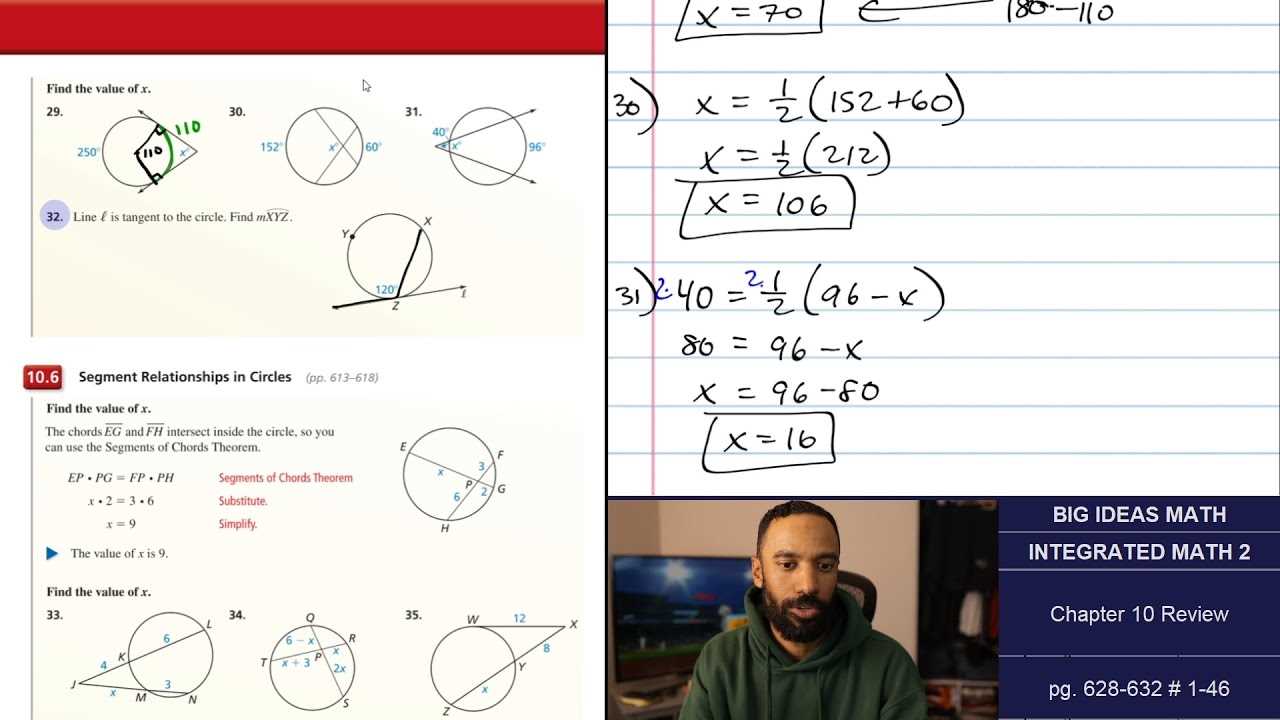
Big Ideas Math Geometry Solutions

Mastering concepts related to spatial relationships and measurements is essential for successfully tackling various challenges. To navigate through these exercises effectively, it’s crucial to understand the underlying principles that guide calculations and problem-solving strategies. By applying the right methods and tools, learners can break down complex tasks into simpler steps, leading to clearer solutions.
Comprehensive solutions provide the necessary framework to solve different types of problems, from basic calculations to more intricate challenges involving shapes, angles, and areas. Using a structured approach, it becomes easier to approach even the most complicated questions with confidence. Systematic techniques help avoid common mistakes and enhance overall accuracy.
Working through various problems and reviewing solutions not only strengthens comprehension but also encourages the development of critical thinking skills. With consistent practice and a focus on key principles, learners can significantly improve their understanding of how geometric principles are applied in real-world contexts.
Understanding Geometry Concepts
Grasping the core principles of shapes, spaces, and their relationships is essential for solving various challenges effectively. By familiarizing oneself with these fundamental ideas, it’s easier to identify patterns and make connections between different concepts. A strong foundation in these principles not only helps in tackling exercises but also enhances analytical thinking.
Some of the most important topics to focus on include:
- Properties of different shapes (triangles, squares, circles, etc.)
- Understanding angles and their relationships
- Measurement of areas and volumes
- Coordinate systems and graphing
- Transformations and symmetry
Each of these concepts forms the building blocks for solving more complex problems. By practicing these fundamental ideas, learners develop a clearer understanding of how they fit together and how they can be applied in a variety of scenarios. This knowledge is not only crucial for academic purposes but also for practical problem-solving in everyday life.
Key Topics in Geometry Explained
Understanding the key topics related to shapes, sizes, and spatial relationships is crucial for solving a wide range of problems. These core principles serve as the foundation for more complex concepts and help develop strong problem-solving skills. In this section, we break down some of the most essential ideas that every learner should focus on to build a solid understanding.
| Topic | Description |
|---|---|
| Shapes and Properties | Learn about different figures, their characteristics, and how to classify them based on sides, angles, and symmetry. |
| Angles and Measurement | Understand various types of angles (acute, obtuse, right) and how to measure them accurately in different contexts. |
| Area and Perimeter | Master the formulas to calculate the area and perimeter of common shapes such as squares, rectangles, and circles. |
| Volume and Surface Area | Learn how to determine the volume and surface area of three-dimensional objects like cubes, spheres, and cylinders. |
| Transformations | Explore various transformations such as rotations, reflections, and translations, and their impact on shapes. |
Each of these topics provides a key element in developing a comprehensive understanding of spatial reasoning and measurement. With consistent practice, learners can gain the confidence to solve problems across different difficulty levels and apply these principles in real-world situations.
Solving Geometry Problems Effectively
Approaching spatial problems with a systematic method can significantly improve accuracy and efficiency. By breaking down complex questions into smaller, manageable steps, it’s possible to reach the solution more quickly and with fewer errors. In this section, we focus on effective strategies and techniques for tackling common problems involving shapes, measurements, and angles.
Key Strategies for Success
To solve problems effectively, it’s important to follow a structured approach:
| Strategy | Explanation |
|---|---|
| Understand the Problem | Read through the problem carefully to identify what is being asked and the known information. |
| Draw Diagrams | Visualize the problem by sketching the shapes and labeling key points, angles, and dimensions. |
| Apply Formulas | Use relevant formulas for area, perimeter, volume, or angles to solve for unknowns. |
| Check Your Work | After solving, verify the calculations and ensure the solution is reasonable within the context of the problem. |
Common Pitfalls to Avoid
Even with the best strategies, some challenges can arise. Here are common mistakes to watch out for:
| Mistake | How to Avoid It |
|---|---|
| Skipping Diagramming | Always draw a diagram to visualize the problem and help understand spatial relationships. |
| Rushing through Calculations | Take your time with each step to avoid miscalculations and ensure accuracy. |
| Ignoring Units | Double-check that the units are consistent throughout your work to avoid errors in your final result. |
By incorporating these strategies and avoiding common mistakes, solving spatial problems becomes a more manageable and efficient process. Practice and careful attention to detail will lead to greater success in this area of study.
Step-by-Step Approach to Geometry
A clear, methodical approach is essential when tackling problems involving shapes, space, and measurements. By breaking down each question into logical steps, you can systematically apply principles to find the solution. This structured process not only simplifies complex challenges but also helps in understanding how different concepts interconnect.
Here is a step-by-step approach to solving problems effectively:
- Read and Analyze the Problem: Carefully read the problem statement to understand what is being asked and what information is given.
- Visualize the Situation: Draw a diagram of the shapes involved, labeling important points, angles, and dimensions where necessary.
- Identify Relevant Formulas: Determine which formulas or theorems are applicable to the problem, such as those for area, perimeter, or volume.
- Perform Calculations: Using the identified formulas, substitute the known values and perform the necessary calculations step by step.
- Double-Check the Work: After obtaining the result, review the calculations to ensure accuracy and verify that the solution is logical in the context of the problem.
By following these steps, learners can reduce errors and approach each challenge in a logical and organized way. With practice, this method becomes intuitive, allowing for more efficient problem-solving in various situations.
Geometry Formulas You Need to Know
To solve problems involving shapes, sizes, and spatial relationships, it’s essential to be familiar with the key formulas that apply to various figures. These equations are foundational tools that allow you to compute areas, perimeters, volumes, and other important properties. Understanding and memorizing these formulas is crucial for effectively solving related challenges.
| Figure | Formula | Explanation |
|---|---|---|
| Circle | Area = πr² | Calculates the area inside a circle, where r is the radius. |
| Rectangle | Area = length × width | Finds the area of a rectangle by multiplying the length by the width. |
| Triangle | Area = ½ × base × height | Computes the area of a triangle, using the base and height values. |
| Square | Area = side² | Finds the area of a square by squaring the length of one side. |
| Sphere | Volume = 4/3 × πr³ | Determines the volume of a sphere, where r is the radius. |
| Cube | Volume = side³ | Calculates the volume of a cube by cubing the length of one side. |
| Cylinder | Volume = πr²h | Finds the volume of a cylinder, where r is the radius and h is the height. |
| Rectangle (Perimeter) | Perimeter = 2 × (length + width) | Computes the perimeter of a rectangle by adding the length and width and multiplying by 2. |
These formulas are the most commonly used and serve as the foundation for solving a variety of spatial problems. By becoming familiar with them and practicing their application, you’ll be well-equipped to handle more complex challenges in this field.
Common Mistakes in Geometry Problems
Even experienced learners can make mistakes when solving problems related to shapes, sizes, and spatial reasoning. Recognizing and understanding these common errors can help you avoid them in the future and improve your overall problem-solving skills. Below are some of the most frequent pitfalls that students encounter and tips on how to avoid them.
Frequent Errors in Calculation
- Incorrect Formula Application: Using the wrong formula or applying it incorrectly can lead to incorrect results. Always double-check which formula is relevant for the problem you’re solving.
- Misunderstanding Units: Failing to convert units properly can result in errors. Ensure that all units are consistent before performing calculations.
- Forgetting to Square or Cube Values: Sometimes students forget to square or cube values when working with areas or volumes, leading to inaccurate solutions.
Errors in Visualizing the Problem

- Omitting a Diagram: Not drawing a diagram can make it hard to visualize the problem. Always sketch out the shapes and label the given information.
- Confusing Angles: Mixing up types of angles (acute, obtuse, right) or failing to apply angle relationships properly can cause mistakes, especially when working with polygons.
- Overlooking Symmetry: Ignoring symmetry can result in missed shortcuts for solving problems. Recognizing symmetrical properties can simplify your calculations significantly.
By staying mindful of these common errors and applying careful attention to each step, you can reduce mistakes and improve your accuracy when solving problems in this field. Practice, along with a clear understanding of the concepts, is key to overcoming these challenges.
Tips for Mastering Geometry Skills
To excel in spatial reasoning and problem-solving, it’s essential to develop a strong grasp of the core principles and techniques. Consistent practice, effective strategies, and a solid understanding of key concepts will help you navigate even the most challenging problems with confidence. In this section, we’ll explore some valuable tips to enhance your proficiency in this area.
Strengthen Your Foundation
Before diving into complex problems, make sure you have a solid understanding of the basic concepts:
- Master Basic Shapes and Properties: Understand the key characteristics of common shapes such as squares, triangles, and circles. Knowing their properties will help you solve problems more efficiently.
- Learn Essential Formulas: Familiarize yourself with important equations for area, volume, and perimeter. A strong command of these formulas is critical for solving related problems.
- Practice Visualization: Develop your ability to visualize shapes and structures in your mind. This skill will help you understand spatial relationships and simplify problem-solving.
Develop Effective Problem-Solving Strategies
Along with mastering the basics, it’s important to adopt effective strategies for tackling more complex challenges:
- Break Down Problems: Divide each problem into smaller, more manageable parts. This approach makes it easier to identify the necessary steps and find a solution.
- Draw Diagrams: Visualizing the problem by drawing accurate diagrams can often reveal useful insights and make the process less abstract.
- Review and Reflect: After solving a problem, take time to review your work. Reflecting on what worked well and where mistakes were made will help you avoid similar issues in the future.
By applying these tips consistently and practicing regularly, you’ll build the skills needed to master this area and approach even the most difficult problems with ease and confidence.
How to Improve Geometry Problem-Solving
To enhance your ability to tackle problems involving shapes, spaces, and measurements, it’s essential to refine both your conceptual understanding and problem-solving techniques. Effective problem-solving requires not only knowledge of formulas but also the ability to approach each question methodically. With consistent practice and the right strategies, you can develop the skills to solve even the most complex challenges with ease.
Focus on Understanding Concepts
It’s crucial to build a solid foundation of the core principles involved in spatial reasoning. Here are some ways to strengthen your understanding:
- Study Core Theorems and Postulates: Understanding foundational concepts such as Pythagoras’ Theorem or the properties of parallel lines will help you recognize patterns in problems.
- Visualize Problems: Always try to draw diagrams or sketches. Visualizing the problem makes it easier to identify relationships and plan your approach.
- Memorize Key Formulas: Knowing the essential equations for areas, volumes, and perimeters will save time when solving problems and allow you to focus on more complex steps.
Adopt Effective Problem-Solving Strategies
Alongside knowledge, adopting a strategic approach is key to solving problems effectively:
- Break Down Complex Problems: Don’t try to solve everything in one step. Break the problem into smaller parts to make it easier to address each component.
- Use Process of Elimination: When unsure of the next step, eliminate clearly incorrect choices or steps. This can help narrow down the possibilities and guide you toward the right solution.
- Review Mistakes and Learn: After solving a problem, review any errors. Understanding what went wrong and why is one of the best ways to improve your skills.
By consistently applying these strategies, you’ll see steady improvement in your problem-solving ability. With time, complex challenges will seem more manageable, and your confidence in approaching spatial problems will grow significantly.
Geometry Visuals and Diagrams Explained
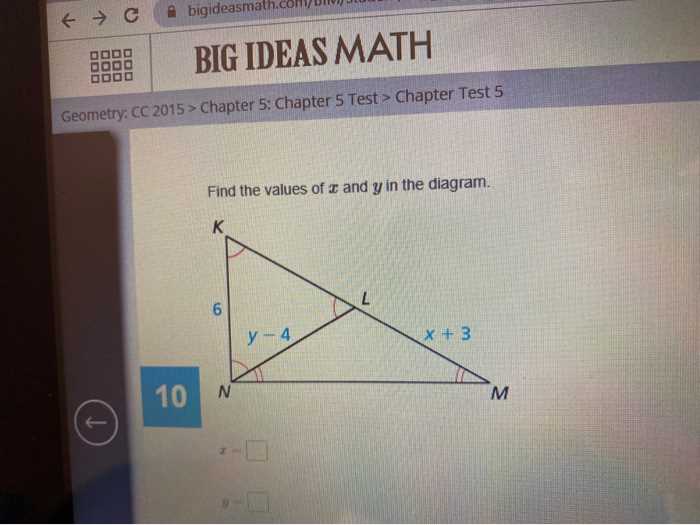
Understanding the visual aspects of spatial problems is crucial for effective problem-solving. Diagrams and illustrations provide clarity, enabling you to better comprehend relationships between elements and make more accurate calculations. By mastering how to read and create these visuals, you’ll improve your ability to interpret and solve complex challenges efficiently.
Importance of Accurate Diagrams
Diagrams serve as a visual aid that helps to translate abstract concepts into something tangible. Here’s why they are essential:
- Clarify Relationships: Diagrams allow you to see how different parts of a figure relate to one another, such as angles, lines, and distances.
- Simplify Complex Problems: Visual representations break down a problem into smaller, more understandable pieces, making it easier to apply the correct formulas or theorems.
- Highlight Key Information: Important elements, such as side lengths, angles, or areas, can be marked in a diagram, reducing the risk of overlooking critical details.
Creating and Interpreting Diagrams
Being able to both interpret and create your own diagrams is an essential skill. Here are some tips for improving this ability:
- Draw to Scale: When possible, ensure that your diagrams are drawn to scale to accurately represent the relationships between parts of the figure.
- Label Clearly: Always label key elements, such as points, lines, and angles, to avoid confusion during calculations or when applying geometric principles.
- Use Color or Shading: Color coding or shading different parts of a diagram can help emphasize certain areas, angles, or regions for easier identification during problem-solving.
By mastering the use of diagrams and visuals, you’ll be able to approach problems with greater confidence and precision, turning complex challenges into manageable tasks.
Application of Geometry in Real Life
The principles of shapes, space, and measurement are not confined to the classroom. They are deeply embedded in many aspects of everyday life, influencing everything from construction and design to navigation and technology. Recognizing the practical uses of these concepts can help you better appreciate their importance and improve your problem-solving skills in real-world scenarios.
Architecture and Construction
One of the most prominent uses of spatial reasoning is in building design and construction. Accurate measurements and an understanding of structure are critical in creating safe, efficient, and aesthetically pleasing buildings and infrastructure.
- Building Layouts: Architects rely on geometric principles to design floor plans, ensuring that spaces are both functional and safe. Precise measurements help to optimize the use of space.
- Structural Integrity: Engineers use shapes like triangles and rectangles to ensure that structures can support weight and withstand environmental factors such as wind or earthquakes.
- Landscaping: Designers use shapes and measurements to create visually appealing gardens, walkways, and outdoor spaces, making efficient use of the land’s natural features.
Technology and Digital Design
The influence of spatial reasoning is also evident in technology, particularly in areas like computer graphics, animation, and 3D modeling. Here, geometric algorithms are used to create realistic simulations and user interfaces.
- Computer Graphics: Video games and movies use geometric shapes to model characters and environments, enabling lifelike movement and interactions.
- Virtual Reality: Virtual worlds are built on the principles of space and distance, allowing users to interact with environments in a way that feels immersive and accurate.
- Architecture Software: Programs like AutoCAD and SketchUp rely on geometric algorithms to help architects and designers create detailed, three-dimensional models of buildings before construction begins.
By understanding how shapes and spatial reasoning play a role in the real world, you can see that these concepts are not just abstract ideas–they are essential tools for shaping the world around us.
Common Geometry Questions Answered
Many individuals encounter challenges when dealing with spatial relationships and measurements, often leaving them with lingering questions. By addressing some of the most frequently asked queries, this section aims to provide clarity and simplify common misconceptions, helping you build a stronger understanding of these essential concepts.
1. How do you calculate the area of irregular shapes?
For irregular shapes, divide them into simpler, familiar figures such as rectangles, triangles, or circles. Calculate the area for each part, then sum the areas to find the total. This method, known as decomposition, is highly effective for complex shapes.
2. What is the difference between perimeter and area?
The perimeter is the total length around a figure, while the area refers to the space contained within that figure. For example, a square’s perimeter is the sum of all its sides, whereas the area is found by squaring the length of one side.
3. Why are angles important in problem-solving?
Angles help determine relationships between lines, shapes, and surfaces, making them crucial in many practical applications. Understanding how angles affect a figure’s structure can guide you in calculating distances, areas, and more.
4. How do you find the volume of 3D shapes?
The volume of three-dimensional objects can be calculated by multiplying length, width, and height for rectangular solids or using specific formulas for other shapes like spheres and cylinders. Each type of solid has its own unique equation, tailored to its dimensions.
5. What is the significance of symmetry?
Symmetry plays a vital role in many fields, from design to nature. It refers to the balance and proportion of parts within an object or figure. Identifying symmetry helps in solving problems related to balance, angles, and even simplifying complex shapes.
By answering these common questions, we aim to clear up some of the confusion that often arises when studying spatial relationships and measurements, giving you the tools to approach new challenges with greater confidence and precision.
How Geometry Relates to Other Subjects
The study of shapes, space, and measurements goes far beyond its own field. These concepts are interconnected with various other disciplines, helping to shape our understanding of the world in multiple ways. Recognizing how spatial reasoning connects with other subjects can enhance your ability to solve problems and apply knowledge across a range of fields.
Relationship with Physics
In physics, understanding the properties of space and matter is crucial. Many physical principles rely on geometric concepts to describe the motion of objects, forces, and energy transfer. For example, vectors, which represent quantities such as velocity or force, are visualized using arrows in a coordinate system, a fundamental geometric concept. Additionally, the study of waves, optics, and mechanics often involves geometric reasoning to understand the behavior of light, sound, and objects in motion.
Connection to Art and Design
The principles of spatial relationships are essential in visual arts, architecture, and design. Artists use geometric shapes to create aesthetically pleasing compositions, whether through symmetry, proportion, or perspective. Architects and designers apply similar concepts to create functional and visually striking structures. The use of scale, proportion, and symmetry can transform a simple design into a work of beauty.
Understanding how these ideas relate to one another not only broadens your knowledge but also allows for more effective application in various real-world scenarios. Whether you’re solving a complex physics problem or designing a building, the underlying principles remain deeply interconnected.
Geometry Test Preparation Strategies
Preparing for a test on spatial relationships, shapes, and measurements requires a focused approach. Effective preparation not only helps you recall formulas and concepts but also improves your ability to apply them in real-world scenarios. A clear strategy can make a significant difference in your performance on these assessments.
Organizing Study Sessions
To maximize your study time, create a structured plan that covers all the necessary topics. Break down the material into smaller sections and focus on one at a time. Here are some key strategies to follow:
- Review Key Formulas: Make a list of the most important equations, such as area, perimeter, volume, and angle relationships, and memorize them. Practice applying these formulas to various problems.
- Practice with Examples: Work through sample problems that cover a range of difficulty levels. This will help you identify patterns and familiarize yourself with problem-solving techniques.
- Use Visual Aids: Diagrams, charts, and visual representations can help you better understand concepts, especially in areas like shapes and their properties.
- Take Breaks: Avoid long, uninterrupted study sessions. Short breaks help maintain focus and prevent burnout.
Active Problem-Solving Techniques
Merely reading through notes is not enough to prepare for an exam. Active engagement with the material is crucial for mastering the subject:
- Work with Peers: Study with classmates or friends to discuss challenging problems. Explaining concepts to others can solidify your own understanding.
- Simulate Test Conditions: Practice under timed conditions to get used to the pressure of the actual exam. This can improve your time management skills.
- Identify Weak Areas: Focus on areas where you struggle the most. Spend extra time on these topics to ensure you’re well-prepared for any surprises on the test.
By combining a structured study plan with active problem-solving practice, you can build both confidence and competence, leading to improved performance on your test.
Advanced Geometry Concepts You Should Know
To truly master the study of shapes, space, and their properties, it’s important to explore advanced principles that extend beyond basic concepts. These advanced ideas not only help solve complex problems but also provide a deeper understanding of how different aspects of space interact with each other. By familiarizing yourself with these principles, you can tackle more sophisticated challenges with ease.
Key Advanced Topics
The following are some of the key advanced concepts that can broaden your understanding and improve your problem-solving skills:
- Transformation Geometry: This includes translations, rotations, reflections, and dilations, which help analyze the effects of changing an object’s position or size within a plane.
- Non-Euclidean Geometry: Explore geometries that deviate from the traditional flat plane model, such as spherical or hyperbolic geometry, to understand space in a more abstract way.
- Coordinate Geometry: This concept uses algebraic equations to represent geometric shapes on a coordinate plane, blending algebra with geometric concepts for more detailed analysis.
- Solid Geometry: This branch deals with three-dimensional objects, such as spheres, cylinders, and cones, focusing on their properties, surface areas, and volumes.
- Vector Geometry: This concept introduces vectors to represent points, lines, and other geometric objects, allowing for a more powerful way to approach problems in higher dimensions.
Table: Important Properties of 3D Shapes
| Shape | Volume Formula | Surface Area Formula |
|---|---|---|
| Cube | V = a³ | SA = 6a² |
| Cylinder | V = πr²h | SA = 2πrh + 2πr² |
| Sphere | V = (4/3)πr³ | SA = 4πr² |
Understanding these advanced principles opens the door to solving more intricate problems and furthering your mastery of spatial relationships. Whether for academic or real-world applications, mastering these ideas enhances your ability to think critically and solve complex puzzles effectively.
Best Resources for Geometry Learning
When it comes to mastering the study of shapes, space, and their properties, using the right materials and tools can make a significant difference. Whether you’re a beginner or looking to deepen your understanding, there are numerous resources available to help guide you through complex concepts and problem-solving techniques. Below are some of the best learning resources to help you excel in this area.
Top Online Platforms
Online platforms offer interactive lessons, tutorials, and practice problems that cater to various learning styles. Here are some of the most effective options:
- Khan Academy: Free video lessons and exercises that cover all skill levels, from basic principles to advanced topics.
- Coursera: Courses from top universities and institutions, often featuring in-depth modules on more advanced aspects of spatial reasoning.
- EdX: Offers free and paid courses, focusing on both basic and advanced spatial concepts with quizzes and peer discussions.
- Brilliant: Interactive problem-solving and challenging puzzles designed to enhance understanding and application of spatial concepts.
Books and Guides
Books can provide structured learning and detailed explanations of key concepts. Here are some of the most useful books for learning:
- “Geometry for Dummies” by Mark Ryan: A beginner-friendly resource that simplifies even the most complex topics, with clear examples and practical applications.
- “Euclidean and Non-Euclidean Geometries” by Marvin J. Greenberg: For those interested in exploring more advanced concepts and alternative models of space.
- “The Art of Problem Solving: Volume 1” by Richard Rusczyk: A comprehensive guide for students who wish to develop problem-solving skills in geometric contexts.
- “How to Solve It” by George Pólya: A classic that focuses on the strategies and methods for solving mathematical problems, with a significant emphasis on geometric reasoning.
These resources combine theory, practice, and critical thinking exercises to ensure a comprehensive understanding of spatial relationships. Whether through hands-on interaction, visual aids, or theoretical exploration, utilizing a variety of learning materials can help improve your skills and confidence in this field.
Overcoming Challenges in Geometry Studies
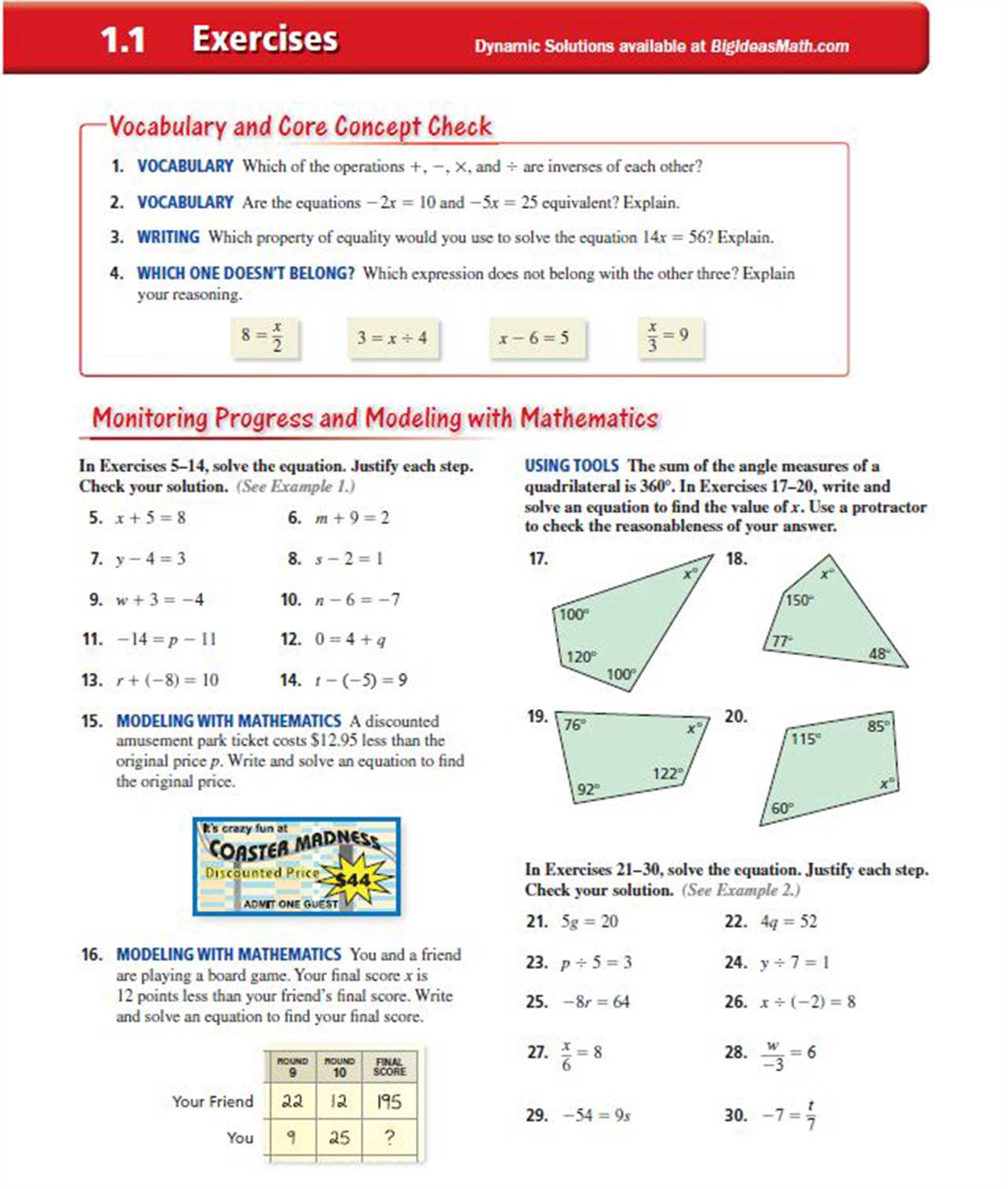
Learning the principles that govern shapes, angles, and their relationships can often present obstacles for many students. Concepts such as spatial reasoning, theorem applications, and visualizing complex figures can be intimidating at first. However, with the right strategies and mindset, these challenges can be overcome. Below are some effective approaches to navigate difficulties and master this subject with confidence.
Understanding and Visualizing Complex Figures
One of the most common hurdles in this field is the difficulty of visualizing three-dimensional objects or abstract diagrams. To tackle this challenge:
- Use physical models: Manipulating physical representations of geometric shapes can make abstract concepts more tangible.
- Draw diagrams: Sketching diagrams by hand or using digital tools can help solidify spatial understanding.
- Break down complex problems: Simplify complicated figures by breaking them into smaller, more manageable parts.
Improving Problem-Solving Techniques
Solving problems can feel daunting when you don’t know where to start. To improve your problem-solving ability:
- Master the basics: Ensure a solid understanding of foundational concepts before tackling advanced topics.
- Practice regularly: The more problems you solve, the more intuitive the process becomes.
- Work through examples: Reviewing solved examples helps understand the step-by-step approach needed to arrive at the solution.
With consistent practice and the application of these strategies, you can turn challenging topics into manageable ones and gain a stronger command over this area of study.