
Preparing for advanced placement exams requires a thorough understanding of the key concepts and effective problem-solving strategies. By familiarizing yourself with the types of questions commonly found on these tests, you can improve your performance and boost your confidence. The multiple-choice section of the exam often poses challenges that require careful analysis and quick thinking.
Exam preparation involves not only reviewing core principles but also practicing with real test questions. In this article, we will explore detailed solutions to questions from a past exam, providing a step-by-step breakdown of how to approach each problem. The goal is to help you understand the reasoning behind each solution and develop strategies for tackling similar questions in the future.
Whether you’re a first-time test-taker or looking to refine your skills, the resources provided here will guide you in improving both your speed and accuracy. With the right techniques and practice, you’ll be better equipped to handle the challenges the exam presents.
1998 AP Calculus AB Multiple Choice Answers
Success in advanced placement exams relies on a deep understanding of key mathematical concepts and effective problem-solving methods. The questions presented in these tests challenge students to apply their knowledge in a structured and time-sensitive manner. By reviewing past test questions and breaking down their solutions, students can gain insight into the types of problems they may encounter and the best ways to tackle them.
Breaking Down the Problems
Each question requires a clear approach to identify the correct solution. For some, the process may involve simplifying complex expressions or applying well-known formulas. Understanding the reasoning behind the correct answers is crucial for improving performance in future exams. In this section, we will examine a range of sample questions from the past test and explore how to reach the right conclusion.
Effective Problem-Solving Techniques
Mastering problem-solving techniques is essential for navigating these exams efficiently. It’s not enough to simply recall formulas; one must also understand how and when to apply them effectively. The detailed walkthroughs provided here aim to strengthen problem-solving skills, ensuring that students can confidently approach similar questions in upcoming assessments.
Overview of 1998 AP Calculus Exam
The advanced placement exam tests a wide range of mathematical knowledge and the ability to apply it under time constraints. Students are challenged to demonstrate proficiency in various mathematical concepts, from basic principles to more advanced techniques. By analyzing the structure and content of past exams, students can gain a clearer understanding of what to expect and how best to prepare.
Exam Structure and Content
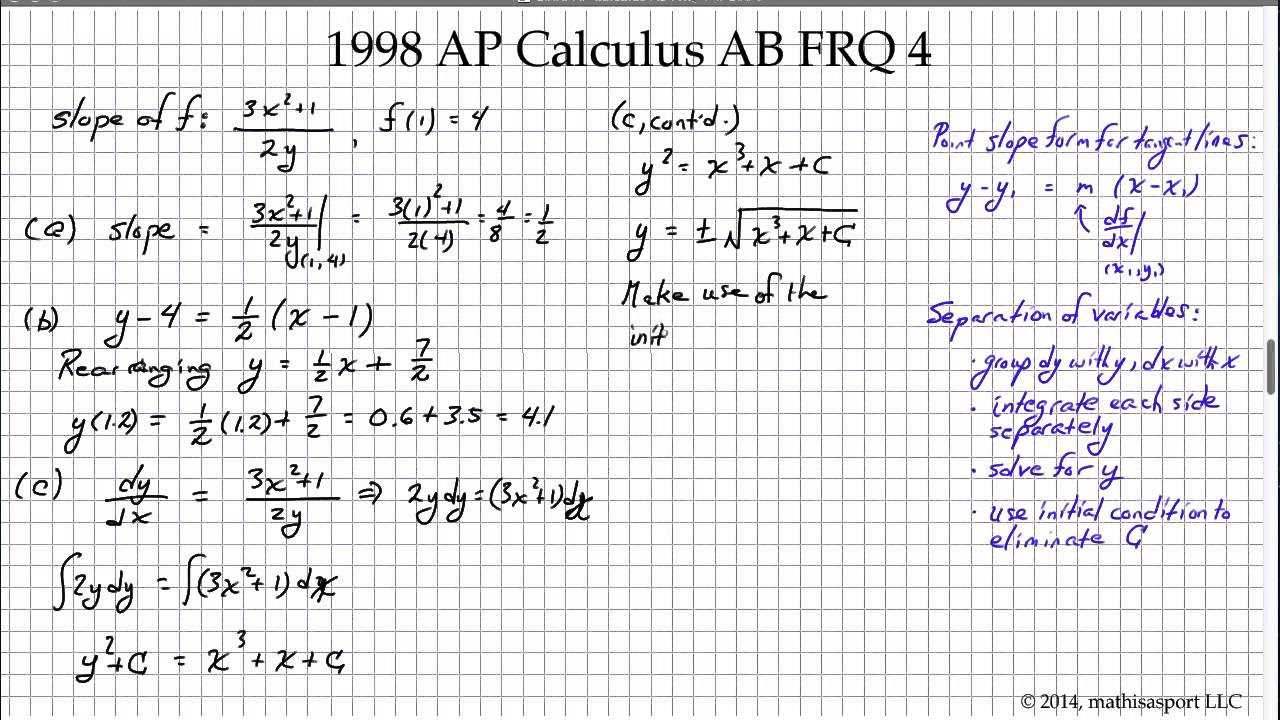
The test is divided into several sections, each designed to assess different aspects of mathematical understanding. The questions typically involve a mix of theoretical concepts and practical problem-solving. These problems require not only a solid grasp of formulas but also the ability to think critically and solve complex problems quickly and accurately.
Key Areas of Focus
Important topics covered in the exam include differential and integral calculus, functions, limits, and the application of mathematical concepts to real-world scenarios. Mastery of these areas is essential for achieving a high score. In this section, we will highlight the most frequently tested topics and provide guidance on how to approach them effectively.
Key Topics Covered in the Exam
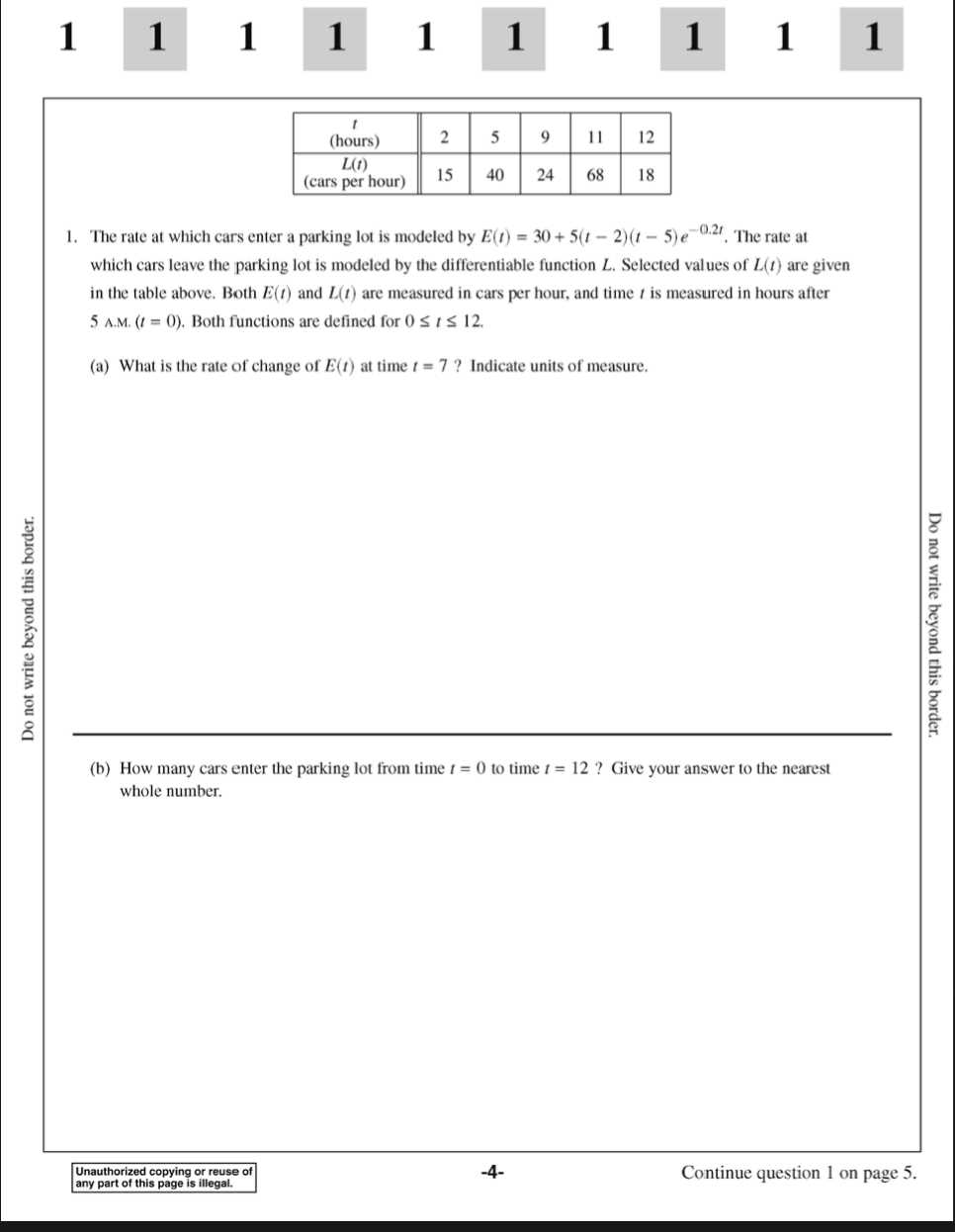
The advanced placement exam covers a wide array of topics that test a student’s mathematical understanding and ability to solve complex problems. These areas are designed to challenge both theoretical knowledge and practical application skills. Mastery of these topics is essential for performing well on the exam, as each section requires a unique set of techniques and strategies.
Fundamentals of Functions and Limits
One of the core areas tested involves understanding and working with functions. Students must be able to interpret and manipulate various types of functions, including polynomials, rational functions, and exponential equations. Additionally, limits play a crucial role in understanding the behavior of functions as they approach certain values, which is vital for solving problems related to continuity and differentiability.
Differentiation and Integration Techniques
Another critical area is the application of differentiation and integration techniques. These fundamental concepts are tested through problems that require students to find derivatives and integrals of various expressions. Understanding how to apply the power rule, product rule, and quotient rule, as well as techniques for solving definite and indefinite integrals, is key to succeeding in this section of the exam.
How to Approach Multiple Choice Questions
When tackling objective test questions, it’s essential to have a systematic approach that maximizes your chances of success. These questions are designed to test both your understanding of key concepts and your ability to solve problems efficiently. Knowing how to navigate them effectively can help you save time and reduce errors.
Step-by-Step Strategy for Solving Problems
To approach these types of questions successfully, follow these steps:
- Read each question carefully: Ensure you fully understand what’s being asked before considering the possible answers.
- Eliminate clearly wrong options: Start by crossing out answers that are obviously incorrect, which narrows down your choices.
- Look for key clues: Often, questions will provide hints in the wording that can guide you toward the correct answer.
- Double-check your calculations: If the question involves computation, ensure all math is done accurately before selecting an answer.
- Manage your time: Don’t spend too long on any one question. If you’re unsure, make an educated guess and move on.
Common Pitfalls to Avoid
While solving objective questions, be mindful of common mistakes:
- Misreading the question: Sometimes, a small detail can change the entire meaning of a question. Read carefully.
- Overthinking: If a question seems too complicated, it may be easier than you think. Trust your instincts and avoid overcomplicating it.
- Skipping difficult questions: Don’t leave hard questions for the end. If you don’t know the answer, guess and move on.
Common Pitfalls in AP Calculus Tests
While preparing for advanced placement exams, students often fall into several common traps that can negatively impact their performance. These pitfalls range from simple mistakes in calculations to more complex issues like misinterpreting questions or rushing through sections. Being aware of these challenges and learning how to avoid them is crucial for achieving a high score.
Here are some of the most frequent mistakes and how to avoid them:
| Pitfall | How to Avoid It |
|---|---|
| Misinterpreting the question | Take extra time to read the problem carefully, and highlight key terms that can guide your solution. |
| Rushing through calculations | Double-check your math and don’t skip steps, especially in complex problems. |
| Overcomplicating the solution | Start with a straightforward approach. If the answer seems complicated, check for simpler alternatives. |
| Forgetting to apply key concepts | Review core concepts before the exam and ensure you recognize when to apply them during the test. |
| Not managing time effectively | Be mindful of the time for each section. Don’t dwell too long on any single question. |
By recognizing these common pitfalls and implementing strategies to avoid them, students can improve their efficiency and accuracy during the exam, ultimately leading to better results.
Detailed Solutions for 1998 Questions
To truly master the content tested in advanced placement exams, it’s important to not only review correct answers but to understand the reasoning behind each solution. In this section, we will walk through detailed solutions for some of the questions from past exams. By analyzing each problem step by step, you will gain valuable insights into how to approach similar problems and improve your problem-solving skills.
Step-by-Step Breakdown
For each question, we will provide a thorough explanation of the steps involved in reaching the correct solution. This will help you understand the logic behind the methods used and enable you to apply these strategies in future tests. Below is an example of how we break down complex problems:
- Read the question carefully: Begin by understanding what is being asked, identifying key information.
- Choose the appropriate strategy: Decide whether the problem requires algebraic manipulation, applying formulas, or another technique.
- Solve step-by-step: Break down the solution into smaller, manageable steps to avoid confusion.
- Check your work: Revisit the steps and calculations to ensure that each part of the solution is correct.
Example Solution
Consider a problem that asks you to find the derivative of a given function. Here’s how you might approach it:
- Step 1: Identify the type of function (e.g., polynomial, rational).
- Step 2: Apply the appropriate differentiation rule (e.g., power rule, product rule).
- Step 3: Simplify the result, and check for any algebraic errors.
By breaking down each question in this way, you will improve your ability to solve similar problems under exam conditions and ensure that you don’t miss important steps.
Strategies for Solving AP Calculus Problems

When approaching complex problems on advanced placement exams, having a set of effective strategies can make a significant difference. The ability to break down problems into manageable steps and recognize patterns is essential for solving each question efficiently. In this section, we will explore proven methods that help improve problem-solving accuracy and speed, ensuring you are well-prepared for any challenge.
By applying the right techniques, you can maximize your efficiency and boost your confidence during the exam. Here are some key strategies to consider:
| Strategy | Description |
|---|---|
| Understand the Concepts | Ensure you have a strong grasp of fundamental mathematical concepts and how they apply to different types of problems. |
| Use Logical Reasoning | Always try to reason through problems step by step, making logical connections between each part of the question. |
| Break Complex Problems Into Parts | If a problem seems overwhelming, break it down into smaller parts and solve each part separately before combining them for the final solution. |
| Practice Estimation | In some cases, estimating the solution can help narrow down the options or give you a quick sense of whether your approach is correct. |
| Check Your Work | Review your steps and calculations after solving a problem to catch any small errors before submitting your answers. |
By consistently applying these strategies, you can build a methodical approach to solving problems, ultimately improving both speed and accuracy on the exam. The key is to practice regularly, refine your problem-solving skills, and stay calm under pressure.
Understanding the Scoring System
Knowing how your performance is evaluated in an advanced placement exam is essential for understanding how to approach the test strategically. The scoring system determines how your responses are graded and how those grades contribute to your final score. Being familiar with how points are awarded and deducted can help you manage your time more effectively during the test and improve your overall score.
How the Scoring Works
The scoring process takes into account both the accuracy and completeness of your responses. Understanding the allocation of points for different sections of the exam and how to maximize your score on each type of question is key. Here’s a breakdown of the scoring system:
| Section | Points per Question | Weight |
|---|---|---|
| Objective Section (Multiple Choice) | 1 point per correct answer | Varies based on difficulty and total number of questions |
| Free Response Section | Up to 9 points per question | Higher weight compared to objective questions |
Impact of Incorrect Responses
While correct answers earn you points, incorrect answers can have different consequences depending on the section. In some cases, no points are deducted for wrong responses, while in others, there may be penalties. It is important to know the specific rules for the exam you’re taking to avoid making unnecessary mistakes.
Being mindful of how your score is calculated allows you to allocate your time and effort effectively, helping you focus on areas that will yield the highest return. Understanding the scoring system also encourages a balanced approach–spending the right amount of time on each section without over-investing in one area.
Time Management Tips for the Exam
Effective time management is a crucial aspect of performing well in any exam. When you are faced with a series of complex problems, being able to allocate your time wisely can make the difference between finishing the test confidently and feeling rushed. In this section, we will explore strategies to help you maximize your time during the exam, ensuring you have enough time to tackle each question and review your work.
Properly managing your time allows you to focus on each question without the pressure of running out of time. The following tips will help you approach the test more efficiently:
- Understand the Exam Structure: Familiarize yourself with the format and the number of questions in each section. This helps you allocate your time based on the weight and complexity of each part.
- Set Time Limits for Each Section: Aim to spend a specific amount of time on each section. For instance, allocate more time to problems that require detailed solutions, and less time to simpler ones.
- Don’t Get Stuck on Hard Questions: If a question is proving difficult, move on and come back to it later. This ensures you don’t waste precious time on a single problem.
- Use Quick Estimations: For certain questions, estimating the solution can help you narrow down options quickly, saving time on more complex calculations.
- Review Your Work: Leave a few minutes at the end of the exam to go over your answers. This is the time to check for any errors or missed steps.
By implementing these time management strategies, you can approach the exam with more confidence and less stress. A clear, organized approach to managing your time will help you perform at your best, ensuring that you have enough time to tackle each section effectively and review your work before submitting your answers.
Practice Techniques for Success
Effective practice is essential for achieving success on any challenging exam. The key to mastering complex concepts is not just to study, but to practice in a way that builds understanding and hones your problem-solving skills. In this section, we will explore several practice techniques that can help you improve your performance and boost your confidence on test day.
By adopting the right practice methods, you can strengthen your abilities and avoid common pitfalls. Here are some techniques to incorporate into your study routine:
- Focus on Understanding, Not Just Memorization: It’s important to fully understand the underlying principles behind the problems you are practicing. This approach ensures you can apply your knowledge in various contexts during the exam.
- Simulate Exam Conditions: Practice under timed conditions to replicate the pressure of the actual test. This will help you manage your time effectively and work efficiently within the constraints.
- Review Mistakes Thoroughly: When you make a mistake, take the time to understand why it happened. Analyzing your errors will help you avoid repeating them and strengthen your overall problem-solving skills.
- Use a Variety of Resources: Don’t limit your practice to just one source. Use a range of materials, such as practice exams, textbooks, and online tools, to expose yourself to different types of problems and approaches.
- Break Down Complex Problems: Tackle difficult questions by breaking them into smaller, manageable parts. This will help you focus on each component and make the overall problem easier to solve.
Incorporating these techniques into your preparation can significantly improve your test-taking skills. By practicing regularly, focusing on understanding, and addressing areas of weakness, you’ll be better equipped to handle the challenges of the exam with confidence and efficiency.
AP Calculus AB Question Format Explained
Understanding the format of the questions on an exam is essential for effective preparation. The way questions are presented can significantly influence how you approach them. In this section, we will explore the structure of the questions typically encountered in an AP exam, providing you with insights on what to expect and how to prepare accordingly.
Each question is designed to test your comprehension and application of core mathematical concepts. The exam typically includes a variety of question types that require both problem-solving skills and the ability to demonstrate reasoning. Here’s a breakdown of how the questions are usually structured:
Types of Questions
Questions on the exam can be divided into two main types: conceptual and computational. Understanding the distinction between them will help you prioritize your study efforts.
- Conceptual Questions: These questions assess your understanding of fundamental principles. They often ask you to explain or identify the reasoning behind a particular concept, rather than simply solving a problem.
- Computational Questions: These require you to apply mathematical techniques to find the correct solution. They may involve algebraic manipulations, calculations, or geometric reasoning to arrive at an answer.
Answer Options
For each question, there are typically several possible answer choices. Only one answer is correct, and your goal is to choose the option that best matches your solution or reasoning. The key to answering these questions effectively is to be familiar with the question’s structure and to approach it systematically.
By understanding the format of the questions, you can better prepare yourself to navigate the exam efficiently. Practicing a variety of question types and honing your problem-solving strategies will increase your chances of success on test day.
Preparing for the Multiple Choice Section
Success in any exam depends not only on mastering the content but also on knowing how to approach each section effectively. The multiple choice part of the test is designed to assess your ability to quickly identify the correct solution from several options. In this section, we will focus on strategies that can help you perform well in this particular format.
Being well-prepared for the multiple selection portion of the exam requires more than just knowledge; it requires strategic thinking, efficient time management, and the ability to quickly assess each option. Below are some tips to guide your preparation:
Key Strategies for Success
- Read Each Question Carefully: Pay attention to the wording of the question and each answer option. Sometimes, small details in the phrasing can make a big difference in selecting the correct option.
- Eliminate Obvious Incorrect Answers: Quickly cross out answers that are clearly wrong. This increases your chances of guessing correctly if you need to skip a question and return to it later.
- Understand the Question Type: Identify whether the question requires a conceptual understanding, a calculation, or both. This will help you decide the best method to use when solving it.
- Manage Your Time: Allocate a specific amount of time to each question and move on if you’re stuck. It’s better to answer all questions, even with a guess, than to spend too much time on a single one.
Effective Practice Techniques
- Take Timed Practice Tests: Simulate the test environment by taking practice exams under timed conditions. This will help you get used to the pace of the exam and identify areas where you need improvement.
- Review Incorrect Answers: After each practice test, review the questions you got wrong. Understand why your initial choice was incorrect and the reasoning behind the correct answer.
- Familiarize Yourself with Common Traps: Many multiple selection questions are designed to include distractors, which are wrong options that seem plausible. Being aware of these traps will help you avoid making mistakes.
By following these strategies and regularly practicing under test conditions, you’ll improve your chances of performing well in the multiple selection section of the exam. A focused and strategic approach will allow you to maximize your potential and handle the questions efficiently.
How to Analyze Answer Choices
One of the most important skills when taking an exam with multiple options is knowing how to evaluate the possible solutions. This process goes beyond simply choosing the first answer that seems correct. Instead, it involves carefully analyzing each option to ensure that you make the right selection. Below are some techniques to improve your ability to assess answer choices effectively.
Key Steps in Answer Evaluation
- Identify the Question Type: Understand what the question is asking before analyzing the options. Whether it requires a direct calculation, conceptual reasoning, or pattern recognition, recognizing the type of question helps you approach it with the right mindset.
- Eliminate Clearly Incorrect Options: Often, there will be one or two options that are obviously wrong. Removing these can immediately narrow down your choices, giving you a higher probability of selecting the correct answer.
- Check for Extremes in Options: Watch out for answers that contain extreme values or radical statements. In many cases, the correct answer will be more moderate or balanced, whereas extreme values can be a red flag.
- Use Logical Deduction: Apply logical reasoning to the remaining options. Sometimes, one or two options may appear plausible but have minor flaws that you can spot through reasoning or estimation.
Advanced Techniques for Answer Analysis
- Look for Subtle Differences: Some answer choices may be very similar but differ in small details. Pay close attention to these differences, as they often indicate nuances in the question that can help you identify the correct answer.
- Cross-Check with Key Concepts: Reflect on key principles or formulas that you have learned. Does the selected answer align with what you know to be true? Verifying this alignment can help reinforce your choice.
- Use the Process of Elimination: If you’re unsure, focus on eliminating options that you know are wrong first. With fewer choices remaining, you can make a more informed decision, even if you have to guess.
By incorporating these strategies into your approach, you’ll be better equipped to navigate complex questions and make well-informed choices. Analyzing answer options critically is a skill that improves with practice, so regularly applying these techniques will boost your confidence and performance on the exam.
Utilizing AP Review Resources
When preparing for an advanced placement exam, having access to the right study materials is essential. Review resources can provide structured guidance, practice problems, and explanations that enhance your understanding of key concepts. These resources, whether online tools, textbooks, or practice exams, can significantly boost your chances of success. It’s important to know how to use these materials effectively in order to maximize your preparation efforts.
Start by identifying the resources that best fit your learning style. Some may prefer online tutorials or video explanations, while others may benefit from printed practice questions or comprehensive study guides. A balanced mix of these resources is often the most effective way to approach the preparation process. Regular practice, along with consistent review of key concepts, will help solidify your knowledge and build confidence as the exam day approaches.
Additionally, some review resources offer explanations for common mistakes and strategies for avoiding pitfalls. These insights can be invaluable, as they allow you to learn from errors without repeating them. By utilizing a variety of review tools, you can ensure a well-rounded and thorough preparation experience.
What to Expect on Exam Day
On exam day, it’s important to be well-prepared both mentally and physically. The testing environment is designed to assess your understanding of key concepts, so familiarity with the process and knowing what to expect can help reduce anxiety and improve your performance. Being aware of the exam format, timing, and rules will allow you to approach the day with confidence and clarity.
Arrival and Setup
When you arrive at the exam location, make sure to arrive early enough to find your seat, settle in, and check that you have all necessary materials, such as pens, pencils, a calculator (if allowed), and your student ID. Once you are seated, the exam proctors will give instructions on how the test will proceed and address any questions about the process. It’s a good idea to stay calm and focused at this stage so you can begin the test without any distractions.
Exam Structure
The exam will be divided into different sections, each designed to assess specific areas of knowledge. Typically, you will face a combination of problem-solving tasks, concept-based questions, and applied scenarios. It’s crucial to manage your time wisely and keep track of how long you have left to complete each section. If you find a question challenging, don’t get stuck–move on and come back to it later if time permits.
Throughout the exam, remain focused, read each question carefully, and follow all instructions. Take advantage of any allotted breaks, as they can help refresh your mind and give you a moment to re-energize before continuing with the next set of questions.
Improving Your Problem-Solving Skills
Developing strong problem-solving abilities is crucial for success in any challenging exam, especially those focused on mathematical and analytical concepts. The key to mastering such questions lies in practice, strategy, and understanding the core principles behind the problems. Improving your ability to approach problems systematically and efficiently will not only help you perform better but also build confidence during your exam.
Understand the Fundamentals
Before diving into complex problems, make sure you have a solid grasp of the foundational concepts. This includes understanding essential mathematical principles, theorems, and functions that are frequently tested. Review key topics and focus on how these concepts relate to one another. With a strong foundation, you’ll be able to identify patterns and quickly recognize the type of problem you’re dealing with.
Practice Problem-Solving Techniques
One of the most effective ways to improve your skills is through consistent practice. Work through problems from various sources to expose yourself to a variety of question types. As you practice, aim to:
- Break down the problem: Identify key information, draw diagrams, and organize your thoughts before attempting to solve.
- Use a step-by-step approach: Don’t rush through the process. Solve problems methodically, taking time to understand each step.
- Check your work: After solving a problem, double-check your solution for accuracy and correctness.
By practicing these techniques, you’ll be able to refine your approach, build problem-solving strategies, and improve your performance over time.