
In this section, we will explore a key component of a challenging academic assessment that tests fundamental mathematical skills. By analyzing a set of questions from a past evaluation, you will gain insight into common problem-solving techniques and strategies. This detailed review helps to strengthen your approach to tackling similar tasks, preparing you for future success in similar tests.
Through careful examination of the problems presented in the past assessment, students can identify patterns and methods that are frequently tested. This analysis not only boosts problem-solving confidence but also enhances critical thinking abilities. Focus on the reasoning behind each solution rather than just memorizing formulas for a deeper understanding of the concepts being tested.
We will break down the various sections to highlight the areas that often present challenges. By familiarizing yourself with these question types, you can improve your performance in upcoming evaluations. Mastering these techniques is essential for anyone looking to excel in advanced mathematical assessments.
AP Calculus AB 2012 Exam Overview
This section provides a comprehensive summary of a significant evaluation aimed at testing advanced mathematical concepts. It is designed to assess students’ ability to solve complex problems, apply theoretical knowledge, and interpret data effectively. The assessment consists of various sections that challenge both theoretical understanding and practical application.
The structure of this evaluation is divided into multiple segments, each focusing on different aspects of mathematical reasoning. Students are expected to demonstrate proficiency in understanding functions, derivatives, integrals, and other key areas that are essential for mastering higher-level mathematics. Each segment tests both conceptual knowledge and the ability to apply this knowledge to solve specific problems.
In this overview, we will look at the key areas covered by the assessment, offering insight into the topics that are frequently tested. By understanding the format and expectations, students can better prepare and approach each section with confidence and clarity.
Exam Format and Structure
The assessment is designed to test a student’s ability to apply mathematical principles across a variety of problem types. It is divided into distinct sections that challenge both theoretical understanding and practical application. Each part of the evaluation focuses on specific skills, from problem-solving to critical thinking, ensuring a comprehensive measure of the student’s capabilities.
The structure includes a combination of question types that require different approaches, ranging from straightforward calculations to more complex scenarios. These sections are designed to assess both speed and accuracy, as well as the depth of knowledge required to solve each problem. The format encourages careful analysis and strategic thinking to ensure success in each segment.
Understanding the layout of this assessment allows students to approach it with greater confidence. With careful preparation, it is possible to navigate through each section efficiently, making the most of the time available to answer each question thoroughly and correctly.
Understanding Multiple Choice Questions
The assessment includes questions that are structured to test a range of mathematical skills in a format that offers several possible solutions. These questions require a careful reading of each option and a methodical approach to identifying the correct answer. The key to success lies in recognizing patterns, understanding the concepts being tested, and applying the appropriate techniques to narrow down the choices.
By understanding the typical structure of these questions, students can approach them with confidence. It is important to read all options thoroughly before selecting an answer, as some may contain subtle differences that can lead to errors if not carefully considered.
| Question Type | Skills Tested | Approach |
|---|---|---|
| Conceptual Questions | Understanding core principles | Identify the fundamental concept and test each option accordingly |
| Calculation Problems | Mathematical accuracy and precision | Perform calculations and compare results with the options |
| Application Questions | Real-world problem solving | Apply theory to practical situations and check consistency |
When approaching these questions, it is important to focus on accuracy and speed, as both are critical in successfully navigating this section. With practice, students can improve their ability to quickly evaluate each option and select the correct one with confidence.
Key Concepts Tested in 2012
The assessment evaluates several core mathematical principles, focusing on topics that are crucial for understanding advanced concepts. Students are required to apply theoretical knowledge to solve problems, demonstrating their ability to reason through complex scenarios. The following sections highlight the primary areas covered in the test, emphasizing their importance in mastering the subject.
Fundamental Theorems and Properties
A significant portion of the assessment involves understanding and applying key theorems and properties that govern mathematical functions. These include the concepts of limits, derivatives, and integrals, which form the foundation for solving a wide variety of problems. The ability to manipulate and interpret these principles is essential for success in more advanced topics.
Problem-Solving Techniques
Another important aspect tested is the ability to solve practical problems using established techniques. This involves not just applying formulas, but also analyzing each problem’s structure and finding the most efficient method for solving it. Whether it’s through graphing, algebraic manipulation, or other strategies, students must show their capability to approach and solve problems systematically.
Common Mistakes to Avoid
When tackling complex mathematical problems, it’s easy to make errors that can significantly affect performance. These mistakes often stem from rushing through the questions, misinterpreting instructions, or overlooking key details. Understanding and avoiding these common pitfalls can greatly improve accuracy and efficiency during the assessment.
Rushing Through Questions
One of the most frequent mistakes is moving too quickly through the questions. In the haste to complete the assessment, students may overlook important information or fail to carefully read all options. Taking the time to thoroughly analyze each question and verify the chosen solution can prevent costly errors.
Misapplying Formulas and Theorems
Another common mistake is using the wrong formula or incorrectly applying mathematical principles. While memorizing formulas is important, it is equally essential to understand when and how to use them. Misapplication of concepts can lead to incorrect results, so it is crucial to carefully consider the appropriate methods for each type of problem.
How to Approach the Exam

Preparing for a challenging test requires a strategic approach to ensure success. It’s important to stay focused, manage time wisely, and carefully evaluate each section. Having a clear strategy can help reduce anxiety and improve performance. Here are some effective steps to follow when approaching this type of assessment:
- Read Instructions Carefully: Make sure to understand the specific requirements of each question before attempting to answer.
- Manage Time Efficiently: Allocate a specific amount of time for each section to avoid rushing towards the end.
- Stay Calm and Focused: Keep a clear mind throughout the test, and don’t let difficult questions throw you off.
- Check Your Work: If time allows, revisit your answers to catch any potential mistakes.
By following these strategies, students can approach the test methodically, minimizing the risk of errors and optimizing their performance.
Time Management Tips for Success
Effective time management is crucial for performing well under pressure. Without a clear plan, it’s easy to waste valuable time on easier sections or become overwhelmed by more challenging problems. The key is balancing speed with accuracy to ensure each section is completed efficiently. Here are some tips to help manage time effectively during the assessment:
Prioritize and Plan

Start by assessing the difficulty of each section and allocate time accordingly. It’s important to tackle the questions you’re most confident about first to build momentum. Use the following strategies:
- Assess the sections: Quickly skim through the test to understand the layout and identify any particularly challenging areas.
- Set time limits: Divide the available time among the sections based on their complexity, leaving some buffer time for review.
- Use time wisely: Don’t dwell too long on a single question. Move on and come back to it later if needed.
Stay Calm and Focused
Maintaining a steady pace is crucial. If you find yourself struggling with a particular question, don’t panic. Instead, stay calm, mark the question, and continue with the rest of the test. Here are some ways to stay focused:
- Avoid distractions: Focus entirely on the test, and try not to think about anything else.
- Keep an eye on the clock: Regularly check the time to ensure you’re on track and adjust your pace if necessary.
By prioritizing tasks and managing your time wisely, you can ensure a balanced approach and maximize your chances of success.
Effective Study Strategies for AP Calculus
Studying for a rigorous subject requires a well-structured approach that helps retain key concepts and strengthens problem-solving skills. A combination of active learning, consistent practice, and time management will ensure that you’re fully prepared. Here are some effective strategies to help you succeed:
1. Start Early and Plan Ahead: Begin your preparation well in advance of the test to avoid last-minute cramming. Creating a study schedule allows you to allocate enough time to cover all topics without feeling rushed.
2. Focus on Conceptual Understanding: Rather than memorizing formulas, focus on understanding the core principles behind them. Knowing how and why certain techniques work will help you apply them to different types of problems.
3. Practice Regularly: The more problems you solve, the more comfortable you become with the types of questions you’ll encounter. Use a variety of practice materials to expose yourself to different scenarios and improve your ability to tackle complex problems.
4. Work with Study Groups: Collaborating with classmates can provide new insights and alternative methods of solving problems. Explaining concepts to others reinforces your own understanding and helps clarify any areas of confusion.
5. Take Breaks and Stay Healthy: Avoid burnout by taking regular breaks during study sessions. Make sure to get plenty of rest, eat well, and stay hydrated to maintain focus and energy during your preparation.
By applying these strategies, you can ensure thorough preparation and increase your chances of success in the subject.
Reviewing the 2012 Multiple Choice Answers
Analyzing past assessment questions is an essential strategy for improving problem-solving skills. By reviewing previous tests, you can identify patterns, understand common challenges, and gain a deeper insight into the types of questions typically asked. This review process helps build familiarity with the format and reinforces your understanding of key concepts.
Key Areas to Focus On
- Problem Types: Pay attention to the different types of questions that were featured. Understanding the structure of these questions will help you recognize similar problems in future assessments.
- Common Mistakes: Identify mistakes made during the review and learn from them. Recognizing where errors occurred will help you avoid them in future practice.
- Concepts Tested: Review the main concepts highlighted in the questions. Ensure that you fully grasp the underlying principles and how they are applied to solve problems.
Tips for Effective Review
- Revisit Incorrect Questions: Focus on the questions you answered incorrectly. Understanding why the correct answer is right and why your answer was wrong can help clarify any misconceptions.
- Track Progress: Keep track of your improvement by regularly reviewing your performance. This helps to gauge which areas need further attention.
- Practice Under Test Conditions: Simulate test conditions when reviewing. Time yourself and try to answer questions without any external help to better prepare for real test scenarios.
By systematically reviewing and analyzing the questions, you will enhance your skills and be better equipped to handle similar problems in the future.
Breaking Down Each Question Set
Understanding the structure and logic behind different question groups is crucial for mastering complex topics. By analyzing each section of a test, you can learn to identify the core focus areas, recognize recurring themes, and develop efficient strategies for tackling similar problems. This approach not only improves performance but also boosts confidence in answering various types of questions.
Identifying Key Themes
Each set of questions typically revolves around specific concepts or principles. By focusing on the key themes, you can streamline your preparation and ensure that you are well-versed in the most important areas. Pay close attention to the patterns and topics that tend to appear most frequently across different sets, as they are often the focal point of assessments.
Analyzing Question Types and Formats
Questions within each set may vary in format, ranging from problem-solving to conceptual understanding. It’s important to understand how each question type tests different skills. For example, some questions may test procedural knowledge, while others may assess your ability to apply theoretical concepts. By recognizing the distinct characteristics of each type, you can develop tailored strategies to answer them effectively.
Interpretation of Graphical Questions

Graphical questions require a different approach compared to traditional problem-solving questions. These types of problems often ask you to interpret and analyze visual data, which may include graphs, charts, or diagrams. Understanding how to extract relevant information from these visuals is key to answering such questions accurately and efficiently. The ability to interpret graphical representations not only tests your comprehension of the material but also your ability to connect visual data with theoretical concepts.
Key Steps in Interpreting Graphical Data
When faced with a graphical question, follow these essential steps:
- Identify the Axes: Always start by reviewing the axes to understand what each represents. The x-axis typically shows the independent variable, while the y-axis shows the dependent variable.
- Look for Key Points: Pay attention to any labeled points or critical values on the graph. These can provide insights into the function or relationship being depicted.
- Examine Trends and Patterns: Identify the overall trend of the graph. Is it increasing, decreasing, or constant? Understanding the general shape of the graph helps in predicting its behavior under different conditions.
- Focus on Key Features: Look for features such as slopes, intercepts, peaks, and valleys. These can indicate important points of interest and are often used to answer specific questions about the graph.
Example of Graph Interpretation

| Point | X-Coordinate | Y-Coordinate |
|---|---|---|
| Point A | 2 | 5 |
| Point B | 4 | 3 |
| Point C | 6 | 0 |
In this example, you can see three points plotted on the graph. By interpreting these points in relation to the overall trend, you can deduce information such as the slope of the graph between points or how the graph behaves in certain intervals.
By following these steps and practicing regularly, you will improve your ability to interpret graphical questions and make better-informed decisions when answering them.
Solving Problems Using Calculus Techniques
When tackling complex problems, applying appropriate mathematical methods is crucial for deriving accurate solutions. In many cases, these problems involve understanding and manipulating mathematical functions, identifying patterns, and using systematic approaches to find solutions. By leveraging various techniques, such as differentiation and integration, one can simplify and solve even the most challenging problems effectively. Mastery of these strategies enhances the ability to address a wide range of questions.
Step-by-Step Problem Solving Process
To solve problems efficiently, follow a structured approach:
- Analyze the Problem: Carefully read the problem to identify key information, such as variables, constants, and any relationships between them. Recognizing what the problem is asking for is the first step in finding a solution.
- Choose the Right Technique: Based on the problem’s requirements, select the most appropriate method. For example, if the problem involves rates of change, differentiation might be the most useful tool. If you’re dealing with areas or accumulated quantities, integration could be the key.
- Apply the Technique: Execute the chosen method step by step, ensuring that each calculation is correct and follows logical reasoning. Always double-check each stage of your work to avoid errors.
- Interpret the Results: Once the solution is obtained, interpret the result in the context of the problem. Ensure that the answer makes sense and answers the original question posed in the problem.
Common Techniques and Their Applications
Here are some of the most commonly used methods for solving mathematical problems:
- Derivatives: Useful for finding rates of change, slopes of curves, and optimization problems.
- Integrals: Essential for calculating areas under curves, accumulated quantities, and solving problems related to accumulation or total change.
- Limits: Helpful for understanding the behavior of functions as they approach specific values, particularly for continuity and convergence analysis.
By mastering these techniques and applying them correctly, you can approach a variety of mathematical problems with confidence and precision.
Practice Problems to Improve Skills
Enhancing problem-solving abilities requires consistent practice and the application of different strategies to a variety of scenarios. By regularly working through problems, you strengthen your understanding of key concepts and improve your ability to think critically and analytically. This approach not only reinforces theoretical knowledge but also builds confidence in tackling unfamiliar challenges.
Essential Problem Types to Master

Focusing on different types of problems will help you develop a well-rounded skill set. Here are some categories of problems that are particularly useful for strengthening your overall abilities:
- Optimization Problems: These problems require you to maximize or minimize a certain quantity, such as area, volume, or cost. They help hone your analytical skills and understanding of rates of change.
- Area and Volume Calculations: Problems involving integration are essential for mastering the process of calculating areas under curves or volumes of solids. This type of practice reinforces your understanding of geometric relationships.
- Rates of Change: Problems related to rates of change allow you to practice the application of derivatives in real-world contexts, like motion, growth, and decay.
- Approximation and Limits: By working on approximation problems, you learn how to estimate values and solve problems involving limits, essential for understanding function behavior.
Strategies for Effective Practice
When practicing problems, it’s important to use a systematic approach to maximize learning:
- Start with Simple Problems: Begin with easier problems to ensure you have a solid grasp of the fundamentals before moving on to more complex scenarios.
- Gradually Increase Difficulty: Once you’re comfortable with simpler problems, gradually work your way up to more difficult ones. This helps build problem-solving resilience and prepares you for more challenging questions.
- Time Yourself: Simulate real-time conditions by setting time limits when practicing. This will help you manage your time effectively and reduce pressure during real tests.
- Review Mistakes: After solving a problem, review your work and analyze any errors. Understanding why a particular approach didn’t work is a powerful learning tool.
Through dedicated practice and a focus on diverse problem types, you can significantly improve your skills and approach even the most complex challenges with ease and confidence.
Top Resources for Preparation
To effectively prepare for any challenging assessment, using the right study materials is crucial. The following resources will provide comprehensive coverage of topics, diverse problem sets, and practical strategies to enhance your understanding and problem-solving abilities. Utilizing a variety of tools can ensure that you are fully equipped for success.
Recommended Books and Guides

Books remain a timeless resource for structured learning and detailed explanations. These resources break down complex ideas and offer practice problems with step-by-step solutions:
- Study Guides: Comprehensive guides are designed specifically to cover key topics and provide extensive practice problems. Popular options include guides from publishers like Barron’s and Princeton Review.
- Problem-Solving Books: Books focused on solving practice problems help you hone your skills through repetition and various approaches. Books like “The Art of Problem Solving” are widely used.
- Reference Books: These books offer in-depth explanations of mathematical principles and formulas, often accompanied by examples to clarify complex concepts.
Online Platforms and Practice Websites
Technology has made it easier to access interactive study materials. Online platforms offer practice tests, tutorials, and video lessons, which can provide dynamic support for your preparation:
- Khan Academy: Known for its clear, concise video lessons, Khan Academy covers a wide range of topics with practice exercises to test your understanding.
- Quizlet: A great tool for reviewing terms and concepts with flashcards, quizzes, and practice tests designed by fellow students and teachers.
- College Board’s Official Resources: The official College Board website offers free practice questions, sample tests, and detailed scoring guides, making it an essential tool for test prep.
- AP Classroom: This official platform provides additional resources, including personalized progress tracking and practice exams designed to mimic real test conditions.
Study Groups and Tutors

Sometimes, collaboration can be the key to success. Joining a study group or working with a tutor can help clarify doubts and reinforce difficult concepts:
- Online Forums: Forums such as Reddit and StackExchange allow students to discuss concepts, ask questions, and share resources with peers.
- Private Tutors: If you prefer one-on-one instruction, hiring a tutor who specializes in the subject can provide personalized guidance to target specific weaknesses.
- Study Groups: Join or
Analyzing the Difficulty Level of 2012
Understanding the complexity of any assessment is essential for proper preparation. By breaking down the individual components and evaluating their relative difficulty, students can better strategize their study plans. This process involves assessing the range of topics, the depth of each question, and the types of skills required to successfully navigate them.
The challenges presented in this particular test varied widely, testing not only basic understanding but also the ability to apply more advanced concepts to solve intricate problems. The questions ranged from fundamental principles to more complex scenarios that required creative problem-solving techniques. Understanding the structure of the questions and the skill set needed to answer them can provide valuable insights into how to approach similar challenges in the future.
Complexity of Question Types
Different types of questions demand different levels of cognitive effort. Some questions required straightforward calculations and formula applications, while others involved higher-order thinking and problem-solving under time constraints. These questions tested the ability to quickly interpret information and make accurate decisions:
- Basic Conceptual Questions: These questions were designed to test foundational knowledge, such as interpreting basic functions or identifying properties of graphs. Although they were relatively simple, they were still crucial for setting a solid base for more difficult problems.
- Application-Based Questions: These required applying learned concepts to real-world scenarios, often demanding multi-step reasoning and precision in calculation.
- Advanced Problem-Solving Questions: These presented challenges that involved multiple concepts or required synthesizing information from different sections of the study material.
Time Management and Strategy
The time allocated for completing each section played a critical role in determining how difficult the test felt. Some questions were straightforward but required significant time to execute correctly, while others could be solved quickly with a precise understanding of the underlying principles:
- Time-Intensive Problems: Some of the problems were designed to be time-consuming, requiring careful consideration and multiple calculations. Proper time management was essential to avoid rushing through these sections.
- Quick Decision Questions: These required the ability to quickly identify patterns or use shortcuts, providing an opportunity for test-takers to save time while still answering correctly.
Overall, the difficulty level was a blend of routine questions and more intricate problems, with a focus on assessing both knowledge and the ability to think critically under pressure. By reviewing the different question types and their respective challenges, students can gain a better understanding of what to expect and how to prepare most effectively.
How Scoring Works on AP Exams
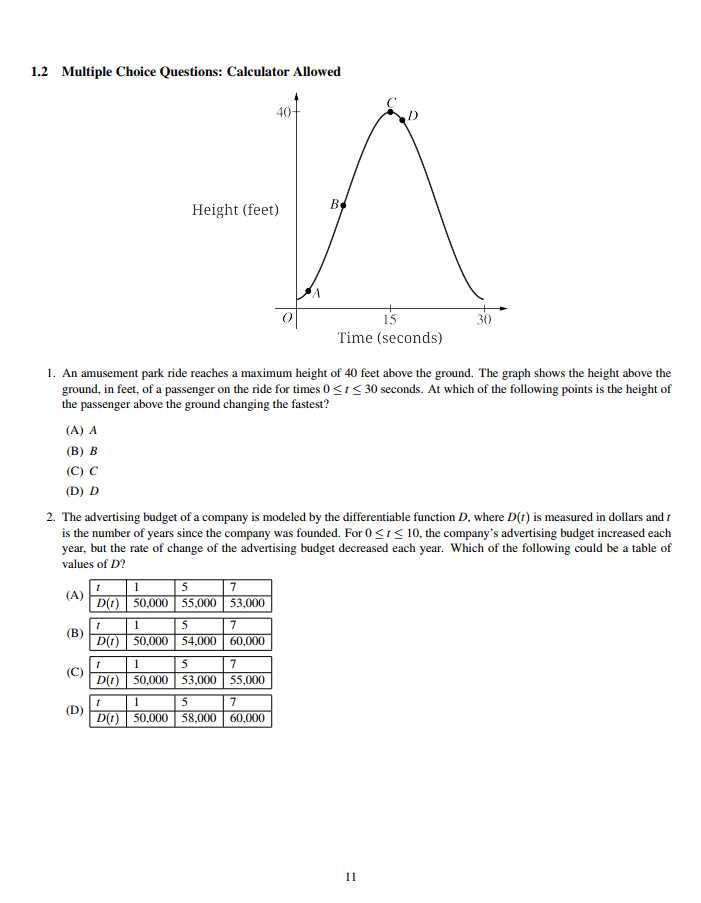
The process of scoring for advanced placement assessments is designed to evaluate both the correctness and the depth of a student’s understanding. Scoring is not merely about the number of correct responses but also takes into account how well the student applies concepts, solves problems, and demonstrates mastery of the subject matter. Understanding how the scoring system works can help students better prepare for the test and manage their expectations.
In general, scoring consists of two key components: raw scores and scaled scores. A raw score is the sum of the points earned for correctly answered questions, while the scaled score adjusts for various factors such as difficulty and testing conditions, ensuring that the score reflects a standardized level of achievement across all students. This scaling system is particularly important for maintaining fairness and consistency in grading across different years and test versions.
Raw Scores
Each correct response on the assessment contributes one point to the raw score. Incorrect answers do not result in penalties, so students are encouraged to answer every question, even if they are uncertain about the correct response. Additionally, some questions may have multiple components, where each part is weighted differently. For example, a question with several steps might award partial credit for correctly solving part of the problem, even if the final answer is incorrect. Understanding this aspect can help students approach questions methodically and maximize their scoring potential.
Scaled Scores
After calculating the raw score, it is converted into a scaled score to account for variations in difficulty across different testing sessions. This ensures that students are evaluated on an equal footing, regardless of when or where they take the assessment. The scaled score typically falls within a range that is used to determine a student’s proficiency level. For instance, a higher scaled score indicates a higher level of mastery over the material. While exact cutoffs may vary, scoring within a certain range may lead to college credit or advanced placement in future courses.
By understanding the mechanics of scoring, students can better focus their efforts on areas that will have the most impact on their overall performance. The system encourages both accuracy and strategic thinking, providing a balanced approach to evaluating knowledge and problem-solving skills. Students who are aware of how their scores are calculated can approach the assessment with confidence, knowing that their performance reflects their true capabilities.
Review of Student Performance on 2012 Assessment
The performance of students on a particular academic evaluation can provide valuable insights into both individual understanding and broader trends in the subject. By reviewing how students performed, we can identify common challenges, areas of strength, and overall effectiveness of the preparation methods. This analysis serves as a reflection of not just the students’ abilities, but also how well they were able to apply their knowledge under testing conditions.
Overall, the results from this evaluation showed a diverse range of performances. While many students demonstrated a strong grasp of key concepts, others struggled with more complex problem-solving questions that required deeper application of learned material. The data suggests that students who had a solid foundational understanding of core principles fared better, while those who lacked fluency in more advanced techniques faced greater difficulty.
Some common patterns of success were observed among students who effectively managed their time and approached the test strategically. These students tended to pace themselves and worked through problems methodically, ensuring that they addressed all questions without rushing. On the other hand, students who struggled with time management often left questions unanswered or gave incomplete responses, which impacted their overall performance.
In terms of specific topics, students excelled in areas involving basic concepts but faced more challenges with questions that required integrating multiple ideas or applying advanced reasoning. The results indicate that targeted preparation in these more complex areas could have a significant impact on overall performance. It also highlights the importance of practicing problem-solving skills that require multi-step thinking and the ability to connect different aspects of the material.
In summary, the review of student performance underscores the need for a balanced approach to preparation, with an emphasis on both foundational understanding and the development of more advanced problem-solving abilities. By focusing on these areas, future participants can improve their chances of achieving strong results and demonstrating a comprehensive mastery of the material.
Final Tips for Last-Minute Review
As the clock ticks down to the big assessment, many students find themselves scrambling for last-minute strategies to maximize their performance. While comprehensive study is ideal, a focused review in the final hours can still make a difference. The key lies in strategic preparation: reviewing key concepts, honing critical problem-solving skills, and maintaining a calm mindset.
Focus on Key Concepts
In the final hours, it’s important to review the most essential topics that are likely to appear. Instead of attempting to learn new material, focus on reinforcing what you already know. Go over key formulas, theorems, and principles that form the foundation of the subject. Flashcards can be an efficient way to quickly revisit critical information and solidify your understanding.
Practice with Purpose
Practice problems are one of the most effective ways to prepare in the final stages. Focus on problems that challenge your weaknesses or those that have been a consistent issue during your study sessions. Don’t waste time on questions you’ve already mastered, but instead, try to tackle more complex or unfamiliar problems. Doing so will increase your confidence and improve your ability to think critically under pressure.
While reviewing, try to simulate the test environment as closely as possible. Set a timer and work through problems without distractions to get used to the pressure of the clock. This will help you manage your time more effectively during the actual test.
Finally, don’t forget to take short breaks to refresh your mind. A clear head will help you think more clearly and avoid unnecessary mistakes. With the right mindset and approach, last-minute review can be a powerful tool to boost your chances for success.